Probabilistic aftershock hazard assessment for Jiuzhaigou MS7.0 earthquake in 2017
-
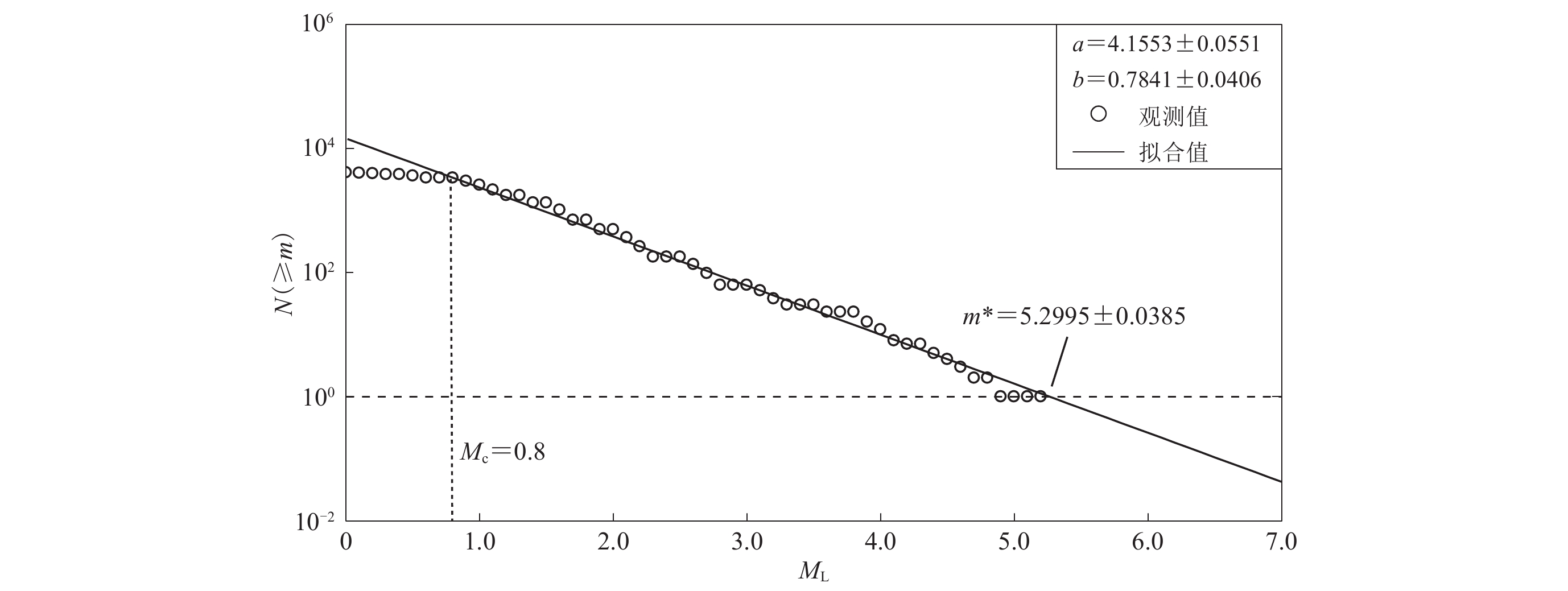
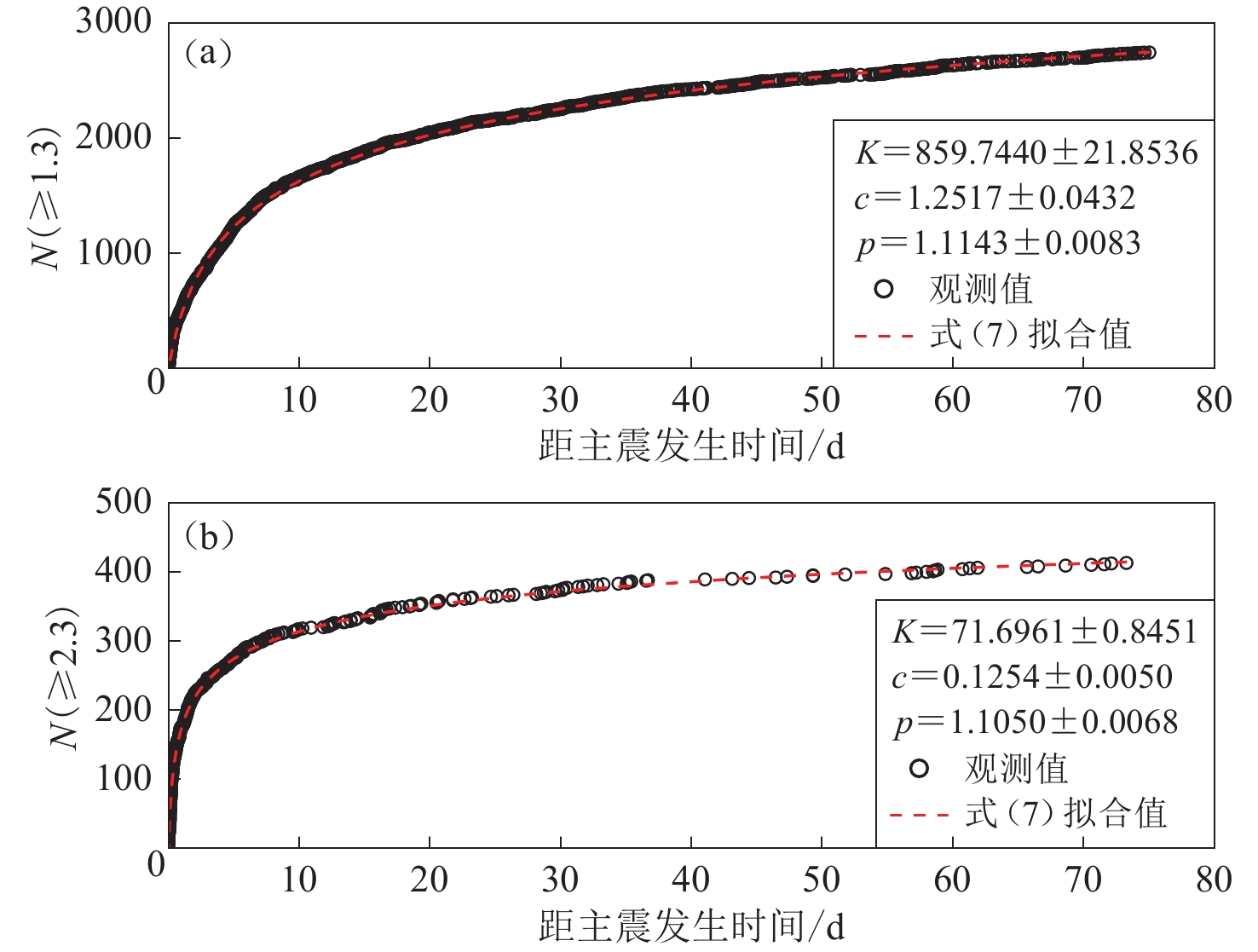
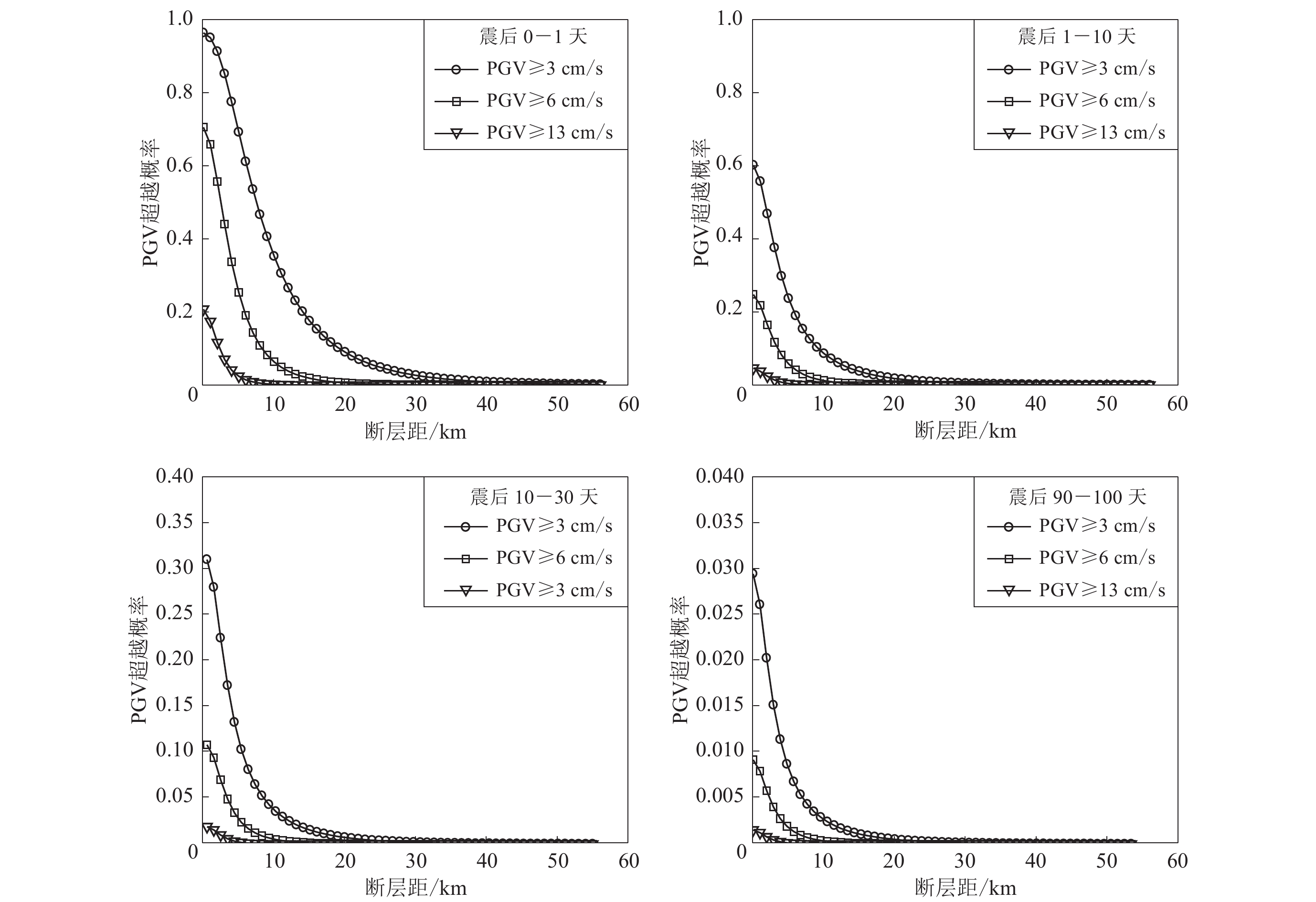
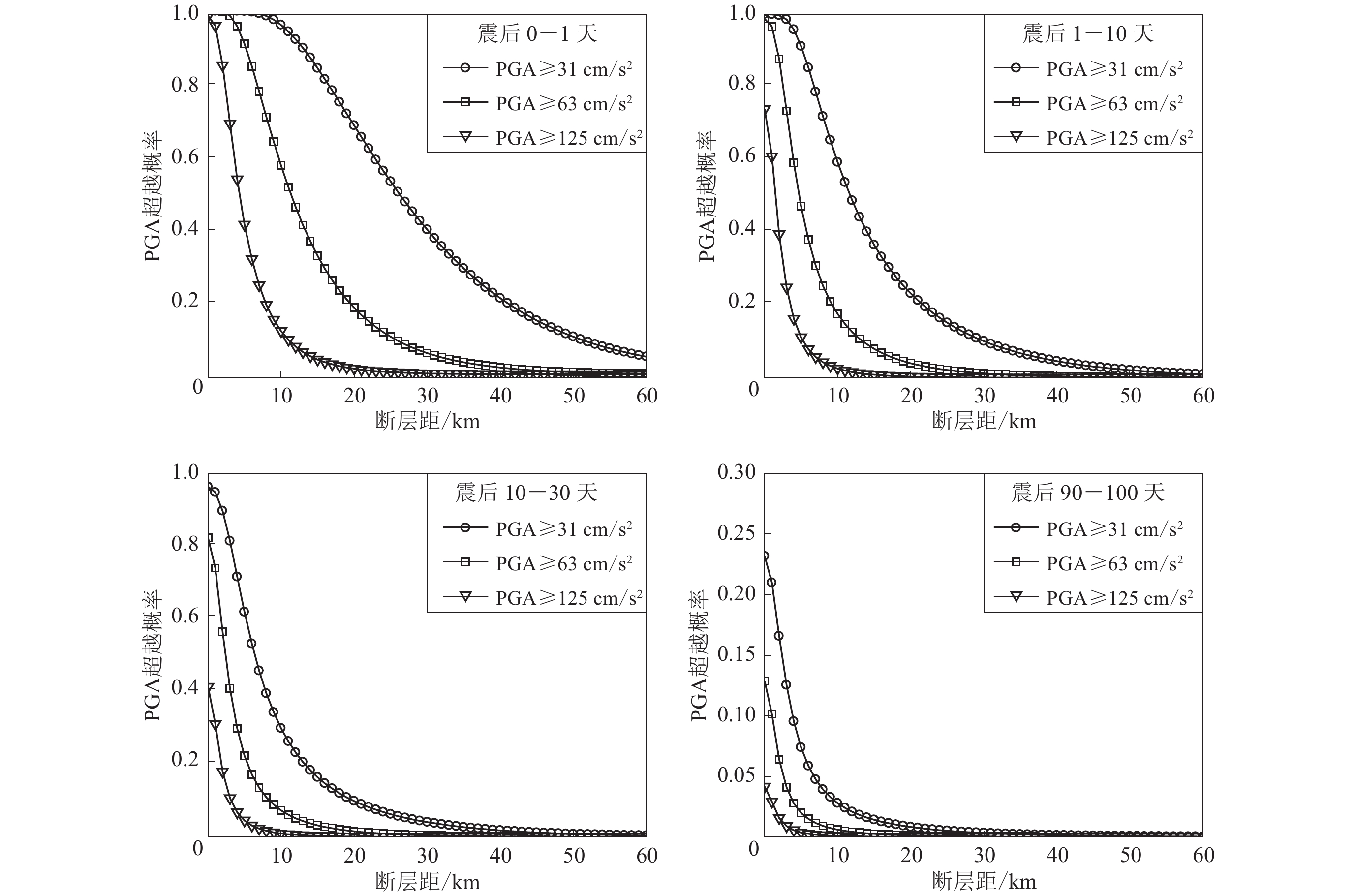
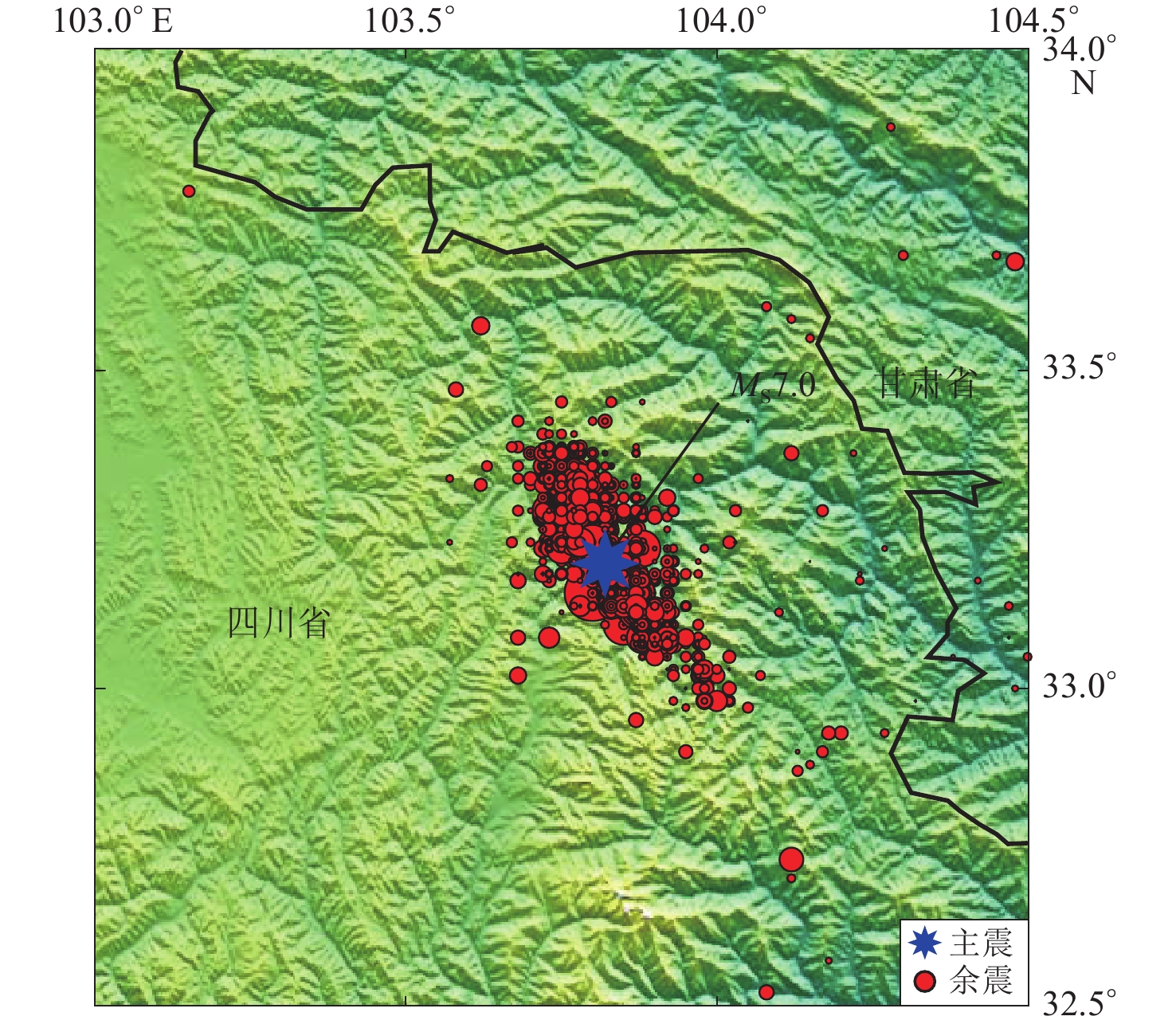
摘要: 本文介绍了余震危险性概率分析的概念，完整列出其相关公式的解析表达式并阐述了余震危险性概率分析的具体方法。以2017年九寨沟MS7.0地震为例，首先，求得该地震余震序列的相关参数，结果显示：九寨沟MS7.0地震余震序列理论最大余震震级约为ML5.3；b值约为0.784 1，明显低于中国西南地区同类型的其它地震，表明九寨沟地震余震区应力水平相对较高；p值约为1.109 7，明显高于中国西南地区同类型的其它地震，表明九寨沟地震余震序列随时间衰减较快。其次，计算此次地震余震与主震释放能量的比例关系，结果显示：在九寨沟MS7.0地震事件中，截止到10月22日约99.69%的能量为主震所释放，0.31%的能量为余震所释放。最后，利用九寨沟MS7.0余震序列参数结果并结合衰减关系，分别计算了主震后0—1天、1—10天、10—30天和90—100天内不同断层距内不同水平的峰值加速度和峰值速度的超越概率，结果显示：随着断层距增加，在相同时间间隔内超越概率的值呈下降趋势；在震后第一天内余震危险性最高，随着震后时间增加，相应的超越概率值呈现出明显下降趋势，表明九寨沟MS7.0地震余震危险性主要来自早期余震。本文的相关成果可以为震后地震危险性分析提供参考，并为短时间内应急救援及灾后重建提供辅助决策意见。Abstract: Probabilistic aftershock hazard assessment is important to assess the hazard of aftershocks. In this paper, we introduce the concept of probabilistic aftershock hazard assessment, and give the formulas related to the method of it.In response to the Jiuzhaigou MS7.0 event in Sichuan Province in 2017, firstly, we obtained the related parameters of the aftershock. The results show that the maximum aftershock magnitude of the given aftershock sequence is about ML5.3. The b value of Jiuzhaigou event is about 0.784 1, which is lower than the same type of earthquake in southwest China, indicating that the stress level of the aftershock area is relatively high. The p value is about 1.109 7, obviously higher than events of the same type, which shows that this aftershock sequence decays faster with time. Secondly, according to the parameters estimation, 99.69% of the energy was released by the mainshock and the rest by the aftershocks. Finally, combining the aftershock sequence parameter and the attenuation relationship, we calculated the probability of exceedance certain level of peak ground acceleration and peak ground velocity in 0−1 day, 1−10 days, 10−30 days and 90−100 days intervals within different fault distances after the mainshock. The results show: ① The value of exceedance probability decreases with fault distance in the same time interval; ② The risk of aftershocks is highest in the first day after the mainshock; the exceedance probability value exhibits a downward trend with the increase of time, indicating that the aftershock hazard of the JiuzhaigouMS7.0 event mainly comes from early aftershocks. These results provide reference for aftershock risk analysis, and auxiliary decision-making opinions for short time emergency rescue and post disaster reconstruction.
-
-
![]()
图 1 九寨沟MS7.0地震震中位置以及截至2017年10月22日的余震序列分布
Figure 1. Location of Jiuzhaigou MS7.0 earthquake and its aftershocks （ML≥0） distribution from the occurrence of the mainshock to October 22，2017
![]()
图 2 九寨沟MS7.0地震余震震级-频度图
Figure 2. Magnitude-frequency distribution of aftershocks of Jiuzhaigou MS7.0 earthquake
![]()
图 3 九寨沟地震余震累积数目随时间的变化曲线
Figure 3. Curve changes of cumulative aftershocks of Jiuzhaigou earthquake with time
![]()
图 5 不同时段内九寨沟MS7.0地震余震PGV超越概率变化示意图
Figure 5. Probabilities of exceeding PGV for aftershocks of Jiuzhaigou MS7.0 event during different periods
-
蒋长胜,庄建仓,吴忠良,毕金孟. 2017. 两种短期概率预测模型在2017年九寨沟7.0级地震中的应用和比较研究[J]. 地球物理学报,60(10):4132–4144 doi: 10.6038/cjg20171038 Jiang C S,Zhuang J C,Wu Z L,Bi J M. 2017. Application and comparison of two short-term probabilistic forecasting models for the 2017 Jiuzhaigou,Sichuan,MS7.0 earthquake[J]. Chinese Journal of Geophysics,60(10):4132–4144 (in Chinese) doi: 10.6038/cjg20171038
蒋海昆,郑建常,吴琼,曲延军,李永莉. 2007. 传染型余震序列模型震后早期参数特征及其地震学意义[J]. 地球物理学报,50(6):1778–1786 Jiang H K,Zheng J C,Wu Q,Qu Y J,Li Y L. 2007. Earlier statistical features of ETAS model parameters and their seismological meanings[J]. Chinese Journal of Geophysics,50(6):1778–1786 (in Chinese)
蒋海昆. 2010. 5·12汶川8.0级地震序列震后早期趋势判定及有关问题讨论[J]. 地球物理学进展,25(5):1528–1538 Jiang H K. 2010. Review of tendency judgement of the 5·12 Wenchuan M8 earthquake and discussion on some problems[J]. Progress in Geophysics,25(5):1528–1538 (in Chinese)
苏有锦,赵小艳. 2008. 全球8级地震序列特征研究[J]. 地震研究,31(4):308–316 Su Y J,Zhao X Y. 2008. Characteristics of global earthquake sequences with MW≥8.0[J]. Journal of Seismological Research,31(4):308–316 (in Chinese)
中国地震台网中心. 2017. 全国统一快报目录[EB/OL]. [2017-10-22]. http://www.csi.ac.cn/publish/main/813/5/index.html. China Earthquake Networks Center. 2017. National bulletin[EB/OL]. [2017-10-22].http://www.csi.ac.cn/publish/main/813/5/index.html （in Chinese）.
Båth M. 1965. Lateral inhomogeneities of the upper mantle[J]. Tectonophysics,2(6):483–514 doi: 10.1016/0040-1951(65)90003-X
Boore D M,Atkinson G M. 2008. Ground-motion prediction equations for the average horizontal component of PGA,PGV,and 5%-damped PSA at spectral periods between 0.01 s and 10.0 s[J]. Earthquake Spectra,24(1):99–138 doi: 10.1193/1.2830434
Campbell K W,Bozorgnia Y. 2008. NGA ground-motion model for the geometric mean horizontal component of PGA,PGV,PGD and 5% damped linear elastic response spectra for periods ranging from 0.01 to 10 s[J]. Earthquake Spectra,24(1):139–171 doi: 10.1193/1.2857546
Chiou B J,Youngs R R. 2008. An NGA model for the average horizontal component of peak ground motion and response spectra[J]. Earthquake Spectra,24(1):173–215
Console R,Lombardi A M,Murru M,Rhoades D. 2003. Båth’s law and the self-similarity of earthquakes[J]. J Geophys Res,108(B2):2128
Cornell C A. 1968. Engineering seismic risk analysis[J]. Bull Seismol Soc Am,58(5):1583–1606
Gallovič F,Brokešová J. 2008. Probabilistic aftershock hazard assessment I:Numerical testing of methodological features[J]. J Seismol,12(1):53–64 doi: 10.1007/s10950-007-9072-0
Gutenberg B,Richter C F. 1942. Earthquake magnitude,intensity,energy and acceleration[J]. Bull Seismol Soc Am,32(3):163–191
Hainzl S,Marsan D. 2008. Dependence of the Omori-Utsu law parameters on main shock magnitude:Observations and modeling[J]. J Geophys Res,113(B10):B10309 doi: 10.1029/2007JB005492
Hamdache M,Peláez J A,Kijko A,Smit A. 2017. Energetic and spatial characterization of seismicity in the Algeria-Morocco region[J]. Nat Hazards,86(S2):273–293 doi: 10.1007/s11069-016-2514-7
Helmstetter A,Sornette D. 2003. Båth’s law derived from the Gutenberg-Richter law and from aftershock properties[J]. Geophys Res Lett,30(20):2069
Kisslinger C,Jones L M. 1991. Properties of aftershocksequences in southern California[J]. J Geophys Res,96(B7):11947–11958 doi: 10.1029/91JB01200
Omori F. 1894. On after-shocks of earthquakes[J]. J Coll Sci Imp Univ Tokyo,7:111–200
Rodriguez-Marek A,Montalva G A,Cotton F,Bonilla F. 2011. Analysis of single-station standard deviation using the KiK-net data[J]. Bull Seismol Soc Am,101(3):1242–1258 doi: 10.1785/0120100252
Rodríguez-Pérez Q,Zúñiga F R. 2016. Båth’s law and its relation to the tectonic environment:A case study for earthquakes in Mexico[J]. Tectonophysics,687:66–77 doi: 10.1016/j.tecto.2016.09.007
Shcherbakov R,Turcotte D L. 2004. A modified form of Båth’s law[J]. Bull Seismol Soc Am,94(5):1968–1975 doi: 10.1785/012003162
Shcherbakov R,Turcotte D L,Rundle J B. 2004. Ageneralized Omori’s law for earthquake after shockdecay[J]. Geophys Res Lett,31(11):L11613 doi: 10.1029/2004GL019808
Shcherbakov R,Turcotte D L,Rundle J B. 2005. Aftershock statistics[J]. Pure Appl Geophys,162(6/7):1051–1076
Shcherbakov R,Goda K,Ivanian A,Atkinson G M. 2013. Aftershock statistics of major subduction earthquakes[J]. Bull Seismol Soc Am,103(6):3222–3234 doi: 10.1785/0120120337
Utsu T. 1961. A statistical study on the occurrence of aftershocks[J]. Geophys Mag,30:521–605
Utsu T,Ogata Y,Matsu'ura R S. 1995. The centenary of the Omori formula for a decay law of aftershock activity[J]. J Phys Earth,43(1):1–33 doi: 10.4294/jpe1952.43.1
Wiemer S,Katsumata K. 1999. Spatial variability of seismicity parameters in aftershock zones[J]. J Geophys Res,104(B6):13135–13151 doi: 10.1029/1999JB900032
Wiemer S. 2000. Introducing probabilistic aftershock hazard mapping[J]. Geophys Res Lett,27(20):3405–3408 doi: 10.1029/2000GL011479
Žalohar J. 2014. Explaining the physical origin of Båth's law[J]. J Struct Geol,60:30–45
下载:
计量
- 文章访问数: 1269
- HTML全文浏览量: 606
- PDF下载量: 61
