Influence of actual topography on the source dynamic rupture process and strong ground motion of the 2010 Yushu MS7.1 earthquake
-
摘要: 基于震源动力学模型，采用曲线网格有限差分法模拟了水平自由地表及实际地形条件下2010年玉树MS7.1地震的震源破裂过程及相应的地面地震动，获取了断层面上的破裂传播过程、最终滑移量分布及峰值地面速度分布，讨论了实际地形对玉树地震破裂过程及相应地震动的影响。基于本文设定的动力学模型，模拟结果显示：断层面上的高应力降是玉树地震出现超剪切破裂传播现象的主要原因；计算区域的实际地形阻碍了由自由地表引起的超剪切破裂的产生，对断层面滑移量的分布特征及滑动速率影响较大，进而在一定程度上降低了地震动峰值，同时对地震动的分布特征产生影响，且地震动平行断层面的水平分量相对受影响更大。Abstract: Based on the source dynamic models, the curved grid finite-difference method was implemented to simulate the dynamic rupture process and the resultant ground motions of the 2010 MS7.1 Yushu earthquake with horizontal free surface and actual topography respectively. Then the rupture propagation, final slip distribution and distribution of peak ground velocity were obtained to investigate the effect of actual topography on the dynamic rupture process of the Yushu earthquake and the resultant strong ground motions. Based on our models, the results show that the super-shear rupture occurred during the rupture propagation process, which was mainly induced by high stress drop on fault plane. The actual topography has prevented the generation of super-shear induced by free surface and has a great influence on the final slip distribution and slip rate on fault plane, then will affect the characteristics of ground motions as well as reduce the peak values of ground motions to a certain extent. The fault-parallel component of ground motion is relatively more affected. The investigation about the fault rupture dynamics will help us to understand well about the source rupture process and explain the characteristics of its strong ground motions, which is of great significance to more reasonably predict the ground motions of possible destructive earthquakes in the future.
-
引言
2008年5月12日汶川MS8.0地震已经过去10年，大地震给社会造成的创伤仍然十分深刻。全球最典型的陆-陆碰撞产物—青藏高原，从新生代开始快速隆起，不但现代构造运动剧烈，而且伴随着频繁的强震活动（Molnar，Tapponnier，1975；Royden et al，1997 ；Taylor，Yin，2009）。从2001年昆仑山MS8.1地震到2008年汶川MS8.0地震，再到2015年尼泊尔MS8.1 （MW7.8）地震（Chen et al，2016 ；Elliott et al，2016 ），时隔14年发生了3次MS8.0强震，这些大地震均围绕着青藏高原及周边构造相继引发了强烈的地壳应力释放（Feldl，Bilham，2006）。
现今青藏高原及周边地区受到印度板块向北的持续推挤和碰撞，产生了明显的地表构造隆起、深部介质变形和物性变化，与这些现象关联的动力学过程和深部介质特征均为地球科学家们研究的热点（Royden et al，2008 ；滕吉文等，2015）。由于地壳界面变形和物质分布不均匀引起的地表重力异常，不断地记录着各个地质年代构造演化的进程，特别是布格（Bouguer）重力异常，常被作为研究地壳形变和构造演化的“化石”。因此，从现今青藏高原及周边的重力异常场入手，结合最新的地球物理观测资料，计算青藏高原及周边地区的物性参数特征和岩石层力学特点，以及结合地表已知活动构造现状，研究青藏高原隆升及构造运动特点均具有十分重要的意义（Wang et al，2008 ）。
布格重力异常通常作为基础地球物理场数据用于研究大地水准面以下地壳密度的不均匀体分布。不同深度场源的异常分量在形态和频率特征上具有一定的差异性，但由于场源异常通常叠加在一起，如果先验资料较少，则很难用于定量研究。本文以最新的Crust1.0地壳模型（Laske et al，2013 ）提供的莫霍界面深度为约束，反演得到了地壳密度的横向差异性特征。在此基础上，结合由重力异常场估算的岩石层有效弹性厚度，基于艾里均衡模型和弹性板均衡模型原理，对青藏高原隆升及变形机制进行了分析和讨论，最后结合跨青藏高原南北向的地震震中剖面，给出了一个典型的4阶段青藏高原隆升机制模型。
1. 青藏高原及周边重力异常特征
围绕青藏高原的地学问题的研究，特别是地震活动与构造运动方面的研究，是国内外研究人员关注的重点之一（张永谦等，2010）。如果将地震看作构造运动的一种表现形式，无疑当今的青藏高原及周缘地区是最活跃的新构造运动区域。图1给出了1900年以来的MS≥6.0地震活动。以2001年昆仑山MS8.1地震为标志，从某种程度上可以认为该区域正处于新的地震活跃期或新的活跃幕开始。这些地震的发生除了与板块周边的动力源相关，还与震区周围的物性结构及力学特征密切相关。
![]()
图 1 青藏高原周缘地区主要构造和强震活动 （图中的构造参考Taylor，Yin，2009）Figure 1. The tectonic background and seismicity in the Tibetan Plateau and its surrounding（The tectonics in this map is after Taylor，Yin，2009）重力场与重力异常是研究地壳内部结构和物质分布的基础地球物理场资料。艾里（Airy）最早发现在高山下部可能存在低密度的山根，并提出了地壳均衡原理（Watts，2001），该原理也是基于喜马拉雅山附近的重力观测数据而发现的。基于艾里均衡原理计算得到的均衡重力异常，常用于研究地震活动（Chen et al，2009 ）。近年来，随着卫星重力、地面重力等观测资料的不断丰富，美国国家地理空间情报局（National Geospatial-Intelligence Agency，简写为NGA）发布了EGM2008全球重力场模型（球谐系数高达2 160阶，空间分辨率约为5′×5′），该模型可提供波长约为10 km的重力异常信息（Pavlis et al，2008，2012）。本文基于EGM2008模型提供的自由空气（free-air）重力异常，通过地形改正、高度改正和均衡改正得到了如图2所示的布格重力异常和均衡重力异常。重力异常的计算采用FA2BOUG程序完成（Fernández et al，2008），图2中的重力异常经过100 km高斯低通滤波，得到区域重力异常。图2a所示的布格重力异常范围介于0— −570×10 −5 m/s2之间，异常最低值在青藏高原中部。艾里均衡重力异常（isostatic gravity anomaly）的计算结果如图2b所示（由于青藏高原地区地壳较厚，因此在该计算中地壳平均厚度采用45 km，相比Balmino等（2012）给出的WGM2012采用全球平均30 km地壳厚度计算得到的艾里均衡异常在整体趋势上基本相同，但是正值异常成份更丰富），其相比布格重力异常呈相对高值，异常范围介于90×10−5— −200×10 −5 m/s2之间，异常高值区主要集中在喜马拉雅造山带前缘地区，整个青藏高原内部均衡重力异常为负值，表明相对于地表隆起而言，高原内部大部分地区的地下存在更深的山根。
![]()
图 2 研究区布格重力异常 （a） 和艾里均衡重力异常 （b）Figure 2. The Bouguer （a） and isostatic （b） gravity anomaly in the studied area新生代以来，由于古特提斯洋的闭合，印度板块与欧亚板块碰撞形成了现在青藏高原的大规模地壳隆起区。高原内部由一系列缝合带和次级活动地块组成，地表观测到的大规模走滑断裂系统，近百年来强震频发，这说明该区域地质构造运动十分活跃（Royden et al，2008 ）。越来越多的最新研究结果认为，青藏高原及周边地区的地壳构造变形和均衡模式复杂，如果采用简单的艾里模型解释可能会过于简化（Banks et al，1977 ；McKenzie，1977；Jin et al，1994 ；Kaban et al，2004 ）。本文从重力异常角度，对比分析了青藏高原周缘造山带与大型走滑及逆冲断裂带周缘的重力异常特征和力学参数，为研究区域地壳均衡模式和识别潜在应力集中区提供地球物理学参考（陈石等，2013，2014）。
2. 弹性板均衡与有效弹性厚度
从青藏高原重力测量到地壳均衡理论的发现，艾里均衡模型和普拉特（Pratt）均衡模型作为最典型的两种地壳均衡模式已经成为经典。若不考虑地质构造运动的时间尺度和地壳的力学特性，最终地壳变形模式总可以认为是这两种均衡模式的复合体（图3a）。但若对比新生代以来的活动构造和古老的克拉通地块之间的重力异常特征，可以发现，处于不同地质构造演化时期的地壳变形具有显著的力学差异特征（Lowry，Smith，1995；Simons，van der Hilst，2002；McKenzie，2003；Audet，Mareschal，2004；Kirby，Swain，2006；Nair et al，2012 ）。这种情况下若采用弹性板均衡模型（图3b），则更适合描述处于不同构造演化时期的地壳变形特点。
![]()
图 3 复合均衡 （a） 和弹性板均衡 （b） 模型示意图ρc，ρm，Te分别为地壳密度，地幔密度和岩石圈有效弹性厚度（修改自Forsyth，1985）Figure 3. The sketch map of the combined （a） and flexural isostatic （b） modelρc，ρm，Te are the density of crust and mantle and the effective elastic thickness of lithosphere respectively （modified from Forsyth，1985）图3b所示的弹性板均衡模型中，通过岩石层有效弹性厚度Te来定量描述其力学特性（Forsyth，1985），即
$D {\text{＝}} \frac{{ET_{\rm{e}}^3}}{{12\left({1 - {\sigma ^2}} \right)}}, $
(1) 式中，E为杨氏模量，D为弹性板刚度，σ为泊松比。
基于重力异常和地形可以定量地计算Te的空间分布。Jordan和Watts （2005）给出了青藏高原及周边地区的弹性板厚度，如图4所示。有效弹性厚度高值区从力学上可解释为因地壳或岩石层不易变形，因此其具有较强的能力承载地表载荷的压力，反之则为易变形区域。从地质构造的时间演化进程来看，对于新构造，由于地壳均衡变形尚未完成，虽然有山但是还没产生对应的根，因此Te值看起来会很大。但是如果水平向的构造应力逐渐消失，垂直向的地形载荷逐渐成为主要的地壳变形力源，且地壳完成均衡调整，达到类似艾里均衡这种理想状态，则整个弹性板的Te值看起来会很小。
从图4所示的弹性板厚度分布可以看出，对于汶川地震和尼泊尔地震这两次逆冲型地震，其震中位置均处在Te高低值的过渡区域，震中两侧的力学差异特性为地震应变能的积累提供了非常合适的构造环境，而昆仑山走滑型地震周边的Te值差异性则不是十分明显。
![]()
图 4 研究区弹性板厚度Te的特征分布 （引自Jordan，Watts，2005）Figure 4. The elastic thickness Te map derived by the flexural isostatic model in the studied area （after Jordan，Watts，2005）本文通过进一步对比图1给出的1900年以来多次地震震中位置的震源深度、地壳厚度和弹性板厚度三者之间的关系，给出了图5所示的统计结果。图5a显示地震震中主要分布在40 km以内；图5b表明地震发生率随弹性板厚度的减小而减小，当Te值介于5—30 km范围时为主要的地震集中区；图5c表明青藏高原及周边地震震中位置的地壳平均厚度集中在33—55 km范围内。
![]()
图 5 地震活动 （a）、弹性板厚Te （b）和地壳厚度 （c） 直方图Figure 5. Histograms of the seismicity （a），elastic thickness Te （b） and crustal thickness （c）一个强震的孕育和发生过程是极其复杂的，但如果从地壳或岩石层变形的力学角度去评估一个强震的孕育环境，从物理上是很容易理解的，而地表变形和布格重力异常作为地壳变形最直接的结果，两者均可以测定。图5给出的青藏高原及周边地区震中位置的定量统计结果，可以为判断潜在强震危险区提供与地壳力学性质有关的定量参考。
3. 地壳横向密度变化与变形特征
莫霍面作为最典型的地震波速度间断面，常作为地壳与地幔的分界面，通过地震学观测可以给出莫霍面的深度分布。而莫霍面也是一个最重要的密度差异性界面，因其两侧起伏变化的密度差异可以产生明显的布格重力异常。Parker （1973）给出了计算密度界面的频率域快速计算方法，该方法将重力位场的积分核函数在频率域内通过泰勒公式展开，由此建立的重力异常谱与不同阶次起伏密度界面谱之间的级数关系为
${{F}}\left[{\Delta g} \right] {\text{＝}} 2{\rm{\pi G}}{{\rm e}^{ - \omega {h_0}}}\mathop \sum \limits_{n {\text{＝}} 1}^\infty \frac{{{{\left({ - \omega } \right)}^{n - 1}}}}{{n!}}{{F}}\left[{\Delta \rho \Delta {h^n}} \right], $
(2) This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
${{F}}\left[{\Delta g} \right] {\text{＝}} 2{{\pi }}{\rm G}{{F}}\left[{\Delta \rho } \right]*\left({{{\rm e}^{ - \omega {h_0}}}\mathop \sum \limits_{n {\text{＝}} 1}^\infty \frac{{{{\left({ - \omega } \right)}^{n - 1}}}}{{n!}}{{F}}\left[{\Delta {h^n}} \right]} \right).$
(3) This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
$\Delta \rho {\text{＝}} \Delta \rho \left({x, y} \right) {\text{＝}} \frac{{\Delta g\left({x, y} \right)}}{{2\pi {\rm{G}} {{F}^{ - 1}}\left[{{{\rm e}^{- \omega {h_0}}}\mathop \sum \limits_{n {\text{＝}} 1}^\infty \displaystyle\frac{{{{\left({- \omega } \right)}^{n- 1}}}}{{n!}}{{F}}\left[{\Delta {h^n}} \right]} \right]}}.$
(4) 通常，根据起伏密度界面的深度计算重力异常称为正演问题，而由重力异常计算起伏密度界面的深度或横向密度变化称为反演问题。本文采用图2a给出的布格重力异常Δg和Crust1.0给出的莫霍面起伏Δh，带入式（4）计算得到青藏高原及其周边的地壳横向密度变化（图6）。从图6中可以看出，青藏高原主体隆升变形区的地壳相对周边呈现低密度特性。与图2a所示的布格重力异常特征整体上较一致，但细节上还存在明显差异，比如塔里木盆地东南的低密度区域等。
![]()
图 6 基于Crust1.0模型计算得到的复合均衡密度异常分布Figure 6. The density anomaly distribution derived from the combined isostatic model with Crust1.0 model图7为沿尼泊尔地震震中位置南北向横跨青藏高原区域的一条剖面，其详细给出了自由空气重力异常、艾里均衡重力异常、布格重力异常、地壳平均横向密度、弹性板厚度、地形、地壳界面起伏等地壳变形几何和物理属性特征。
![]()
图 7 青藏高原南北向地壳结构、有效弹性厚度Te与重力异常特征Figure 7. The crustal structure，elastic thickness Te and gravity anomaly across the Tibetan Plateau along with south-north direction![]()
图 8 复合均衡系统下的青藏高原地壳垂向变形机制Figure 8. The vertical deformation of the Tibetan Plateau controlled by the combined isostatic system通过综合分析图7给出的地壳几何变形和物性特征，给出了图8所示的青藏高原隆升与地壳均衡模式图，并结合印度板块和欧亚板块的陆陆碰撞变形历史，给出了青藏高原南北向均衡变形的4阶段模式图，图8d为现阶段地壳均衡模式。在青藏高原南部，由于印度板块的向北推挤引起大范围的垂直变形作用，地壳均衡模式更适合用弹性板模型来解释，而向北弹性板厚度Te逐渐减小（图7），地壳均衡模式呈现艾里和普拉特相结合的复合均衡特点。
4. 讨论与结论
本文依据EGM2008模型计算得到的布格重力异常，参考Crust1.0等地壳模型，系统地从地壳力学变形特征和密度变化等角度，通过统计等方法给出了强震震中区位置的地壳属性特征，并结合青藏高原变形过程给出了一种南北区域性差异的综合均衡解释模型。主要结论如下：
1） 青藏高原与周边块体之间的地壳平均密度（视密度）存在显著差异，尤其是印度板块与青藏高原碰撞带之间的密度差异最明显；
2） 青藏高原的重力均衡模式十分复杂，不能用简单的艾里或普拉特均衡模型来解释（张永谦等，2010），也不能简单地用弹性板均衡模型去解释，其均衡模式不但与水平向的推挤作用相关，还与高原的形成时间有一定联系，更多地显示出一种混合型的均衡特点；
3） 与青藏高原内部相比，其周缘地区不但密度差异性不明显，从力学强度上看差异性也不大，这可能是巨大地震多发生于青藏高原周缘地区的决定因素之一；
4） 基于现今观测到的青藏高原及周边的重力异常特征，依据地壳结构模型和均衡假说的拟合结果，得出青藏高原现今的变形模式应为分阶段进行，隆升应该从高原中心开始，隆升起来的地形由于重力均衡作用，又反作用于地壳引起其底部变形，产生山根，而印度板块和青藏高原的碰撞边界由于印度板块显示出的高密度刚性特征而具备一定的承载能力，并未由于隆起地形的均衡力产生显著的山根，而高原中心地区的地壳强度并不高，因此，由于重力均衡作用，地形持续下降，这可能也是现今青藏高原最高峰存在于碰撞边缘的原因之一。
本文以复合均衡模型为指导，得到的地壳视密度结果可以解释高原内部断块体系之间的差异，相比均衡异常结果，意义更明确。以弹性板挠曲理论为指导，得到的岩石层有效弹性厚度与强震的发生具有一定相关性，可以很好地解释青藏高原主断裂边界周围的地震活动。本研究得到的结论，对于未来强化青藏高原周边地震监测手段的布设具有一定的参考作用，可以为进一步解释高原内部块体间的相互作用机制和构建数值模型的物性选择提供合理的参考。
本文在研究过程中与中国地震局地球物理研究所李永华研究员、楼海研究员进行了多次有益的讨论，作者在此一并表示感谢。
-
![]()
图 1 模拟计算区域（矩形）及破裂起始点在地表的投影位置（红色圆点）示意
Figure 1. Location of the simulation area （rectangle frame） and surface projection of rupture initial point （red dot）
![]()
图 2 计算区域实际地形
红色圆点表示破裂起始点在地表的投影位置，红色实线表示断层迹线
Figure 2. Actual topography of computational area
The red dot indicates surface projection of the rupture initial point and the red line indicates the fault trace
![]()
图 3 断层面初始应力分布（图中数字表示不同应力分布区域的编号）
Figure 3. Distribution of initial stress on fault plane
The numbers in the fig. indicate the serial numbers of areas with different stress distribution
![]()
图 4 水平自由地表模型（模型Ⅰ，a）和实际地形模型（模型Ⅱ，b）中断层面上最终滑移量分布
Figure 4. Distribution of final slip on fault plane for horizontal free surface model （model Ⅰ ，a） and actual topography model （model Ⅱ ，b）
![]()
图 5 水平自由地表模型（模型Ⅰ，a）和实际地形模型（模型Ⅱ，b）中断层面上滑动速率快照
Figure 5. Snapshots of slip rate on fault plane for horizontal free surface model （model Ⅰ ，a） and actual topography model （model Ⅱ ，b）
![]()
图 6 水平自由地表模型（模型Ⅰ，a）和实际地形模型（模型Ⅱ，b）中破裂起始点深度上沿走向方向滑动速率随时空变化图像
Figure 6. Time-space plot of slip rate at the hypocentral depth along strike for horizontal free surface model （model Ⅰ ，a） and actual topography model （model Ⅱ ，b）
![]()
图 7 水平自由地表模型（模型Ⅰ，a）和实际地形模型（模型Ⅱ，b）中地面质点振动速度平行于断层面的水平分量快照
Figure 7. Snapshots of fault-parallel component of particle velocities for horizontal free surface model （model Ⅰ ，a） and actual topography model （model Ⅱ ，b）
![]()
图 8 水平自由地表模型（模型Ⅰ，左侧）和实际地形模型（模型Ⅱ，右侧）中峰值地面速度三分量分布
（a） 垂直于断层面的水平分量；（b） 平行于断层面的水平分量；（c） 垂向分量
Figure 8. Three components of peak ground velocity distribution for horizontal free surface model （model Ⅰ ，left） and actual topography model （model Ⅱ ，right）
（a） Fault-normal component；（b） Fault-parallel component；（c） Vertical component
![]()
图 9 均匀初始应力下水平自由地表模型（模型Ⅲ，a）和实际地形模型（模型Ⅳ，b）中断层面上滑动速率快照
Figure 9. Snapshots of slip rate on fault plane with uniform initial stress for horizontal free surface model （model Ⅲ ，a） and actual topography model （model Ⅳ ，b）
![]()
图 10 障碍体区域6的屈服应力降至5σu时水平自由地表模型（左侧）和实际地形模型（右侧）中断层面上最终滑动量分布
Figure 10. Distribution of final slip on fault plane for horizontal free surface model （left） and actual topography model （right） when the yield stress of the barrier region 6 drops to 5σu
![]()
图 11 水平自由地表模型（左侧）和实际地形模型（右侧）中断层面上破裂起始点深度上沿走向方向滑动速率随时空变化图像
Figure 11. Distribution of time-space of slip rate at the hypocentral depth along strike on fault plane for horizontal free surface model （left） and actual topography model （right）
表 1 断层面上各区域应力参数
Table 1 Stress parameters for the areas on fault plane
区域 初始剪切
应力$ {\sigma }_{0} $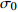 
剪切破裂
强度${\sigma }_{{\rm{u}}}$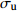 
临界滑动弱化
距离Dc/m1 1.01 1.0 0 2 0.60 1.0 0.15 3 0.96 1.5 0.15 4 0.72 1.2 0.15 5 0.20 16.0 40.0 6 0.20 16.0 40.0 7 0.20 16.0 40.0 断层其它区域 0.49 1.0 0.15 断层外 0.20 200.0 40.0 
下载: 导出CSV
-
胡峰. 2014. 非平面断层与等效平面断层的破裂动力学及辐射特征研究[D]. 合肥: 中国科学技术大学: 9–20. Hu F. 2014. Research on Nonplanar Fault and ESPFM of Dynamic Rupture and Radiation Pattern[D]. Hefei: University of Science and Technology of China: 9–20 （in Chinese）.
刘超,许力生,陈运泰. 2010. 2010年4月14日青海玉树地震快速矩张量解[J]. 地震学报,32(3):366–368. Liu C,Xu L S,Chen Y T. 2010. Quick moment tensor solution for 14 April 2010 Yushu,Qinghai,earthquake[J]. Acta Seismologica Sinica,32(3):366–368 (in Chinese).
王海云. 2010. 2010年4月14日玉树MS7.1地震加速度场预测[J]. 地球物理学报,53(10):2345–2354. Wang H Y. 2010. Prediction of acceleration field of the 14 April 2010 Yushu earthquake[J]. Chinese Journal of Geophysics,53(10):2345–2354 (in Chinese).
王铭锋. 2018. 震源动力学破裂过程影响因素及强地震动场特征的研究[D]. 北京: 中国科学院大学: 24–25. Wang M F. 2018. Study on the Influence of Different Factors on Earthquake Dynamic Rupture Process and Its Strong Ground Motions[D]. Beijing: University of Chinese Academy of Sciences: 24–25 （in Chinese）.
王铭锋,郑傲,于湘伟,章文波. 2018. 局部山体地形对断层动力学破裂过程的影响研究[J]. 地震学报,40(6):737–752. Wang M F,Zheng A,Yu X W,Zhang W B. 2018. Study on the influence of local mountainous topography to fault dynamic rupture[J]. Acta Seismologica Sinica,40(6):737–752 (in Chinese).
王洵,周云,孙蒙,王卫民. 2014. 青海玉树MW6.9级地震震源破裂过程[J]. 地质通报,33(4):517–523. Wang X,Zhou Y,Sun M,Wang W M. 2014. Rupture process of the 2010 MW6.9 Yushu earthquake in Qinghai Province[J]. Geological Bulletin of China,33(4):517–523 (in Chinese).
张丽芬. 2016. 不同因素对于断层动力学破裂过程复杂性的影响研究[D]. 北京: 中国地震局地球物理研究所: 104–114. Zhang L F. 2016. Influences of Different Factors on the Complexities of Fault Dynamic Rupture Propagation[D]. Beijing: Institute of Geophysics, China Earthquake Administration: 104–114 （in Chinese）.
张伟. 2006. 含起伏地形的三维非均匀介质中地震波传播的有限差分算法及其在强地面震动模拟中的应用[D]. 北京: 北京大学: 7–109. Zhang W. 2006. Finite Difference Seismic Wave Modelling in 3D Heterogeneous Media With Surface Topography and Its Implementation in Strong Ground Motion Study[D]. Beijing: Peking University: 7–109 （in Chinese）.
张勇,许力生,陈运泰. 2010. 2010年青海玉树地震震源过程[J]. 中国科学:地球科学,40(7):819–821. Zhang Y,Xu L S,Chen Y T. 2010. Source process of the 2010 Yushu,Qinghai,earthquake[J]. Science China Earth Sciences,53(9):1249–1251. doi: 10.1007/s11430-010-4045-5
周慧芳,张景发,胡乐银,罗毅. 2011. 青海玉树地震的InSAR数据同震形变场模拟与参数反演分析[J]. 地球信息科学学报,13(3):418–423. Zhou H F,Zhang J F,Hu L Y,Luo Y. 2011. Co-seismic deformation field and parameters inversion of the Yushu earthquake from InSAR[J]. Journal Geo-Information Science,13(3):418–423 (in Chinese). doi: 10.3724/SP.J.1047.2011.00418
朱守彪,袁杰,缪淼. 2017. 青海玉树地震(MS=7.1)产生超剪切破裂过程的动力学机制研究[J]. 地球物理学报,60(10):3832–3843. Zhu S B,Yuan J,Miao M. 2017. Dynamic mechanisms for supershear rupture processes of the Yushu earthquake (MS=7.1)[J]. Chinese Journal of Geophysics,60(10):3832–3843 (in Chinese).
Andrews D J. 1976. Rupture velocity of plane strain shear cracks[J]. J Geophys Res,81(32):5679–5687. doi: 10.1029/JB081i032p05679
Andrews D J. 2010. Ground motion hazard from supershear rupture[J]. Tectonophysics,493(3/4):216–221.
Ben-Menahem A,Shingh S J. 1987. Supershear accelerations and Mach-waves from a rupturing front. Part I. Theoretical model and implications[J]. J Phys Earth,35(5):347–365. doi: 10.4294/jpe1952.35.347
Burridge R. 1973. Admissible speeds for plane-strain self-similar shear cracks with friction but lacking cohesion[J]. J Geophys Res,35(4):439–455.
Dalguer L A,Day S M. 2007. Staggered-grid split-node method for spontaneous rupture simulation[J]. J Geophys Res,112(B2):B02302.
Day S M. 1982. Three-dimensional finite difference simulation of fault dynamics:Rectangular faults with fixed rupture velocity[J]. Bull Seismol Soc Am,72(3):705–727.
Ely G P,Day S M,Minster J B. 2010. Dynamic rupture models for the southern San Andreas fault[J]. Bull Seismol Soc Am,100(1):131–150. doi: 10.1785/0120090187
Harris R A,Barall M,Aagaard B,Ma S,Roten D,Olsen K,Duan B C,Liu D Y,Luo B,Bai K C,Ampuero J P,Kaneko Y,Gabriel A A,Duru K,Ulrich T,Wollherr S,Shi Z Q,Dunham E,Bydlon S,Zhang Z G,Chen X F,Somala S N,Pelties C,Tago J,Cruz-Atienza V M,Kozdon J,Daub E,Aslam K,Kase Y,Withers K,Dalguer L. 2018. A suite of exercises for verifying dynamic earthquake rupture codes[J]. Seismol Res Lett,89(3):1146–1162. doi: 10.1785/0220170222
Huang H Q,Zhang Z G,Chen X F. 2018. Investigation of topographical effects on rupture dynamics and resultant ground motions[J]. Geophys J Int,212(1):311–323. doi: 10.1093/gji/ggx425
Ida Y. 1972. Cohesive force across the tip of a longitudinal-shear crack and Griffith’s specific surface energy[J]. J Geophys Res,77(20):3796–3805. doi: 10.1029/JB077i020p03796
Kaneko Y,Lapusta N. 2010. Supershear transition due to a free surface in 3-D simulations of spontaneous dynamic rupture on vertical strike-slip faults[J]. Tectonophysics,493(3/4):272–284.
Kyriakopoulos C,Oglesby D D,Funning G J,Ryan K J. 2017. Dynamic rupture modeling of the M7.2 2010 EI Mayor-Cucapah earthquake:Comparison with a geodetic model[J]. J Geophys Res:Solid Earth,122(12):10263–10279. doi: 10.1002/2017JB014294
Li Z H,Elliott J R,Feng W P,Jackson J A,Parsons B E,Walters R J. 2011. The 2010 MW6.8 Yushu (Qinghai,China) earthquake:Constraints provided by InSAR and body wave seismology[J]. J Geophys Res,116(B10):B10302. doi: 10.1029/2011JB008358
Madariaga R. 1976. Dynamics of an expanding circular fault[J]. Bull Seismol Soc Am,66(3):639–666.
Oglesby D D,Day S M. 2001. Fault geometry and the dynamics of the 1999 Chi-Chi (Taiwan) earthquake[J]. Bull Seismol Soc Am,91(5):1099–1111.
Somerville P,Irikura K,Graves R,Sawda S,Wald D,Abrahamson N,Iwasaki Y,Kagawa T,Smith N,Kowada A. 1999. Characterizing crustal earthquake slip models for the prediction of strong ground motion[J]. Seismol Res Lett,70(1):59–80. doi: 10.1785/gssrl.70.1.59
USGS. 2014. M6.9: Southern Qinghai, China[EB/OL]. [2020-03-10]. https://earthquake.usgs.gov/earthquakes/eventpage/usp000hbbt/focal-mechanism.
Wang D,Mori J. 2012. The 2010 Qinghai,China,earthquake:A moderate earthquake with supershear rupture[J]. Bull Seismol Soc Am,102(1):301–308. doi: 10.1785/0120110034
Zhang W,Zhang Z G,Chen X F. 2012. Three-dimensional elastic wave numerical modelling in the presence of surface topography by a collocated-grid finite-difference method on curvilinear grids[J]. Geophys J Int,190(1):358–378. doi: 10.1111/j.1365-246X.2012.05472.x
Zhang W B,Iwata T,Irikura K. 2006. Dynamic simulation of a dipping fault using a three-dimensional finite difference method with nonuniform grid spacing[J]. J Geophys Res,111(B5):B05301.
Zhang W B,Iwata T,Irikura K. 2010. Dynamic simulation of the 1999 Chi-Chi,Taiwan,earthquake[J]. J Geophys Res,115(B4):B04305.
Zhang Z G,Zhang W,Chen X F. 2014. Three-dimensional curved grid finite-difference modelling for non-planar rupture dynamics[J]. Geophys J Int,199(2):860–879. doi: 10.1093/gji/ggu308
-
期刊类型引用(7)
1. 唐春呈，张仁鹏，焉恒琦，张帆，乔天罡. 双阳水管NS分量异常分析及其预报效能评估. 防灾减灾学报. 2024(02): 57-62 . 
百度学术
2. 苑争一，姜祥华，刘琦. 基于R值和统计分布检验的年度地震危险区个数评估. 中国地震. 2023(01): 88-97 . 百度学术
3. Jin Wei，Huining Huang，Ying Jiang，Ziwei Liu. Abnormal characteristics and effectiveness evaluation of the micro-seismic signal before the Debao M_S4.8 earthquake. Geodesy and Geodynamics. 2023(06): 605-613 . 必应学术
4. 关玉梅，王紫燕，杨锦玲，秦双龙. 华南地区定点形变预测指标建设. 华南地震. 2022(04): 34-40 . 百度学术
5. 杨红樱，于章棣，黄瑞滨，齐彬彬，裴东洋，王磊. 赤峰地震台定点形变2021年短期大幅变化分析. 地震地磁观测与研究. 2022(06): 159-165 . 百度学术
6. 苑争一，闫伟，牛安福，赵静，高歌. 定点形变破年变异常自动识别应用研究. 地震研究. 2020(02): 394-401 . 百度学术
7. 王婷，延军平，李双双，万佳，张玉凤. 帕米尔高原Mw≥6.6级地震时间韵律特征. 高原地震. 2020(04): 6-16 . 百度学术
其他类型引用(1)
计量
- 文章访问数: 843
- HTML全文浏览量: 333
- PDF下载量: 82
- 被引次数: 8
