Uncertainties in probabilistic tsunami hazard assessment
-
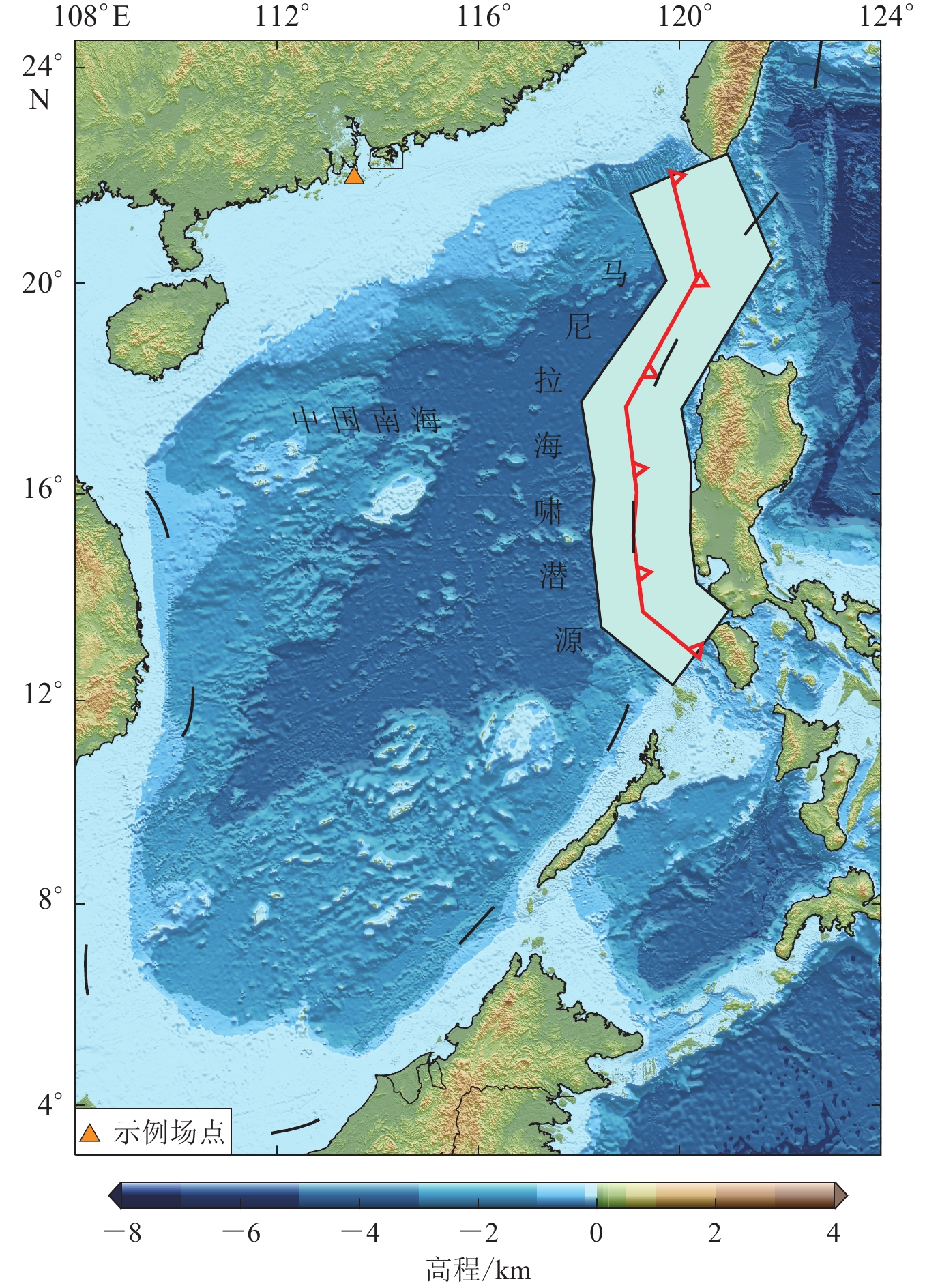
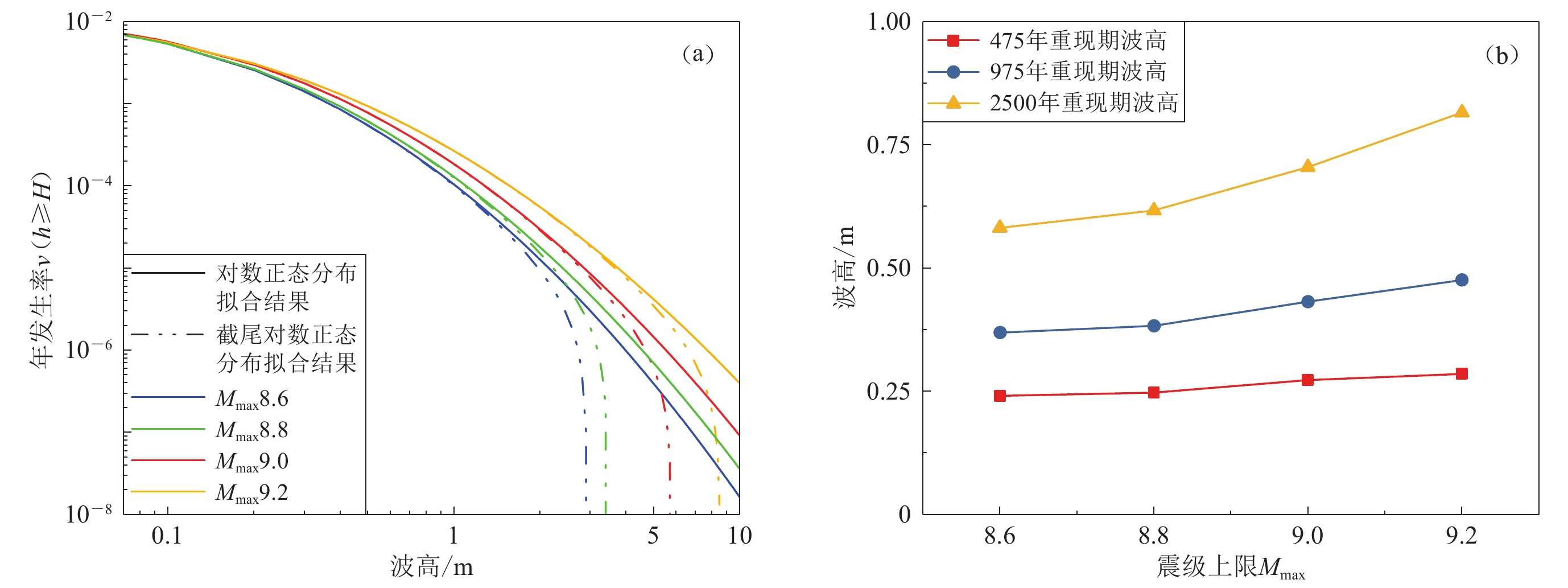
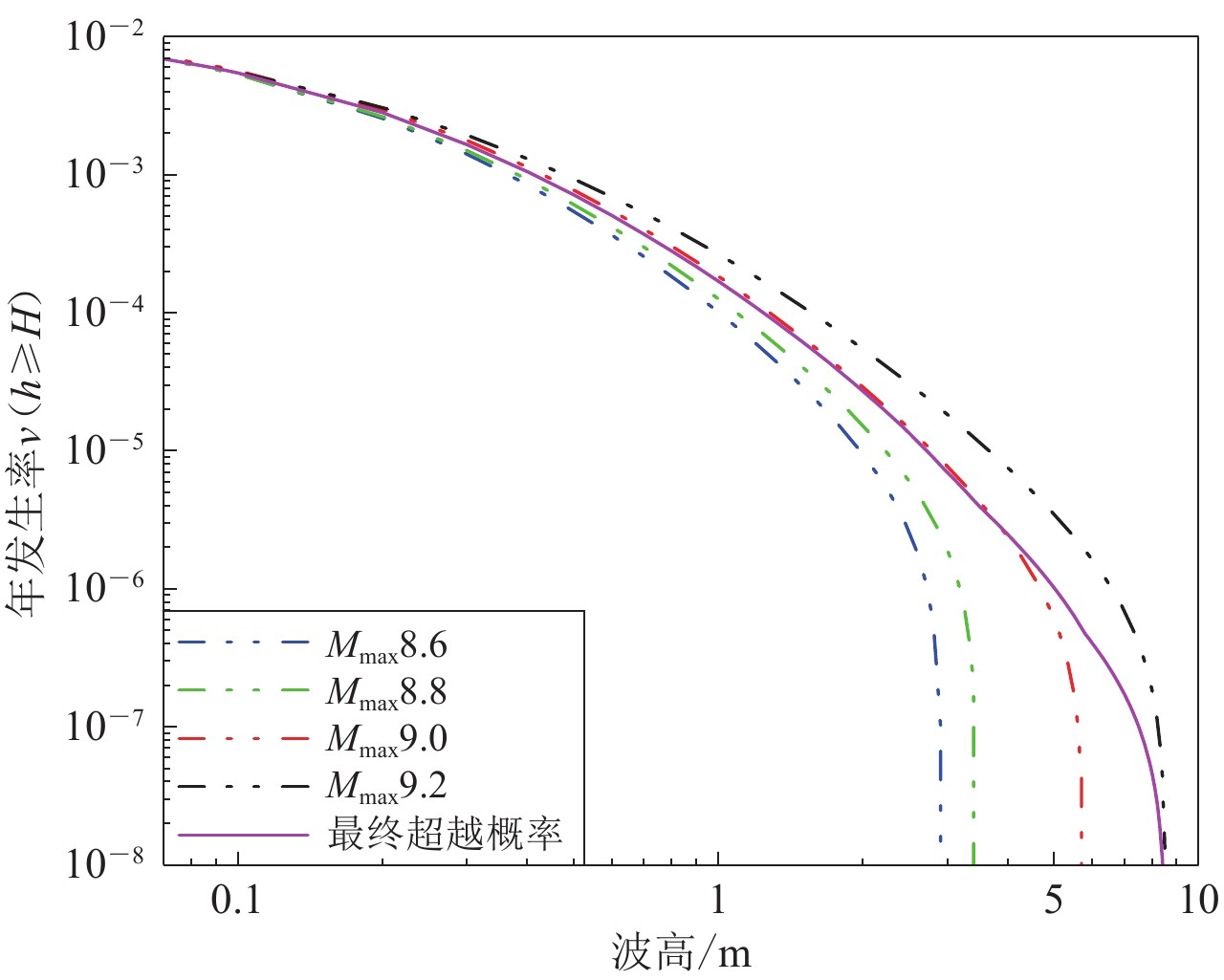
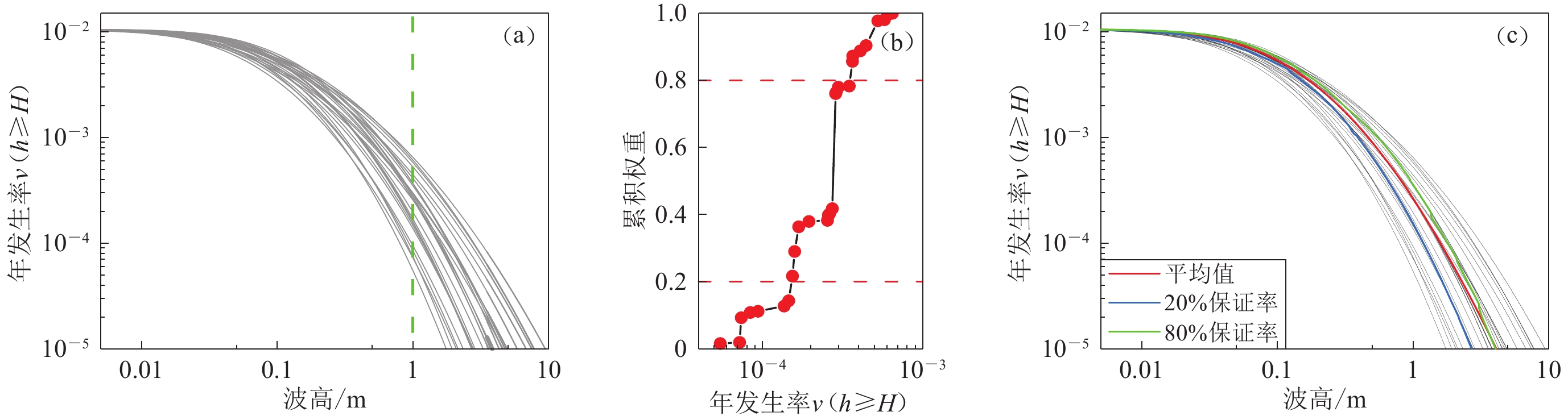
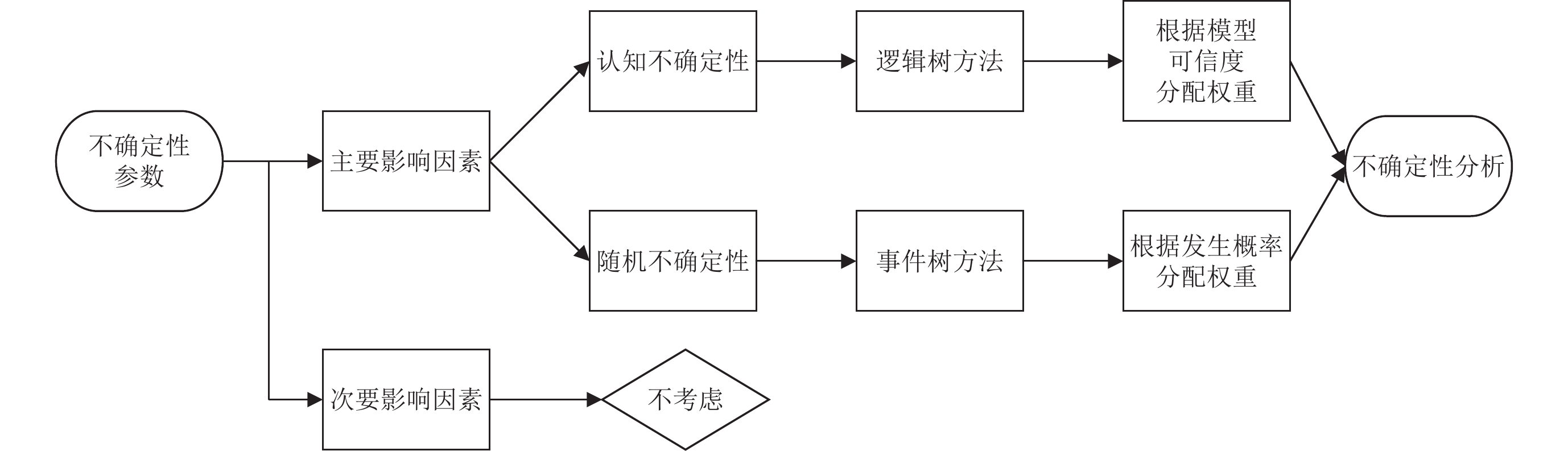
摘要: 针对海啸危险性概率分析（PTHA）存在的较大不确定性问题，对不确定性产生来源进行了归纳和分类，提出了基于逻辑树与事件树方法合理量化不确定性的思路框架，并以马尼拉海啸潜源为研究对象，给出了量化震级上限、破裂面参数不确定的过程示例。数值模拟分析结果表明：海啸潜源震级上限的改变对危险性评估结果产生了显著影响，通过逻辑树方法可合理量化这种不确定性；地震破裂面的倾角、滑移角和破裂面积的随机不确定性对海啸危险性分析结果产生较为显著的影响，经事件树方法处理后的危险性结果保证率远高于20%，略低于80%，可基本满足工程抗海啸设计要求。Abstract: Regarding the extensive uncertainties result from in the probabilistic tsunami hazard analysis （PTHA）, this study summarized the sources of these uncertainties and classified their categories. The methodologies based on logic-tree and event-tree approaches were proposed to quantify uncertainties in PTHA. And then, taking the potential tsunami source （PTS） of Manila trench as an example, both methodologies were performed to illustrate their effectiveness on quantifing the uncertainties derived from the magnitude upper-limit and rupture plane parameters. Some conclusions were drawn as follows: The variability of magnitude upper-limits of PTS affects remarkably the result of PTHA, suggesting a particular consideration that could be quantified effectively using the logic-tree approach. The dip, rake and rupture areas of PTS affect moderately the result of PTHA. The guarantee rate of tsunami hazard given by PTHA will be considerably higher than 20% and slightly lower than 80% when the uncertainties are quantified by an event-tree approach, meeting the requirements of tsunami-resilient structural design.
-
-
![]()
图 1 PTHA不确定性分析流程图
Figure 1. Illustration of the process to quantifying uncertainties in PTHA
![]()
图 2 马尼拉海啸潜源及示例场点位置
Figure 2. The location of Manila potential tsunami source and example sites
![]()
图 3 示例场点的海啸波高年发生率曲线（a）与不同重现期的海啸波高值（b）
Figure 3. Annual occurrence rate of tsunami waves exceeding a given height （a） and tsunami wave heights in terms of different return period （b） for the sample site
![]()
图 4 逻辑树处理震级上限不确定性的示意图
Figure 4. Outline of the logic-tree approach processing uncertainity of magnitude upper-limits
![]()
图 5 通过逻辑树方法确定示例场点的海啸波高年发生率
Figure 5. Annual occurrence rate of tsunami waves exceeding a given height by the logic-tree approach for the sample site
![]()
图 6 马尼拉潜源统计区内历史地震的破裂面倾角（a）和滑移角（b）的累积概率分布
Figure 6. Cumulative distribution function of dip （a） and rake （b） from historical seismic data in Manila regional PTS statistical area
![]()
图 7 事件树表现形式和各分支节点的权重（分支线上数值）
Figure 7. Outline of the event-tree approach and the weight of the nodes on of each branch
![]()
图 8 （a） 采用事件树方法计算得到的某示例场点的海啸危险性曲线；（b） 海啸波高超过1 m的年发生率累计权重分布；（c） 不同保证率的危险性曲线
Figure 8. （a） Tsunami hazard curves processed by the event-tree method for the sample site；（b） Cumulative weight of the annual rate of tsunami wave exceeding 1 m；（c） Tsunami hazard curves with different guarantee rate
表 1 随机不确定性与认知不确定的比较
Table 1 Comparison of aleatory uncertainty and epistemic uncertainty
原因 表现 取值 举例 随机不确定性 自然现象自身随机性导致
不可避免的参数的确定 随机性由概率
分布确定倾角、滑移角、破裂面积
等取值的变异性认知不确定性 认识不足导致，能通过研
究的深入减少理论、模型、方法的选取 不同的方法 模型的差别（经验公式的
选取）和破裂模型的选取
下载: 导出CSV
表 2 PSHA中不确定性分类（引自McGuire，2004）
Table 2 classification of uncertainties in PSHA （after McGuire，2004）
分类 PSHA的相关因素 随机不确定性 地震震中位置 地震震源特性（例如，震级） 目标场点给定中位值的地震动 断层破裂过程的细节（例如，破裂方向） 认知不确定性 发震区域的几何形态 震源参数的分布模型（b值，最大震级等） 给定震源特性的地震动中位值 地震动的上限值
下载: 导出CSV
表 3 PTHA中涉及参数的不确定性类型
Table 3 Classification of uncertainties in PTHA
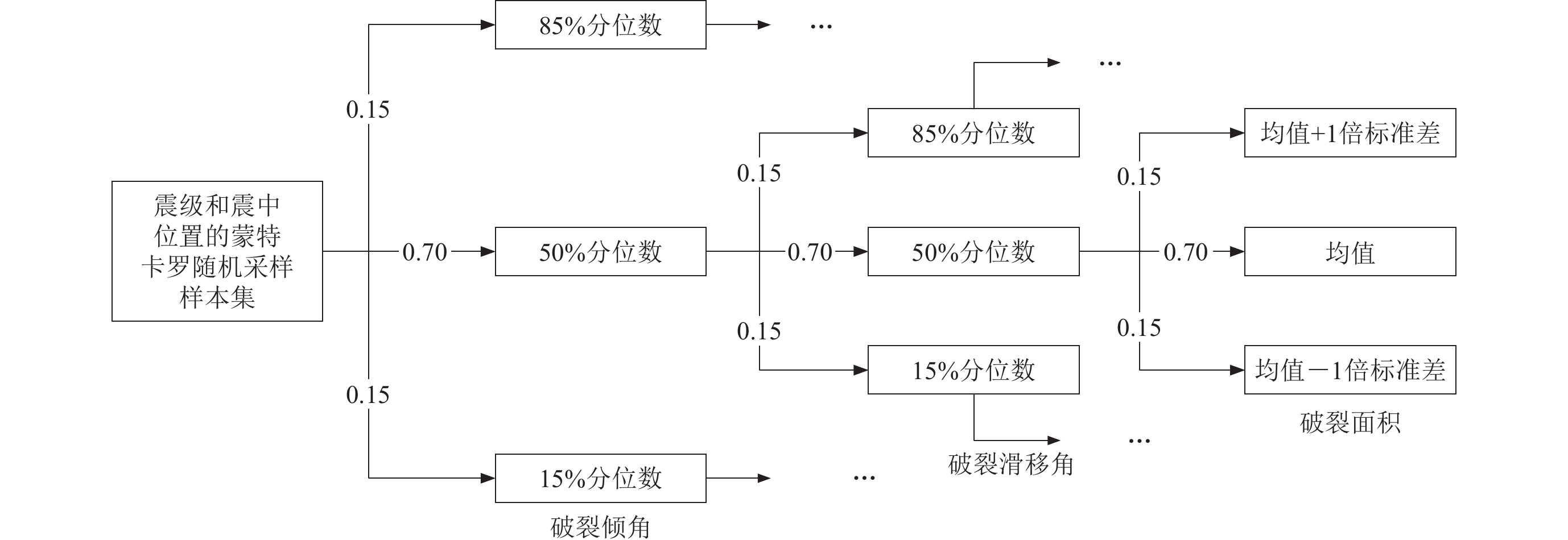
模型及参数 不确定性类型 传播过程 海啸传播模型 认知不确定性 海洋水深数据 认知不确定性 生成过程 地震重现期模型 认知不确定性 滑移分布模型 认知不确定性 地震位置分布模型 认知不确定性 倾角分布模型 认知不确定性 破裂面面积模型 认知不确定性 震级上限 认知不确定性 地震震级 随机不确定性 地震位置 随机不确定性 地震深度 随机不确定性 倾角 随机不确定性 滑移角 随机不确定性 破裂面积 随机不确定性
下载: 导出CSV
表 4 事件树各个分支的破裂倾角、滑移角和破裂面积取值及该分支的的权重
Table 4 The vaule of dip，rake and rupture area for each branch and the the weight of each branch
分支
编号破裂面
倾角/°破裂面
滑移角/°破裂面
面积/km2权重 分支
编号破裂面
倾角/°破裂面
滑移角/°破裂面
面积/km2权重 1 38.611 52 48.7 μ－σ 0.015 75 15 24.015 15 82.6 μ＋σ 0.015 75 2 38.611 52 48.7 μ 0.073 5 16 24.015 15 125.7 μ－σ 0.003 375 3 38.611 52 48.7 μ＋σ 0.015 75 17 24.015 15 125.7 μ 0.015 75 4 38.611 52 82.6 μ－σ 0.073 5 18 24.015 15 125.7 μ＋σ 0.003 375 5 38.611 52 82.6 μ 0.343 19 62.079 55 48.7 μ－σ 0.003 375 6 38.611 52 82.6 μ＋σ 0.073 5 20 62.079 55 48.7 μ 0.015 75 7 38.611 52 125.7 μ－σ 0.015 75 21 62.079 55 48.7 μ＋σ 0.003 375 8 38.611 52 125.7 μ 0.073 5 22 62.079 55 82.6 μ－σ 0.015 75 9 38.611 52 125.7 μ＋σ 0.015 75 23 62.079 55 82.6 μ 0.073 5 10 24.015 15 48.7 μ－σ 0.003 375 24 62.079 55 82.6 μ＋σ 0.015 75 11 24.015 15 48.7 μ 0.015 75 25 62.079 55 125.7 μ－σ 0.003 375 12 24.015 15 48.7 μ＋σ 0.003 375 26 62.079 55 125.7 μ 0.015 75 13 24.015 15 82.6 μ－σ 0.015 75 27 62.079 55 125.7 μ＋σ 0.003 375 14 24.015 15 82.6 μ 0.073 5 注：表中μ为利用式（5）确定的破裂面面积平均值，σ为统计标准差。
下载: 导出CSV
-
洪明理,任鲁川,霍振香. 2014. 基于E-FAST法分析海啸波高对潜在海啸源参数的敏感性[J]. 地震学报,36(2):252–260. doi: 10.3969/j.issn.0253-3782.2014.02.010 Hong M L,Ren L C,Huo Z X. 2014. Sensitivity analysis on maximum tsunami wave heights to the potential tsunami source parameters based on extended FAST method[J]. Acta Seismologica Sinica,36(2):252–260 (in Chinese).
任鲁川,霍振香,洪明理. 2014. 耦合潜源参数不确定性效应的地震海啸危险性分析:原理与方法[J]. 海洋预报,31(6):7–13. doi: 10.11737/j.issn.1003-0239.2014.06.002 Ren L C,Huo Z X,Hong M L. 2014. Principle and method of the seismic tsunami hazard analysis coupling uncertainty effect of potential source parameters[J]. Marine Forecasts,31(6):7–13 (in Chinese).
任叶飞,杨智博,温瑞智,金波. 2015. 地震海啸数值模拟中海洋水深数据的敏感性研究[J]. 自然灾害学报,24(2):15–22. doi: 10.13577/j.jnd.2015.0203 Ren Y F,Yang Z B,Wen R Z,Jin B. 2015. Bathymetry data:Sensitivity in the numerical simulation of earthquake tsunami[J]. Journal of Natural Disasters,24(2):15–22 (in Chinese).
王培涛,于福江,原野,闪迪,赵联大. 2016. 海底地震有限断层破裂模型对近场海啸数值预报的影响[J]. 地球物理学报,59(3):1030–1045. doi: 10.6038/cjg20160324 Wang P T,Yu F J,Yuan Y,Shan D,Zhao L D. 2016. Effects of finite fault rupture models of submarine earthquakes on numerical forecasting of near-field tsunami[J]. Chinese Journal of Geophysics,59(3):1030–1045 (in Chinese).
周本刚, 何宏林, 安艳芬. 2011. 琉球海沟、马尼拉海沟地震构造背景及震源参数评估报告[R]. 北京: 中国地震局地质研究所, 中国地震局地球物理研究所, 中国地震局地震预测研究所: 1–2. Zhou B G, He H L, An Y F. 2011. Report of the Evaluation of the Seismotectonic Background and Source Parameters in the Ryukyu Trench and Manila Trench[R]. Beijing: Institute of Geology, Institute of Geophysics, Institute of Earthquake Science, China Earthquake Administration: 1–2 （in Chinese）.
Andrews J D, Moss T R. 2002. Reliability and Risk Assessment[M]. 2nd ed. London: Professional Engineering Publishing Limited: 1–2.
Budnitz R J, Apostolakis G, Boore D M. 1997. Recommendations for Probabilistic Seismic Hazard Analysis: Guidance on Uncertainty and Use of Experts[R]. Washington D C: Office of Scientific & Technical Information: 2–3.
Choi B H,Min B I,Pelinovsky E,Tsuji Y,Kim K O. 2012. Comparable analysis of the distribution functions of runup heights of the 1896,1933 and 2011 Japanese Tsunamis in the Sanriku area[J]. Nat Hazards Earth Syst Sci,12(5):1463–1467.
Delavaud E,Cotton F,Akkar S,Scherbaum F,Danciu L,Beauval C,Drouet S,Douglas J,Basili R,Sandikkaya M A,Segou M,Faccioli E,Theodoulidis N. 2012. Toward a ground-motion logic tree for probabilistic seismic hazard assessment in Europe[J]. J Seismol,16(3):451–473. doi: 10.1007/s10950-012-9281-z
Gica E,Teng M H,Liu P L F,Titov V,Zhou H Q. 2007. Sensitivity analysis of source parameters for earthquake-generated distant tsunamis[J]. J Waterw Port Coastal Ocean Eng,133(6):429–441. doi: 10.1061/(ASCE)0733-950X(2007)133:6(429)
Hoffman F O,Hammonds J S. 1994. Propagation of uncertainty in risk assessments:The need to distinguish between uncertainty due to lack of knowledge and uncertainty due to variability[J]. Risk Anal,14(5):707–712. doi: 10.1111/j.1539-6924.1994.tb00281.x
Kulkarni R, Youngs R, Coppersmith K. 1984. Assessment of confidence intervals for results of seismic hazard analysis[C]//Proceedings of the Eighth World Conference on Earthquake Engineering. San Francisco: prentice-Hall: 263–270.
Li H W,Yuan Y,Xu Z G,Wang Z C,Wang J C,Wang P T,Gao Y,Hou J M,Shan D. 2017. The dependency of probabilistic tsunami hazard assessment on magnitude limits of seismic sources in the south China sea and adjoining basins[J]. Pure Appl Geophys,174(6):2351–2370.
Li L L,Switzer A D,Chan C H,Wang Y,Weiss R,Qiu Q. 2016. How heterogeneous coseismic slip affects regional probabilistic tsunami hazard assessment:A case study in the South China Sea[J]. J Geophys Res Solid Earth,121(8):6250–6272. doi: 10.1002/2016JB013111
McGuire R K. 2004. Seismic Hazard and Risk Analysis[M]. Oakland: Earthquake Engineering Research Institute: 37.
Necmioğlu Ö,Özel N M. 2014. An earthquake source sensitivity analysis for tsunami propagation in the eastern Mediterranean[J]. Oceanography,27(2):76–85. doi: 10.5670/oceanog.2014.42
Papazachos B C,Scordilis E M,Panagiotopoulos D G,Papazachos C B,Karakaisis G F. 2004. Global relations between seismic fault parameters and moment magnitude of earthquakes[J]. Bull Geol Soc Greece,36(3):1482–1489. doi: 10.12681/bgsg.16538
Ren Y F,Wen R Z,Zhang P,Yang Z B,Pan R,Li X J. 2017. Implications of local sources to probabilistic tsunami hazard analysis in South Chinese coastal area[J]. J Earthq Tsunami,11(1):1740001. doi: 10.1142/S1793431117400012
Sørensen M B,Spada M,Babeyko A,Wiemer S,Grünthal G. 2012. Probabilistic tsunami hazard in the Mediterranean sea[J]. J Geophys Res Solid Earth,117(B1):B01305.
Thio H K, Wilson R I, Miller K. 2014. Evaluation and application of probabilistic tsunami hazard analysis in California[C]//2014 AGU Fall Meeting. SanFrancisco: American Geophysical Union: NH12A-01.
-
期刊类型引用(2)
1. 汪小厉，周冬瑞，李玲利，张炳，柳建，李军辉. “霍山地震窗”小震检测及其在地震预测中的应用. 地震. 2024(02): 135-146 . 
百度学术
2. 郝美仙，陈立峰，张帆，张珂，尹战军，王禄军. 蒙冀辽交界地区地壳应力场研究. 中国地震. 2024(03): 700-708 . 百度学术
其他类型引用(0)
计量
- 文章访问数: 475
- HTML全文浏览量: 248
- PDF下载量: 69
- 被引次数: 2
