Strong ground motion simulation for the 2014 MW6.1 Ludian，Yunnan earthquake
-
摘要:
基于震源运动学模型，采用宽频带混合模拟方法，对2014年鲁甸MW6.1地震开展了考虑复杂震源破裂过程的地震动模拟，将代表性场点的模拟结果与实际观测的强震记录进行对比，分析了不同震源模型对场点强地面运动特征影响的差异。结果表明：就震源尺度有限的中等地震而言，地震矩相近的不同震源破裂模型对震中距稍远场点的地震动影响差异相对较小，而对近震源区场点的地震动特征影响差异则较为明显。此外，不同震源模型模拟的地震动参数空间分布结果显示，不同震源模型对地震动的空间分布形态具有显著影响。
Abstract:Based on the intensity and aftershock distribution, researchers unanimously agree that the rupture of the 2014 MW6.1 Ludian, Yunnan earthquake exhibits a certain degree of complexity. Zhang et al （2015） used broadband strong motion records within a 250 km range from the epicenter, along with full waveform data of near-field and far-field broadband body wave data, to invert the rupture process of the Ludian MW6.1 earthquake base on both a single fault model and two intersecting conjugate fault models. Their results indicate that the Ludian MW6.1 earthquake was a complex seismic event, characterized by the successive rupture of two conjugate faults in the northwest and northeast directions. Significant progress has been made in studying the characteristics of strong ground motion related to the Ludian earthquake and associated issues. The existing conclusions about the distribution of seismic parameters in affected region are directly inferred or indirectly synthesized from existing data, yet the seismic characteristics caused by complex seismic source ruptures have not been considered.
To investigate the influence of different rupture process models on ground motion simulation of the MW6.1 Ludian earthquake, a hybrid broadband ground motion simulation method known as the GP method （Graves, Pitraka, 2010） was applied to synthesize acceleration, velocity waveforms, and acceleration response spectra in the near-field area based on kinematic source models. The source models used for comparison in this study are derived from Zhang et al （2015）, which includes two single fault rupture models and one intersecting conjugate fault model. The study adopted the crustal velocity structure model of the Yunnan region to calculate the Green’s function.
The GP method combines a deterministic approach in the low-frequency range （ f＜1 Hz） with a semi-stochastic approach in the high-frequency range （ f ＞1 Hz）. For f＜1 Hz, this methodology allows for a theoretically rigorous representation of fault rupture and wave propagation effects, to generate ground motion waveforms and amplitudes. In contrast, high-frequency （ f ＞1 Hz） ground motions are modeled through random source radiation, simplified theoretical wave propagation, and scattering effects.Simulation results for representative sites were compared with observed strong motion recordings, and the impacts of three different source rupture models on strong ground motions were analyzed. The results demonstrate that for moderate earthquakes with limited fault dimensions, differences in strong ground motion characoncernedcteristics among different source rupture models with similar seismic moments are not pronounced in far fields but are distinct in near-fields. Additionally, the distributions of intensity measurements of ground motions are provided, and it is found that the simulation results based on the conjugate fault model are more consistent with the field-surveyed macroseismic intensity of MW6.1 Ludian earthquake, Yunnan in 2014. This indicates that the source rupture model plays a crucial role in the spatial distribution pattern of strong ground motions and their characteristics. Therefore, elaborate source rupture models are of great value for reasonably estimating the peak ground acceleration, peak ground velocity, frequency spectrum, duration, and time history of seismic motion in the near-source area, as well as assessing seismic hazard in area.
This article reveals the influence of complex focal rupture processes on the characteristics and spatial distribution of strong ground motion.
-
引言
2014年8月3日云南鲁甸发生了MW6.1地震。此次地震造成了617人死亡，112人失踪，3 143人受伤，灾区房屋建筑、工程设施大量损毁，诱发了大规模的地震地质灾害（卢永坤等，2014；张振国等，2014），引起了地震学界的广泛关注。鲁甸地震的余震分布呈近东西向和北西向的不对称共轭状分布（房立华等，2014；王未来等，2014；张广伟等，2014），表明此次地震的发震构造和破裂过程可能具有一定的复杂性。烈度分布和早期的破裂过程研究结果推断此次地震的发震构造为近南北向的小河—包谷垴断裂（徐锡伟等，2014；张勇等，2015；刘成利等，2014）；许力生等（2014）的视震源时间函数分析结果显示鲁甸MW6.1地震可能是共轭断层先后破裂的结果；张广伟等（2014）根据余震分布和震源机制解结果也提出了鲁甸MW6.1地震存在共轭破裂的可能性；张勇等（2015）利用距震中250 km范围内的强震记录近震宽频带的全波形数据及远震宽频带体波数据，分别以单一断层和两条交叉的共轭断层模型，对鲁甸MW6.1地震的破裂过程进行反演，结果表明鲁甸MW6.1地震是北西向和北东向两条共轭的断层先后破裂的一次复杂地震事件。
关于鲁甸MW6.1地震强地震动特征及其相关问题的研究已取得重要进展。中国数字强震台网有81个台站获得了此次地震的强震记录（崔建文等，2014），其中，断层距小于300 km有32个台站的加速度记录可用，震中距小于50 km有7个台站获得加速度记录，震中附近获得的强震记录相对较少。冀昆等（2014）将鲁甸地震各台站的强震动记录与国内西部地区常用衰减关系对比，结果表明，除近场的个别台站外，鲁甸地震各台站的峰值加速度、峰值速度观测值均不同程度地低于地震动参数衰减模型的预测值。魏勇等（2018）研究表明，位于极震区的龙头山镇台站，其强震记录的峰值加速度较大，主要与龙头山强震台场地较强的放大作用有关。张振国等（2014）采用三维曲线有限差分方法模拟了鲁甸地震的波场传播过程，初步模拟了区域地震烈度分布。陈鲲等（2015）综合考虑震中地区地质构造背景、震源机制解、余震分布以及我国西部地区地震动参数衰减特征，快速给出了峰值加速度分布图。张斌等（2020）以断层距300 km以内32个台站的水平向加速度记录作为数据资料研究了地震动衰减关系模型的适用性。Hu等（2016）研究了此次地震动参数的衰减特征，发现地震动衰减具有方向性特征。断层模型设定为近南北向单断层破裂的情况下未观察到明显的上下盘效应，且短周期地震动小于衰减关系预测值，他们推测这现象与走滑机制有关。上述研究通过已有数据直接推断或者间接合成区域内地震动参数分布情况，但尚未给出考虑复杂震源破裂引起的地震动特性。而张勇等（2015）研究已经表明参与鲁甸地震震源破裂过程中的两条共轭断层相互关联。Wang和Wen （2019）采用随机有限断层地震动合成方法，给出了考虑两条L形断层参与地震破裂过程的地震动空间分布，该研究是目前最接近共轭破裂模式的地震动模拟工作。
本文拟利用张勇等（2015）基于近场宽频带资料和强地震动记录和远震体波资料反演的鲁甸地震震源破裂模型，结合云南地区的地壳速度结构模型，借助宽频带地震动混合模拟方法（Graves and Pitarka，缩写为GP）（Graves，Pitarka，2010）计算近场区域加速度、速度波形及加速度反应谱等，并与观测记录进行对比，得到鲁甸地震相互关联的共轭断层共同参与的震源破裂过程对强地震动特性及其空间分布的影响，以期揭示复杂震源破裂过程对强地面运动特征及其空间分布的影响特征。
1. 宽频带强地面运动混合模拟方法
本文研究采用GP混合模拟方法（Graves，Pitarka，2010）计算宽频带强地面运动。其中：高频（ f ＞1 Hz）地震动以随机有限断层方法模拟（Boore，1983，2003）；低频地震动（ f ＜1 Hz）以三维有限差分模拟（Graves，1996）。最终应用四阶巴特沃斯（Butterworh）滤波器将低频和高频响应组合成一个单一的宽带时间序列，该滤波器具有共同的截至频率为1 Hz，合成的宽频带地震动范围为0.01—40 Hz。
模拟高频地震动的随机有限断层方法（Boore，2003）是将断层划分为一系列可看作点源模型（Brune，1970）的子断层，计算每一个子断层在目标场点的地震动，考虑破裂时间与地震波传播相对于加速度时程的延迟，将所有子断层在场点的地震动累加起来，得到整个断层在该场点的地震动。
子断层的加速度谱可表示为
$$ {A}_{i} （ f ） ＝\sum _{j＝1， M}{C}_{ij}{S}_{ i} （ f ） {G}_{ij} （ f ） P （ f ） \text{，} $$ (1) 式中：$ {G}_{ij} （ f ） \mathrm{为}\mathrm{格}\mathrm{林}\mathrm{函}\mathrm{数}；P （ f ） \mathrm{为}\mathrm{高}\mathrm{频}\mathrm{衰}\mathrm{减}\mathrm{项} $；Cij为辐射因子，表示为
$$ {C}_{ij}＝\frac{{R}_{\mathrm{P}ij}}{2\pi {\rho }_{i}{\beta }_{i}^{3}}\text{，} $$ (2) 式中，RP为辐射花样，ρ和β为密度和剪切波速；Si（f）为震源谱，表示为
$$ {S}_{i} （ f ） ＝\frac{{m}_{i}F{f}^{2}}{1＋F{\left(\dfrac{f}{{f}_{\mathrm{c}i}}\right)}^{2}}， $$ (3) 式中，${m}_{i}＝{d}_{i}{\mu }_{i}{A}_{{\rm{T}}}/{M}_{0}$为子源地震矩，${\mu }_{i}$和$ {d}_{i} $分别为子断层的刚度系数和滑动量，${A}_{{\rm{T}}}$和$ {M}_{0} $对应断层总尺寸和整个断层的地震矩，F表明子源和总断层地震矩的关系（Frankel et al，1998）表示为
$$ F＝\frac{{M}_{0}}{N{\sigma }_{\mathrm{P}}{l}^{3}}\text{，} $$ (4) 式中，$ {\sigma }_{\mathrm{P}} $为Brune应力参数，在GP方法中设定为5 MPa，N和l是子断层个数和尺寸，子断层拐角频率$ {f}_{\mathrm{c}i} $则为
$$ {f}_{{\rm{c}}i}＝\frac{{c}_{0}{V}_{{\rm{R}}i}}{{\alpha }_{\tau }\pi dl}， $$ (5) 式中，$ {c}_{0}＝2.1 $为经验所得参数，$ {V}_{\mathrm{R}i} $和$ {\alpha }_{\tau } $分别为子断层破裂速度和上升时间，根据震源模型中子断层震源时间函数给定。
式（1）中格林函数为：
$$ {G}_{ij} （ f ） ＝\frac{{I}_{i} （ f ） }{{r}_{ij}}\mathrm{e}\mathrm{x}\mathrm{p}\left[-\pi {f}^{1－x}\sum _{k＝1， L}\frac{{t}_{ijk}}{{q}_{k}}\right]\text{，} $$ (6) 式中，$ {r}_{ij} $是场点第j条射线到第i个子源的距离；总阻抗$ {I}_{i} （ f ） $由Boore和Joyner （1997）用四分之一波长理论计算；e的指数项表征粘弹性衰减项，$ {t}_{ijk} $为到时，Ou和Herrmann （1990）将Q值表示为依赖频率和距离变化的形式$Q＝Q （ f， r ） $，在此品质因子Q值用式（7）表示为与剪切波速线性相关的形式：
$$ {Q}_{k}＝a＋{b\beta }_{k}\text{，} $$ (7) 式中，a，b为经验系数。高频衰减项P（f）与介质品质因子Q和剪切波速β相关（Anderson，Hough，1984）：
$$ P （ f ） ＝{{A}_{0}\mathrm{e}}^{-\pi f{t}^{*}}，\quad {t}^{*}＝\int \frac{\mathrm{d}r}{{Q}_{\beta } （ r ） \beta （ r ） }. $$ (8) 低频地震动采用三维黏弹性有限差分算法，直接求解包含震源破裂过程和传播路径影响的弹性动力学波动方程。Graves （1996）推导了交错网格有限差分技术应用在三维弹性介质中波传播问题的详细公式，实现了在时域上用有限差分稳定、准确地计算三维时空运动（平面自由边界）。
场地影响以场点地表下30 m处剪切波速$ {v}_{\mathrm{S}30} $来表征场地放大系数（Walling et al，2008）。
2. 模型参数
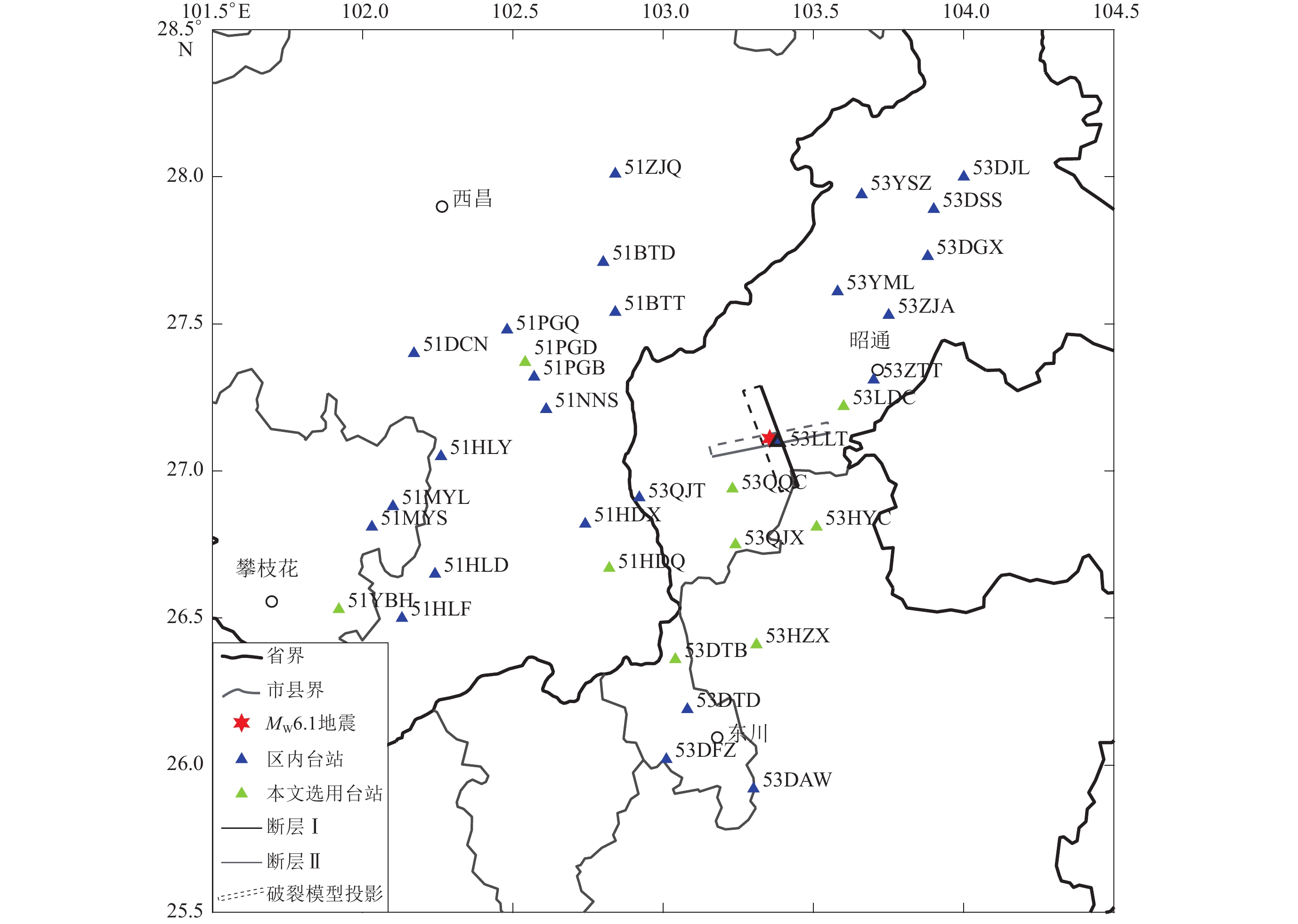
使用宽频带地震动主要模拟近场区域内记录台站的波形数据和地震动参数，震中距160 km范围内有数据记录34个台站，具体位置见图1。
![]()
图 1 鲁甸地震研究区域内断层面投影及台站位置Figure 1. Fault plane projection and station location of Ludian earthquake in the study region2.1 震源破裂模型
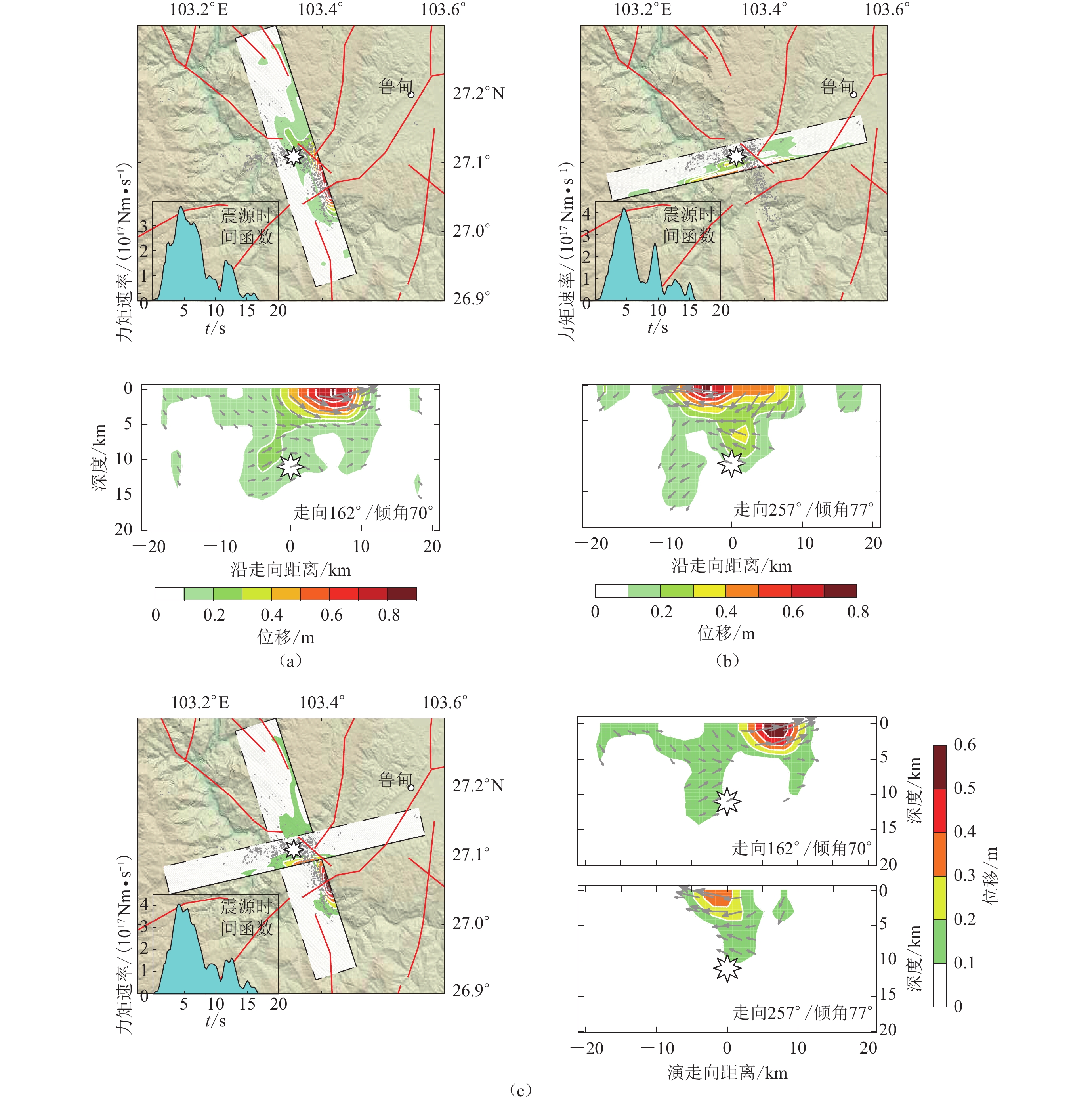
张勇等（2015）根据鲁甸地震远震体波资料与近震宽频带和强震记录联合反演，建立了相互关联的共轭断层破裂模型，其地表投影如图1所示，显示出鲁甸地震是一次在北西向主压应力和北东向主张应力的统一作用下发生的两条共轭断层先后破裂的复杂地震事件。该模型是国内关于此次地震破裂认可度较高的模型，因此，本文选取张勇等（2015）给出的鲁甸地震有限断层模型（断层破裂震源模型 Ⅰ 和Ⅱ及 Ⅰ 与Ⅱ共轭断层破裂震源模型）进行地震动模拟，模型参数（断层产状、尺度及地震矩）列于表1。断层 Ⅰ 和断层Ⅱ的长度和宽度均为21 km和10 km；断层模型 Ⅰ 、断层模型Ⅱ及共轭断层复合模型的地震矩分别为1.79×1018 N·m，1.80×1018 N·m和2.05×1018 N·m。断层 Ⅰ 释放能量62%，断层Ⅱ释放能量38%。断层破裂震源模型 Ⅰ 和Ⅱ及Ⅰ与Ⅱ共轭断层破裂震源模型的滑动分布如图2所示。
表 1 两个单断层模型及共轭复合断层震源模型参数（张勇，2015）Table 1. Parameters of two single fault plane models and conjugated faults model （Zhang，2015）震源模型 走向/° 倾向/° 长度/km 宽度/km 子断层尺寸 地震矩/（1018 N·m） MW 断层Ⅰ模型 162 70 21 10 2 km×2 km 1.79 6.13 断层Ⅱ模型 257 77 21 10 2 km×2 km 1.80 6.14 共轭断层模型 复合 复合 复合 复合 2 km×2 km 2.05 6.17 ![]()
图 2 断层Ⅰ（a）、断层Ⅱ（b）及共轭复合断层（c）的震源模型（张勇等，2015）Figure 2. Geometry，source time function and final slip distributions of source faultⅠmodel （a），fault Ⅱ model （b） and conjugated fault model （c）（Zhang et al，2015）2.2 速度结构模型
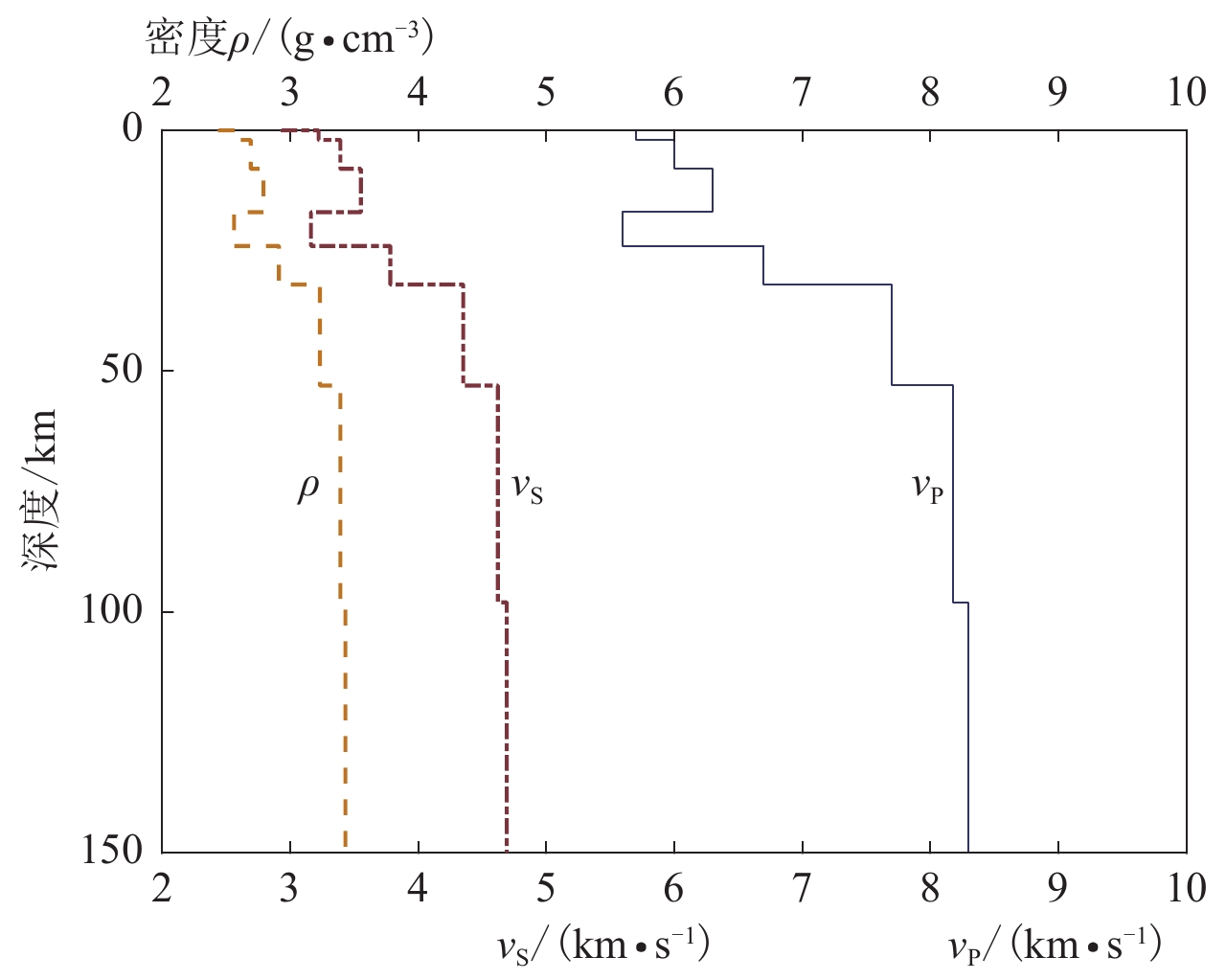
地震动模拟采用的P 波、S波及介质密度一维结构模型，如图3所示。其中，鲁甸地区P波一维速度模型是采用房立华等（2014）根据徐涛等（2014）给出的地壳浅部速度结构信息给出的结果；S波波速模型是由P波与S波波速之比1.73得到的（王未来等，2014）；介质密度模型是根据P波波速结构模型和密度与P波速度的经验关系$d＝0.32{v}_{{\rm{P}}}＋0.77$外推获得的，其中d为深度。
![]()
图 3 鲁甸地区一维速度结构及介质密度模型Figure 3. One-dimensional velocity structure and medium density model in Ludian region2.3 台站信息及场地条件
场地影响以地表以下30 m内的平均剪切波速${v}_{{\rm{S}} 30}$作为参考。根据震中距从近到远、覆盖断层不同方位，随机挑选了9个台站进行讨论（图1绿色三角形标记）。表2给出了这9个台站位置、场地类别、震中距等信息。${v}_{{\rm{S}}30}$根据Yu等（2016）结果得到，钻孔不足30 m的或者缺失的台站数据根据速度梯度延拓线性模型外推（Boore，2004）或者邻近场地近似。
表 2 震中距160 km内部分台站相关信息（顺序依震中距排列）Table 2. List of relevant information of part discussed stations within 160 km of the epicenter （arranged according to the epicenter distance）台站 东经/° 北纬/° 场地类型 震中距/km 断层Ⅰ断层距/km 断层Ⅱ断层距/km $ {v}_{\mathrm{S}30} $ 53QQC 103.23 26.94 土 19.09 14.77 13.24 527# 53LDC 103.60 27.22 岩 32.54 28.99 13.93 424 53HYC 103.51 26.81 土 38.38 17.26 33.52 497* 53QJX 103.24 35.75 土 39.37 24.44 33.97 527# 51HDQ 102.82 26.67 土 67.47 60.57 49.07 747 53HZX 103.31 26.41 土 76.73 57.60 72.24 318* 51PGD 102.54 27.37 土 80.92 69.31 65.85 688 53DTB 103.04 26.36 岩 86.24 70.21 75.09 760 51YBH 101.92 26.53 土 150.90 147.53 129.68 376 注：标*数据由Boor （2 004）的速度梯度延拓线性模型外推计算而得，标#数据根据邻近场地插值计算得到。 3. 强地面运动模拟结果
3.1 加速度时程
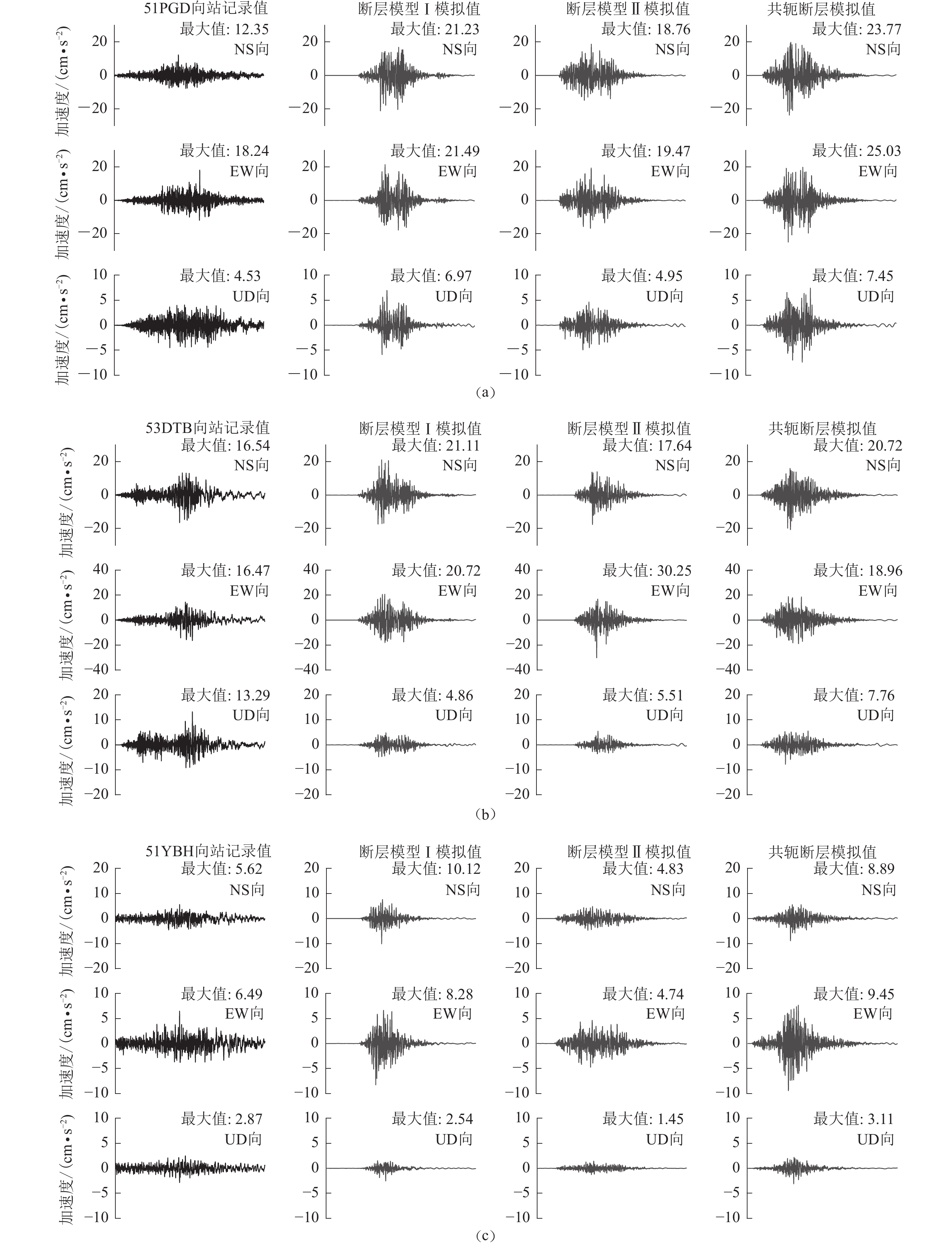
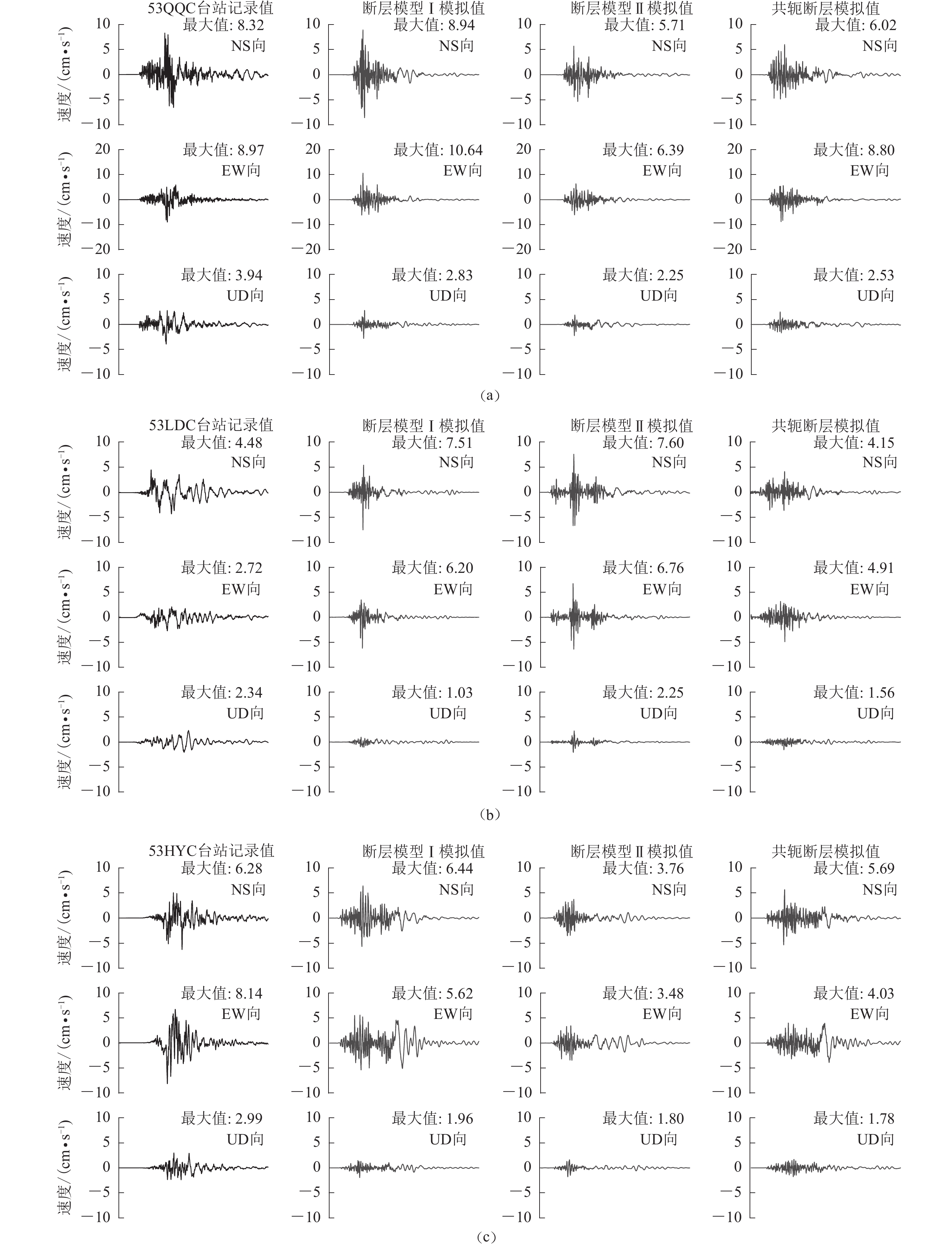
所有台站的记录值和模拟结果都显示出水平向峰值加速度（peak ground acceleration，缩写为PGA）明显高于竖向。由于篇幅所限，图4，5，6仅给出53QQC，53LDC，53HYC，53QJX，51HDQ，53HZX，51PGD，53DTB和51YBH的加速度实际记录和基于单断层Ⅰ震源模型、单断层Ⅱ震源模型及共轭复合断层震源模型模拟的加速度时程。从图4-6可以看出距震源区一定距离的台站基于单断层Ⅰ震源模型、单断层Ⅱ震源模型及共轭复合断层模型的模拟结果比较接近，且与实际观测记录波形和幅值符合得较好，即在远场区域，地震矩相近的不同震源模型的地震动特征很相近（比如台站51HDQ，53HZX，51PGD，53DTB和51YBH）。但是，对于距震源区较近的台站，基于不同断层震源模型的地震动特征差异显著，53HYC台站处于断层Ⅰ沿线上，沿线垂直于断层Ⅱ，震中距（38.38 km）接近断层Ⅱ断层距（33.52 km）但距离断层Ⅰ更近（17.26 km），其模拟结果显示断层Ⅰ震源模型模拟PGA略高于断层Ⅱ震源模型的模拟值，基于共轭复合断层震源模型的模拟值介于二者之间，且与实际加速度记录更为接近；53LDC台站则刚好相反，处于断层Ⅱ沿线上，距离断层面Ⅰ的断层距超过断层Ⅱ断层距两倍以上，其模拟结果显示出基于断层Ⅱ震源模型的模拟峰值高于基于断层Ⅰ震源模型和共轭复合断层震源模型的模拟值。另外一个近场台站53QQC相对于两个断层面距离差距不大，三个震源模型的模拟结果比较接近。总之，对比模拟结果与实际观察记录显示：距离两个断层稍远的台站，基于不同震源模型的模拟结果差异不明显；对于近震源区的场点，相对断层不同位置、不同震源破裂模型的地震动模拟结果差异显著。此外，对于53LDC台站，模拟加速度时程比实际加速度记录波形偏高，有待进一步研究。
![]()
图 4 台站53QQC （a）、53LDC （b）和53HYC （c）的加速度记录及基于断层Ⅰ震源模型、断层Ⅱ震源模型和共轭复合震源模型合成的三分量加速度时程（持续时间35 s）Figure 4. Recordings and simulated three component acceleration time-histories of the representative stations 53QQC （a），53LDC （b） and 53HYC （c） from source faultⅠmodel，fault Ⅱ model and conjugated fault model （duration of 35 s）![]()
图 5 台站53QJX （a）、51HDQ （b）和53HZX （c）的加速度记录及基于断层Ⅰ震源模型、断层Ⅱ震源模型和共轭复合震源模型合成的三分量加速度时程（持续时间35 s）Figure 5. Recordings and simulated three component acceleration time-histories of the representative stations 53QJX （a），51HDQ （b） and 53HZX （c） from source faultⅠmodel，fault Ⅱ model and conjugated fault model （duration of 35 s）![]()
图 6 台站51PGD （a）、53DTB （b）和51YBH （c）的加速度记录及基于断层Ⅰ震源模型、断层Ⅱ震源模型和共轭复合震源模型合成的三分量加速度时程（持续时间35 s）Figure 6. Recordings and simulated three component acceleration time-histories of the representative stations 51PGD （a），53DTB （b） and 51YBH （c） from source faultⅠmodel，fault Ⅱ model and conjugated fault model （duration of 35 s）3.2 加速度反应谱
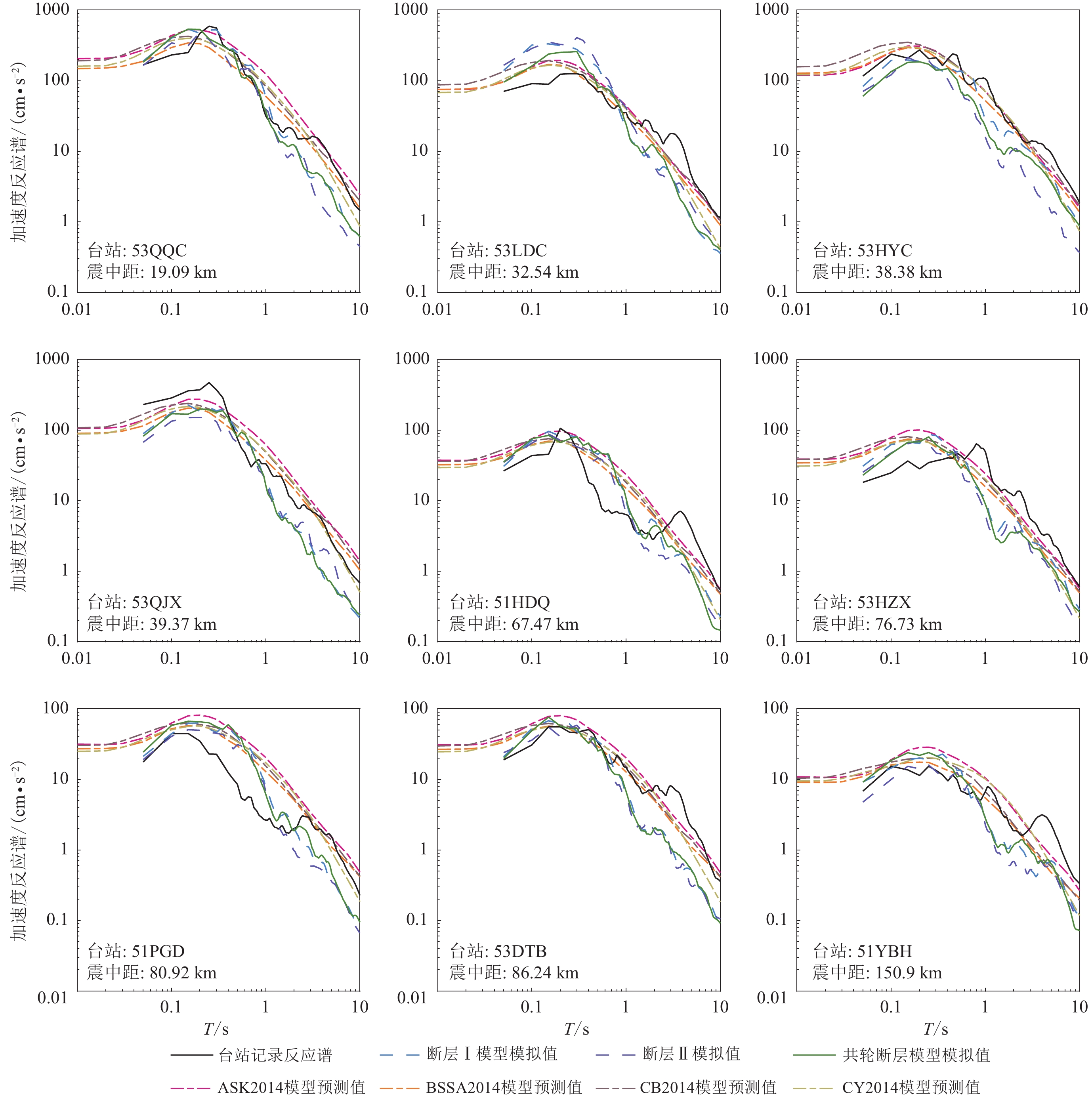
图7依据震中距远近分别给出了53QQC，53LDC，53HYC，53QJX，51HDQ，53HZX，51PGD，53DTB和51YBH的实际记录加速度反应谱与基于单断层Ⅰ震源模型、单断层Ⅱ震源模型及共轭复合断层震源模型模拟的加速度反应谱（0.01—10 s水平平均加速度反应谱）和NGA-West2预测加速度反应谱（Abrahamson et al，2014；Boore et al，2014；Campbell， Bozorgnia，2014；Chiou，Youngs，2014），NGA-West2四个预测方程是根据多条地震动的统计回归模型，根据一般中强地震的震源参数（矩震级、断层类型、几何尺寸、走向、倾角、滑动角）、台站相对位置（断层距）和场地条件（$ {v}_{{\rm{S}}30} $）（表2）计算具体台站的地震动参数预测值。
![]()
图 7 9个代表性台站的加速度反应谱与基于单断层Ⅰ震源模型、单断层Ⅱ震源模型及共轭复合断层震源模型模拟的加速度反应谱、加速度反应谱衰减模型的对比Figure 7. Comparison of the recorded acceleration response spectra of 9 representative stations with the simulated acceleration response spectra from source fault Ⅰ model ，fault Ⅱ model and conjugated fault model and NGA-West2 attenuation models从图7可以看出：在高频段T＜1 s时基于三个震源模型的模拟值整体上高于实际记录的谱值，且与加速度反应谱衰减模型较为接近；而在低频段T ＞1 s时基于断层Ⅰ，Ⅱ及共轭震源模型的模拟值迅速减小至小于实际记录谱值，且低于加速度反应谱衰减模型。另外，对比距震源较远的台站实际记录加速度反应谱与基于断层Ⅰ，Ⅱ及共轭震源模型的计算值，可以发现基于这三个断层震源模型的加速度反应谱模拟计算结果都很相近，即基于不同震源的模拟值彼此之间差异不显著。对比距震源较近的台站实际记录加速度反应谱、基于不同断层震源模型的模拟值与加速度对比结果类似，断层Ⅰ和断层Ⅱ断层距相近的台站如53QQC等三个震源模型结果差异也不大，均与记录值和NGA-West2预测值符合较好，但对震源断层模型Ⅰ和断层模型Ⅱ来说，两个断层距相差较大的台站53LDC和53HYC，则呈现出差异性，具体为断层距较小的震源模型其模拟加速度反应谱更大，共轭震源模型模拟值则处于两个单断层模型之间，不同断层震源模型模拟值之间的差异仍在接受范围内。
3.3 速度时程
鲁甸地震为中等地震，所有台站的峰值速度PGV （记录和模拟）均小于10 cm/s。图8仅给出近震源区的台站53QQC，53LDC和53HYC速度记录及基于单断层Ⅰ震源模型、单断层Ⅱ震源模型及共轭复合断层震源模型模拟的速度时程，可以发现尽管53LDC台站模拟的峰值加速度大于实际记录的PGA，但模拟的速度时程与速度记录的波形接近。与加速度模拟结果类似，是因为台站与断层的特殊位置，53HYC台站的断层Ⅱ模型模拟值低于断层Ⅰ模型和共轭断层模型，后者更接近记录值。其它距震源较远台站的峰值速度都较小，但基于单断层Ⅰ震源模型、单断层Ⅱ震源模型及共轭复合断层震源模型模拟的速度时程彼此相近，无显著差异，且与实际记录吻合良好。限于篇幅，不一一列举。
![]()
图 8 台站53QQC （a）、53LDC （b）和53HYC （c）的记录和Figure 8. The recorded three component velocity time-histories of the stations 53QQC，53LDC and 53HYC and the simulated velocity time-histories of different source models （duration of 40 s）3.4 区域地震动空间分布
基于单断层Ⅰ震源模型、单断层Ⅱ震源模型及共轭复合断层震源模型模拟2014年鲁甸MW6.1地震动影响场，能进一步理解地震动影响作用的总体特征。将研究区域划分成0.15°×0.15°网格，计算441个场点的地震动时程。图9显示了单断层Ⅰ震源模型、单断层Ⅱ震源模型及共轭复合断层震源模型产生的PGA分布图，等值线由441个模拟点的水平几何平均值用自然邻点法插值计算而得；图9还分别给出单断层Ⅰ震源模型、单断层Ⅱ震源模型及共轭复合断层震源模型产生的PGV分布图，可以看出：在震源附近区域不同震源模型产生的峰值加速度、峰值速度有显著的差异；但在离震源较远的区域，不同震源模型产出的峰值加速度和峰值速度差异不明显。即地震矩相近的不同震源模型产生的地震动影响场的差异主要体现在近震源区，远场彼此差异不显著。
![]()
图 9 三个震源模型产生的水平向PGA （左）和PVG （右）分布Figure 9. Distributions of horizontal PGA （left） and PGV （right） generated from three source fault models图10分别是在周期T＝0.5 s和1.5 s时用断层Ⅰ、断层Ⅱ和共轭断层模型模拟的加速度反应谱（PSA）分布，由于震级中等，三个模型的反应谱随周期迅速衰减，从1.5 s周期的反应谱分布更能明显地看到断层几何参数对近场区域的主导作用。
![]()
图 10 三个震源模型模拟的加速度反应谱（PSA）在周期0.5 s （a）和1.5 s （b）时的分布Figure 10. Distributions of simulated pseudo-spectral acceleration （PSA） at period of 0.5 s （a） and 1.5 s （b） that from three source fault modelsPGA、PGV和各周期反应谱近断层分布主要受断层模型影响，各地震动参数分布形态类似，等值线基本呈沿着断层的包络形态分布，共轭断层模型的模拟地震动分布更接近陈鲲等（2015）给出的快速峰值加速度震动图。与中国地震局发布的云南鲁甸MS6.5 （MW6.1）地震烈度图对比也可以看出：基于共轭断层模型的模拟地震动参数分布与2014年云南鲁甸地震MW6.1宏观烈度分布的总体形态更趋一致。
4. 讨论与结论
从峰值加速度PGV、峰值速度PGV以及不同周期加速度反应谱PSA的分布上看，模拟的近断层地震动参数表现出很好的破裂方向性效应，两个单断层震源模型的地震动参数等值线分别按照其断层走向呈椭圆形衰减，超过一定距离后垂直和平行断层方向上的场点差异逐渐减小，可近似为圆形。因此，在模拟中强震近断层区域地震动时，不能忽略断层尺寸的影响。大部分台站加速度反应谱在周期2 s后有一个小幅上升的走势而后再随周期衰减，这与台站的场地低频放大效应有关，这在模拟的反应谱中也有所体现，而在此区间内NGA-WEST2预测反应谱呈周期单调衰减，说明考虑了具体场地条件相关参数的宽频带地震动模拟能捕捉到强地面运动细节和地震动参数特性，相比于一般的经验回归和统计模型有一定优势。基于张勇等（2015）反演给出2014年云南鲁甸地震MW6.1地震震源破裂模型和区域地震速度结构模型，借助宽频带混合地震动模拟方法，研究了震源破裂过程对宽频带地震动的影响。研究表明，不同震源破裂模型对地震动空间分布形态有显著影响。对于震源尺度有限的中等地震而言，不同震源破裂模型对地震动特性影响的差异，主要呈现在近震源区；离震源区较远的区域，不同破裂模型对地震动特性影响差异相对较小。另外，基于共轭断层模型的模拟结果与2014年云南鲁甸地震MW6.1宏观烈度分布的总体形态更趋一致。因此，精细的震源破裂模型对合理估计近震源区地震动峰值、频谱、持续时间及其地震动时程和近震源区的地震危险性具有重要价值。
本文台站数据资料来自国家强震动台网中心网站。美国南加州地震研究中心Graves Robert和Fabio Silva为本文提供了宽频带地震动模拟方法和技术支持，北京大学张勇教授对单断层模型和共轭断层模型的详细解析，中国地震局地球物理研究所房立华研究员为本文提供了速度结构和经验模型等数据，审稿专家对本文提出了有益的意见，作者在此一并表示感谢。
-
![]()
图 10 三个震源模型模拟的加速度反应谱（PSA）在周期0.5 s （a）和1.5 s （b）时的分布
Figure 10. Distributions of simulated pseudo-spectral acceleration （PSA） at period of 0.5 s （a） and 1.5 s （b） that from three source fault models
![]()
图 1 鲁甸地震研究区域内断层面投影及台站位置
Figure 1. Fault plane projection and station location of Ludian earthquake in the study region
![]()
图 2 断层Ⅰ（a）、断层Ⅱ（b）及共轭复合断层（c）的震源模型（张勇等，2015）
Figure 2. Geometry，source time function and final slip distributions of source faultⅠmodel （a），fault Ⅱ model （b） and conjugated fault model （c）（Zhang et al，2015）
![]()
图 3 鲁甸地区一维速度结构及介质密度模型
Figure 3. One-dimensional velocity structure and medium density model in Ludian region
![]()
图 4 台站53QQC （a）、53LDC （b）和53HYC （c）的加速度记录及基于断层Ⅰ震源模型、断层Ⅱ震源模型和共轭复合震源模型合成的三分量加速度时程（持续时间35 s）
Figure 4. Recordings and simulated three component acceleration time-histories of the representative stations 53QQC （a），53LDC （b） and 53HYC （c） from source faultⅠmodel，fault Ⅱ model and conjugated fault model （duration of 35 s）
![]()
图 5 台站53QJX （a）、51HDQ （b）和53HZX （c）的加速度记录及基于断层Ⅰ震源模型、断层Ⅱ震源模型和共轭复合震源模型合成的三分量加速度时程（持续时间35 s）
Figure 5. Recordings and simulated three component acceleration time-histories of the representative stations 53QJX （a），51HDQ （b） and 53HZX （c） from source faultⅠmodel，fault Ⅱ model and conjugated fault model （duration of 35 s）
![]()
图 6 台站51PGD （a）、53DTB （b）和51YBH （c）的加速度记录及基于断层Ⅰ震源模型、断层Ⅱ震源模型和共轭复合震源模型合成的三分量加速度时程（持续时间35 s）
Figure 6. Recordings and simulated three component acceleration time-histories of the representative stations 51PGD （a），53DTB （b） and 51YBH （c） from source faultⅠmodel，fault Ⅱ model and conjugated fault model （duration of 35 s）
![]()
图 7 9个代表性台站的加速度反应谱与基于单断层Ⅰ震源模型、单断层Ⅱ震源模型及共轭复合断层震源模型模拟的加速度反应谱、加速度反应谱衰减模型的对比
Figure 7. Comparison of the recorded acceleration response spectra of 9 representative stations with the simulated acceleration response spectra from source fault Ⅰ model ，fault Ⅱ model and conjugated fault model and NGA-West2 attenuation models
![]()
图 8 台站53QQC （a）、53LDC （b）和53HYC （c）的记录和
Figure 8. The recorded three component velocity time-histories of the stations 53QQC，53LDC and 53HYC and the simulated velocity time-histories of different source models （duration of 40 s）
![]()
图 9 三个震源模型产生的水平向PGA （左）和PVG （右）分布
Figure 9. Distributions of horizontal PGA （left） and PGV （right） generated from three source fault models
表 1 两个单断层模型及共轭复合断层震源模型参数（张勇，2015）
Table 1 Parameters of two single fault plane models and conjugated faults model （Zhang，2015）
震源模型 走向/° 倾向/° 长度/km 宽度/km 子断层尺寸 地震矩/（1018 N·m） MW 断层Ⅰ模型 162 70 21 10 2 km×2 km 1.79 6.13 断层Ⅱ模型 257 77 21 10 2 km×2 km 1.80 6.14 共轭断层模型 复合 复合 复合 复合 2 km×2 km 2.05 6.17 
下载: 导出CSV
表 2 震中距160 km内部分台站相关信息（顺序依震中距排列）
Table 2 List of relevant information of part discussed stations within 160 km of the epicenter （arranged according to the epicenter distance）
台站 东经/° 北纬/° 场地类型 震中距/km 断层Ⅰ断层距/km 断层Ⅱ断层距/km $ {v}_{\mathrm{S}30} $ 53QQC 103.23 26.94 土 19.09 14.77 13.24 527# 53LDC 103.60 27.22 岩 32.54 28.99 13.93 424 53HYC 103.51 26.81 土 38.38 17.26 33.52 497* 53QJX 103.24 35.75 土 39.37 24.44 33.97 527# 51HDQ 102.82 26.67 土 67.47 60.57 49.07 747 53HZX 103.31 26.41 土 76.73 57.60 72.24 318* 51PGD 102.54 27.37 土 80.92 69.31 65.85 688 53DTB 103.04 26.36 岩 86.24 70.21 75.09 760 51YBH 101.92 26.53 土 150.90 147.53 129.68 376 注：标*数据由Boor （2 004）的速度梯度延拓线性模型外推计算而得，标#数据根据邻近场地插值计算得到。
下载: 导出CSV
-
陈鲲,俞言祥,高孟潭,亢川川. 2015. 2014年云南鲁甸MS6.5地震峰值加速度震动图[J]. 地震学报,37(3):429–436. doi: 10.11939/j.issn:0253-3782.2015.03.006 Chen K,Yu Y X,Gao M T,Kang C C. 2015. Shakemap of peak ground acceleration for 2014 Ludian,Yunnan,MS6.5 earthquake[J]. Acta Seismologica Sinica,37(3):429–436 (in Chinese).
崔建文,刘琼仙,段建新,杨黎薇,高东,李世成,徐硕,林国良,张潜,包一峰,段洪杰. 2014. 2014年云南鲁甸6.5级地震强震动观测记录及初步分析[J]. 地震研究,37(4):542–548. doi: 10.3969/j.issn.1000-0666.2014.04.009 Cui J W,Liu Q X,Duan J X,Yang L W,Gao D,Li S C,Xu S,Lin G L,Zhang Q,Bao Y F,Duan H J. 2014. Strong-motion recordings of MS6.5 Ludian earthquake in Yunnan in 2014 and their preliminary analysis[J]. Journal of Seismological Research,37(4):542–548 (in Chinese).
房立华,吴建平,王未来,吕作勇,王长在,杨婷,钟世军. 2014. 云南鲁甸MS 6.5地震余震重定位及其发震构造[J]. 地震地质,36(4):1173–1185. doi: 10.3969/j.issn.0253-4967.2014.04.019 Fang L H,Wu J P,Wang W L,Lü Z Y,Wang C Z,Yang T,Zhong S J. 2014. Relocation of the aftershock sequence of the MS6.5 Ludian earthquake and its seismogenic structure[J]. Seismology and Geology,36(4):1173–1185 (in Chinese).
冀昆,温瑞智,崔建文,王宏伟,任叶飞. 2014. 鲁甸MS6.5 级地震强震动记录及震害分析[J]. 震灾防御技术,9(3):325–339. doi: 10.11899/zzfy20140301 Ji K,Wen R Z,Cui J W,Wang H W,Ren Y F. 2014. Observation of strong motion and damage investigation for MS6.5 Ludian earthquake[J]. Technology for Earthquake Disaster Prevention,9(3):325–339 (in Chinese).
刘成利,郑勇,熊熊,付芮,单斌,刁法启. 2014. 利用区域宽频带数据反演鲁甸MS6.5 级地震震源破裂过程[J]. 地球物理学报,57(9):3028–3037. doi: 10.6038/cjg20140927 Liu C L,Zheng Y,Xiong X,Fu R,Shan B,Diao F Q. 2014. Rupture process of MS6.5 Ludian earthquake constrained by regional broadband seismograms[J]. Chinese Journal of Geophysics,57(9):3028–3037 (in Chinese).
卢永坤,张建国,宋立军,代博洋,和嘉吉,庞卫东. 2014. 2014年云南鲁甸6.5级地震烈度分布与房屋震害特征[J]. 地震研究,37(4):549–557. doi: 10.3969/j.issn.1000-0666.2014.04.010 Lu Y K,Zhang J G,Song L J,Dai B Y,He J J,Pang W D. 2014. Analysis on intensity distribution and seismic disaster characteristics of building of Yunnan Ludian MS6.5 earthquake in 2014[J]. Journal of Seismological Research,37(4):549–557 (in Chinese).
王未来,吴建平,房立华,来贵娟. 2014. 2014年云南鲁甸MS6.5地震序列的双差定位[J]. 地球物理学报,57(9):3042–3051. Wang W L,Wu J P,Fang L H,Lai G J. 2014. Double difference location of the Ludian MS6.5 earthquake sequences in Yunnan Province in 2014[J]. Chinese Journal of Geophysics,57(9):3042–3051 (in Chinese).
魏勇,崔建文,王秋良,沈雨忆. 2018. 基于合成地震动的2014年鲁甸MS6.5地震场地效应分析[J]. 地震研究,41(1):32–37. doi: 10.3969/j.issn.1000-0666.2018.01.004 Wei Y,Cui J W,Wang Q L,Shen Y Y. 2018. Analysis on site effect of 2014 Yunnan Ludian MS6.5 earthquake based on simulating ground motion[J]. Journal of Seismological Research,41(1):32–37 (in Chinese).
许力生,张旭,严川,李春来. 2014. 基于勒夫波的鲁甸MS6.5地震震源复杂性分析[J]. 地球物理学报,57(9):3006–3017. doi: 10.6038/cjg20140925 Xu L S,Zhang X,Yan C,Li C L. 2014. Analysis of the Love waves for the source complexity of the Ludian MS6.5 earthquake[J]. Chinese Journal of Geophysics,57(9):3006–3017 (in Chinese).
徐涛,张明辉,田小波,郑勇,白志明,武澄泷,张忠杰,滕吉文. 2014. 丽江—清镇剖面上地壳速度结构及其与鲁甸MS6.5级地震孕震环境的关系[J]. 地球物理学报,57(9):3069–3079. doi: 10.6038/cjg20140932 Xu T,Zhang M H,Tian X B,Zheng Y,Bai Z M,Wu C L,Zhang Z J,Teng J W. 2014. Upper crustal velocity of Lijiang-Qingzhen profile and its relationship with the seismogenic environment of the MS6.5 Ludian earthquake[J]. Chinese Journal of Geophysics,57(9):3069–3079 (in Chinese).
徐锡伟,江国焰,于贵华,吴熙彦,张建国,李西. 2014. 鲁甸6.5级地震发震断层判定及其构造属性讨论[J]. 地球物理学报,57(9):3060–3068. doi: 10.6038/cjg20140931 Xu X W,Jiang G Y,Yu G H,Wu X Y,Zhang J G,Li X. 2014. Discussion on seismogenic fault of the Ludian MS6.5 earthquake and its tectonic attribution[J]. Chinese Journal of Geophysics,57(9):3060–3068 (in Chinese).
张斌,李小军,俞言祥,李娜,朱俊. 2020. 鲁甸地震强震动记录与地震动衰减模型的对比研究[J]. 地球物理学报,63(8):2999–3014. doi: 10.6038/cjg2020O0045 Zhang B,Li X J,Yu Y X,Li N,Zhu J. 2020. Comparison of strong ground motion records from Ludian,China,earthquake with ground-motion attenuation models[J]. Chinese Journal of Geophysics,63(8):2999–3014 (in Chinese).
张广伟,雷建设,梁姗姗,孙长青. 2014. 2014年8月3日云南鲁甸MS6.5级地震序列重定位与震源机制研究[J]. 地球物理学报,57(9):3018–3027. doi: 10.6038/cjg20140926 Zhang G W,Lei J S,Liang S S,Sun C Q. 2014. Relocations and focal mechanism solutions of the 3 August 2014 Ludian,Yunnan MS6.5 earthquake sequence[J]. Chinese Journal of Geophysics,57(9):3018–3027 (in Chinese).
张勇,陈运泰,许力生,魏星,金明培,张森. 2015. 2014 年云南鲁甸 MW6.1地震:一次共轭破裂地震[J]. 地球物理学报,58(1):153–162. doi: 10.6038/cjg20150113 Zhang Y,Chen Y T,Xu L S,Wei X,Jin M P,Zhang S. 2015. The 2014 MW6.1 Ludian,Yunnan,earthquake:A complex conjugated ruptured earthquake[J]. Chinese Journal of Geophysics,58(1):153–162 (in Chinese).
张振国,孙耀充,徐建宽,张伟,陈晓非. 2014. 2014年8月3日云南鲁甸地震强地面运动初步模拟及烈度预测[J]. 地球物理学报,57(9):3038–3041. doi: 10.6038/cjg20140928 Zhang Z G,Sun Y C,Xu J K,Zhang W,Chen X F. 2014. Preliminary simulation of strong ground motion for Ludian,Yunnan earthquake of 3 August 2014,and hazard implication[J]. Chinese Journal of Geophysics,57(9):3038–3041 (in Chinese).
Abrahamson N A,Silva W J,Kamai R. 2014. Summary of the ASK14 ground motion relation for active crustal regions[J]. Earthq Spectra,30(3):1025–1055. doi: 10.1193/070913EQS198M
Anderson J G,Hough S E. 1984. A model for the shape of the fourier amplitude spectrum of acceleration at high frequencies[J]. Bull Seismol Soc Am,74(5):1969–1993.
Boore D M. 1983. Stochastic simulation of high-frequency ground motions based on seismological models of the radiated spectra[J]. Bull Seismol Soc Am,73(6A):1865–1894.
Boore D M,Joyner W B. 1997. Site amplifications for generic rock sites[J]. Bull Seismol Soc Am,87(2):327–341. doi: 10.1785/BSSA0870020327
Boore D M. 2003. Simulation of ground motion using the stochastic method[J]. Pure Appl Geophys,160(3/4):635–676.
Boore D M. 2004. Estimating v̄S(30) (or NEHRP site classes) from shallow velocity models (depths < 30 m)[J]. Bull Seismol Soc Am,94(2):591–597. doi: 10.1785/0120030105
Boore D M,Stewart J P,Seyhan E,Atkinson G M. 2014. NGA-West2 equations for predicting PGA,PGV,and 5% damped PSA for shallow crustal earthquakes[J]. Earthq Spectra,30(3):1057–1085. doi: 10.1193/070113EQS184M
Brune J N. 1970. Tectonic stress and the spectra of seismic shear waves from earthquakes[J]. J Geophys Res,75(26):4997–5009. doi: 10.1029/JB075i026p04997
Campbell K W,Bozorgnia Y. 2014. NGA-West2 ground motion model for the average horizontal components of PGA,PGV,and 5% damped linear acceleration response spectra[J]. Earthq Spectra,30(3):1087–1115. doi: 10.1193/062913EQS175M
Chiou B S J,Youngs R R. 2014. Update of the Chiou and Youngs NGA model for the average horizontal component of peak ground motion and response spectra[J]. Earthq Spectra,30(3):1117–1153. doi: 10.1193/072813EQS219M
Frankel A. 1998. Simulating strong motions of large earthquakes using recordings of small earthquakes:The Loma Prieta mainshock as a test case[J]. Bull Seismol Soc Am,85(4):1144–1160.
Graves R W. 1996. Simulating seismic wave propagation in 3D elastic media using staggered-grid finite differences[J]. Bull Seismol Soc Am,86(4):1091–1106.
Graves R W,Pitarka A. 2010. Broadband ground-motion simulation using a hybrid approach[J]. Bull Seismol Soc Am,100(5A):2095–2123. doi: 10.1785/0120100057
Hu J J,Zhang Q,Jiang Z J,Xie L L,Zhou B F. 2016. Characteristics of strong ground motions in the 2014 MS6.5 Ludian earthquake,Yunnan,China[J]. J Seismol,20(1):361–373. doi: 10.1007/s10950-015-9532-x
Ou G B,Herrmann R B. 1990. A statistical model for ground motion produced by earthquakes at local and regional distances[J]. Bull Seismol Soc Am,80(6A):1397–1417. doi: 10.1785/BSSA08006A1397
Walling M,Silva W,Abrahamson N. 2008. Nonlinear site amplification factors for constraining the NGA models[J]. Earthq Spectra,24(1):243–255. doi: 10.1193/1.2934350
Wang H W,Wen R Z. 2019. Ground-motion simulation for the MW6.1 Ludian earthquake on 3 August 2014 using the stochastic finite-fault method[J]. Earthquake Science,32(3/4):101–114.
Yu Y, Silva W J, Darragh B, Li X J. 2016. vS30 estimate for Southwest China[J]. Int J Geophys: 9305095.
计量
- 文章访问数: 339
- HTML全文浏览量: 99
- PDF下载量: 147
