Numerical modelling of sloshing responses in a cylindrical tank under seismic excitations
-
摘要:
为了研究地震载荷下圆柱型储罐内液体的晃荡特性，选用15种典型的地震信号，采用计算流体力学软件Fluent进行数值仿真，以探究地震的频率、频率成分、峰值速度以及峰值加速度对晃荡波高和水动压的作用规律。结果表明：① 地震主频是影响自由液面响应的主要因素之一，当其接近储液的一阶固有频率时，会激发强烈的非线性晃荡现象，工程中应添加减晃装置；② 波高与地震峰值速度呈较强的正相关，并且低频成分的地震信号激发的波浪较其它频率成分的地震信号更为剧烈；③ 水动压在储罐上部呈对流模式分布，主要受地震主频和频率成分的影响，并与地震峰值速度和频率成分呈正相关；④ 水动压在储罐中、下部为脉冲模式分布，与地震峰值加速度呈线性正相关且下部的水动压增长速率明显大于中部。因此在抗震设计中，应加强罐壁下部的强度，尤其是峰值加速度较大的储罐放置场地。
Abstract:China is a country with frequent and high-intensity earthquakes and has arranged a large amount of storage tanks to conserve liquid materials, which can cause violent liquid sloshing in tanks, resulting in wall buckling, roof breaking and overflow. To comprehensively investigate the sloshing characteristics in cylindrical tanks under seismic excitations, seismic parameters covering a broad range of seismic frequency, frequency content, peak ground velocity （PGV） and peak ground acceleration （PGA）, which were four main concerns of seismic excitations, need to be traversed. Then, totally 12 seismic events involving 15 kinds of seismic records at home and abroad were selected to explore as much as possible about the key factors affecting the sloshing responses. The seismic frequency ranged from 0.11 Hz to 2.545 Hz, which covered the several natural frequencies of the fluid. The frequency content of all seismic records included the low frequency, intermediate frequency and high frequency. PGA increased from 0.081g to 1.79g, and PGV ranged from 0.22 m/s to 1.76 m/s. Numerical simulations, which could avoid the constraints of the theoretical assumption and experimental facility in prototype tanks, were carried out by using the computational fluid dynamics software Fluent to investigate the effects of seismic frequency, frequency content, PGV and PGA on the sloshing height and hydrodynamic pressure. After full validations against available numerical and experimental results in literatures, systematic simulations were carried out. The results suggest that: ① the dominant frequency of the seismic excitation is one main factor affecting the free-surface response, when it is close to the first-order natural frequency of the liquid and meantime lasts a longer duration, a strong non-linear phenomenon occurs, thereby the inhibition devices should be introduced in application; ② the wave height and the PGV exhibit a strong positive correlation in most cases; and the low-frequency content can excite more intense sloshing wave than those with other frequency contents, since the frequency content is a representative index to identify the overall situation of the seismic frequency; ③ the hydrodynamic pressure in the upper part of the tank shows a convective mode, namely the dominant response frequency of the pressure is the natural frequency of the fluid and the secondary response frequencies are the seismic frequencies, which is mainly affected by the dominant frequency and frequency content, and is also positively correlated with the PGV and frequency content; ④ the hydrodynamic pressures in the middle and lower parts are linearly and positively correlated with the PGA and remain pulse-like which is extremely obvious in the lower parts since the impulsive mode is dominated in the lower parts, and the growth rate in the lower part is also significantly higher than that in the middle part. Thereby, to ensure the tank safety, in the seismic design, the lower part of the tank sidewall should be strengthened especially in site conditions with potential strong PGA.
-
引言
圆柱型储罐因其具有容量大、用钢量少、占地面积小、结构和施工简单等优点已成为我国战略储备石油的主要设备。我国地震频发且烈度高，储罐在其作用下会随机运动，因而诱发流体剧烈的非线性晃荡（孙颖，2012），伴随的强烈抨击荷载和倾覆力矩影响着储罐的在位稳定性，储罐一旦破损，不仅会造成经济损失，也会带来严重的次生灾害，如环境污染和火灾事故（方浩等，2012）。因此，厘清地震载荷下储罐内流体的水动力特性对储罐的安全分析与结构优化设计具有重要的工程价值。
Virella等（2006）通过附加质量法分析了水平简谐载荷下圆柱型储罐内液体晃荡的脉冲模式，Chen等（2007）对圆柱型储罐在简谐载荷与地震载荷下的液体晃荡现象进行了数值研究和试验研究，其结果表明当外部载荷的主频接近于储液的固有频率时，外部载荷会诱发流体共振。杨杰等（2016）使用附加质量法对水平地震载荷下的大型渡槽中的流体非线性晃荡进行了研究。Caron等（2018）通过物理模型试验并结合数值模拟研究了圆柱型储罐在共振简谐载荷下自由液面的晃动情况。周利剑等（2019）通过物理模型试验与弹簧-质量法探究了地震频率对圆柱型储罐中流体运动的影响，其结果表明长周期地震引起的波高明显大于其它类型地震，且晃荡模态为多阶振型的叠加。Cheng等（2019）以及Hejazi和Mohammadi （2019）分别基于势流理论和物理模型实验分析了地震载荷下的液体晃荡现象，结果表明影响晃荡波高的主导因素是地震峰值加速度（peak ground acceleration，缩写为PGA）和主要频率。罗东雨等（2020）应用有限元软件ADINA研究16×104 m3液化天然气（liquefied natural gas，缩写为LNG）储罐在地震载荷下的响应，其结果显示地震频率是影响储液晃荡的主要因素。Hernandez-Hernandez等（2021）试验研究了PGA对储罐内流体晃荡的影响，其结果显示，最大环向应力与PGA线性相关。Jin等（2021）基于Navier-Stokes数值模型研究了天然地震对晃荡波高的影响规律，发现地震峰值速度（peak ground velocity，缩写为PGV）对峰值波高起着决定性作用。尽管地震是多向耦合的随机振动，但部分研究显示竖向地震对液体晃荡的影响并不显著（刘勇，2007；陈贵清等，2012；张如林等，2017）。
上述研究主要针对简谐共振或低频地震载荷下的液体晃荡现象，且多以附加质量法和弹簧-质量法来分析流固耦合问题，关于地震频率、PGA与PGV等因素对晃荡响应的影响规律还有待进一步揭示。为此，本研究拟采用计算流体力学软件Fluent对流体晃荡进行数值模拟，建立储罐与液体的耦合模型，选用包括低、中、高频在内的15种地震信号，探究地震信号的频率、PGA和PGV对储液的晃荡模式以及不同位置水动力特性的影响规律，以期为大型储罐的优化设计提供一定的参考。
1. 数值模型与验证
本研究采用Fluent进行数值模拟研究。流体在地震载荷下的晃荡通过多参考系法（multiple reference frame，缩写为MRF）实现。假设储液不可压，罐壁为刚性薄壁（Luo et al，2016），选用流体体积法（volume of fluid，缩写为VOF）追踪自由液面（Hirt，Nichols，1981）。
1.1 控制方程
不可压流动由连续性方程和Navier-Stokes方程描述，具体如下：
$$ \nabla \, {\bullet} \,\, {\boldsymbol{u}}＝0 \text{，} $$ (1) $$\frac{\partial \boldsymbol{u}}{\partial t} ＋ \nabla \, {\bullet} \,\, （ \boldsymbol{u} \boldsymbol{u} ） ＝－\frac{1}{\rho} \nabla p ＋ \nabla \, {\bullet} \,\, \left[\mu\left(\nabla \boldsymbol{u} ＋ （ \nabla \boldsymbol{u} ） ^{\mathrm{T}}\right) \right] ＋ f_i \text{，} $$ (2) 式中：ρ为流体密度；$\nabla $为哈密顿算子；速度矢量u＝$ ［ $u， v， w$ ］ $，其中u，v和w分别为液体在x，y和z方向上的速度分量；p为压强；μ为运动黏度；fi为单位体积流体所受到的体积力。选用多参考系法实现储罐内流体晃荡模块，控制方程可参照Shu等（2018）。
自由液面采用流体体积法进行追踪，通过求解以下通量方程来实现：
$$\begin{gathered}\frac{\partial \alpha_{\mathrm{w}}}{\partial t} ＋ \boldsymbol{U} \, {\bullet} \,\, \nabla \alpha_{\mathrm{w}}＝0 \text{，} \alpha_{\mathrm{a}}＋\alpha_{\mathrm{w}}＝1 \text{，} \end{gathered}$$ (3) 式中，αa和αw分别为控制体中空气和水的体积分数，αa＝1表示单元内为空气，αw＝1表示单元内为水，0＜αa＜1表示单元内为水和空气。
1.2 数值模型准确性验证
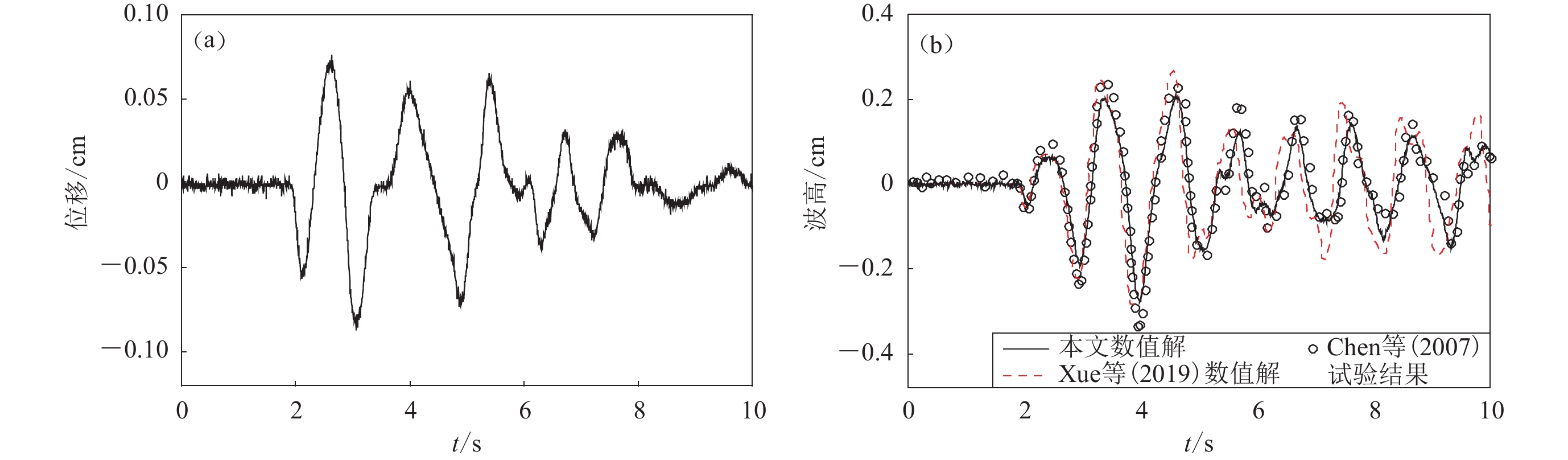
Chen等（2007）和Xue等（2019）研究了1995年神户M7地震载荷（图1a）下的流体晃荡现象，其中圆柱型储罐的尺寸为：半径R＝30 cm，静水水深H＝10 cm。本文对该工况进行数值模拟。图1b给出了罐壁右侧波高的试验结果（Chen et al，2007）和数值解（Xue et al，2019）与本文数值解的对比。尽管本文数值解与Xue等（2019）的数值解、Chen等（2007）的试验结果在峰值处有差异，但最大误差不超过12.4%，三者间几乎没有相位差，整体吻合得较好，说明本文所采用的数值方法具有较高的精度，可用于后续研究。
![]()
图 1 1995年神户M7地震的位移时程（a）以及神户地震载荷下罐壁右侧波高的时程曲线对比（b）Figure 1. Time history of ground displacement of Kobe seismic excitation （a） and comparison of time histories of wave heights at the right sidewall of the cylindrical tank under 1997 M7 Kobe seismic excitation （b）2. 地震的选取
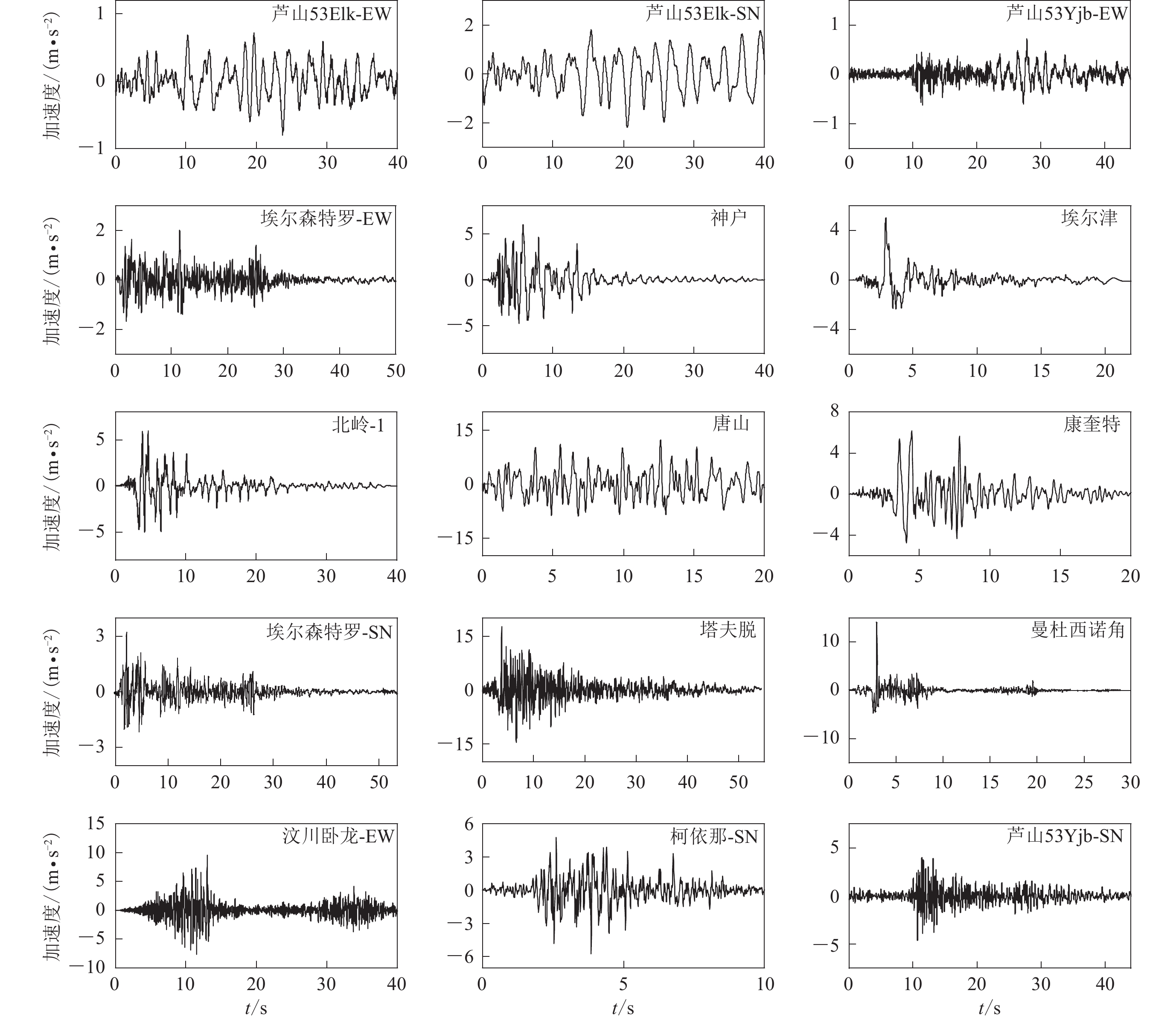
本研究选取12个地震事件，涉及15种地震信号，相应的地震参数列于表1，相应的加速度时程如图2所示。PGA从0.081g增长到1.79g；PGV最低为0.22 m/s，最高可达1.76 m/s。地震的频率成分根据PGA与PGV的比值划分为低频、中频与高频等三大类（Tso et al，1992；Kianoush，Ghaemmaghami，2011）：PGA/PGV＞1.2为高频，0.8＜PGA/PGV＜1.2为中频，PGA/PGV＜0.8为低频。因此，低频、中频和高频地震分别有7、5和3个。
表 1 本研究涉及地震的相关参数Table 1. The characteristics of considered seismic excitations in this study地震信号 频率类别 主要频率/Hz PGA/g PGV/（m·s−1） PGA/PGV 芦山53Elk-EW 低频 0.425，0.625 0.081 0.377 0.215 芦山53Elk-SN 低频 0.325，0.4 0.224 0.866 0.259 芦山53Yjb-EW 低频 0.658，0.703 0.075 0.232 0.323 埃尔森特罗（El Centro）-EW 低频 0.449，0.842 0.214 0.48 0.446 神户（Kobe） 低频 0.269，0.537，0.879 0.611 1.27 0.48 埃尔津（Erzican） 低频 0.42，0.563，0.798 0.52 0.84 0.619 北岭-1（Northridge-1） 低频 0.353，0.873，1.08 0.607 0.8 0.754 唐山 中频 0.65，0.8，1.2 1.268 1.584 0.8 康奎特（Concrete） 中频 0.49，0.6，0.98，1.1 0.63 0.758 0.83 埃尔森特罗（El Centro）-SN 中频 0.561，0.839，1.16 0.349 0.38 0.91 塔夫脱（Taft） 中频 0.11，0.37，0.61，1.2 1.79 1.76 1.02 曼杜西诺角（Cape Mendocino） 中频 0.868，1.43，2.14 1.45 1.26 1.15 汶川卧龙-EW 高频 0.622，1.38，2.35 0.97 0.585 1.66 柯依那（Koyna）-SN 高频 0.87，1.55 0.585 0.31 1.89 芦山53Yjb-SN 高频 0.841，2.545 0.473 0.22 2.15 注：第一列中的53Elk，53Yjb和卧龙为站点名称，EW和SN为地震信号的东西分量和南北分量。 ![]()
图 2 本文选取地震的加速度时程曲线Figure 2. Time histories of considered seismic accelerations used in this study3. 地震载荷下的晃荡特性
本节对地震载荷下圆柱型储罐内的晃荡模式和水动力特性进行讨论。液体的一阶固有频率满足如下公式（Chen et al，2007）：
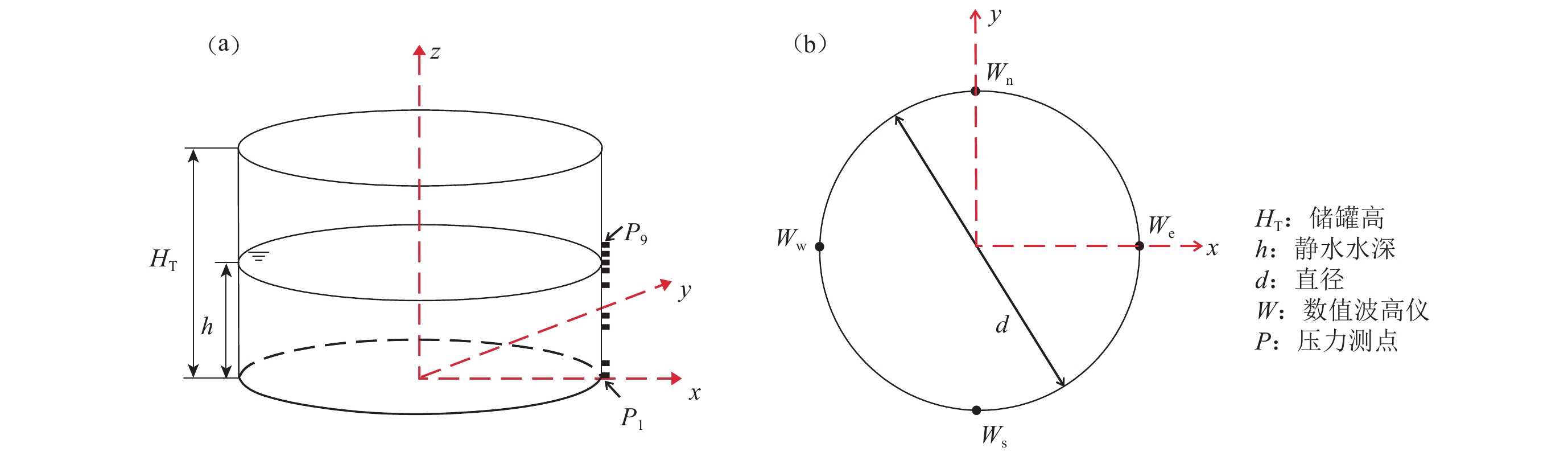
$$\begin{split} \omega_1＝\sqrt{\frac{2 \lambda_1 g}{d} \tanh \left(\lambda_1 \frac{h}{R}\right)} \text{，} \end{split}$$ (4) 式中：$\lambda_1 $为一阶贝塞尔函数的一阶导数，$\lambda_1 $＝1.841。本文中，储罐高HT＝15 m，直径d＝15 m，静水水深h＝10 m （Djermane et al，2014）。根据式（4）计算出ω1＝1.54 rad/s。储罐东侧布置9个压力测点，标记为P1，P2，···，P9 （分别距底部0 m，1 m，5 m，6 m，8 m，9.5 m，10 m，10.5 m，11 m）；四只数值波高仪排布在罐体的东、南、西、北四个方向，分别标记为We，Ws，Ww和Wn，如图3所示。
![]()
图 3 圆柱型储罐的整体视图（a）和顶视图（b）Figure 3. The overall-view （a） and top-view （b） geometries of the cylindrical tank3.1 网格无关性验证
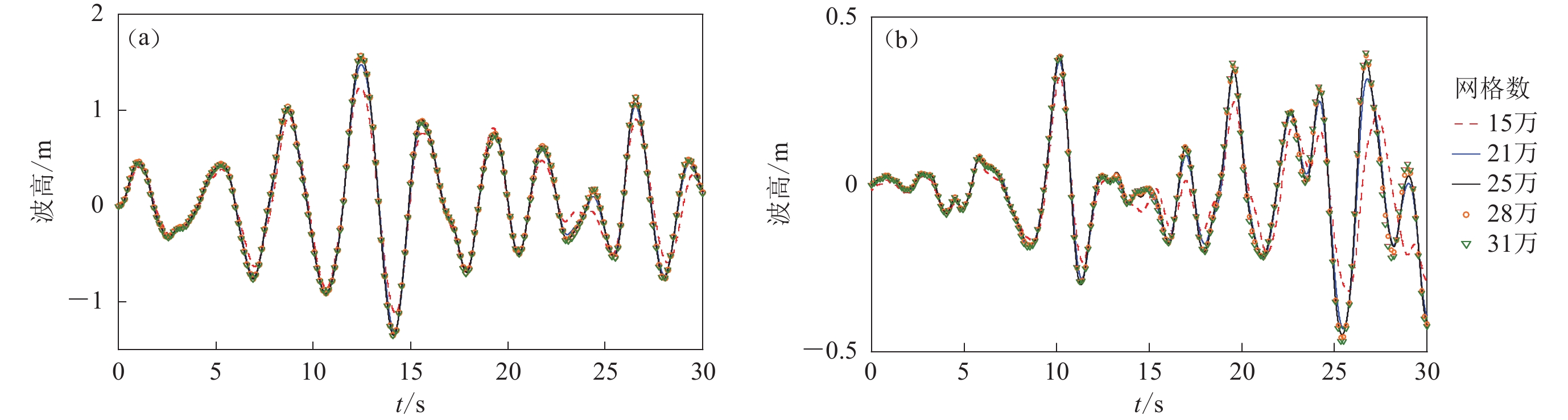
基于网格法的数值仿真需要评估网格数对计算精度的影响。本文选用15万、21万、25万、28万和31万等五种网格数进行网格无关性验证。图4给出了芦山53Elk-SN载荷下，不同网格数下东侧（We）和北侧（Wn）处波高的对比。可见：当网格数由15万增至25万时，波高略微增大，进一步增至31万时，波高曲线几乎重合。综合考虑计算效率与精度，本文选取25万网格数开展后续研究。
![]()
图 4 芦山53Elk-SN载荷下储罐东侧We （a）和北侧Wn （b）处不同网格数下波高的对比Figure 4. Comparison of time histories of the wave heights at We （a） and Wn （b） from various grids under Lushan 53Elk SN excitation3.2 模拟结果与讨论
本节重点分析地震频率、PGV和PGA对晃荡波高、水动压的影响。
3.2.1 晃荡波高
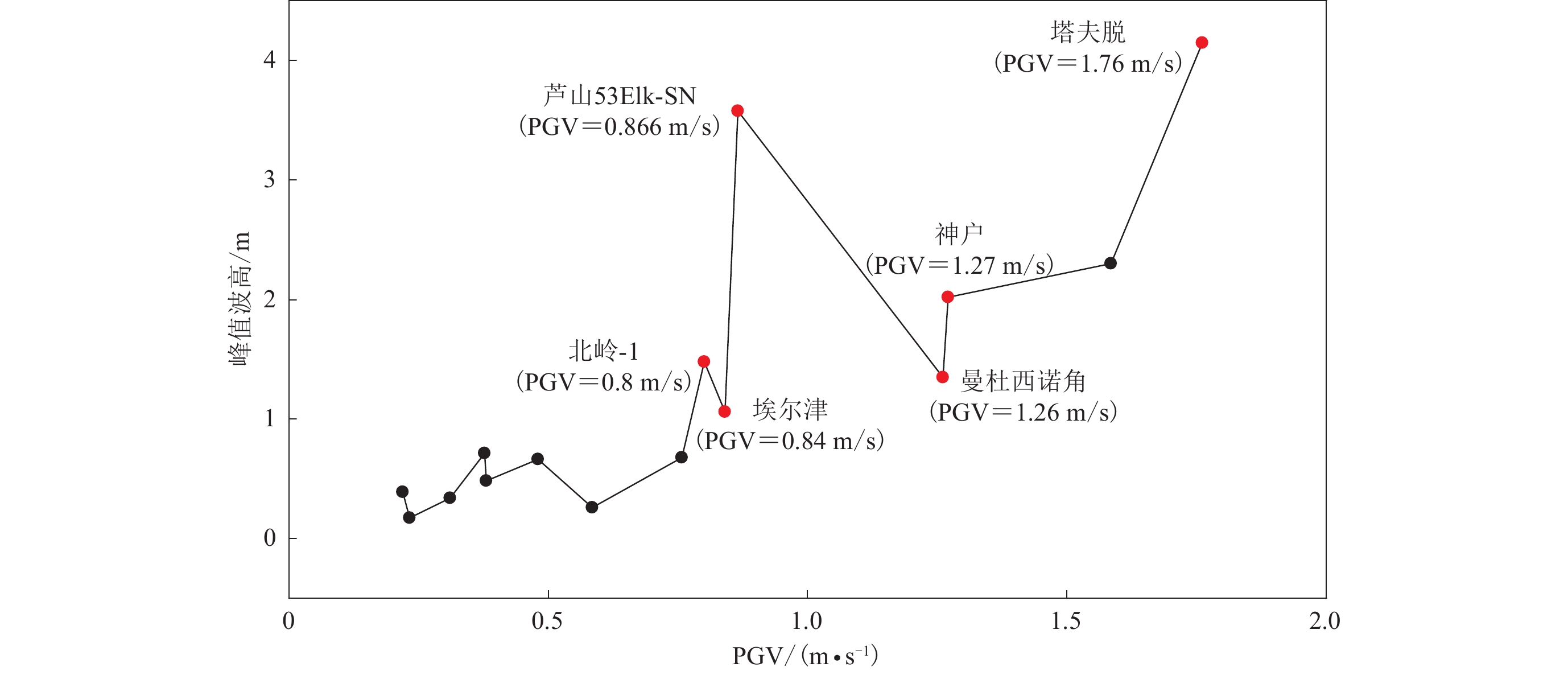
不同地震载荷下晃荡波高的时程曲线如图5所示。主频接近储液固有频率的地震信号会引发共振晃荡（张如林等，2017；Jin et al，2021）。例如：芦山53Elk-SN、神户以及北岭-1地震信号的主频分别为0.325 Hz，0.269 Hz和0.353 Hz，接近于储液的一阶固有频率（0.245 Hz），相应的波高时程如图5b，e，g所示。从该图可见，三个工况均为共振波且远大于非共振工况下的波高。15种工况下地震的主频、PGV和东侧（We）处相应的峰值波高列于表2。一般情况下，中、高频地震载荷引起的晃荡波高小于低频地震引起的波高，但也存在一些特例。例如：低频的芦山53Yjb-EW诱发的峰值波高为0.17 m，而主频较高的低频的埃尔津地震和中频的曼杜西诺角地震却激发了1.06 m和1.35 m的波高，分别为前者的6.2倍和8倍。由表1可知，虽然芦山53Yjb-EW的主频低，但其PGV仅为0.232 m/s，而埃尔津和曼杜西诺角的PGV却达到0.84 m/s和1.26 m/s，分别为前者的3.6倍和5.4倍。由此可见，PGV对晃荡波高的影响不可忽略，有必要对地震主频和PGV对晃荡波高的影响进一步分析。
![]()
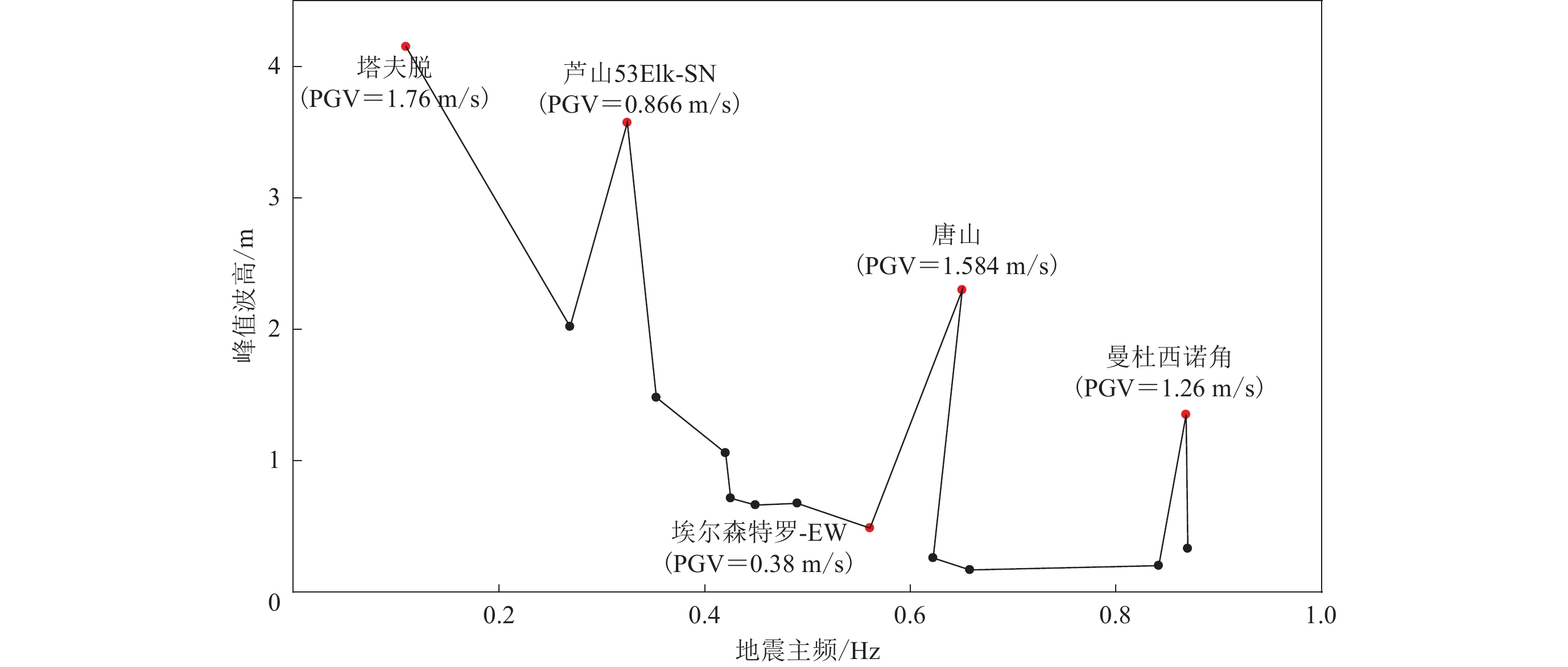
图 5 不同地震载荷下储罐东侧We和北侧Wn处的波高时程曲线（a） 芦山53Elk-EW；（b） 芦山53Elk-SN；（c） 芦山53Yjb-EW；（d） 埃尔森特罗-EW；（e） 神户地震；（f） 埃尔津；（g） 北岭-1；（h） 唐山；（i） 康奎特；（j） 埃尔森特罗-SN；（k） 塔夫脱；（l） 曼杜西诺角；（m） 汶川卧龙-EW；（n） 柯依娜-SN；（o） 芦山53Yjb-SNFigure 5. Time histories of wave heights under various seismic excitations（a） Lushan53Elk-EW；（b） Lushan53Elk-SN；（c） Lushan53Yjb-EW；（d） El Centro-EW；（e） Kobe；（f） Erzican；（g） Northridge-1；（h） Tangshan；（i） Concrete；（j） El Centro-SN；（k） Taft；（l） Cape Mendocino；（m） WenchuanWolong-EW；（n） Koyna-SN；（o） Lushan53Yjb-SN表 2 地震主频、PGV和东侧We处相应的峰值波高Table 2. The dominant frequency and PGV of seismic excitations and corresponding maximum wave heights at east side We of the tank地震信号 主频/Hz PGV/（m·s−1） 峰值波高/m 地震信号 主频/Hz PGV/（m·s−1） 峰值波高/m 塔夫脱 0.11 1.76 4.15 埃尔森特罗-SN 0.561 0.38 0.484 神户 0.269 1.27 2.02 汶川卧龙-EW 0.622 0.585 0.26 芦山53Elk-SN 0.325 0.866 3.574 唐山 0.65 1.584 2.3 北岭-1 0.353 0.8 1.48 芦山53Yjb-EW 0.658 0.232 0.17 埃尔津 0.42 0.84 1.06 芦山53Elk-SN 0.841 0.22 0.39 芦山53Elk-EW 0.425 0.377 0.713 曼杜西诺角 0.868 1.26 1.35 埃尔森特罗-EW 0.449 0.48 0.664 柯依那-SN 0.87 0.31 0.334 康奎特 0.49 0.758 0.675 峰值波高与地震主频的关系如图6所示。不难发现，峰值波高随地震主频的升高逐渐减小，但也有例外。例如：芦山53Elk-SN地震，其主频接近储液一阶固有频率，共振晃荡引起较大的波高，尽管其PGV仅为塔夫脱地震的一半，但其波高仍接近塔夫脱地震激发的波高；主频较低的埃尔森特罗-SN激发了0.484 m的波高，而主频较高的曼杜西诺角地震和唐山地震却诱发了1.35 m和2.3 m的波高，为前者的2.79倍和4.75倍，主要原因是：尽管曼杜西诺角地震和唐山地震的主频高于埃尔森特罗-SN，但二者的PGV较大，分别是埃尔森特罗-SN的3.32倍和4.17倍。因此，PGV也是影响晃荡的主要因素之一。
![]()
图 6 储罐东侧We处峰值波高与地震主频的关系Figure 6. The relationship between the maximum wave height at east side We of the tank and dominant frequency of earthquake储罐东侧We处的峰值波高与PGV的关系如图7所示。整体而言，峰值波高随PGV的增大而上升，二者呈正相关，如具有最大PGV的中频塔夫脱地震，激发的晃荡波最剧烈，波高可达4.15 m。当地震载荷的PGV相近时，主频低的地震诱发的波高较大，如：北岭-1地震与埃尔津地震的PGV相近，分别为0.8 m/s和0.84 m/s，由于二者的主频分别为0.353 Hz和0.563 Hz，差异明显，主频低的北岭-1激发的峰值波高（1.48 m）为埃尔津地震（1.06 m）的1.4倍。尽管神户地震的PGV （1.27 m/s）与曼杜西诺角的PGV （1.26 m/s）相近，二者引起的峰值波高分别为2.02 m和1.35 m，前者为后者的1.5倍，这主要源于其主频差异明显，分别为0.269 Hz和0.868 Hz。
![]()
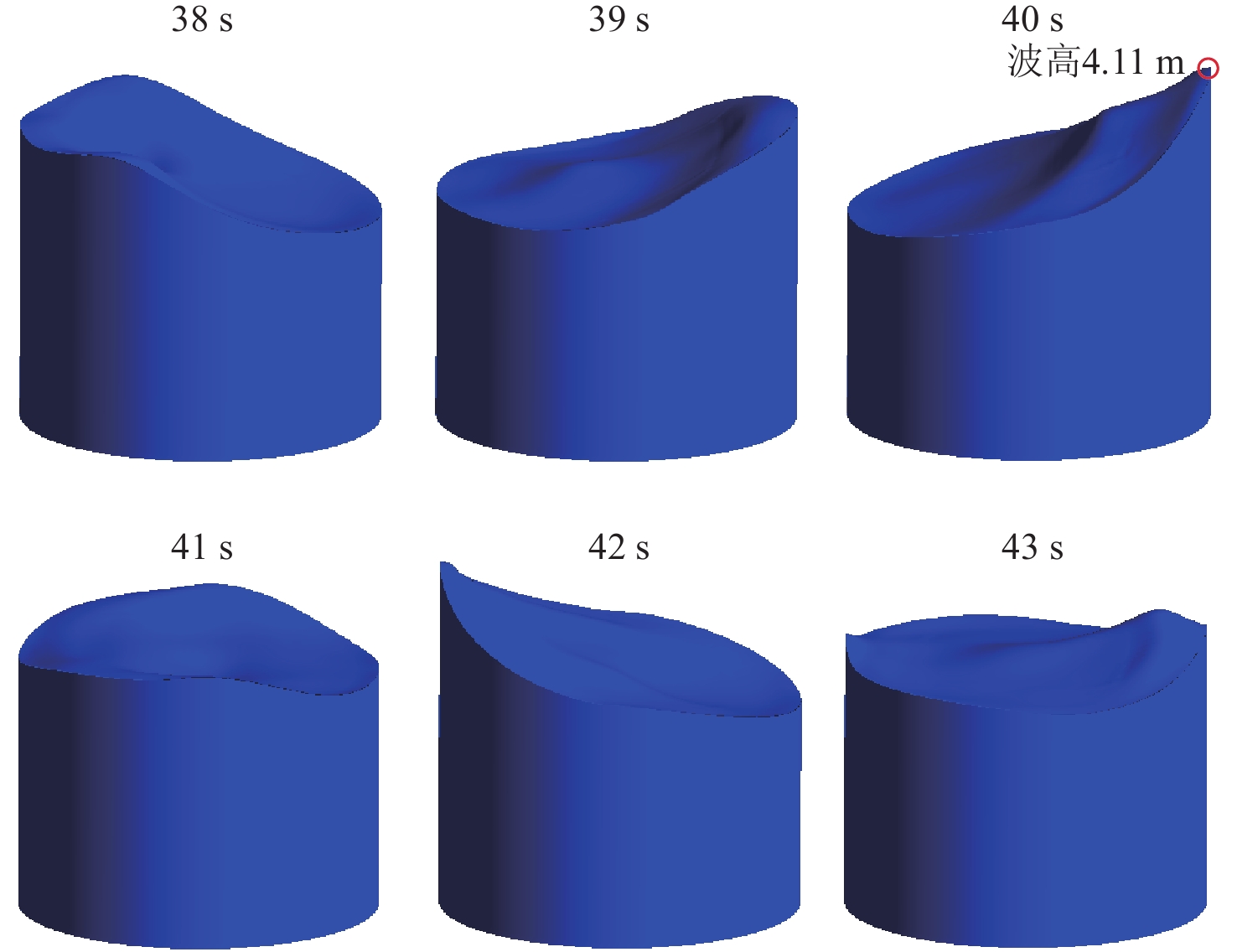
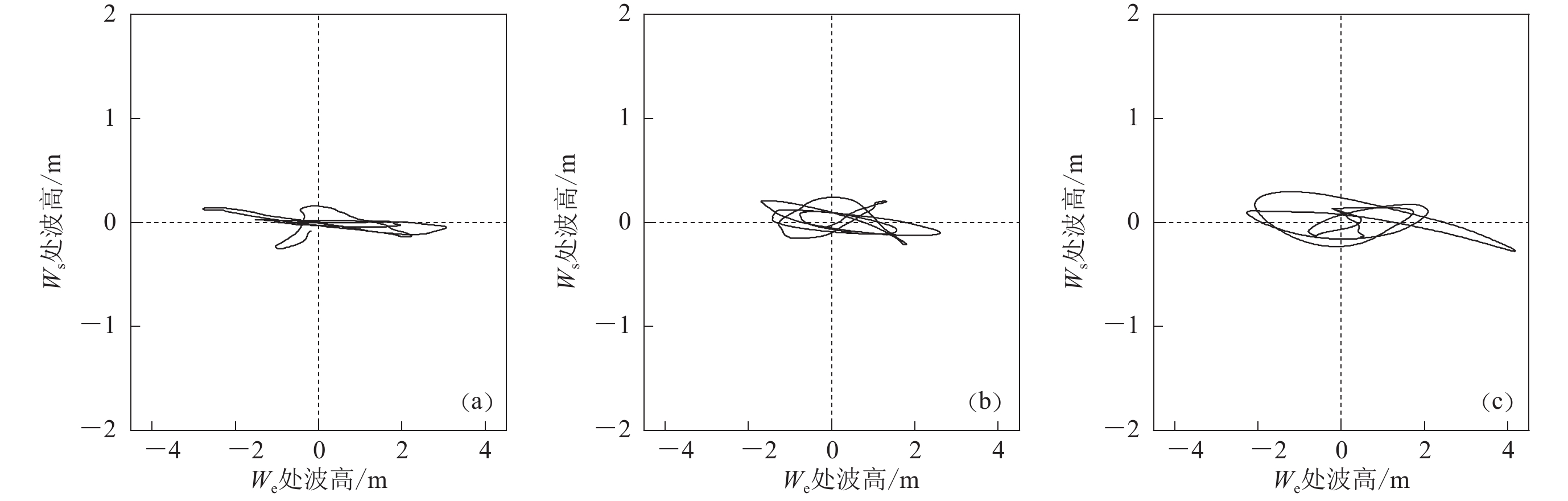
图 7 储罐东侧We处峰值波高与PGV的关系Figure 7. The relationship between the maximum wave height at the east side We of the tank and PGV塔夫脱地震的主频（0.11 Hz）偏离储液的固有频率，塔夫脱地震载荷下部分时刻的自由液面如图8所示，可以看出：液面晃荡幅度大，但未出现波浪破碎、液体飞溅等强非线性行为，形成驻波的波面比较光滑，在40.0 s时刻，爬升至最高处。塔夫脱地震载荷下自由液面的波高相位如图9所示，可见：自由液面位移在载荷方向上变化明显，在非载荷方向上并不显著；在0 s至15 s时段（图9a），Ws处的自由液面位移与We处结果呈线性关系，即正向与负向的振幅几乎一致，储液沿外部载荷方向运动，表现为平面波（Faltinsen et al，2003）；在15 s至45 s时段（图9b，c），自由液面呈非线性关系，不对称性加剧，正向振幅接近负向振幅的两倍。
![]()
图 8 塔夫脱地震载荷下的自由液面Figure 8. Free surface snapshots under excitation of Taft earthquake![]()
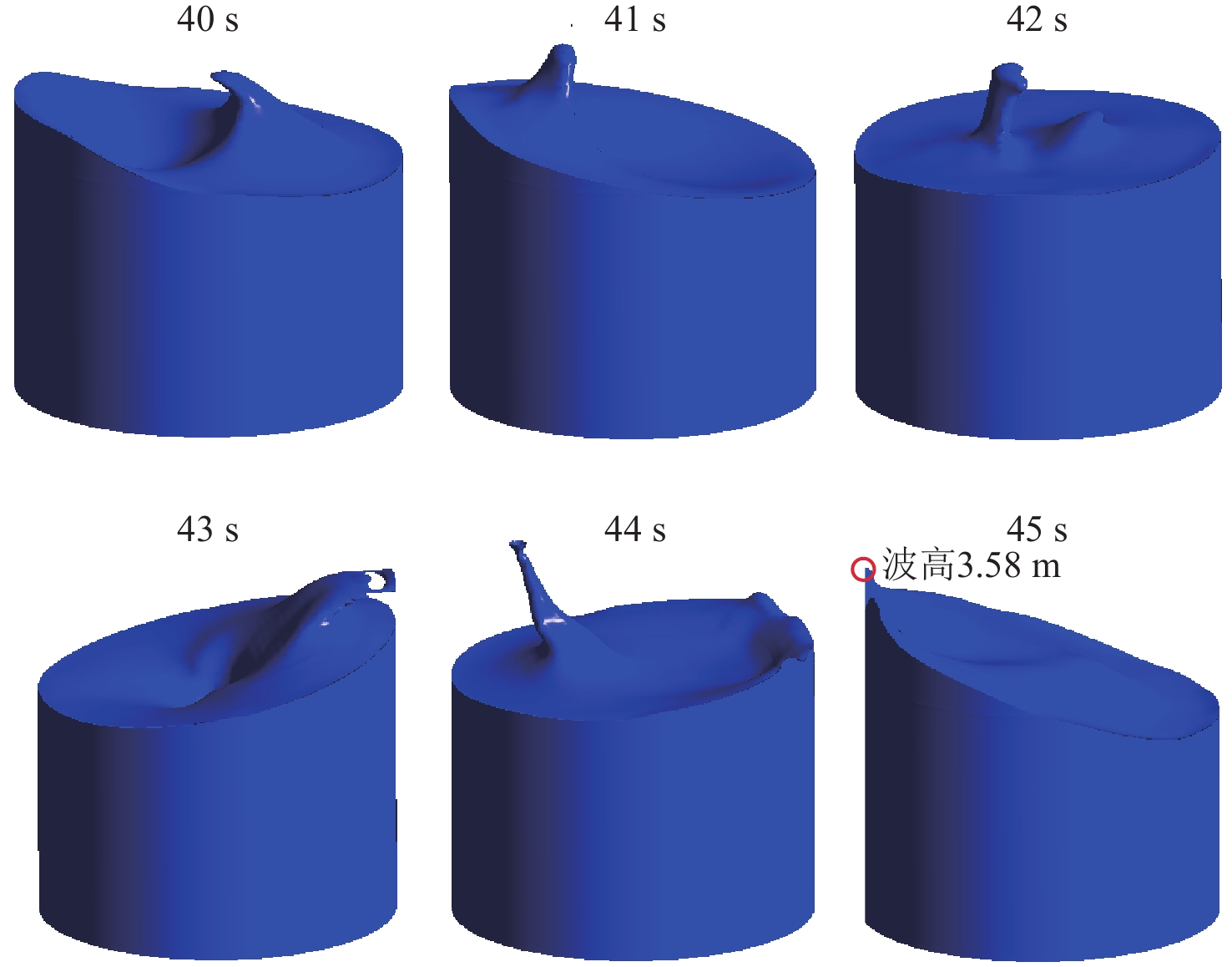
图 9 塔夫脱地震载荷下自由液面的波高相位图Figure 9. Phase diagrams of wave heights under excitation of Taft earthquake（a） 0—15 s；（b） 15—30 s；（c） 20—45 s多数工况符合上述规律，但也存在特例，如芦山53Elk-SN。尽管其PGV在所有地震信号中处于中间值，却激发了较周围工况更为剧烈的晃荡波，主要原因是其主频与储液的一阶固有频率接近且具有较大PGV。部分时刻的自由液面如图10所示，可见：液体在储罐内形成了共振行进波，在第40 s和第43 s时刻形成了波斗较大的波面，最终发生了明显的水跃，造成波高局部增大；第44 s波浪破碎且局部飞溅；随后，爬升的液体落回自由液面，与向左运动的液体一起冲击罐壁，并在第45 s爬升至最高处，波高为3.58 m。该工况包含强非线性的物理现象，如液面翻转、破碎等，增加了流场的随机性（Wu，Chen，2009）。
![]()
图 10 芦山53Elk-SN地震载荷下的自由液面Figure 10. Free surface snapshots under Lushan 53Elk-SN excitation以上讨论表明：地震主频也是影响自由液面响应的主因之一，晃荡波高随地震主频的升高逐渐减小，当主频与储液的一阶固有频率相近时，易引起强烈的非线性共振晃荡；峰值波高与PGV呈较强的正相关；最剧烈的工况一般出现在低频且具有较大PGV的地震载荷，在设计储罐时应考虑减晃装置。
3.2.2 水动压
非破碎晃荡波对罐壁产生的水动压分为脉冲压力和对流压力两种模式（薛米安等，2019）。脉冲压力是由地震载荷直接导致的瞬时冲击压力，对流压力与自由液面运动密切相关。图11为峰值水动压沿罐壁面的分布。可见：除芦山53Elk-SN，其它工况基本一致，即峰值水动压从底部到自由液面逐步减小，且增长速率逐渐减缓，极大值总出现在底部。
![]()
图 11 峰值水动压沿罐壁的分布Figure 11. Distributions of peak hydrodynamic pressures along the tank sidewall图12为芦山53Elk-SN载荷下罐内水动压部分时刻的分布情况。可见：液体向右侧聚集后出现水跃，从43.2 s到43.4 s，液体裹挟空气抨击罐壁，自由液面附近出现峰值水动压（约为20.1 kPa）。其余工况以对流压力特性为主导。
![]()
图 12 芦山53Elk-SN地震载荷下罐内水动压分布Figure 12. Distributions of hydrodynamic pressures in the tank under Lushan 53Elk-SN excitation储罐上、中、下三个测点P6，P4和P2的水动压频谱特性如图13a和13b所示，可见：P6处出现多个谱峰，最大谱峰值出现在储液一阶固有频率，说明储罐上部水动压为低频的共振对流；P4处的最大谱峰值也出现在储液一阶固有频率，并且次要谱峰也出现在地震主要频率处，表明水动压为对流压力与脉冲压力的组合模式，但以对流压力为主导；P2处水动压的谱峰值与地震主要频率完全一致，表明其为脉冲冲击模式。
![]()
13 储罐内水动压的快速傅里叶分析结果13. Fast Fourier transform results of hydrodynamic pressures in the tank![]()
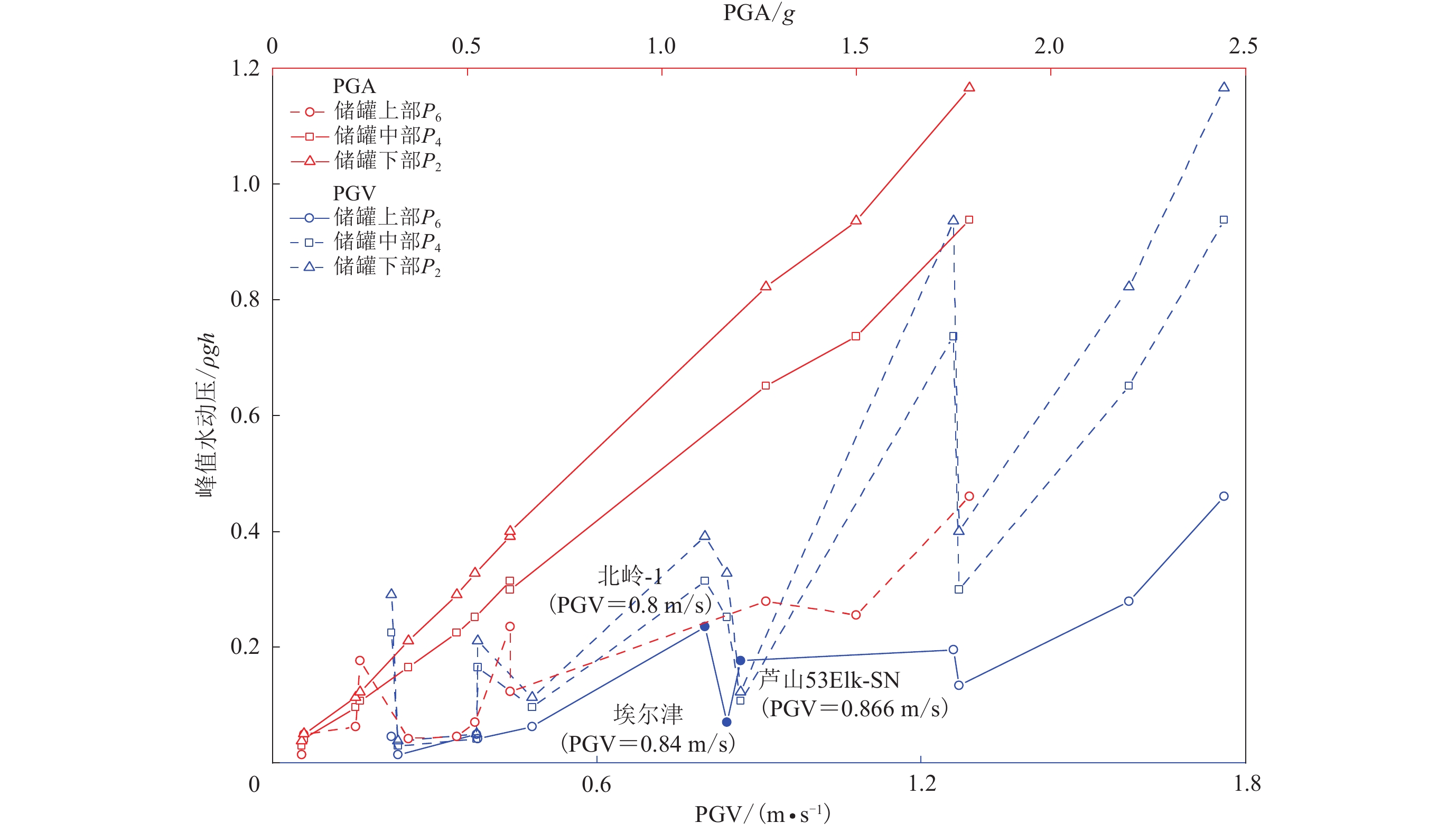
13 储罐内水动压的快速傅里叶分析结果13. Fast Fourier transform results of hydrodynamic pressures in the tankPGV，PGA与峰值水动压的关系如图14所示，可见：P4和P2处的峰值水动压与PGV的关系并不明显，但其与PGA呈明显的线性关系，且PGA较大的地震能激发较高的水动压；峰值水动压在下部（P2点）的增长速率明显大于中部（P4点），说明越靠近底部，水动压的脉冲特性越强，因此在储罐设计时，要充分考虑当地的场地条件（重点关注PGA较大的区域），并适当提高设计指标确保罐壁下部的强度；P6处水动压表现为共振对流模式，同属低频的埃尔津、芦山53Elk-SN及北岭-1地震具有相近的PGV （分别为0.84 m/s，0.866 m/s和0.8 m/s），但埃尔津的主频（0.563 Hz）远离储液一阶固有频率，而芦山53Elk-SN和北岭-1的主频（分别为0.325 Hz和0.353 Hz）更加接近储液一阶固有频率，因此，二者激发的共振晃荡产生的对流压力使得储罐上部（P6点）的水动压远大于非共振时的工况，其峰值分别为前者的2.51倍和3.35倍。上述讨论表明，地震主频是影响储罐上部水动压的主要因素，且其峰值随PGV的增大而上升。
![]()
图 14 各工况下PGV和PGA与峰值水动压的关系Figure 14. The relationships between PGV （or PGA） and peak hydrodynamic pressure for all cases4. 讨论与结论
本文基于计算流体力学软件Fluent，采用多参考系法对流体晃荡进行数值模拟研究，探究地震载荷下圆柱型储罐内储液的晃荡特性，得出以下结论：
1） 地震的频率成分可用于表征地震的主周期，与储罐模型共同决定晃荡波的共振模式，能反映出晃荡的共振状态，同时共振模式还受地震主频和PGV的影响。
2） 自由液面响应以对流为主，随着PGV的增加，波高整体增大，但存在特例，如：北岭-1与埃尔津地震（或神户与曼杜西诺角地震）的PGV相近，前者的主频低，诱发的波高是后者的1.4倍（或1.5倍）。最剧烈的工况为塔夫脱地震，其具有最大的PGV且主频最低。
3） 水动压表现为脉冲和对流两种模式。上部为对流模式，受地震主频和PGV影响；中部为对流和脉冲的组合模式；下部为脉冲模式，其峰值与PGA呈线性关系。该发现为储罐结构自上而下的整体振动响应设计提供了科学依据，有助于找到降低结构振动响应的工程方案。
4） 峰值水动压一般出现在罐壁底部，对于波面破碎的工况，峰值水动压则出现在自由液面附近。因此在储罐抗震设计时，应加强罐壁下部的强度，同时兼顾液面附近破碎冲击带来的强冲击。
本研究给出了极端工况的激发机制，即：地震属于低频且具有强PGV，以及水动力载荷峰值和频谱特性沿储罐壁面的演化规律。后续将针对此类工况下的结构振动分析和流体减晃研究，在液舱内布置刚性或柔性结构，以降低晃荡波高和水动力载荷。
-
![]()
图 1 1995年神户M7地震的位移时程（a）以及神户地震载荷下罐壁右侧波高的时程曲线对比（b）
Figure 1. Time history of ground displacement of Kobe seismic excitation （a） and comparison of time histories of wave heights at the right sidewall of the cylindrical tank under 1997 M7 Kobe seismic excitation （b）
![]()
图 2 本文选取地震的加速度时程曲线
Figure 2. Time histories of considered seismic accelerations used in this study
![]()
图 3 圆柱型储罐的整体视图（a）和顶视图（b）
Figure 3. The overall-view （a） and top-view （b） geometries of the cylindrical tank
![]()
图 4 芦山53Elk-SN载荷下储罐东侧We （a）和北侧Wn （b）处不同网格数下波高的对比
Figure 4. Comparison of time histories of the wave heights at We （a） and Wn （b） from various grids under Lushan 53Elk SN excitation
![]()
图 5 不同地震载荷下储罐东侧We和北侧Wn处的波高时程曲线
（a） 芦山53Elk-EW；（b） 芦山53Elk-SN；（c） 芦山53Yjb-EW；（d） 埃尔森特罗-EW；（e） 神户地震；（f） 埃尔津；（g） 北岭-1；（h） 唐山；（i） 康奎特；（j） 埃尔森特罗-SN；（k） 塔夫脱；（l） 曼杜西诺角；（m） 汶川卧龙-EW；（n） 柯依娜-SN；（o） 芦山53Yjb-SN
Figure 5. Time histories of wave heights under various seismic excitations
（a） Lushan53Elk-EW；（b） Lushan53Elk-SN；（c） Lushan53Yjb-EW；（d） El Centro-EW；（e） Kobe；（f） Erzican；（g） Northridge-1；（h） Tangshan；（i） Concrete；（j） El Centro-SN；（k） Taft；（l） Cape Mendocino；（m） WenchuanWolong-EW；（n） Koyna-SN；（o） Lushan53Yjb-SN
![]()
图 6 储罐东侧We处峰值波高与地震主频的关系
Figure 6. The relationship between the maximum wave height at east side We of the tank and dominant frequency of earthquake
![]()
图 7 储罐东侧We处峰值波高与PGV的关系
Figure 7. The relationship between the maximum wave height at the east side We of the tank and PGV
![]()
图 8 塔夫脱地震载荷下的自由液面
Figure 8. Free surface snapshots under excitation of Taft earthquake
![]()
图 9 塔夫脱地震载荷下自由液面的波高相位图
Figure 9. Phase diagrams of wave heights under excitation of Taft earthquake
（a） 0—15 s；（b） 15—30 s；（c） 20—45 s
![]()
图 10 芦山53Elk-SN地震载荷下的自由液面
Figure 10. Free surface snapshots under Lushan 53Elk-SN excitation
![]()
图 11 峰值水动压沿罐壁的分布
Figure 11. Distributions of peak hydrodynamic pressures along the tank sidewall
![]()
图 12 芦山53Elk-SN地震载荷下罐内水动压分布
Figure 12. Distributions of hydrodynamic pressures in the tank under Lushan 53Elk-SN excitation
![]()
13 储罐内水动压的快速傅里叶分析结果
13. Fast Fourier transform results of hydrodynamic pressures in the tank
![]()
13 储罐内水动压的快速傅里叶分析结果
13. Fast Fourier transform results of hydrodynamic pressures in the tank
![]()
图 14 各工况下PGV和PGA与峰值水动压的关系
Figure 14. The relationships between PGV （or PGA） and peak hydrodynamic pressure for all cases
表 1 本研究涉及地震的相关参数
Table 1 The characteristics of considered seismic excitations in this study
地震信号 频率类别 主要频率/Hz PGA/g PGV/（m·s−1） PGA/PGV 芦山53Elk-EW 低频 0.425，0.625 0.081 0.377 0.215 芦山53Elk-SN 低频 0.325，0.4 0.224 0.866 0.259 芦山53Yjb-EW 低频 0.658，0.703 0.075 0.232 0.323 埃尔森特罗（El Centro）-EW 低频 0.449，0.842 0.214 0.48 0.446 神户（Kobe） 低频 0.269，0.537，0.879 0.611 1.27 0.48 埃尔津（Erzican） 低频 0.42，0.563，0.798 0.52 0.84 0.619 北岭-1（Northridge-1） 低频 0.353，0.873，1.08 0.607 0.8 0.754 唐山 中频 0.65，0.8，1.2 1.268 1.584 0.8 康奎特（Concrete） 中频 0.49，0.6，0.98，1.1 0.63 0.758 0.83 埃尔森特罗（El Centro）-SN 中频 0.561，0.839，1.16 0.349 0.38 0.91 塔夫脱（Taft） 中频 0.11，0.37，0.61，1.2 1.79 1.76 1.02 曼杜西诺角（Cape Mendocino） 中频 0.868，1.43，2.14 1.45 1.26 1.15 汶川卧龙-EW 高频 0.622，1.38，2.35 0.97 0.585 1.66 柯依那（Koyna）-SN 高频 0.87，1.55 0.585 0.31 1.89 芦山53Yjb-SN 高频 0.841，2.545 0.473 0.22 2.15 注：第一列中的53Elk，53Yjb和卧龙为站点名称，EW和SN为地震信号的东西分量和南北分量。 
下载: 导出CSV
表 2 地震主频、PGV和东侧We处相应的峰值波高
Table 2 The dominant frequency and PGV of seismic excitations and corresponding maximum wave heights at east side We of the tank
地震信号 主频/Hz PGV/（m·s−1） 峰值波高/m 地震信号 主频/Hz PGV/（m·s−1） 峰值波高/m 塔夫脱 0.11 1.76 4.15 埃尔森特罗-SN 0.561 0.38 0.484 神户 0.269 1.27 2.02 汶川卧龙-EW 0.622 0.585 0.26 芦山53Elk-SN 0.325 0.866 3.574 唐山 0.65 1.584 2.3 北岭-1 0.353 0.8 1.48 芦山53Yjb-EW 0.658 0.232 0.17 埃尔津 0.42 0.84 1.06 芦山53Elk-SN 0.841 0.22 0.39 芦山53Elk-EW 0.425 0.377 0.713 曼杜西诺角 0.868 1.26 1.35 埃尔森特罗-EW 0.449 0.48 0.664 柯依那-SN 0.87 0.31 0.334 康奎特 0.49 0.758 0.675
下载: 导出CSV
-
陈贵清,刘望峰,赵晓波,梁乐杰. 2012. 水平和竖向地震激励下大型储液罐响应分析[J]. 唐山学院学报,25(6):33–36. doi: 10.3969/j.issn.1672-349X.2012.06.012 Chen G Q,Liu W F,Zhao X B,Liang L J. 2012. The response analysis of liquid storage tank under horizontal and vertical seismic excitation[J]. Journal of Tangshan College,25(6):33–36 (in Chinese).
方浩,吴昊,王笃国,陈国星. 2012. 储液罐地震安全问题研究综述[J]. 震灾防御技术,7(2):144–151. doi: 10.3969/j.issn.1673-5722.2012.02.005 Fang H,Wu H,Wang D G,Chen G X. 2012. An overview on earthquake safety of liquid storage tank[J]. Technology for Earthquake Disaster Prevention,7(2):144–151 (in Chinese).
刘勇. 2007. 水平与竖向地震激励下储液罐的动力分析[D]. 杭州:浙江大学:47. Liu Y. 2007. Dynamical Analysis of Liquid Storage Tank Excited by Horizontal and Vertical Earthquakes[D]. Hangzhou:Zhejiang University:47 (in Chinese).
罗东雨,孙建刚,柳春光,崔利富,王振. 2020. 近断层地震动作用下大型LNG储罐晃动效应研究[J]. 自然灾害学报,29(5):99–107. Luo D Y,Sun J G,Liu C G,Cui L F,Wang Z. 2020. Research on sloshing effect of large LNG storage tank under near fault ground motions[J]. Journal of Natural Disasters,29(5):99–107 (in Chinese).
孙颖. 2012. 大型立式储罐抗震性能与隔震效应数值分析[D]. 大庆:东北石油大学:1−7. Sun Y. 2012. Seismic Performance and Isolation Effective of Large Vertical Liquid Storage Tank[D]. Daqing:Northeast Petroleum University:1−7 (in Chinese).
薛米安,陈奕超,苑晓丽,邢建建,张冠卿,朱瑞虎. 2019. 低载液率液体晃荡冲击压力的试验研究[J]. 振动与冲击,38(14):239–245. Xue M A,Chen Y C,Yuan X L,Xing J J,Zhang G Q,Zhu R H. 2019. Experimental study on the impact pressure of sloshing liquid with low filling level[J]. Journal of Vibration and Shock,38(14):239–245 (in Chinese).
杨杰,成婷婷,程琳,于崇祯. 2016. 不同地震波作用方向的大型渡槽动力响应分析[J]. 水资源与水工程学报,27(1):195–200. doi: 10.11705/j.issn.1672-643X.2016.01.36 Yang J,Cheng T T,Cheng L,Yu C Z. 2016. Analysis of dynamic response of large aqueduct under different directions of seismic wave[J]. Journal of Water Resources and Water Engineering,27(1):195–200 (in Chinese).
张如林,程旭东,王淮峰,管友海. 2017. 竖向地震作用对储液罐地震响应的影响分析[J]. 地震工程学报,39(4):592–599. doi: 10.3969/j.issn.1000-0844.2017.04.0592 Zhang R L,Cheng X D,Wang H F,Guan Y H. 2017. Analysis of influence of vertical earthquake action on the seismic response of liquid storage tank[J]. China Earthquake Engineering Journal,39(4):592–599 (in Chinese).
周利剑,吴育建,孙建刚,崔利富,吕远,罗东雨. 2019. 长周期地震动作用下大型立式储罐晃动波高问题研究[J]. 自然灾害学报,28(3):87–95. Zhou L J,Wu Y J,Sun J G,Cui L F,Lü Y,Luo D Y. 2019. Study on sloshing wave height of large vertical storage tanks under long period earthquake[J]. Journal of Natural Disasters,28(3):87–95 (in Chinese).
Caron P A,Cruchaga M A,Larreteguy A E. 2018. Study of 3D sloshing in a vertical cylindrical tank[J]. Phys Fluids,30(8):082112. doi: 10.1063/1.5043366
Chen Y H,Hwang W S,Ko C H. 2007. Sloshing behaviours of rectangular and cylindrical liquid tanks subjected to harmonic and seismic excitations[J]. Earthq Eng Struct Dyn,36(12):1701–1717. doi: 10.1002/eqe.713
Cheng X S,Jing W,Feng H. 2019. Nonlinear dynamic responses of sliding isolation concrete liquid storage tank with limiting-devices[J]. KSCE J Civ Eng,23(7):3005–3020. doi: 10.1007/s12205-019-1480-5
Djermane M,Zaoui D,Labbaci B,Hammadi F. 2014. Dynamic buckling of steel tanks under seismic excitation:Numerical evaluation of code provisions[J]. Eng Struct,70:181–196. doi: 10.1016/j.engstruct.2014.03.037
Faltinsen O M,Rognebakke O F,Timokha A N. 2003. Resonant three-dimensional nonlinear sloshing in a square-base basin[J]. J Fluid Mech,487:1–42. doi: 10.1017/S0022112003004816
Hejazi F S A,Mohammadi M K. 2019. Investigation on sloshing response of water rectangular tanks under horizontal and vertical near fault seismic excitations[J]. Soil Dyn Earthq Eng,116:637–653. doi: 10.1016/j.soildyn.2018.10.015
Hernandez-Hernandez D,Larkin T,Chouw N. 2021. Evaluation of the adequacy of a spring-mass model in analyses of liquid sloshing in anchored storage tanks[J]. Earthq Eng Struct Dyn,50(14):3916–3935. doi: 10.1002/eqe.3539
Hirt C W,Nichols B D. 1981. Volume of fluid (VOF) method for the dynamics of free boundaries[J]. J Comput Phys,39(1):201–225. doi: 10.1016/0021-9991(81)90145-5
Jin X,Xue M A,Lin P Z. 2021. Numerical modeling and formulation of the runup of seismically-induced surge waves in idealized reservoirs[J]. Soil Dyn Earthq Eng,143:106625. doi: 10.1016/j.soildyn.2021.106625
Kianoush M R,Ghaemmaghami A R. 2011. The effect of earthquake frequency content on the seismic behavior of concrete rectangular liquid tanks using the finite element method incorporating soil-structure interaction[J]. Eng Struct,33(7):2186–2200. doi: 10.1016/j.engstruct.2011.03.009
Luo M,Koh C G,Bai W. 2016. A three-dimensional particle method for violent sloshing under regular and irregular excitations[J]. Ocean Eng,120:52–63. doi: 10.1016/j.oceaneng.2016.05.015
Shu L C,Li H T,Hu Q,Jiang X L,Qiu G,He G H,Liu Y Q. 2018. 3D numerical simulation of aerodynamic performance of iced contaminated wind turbine rotors[J]. Cold Reg Sci Technol,148:50–62. doi: 10.1016/j.coldregions.2018.01.008
Tso W K,Zhu T J,Heidebrecht A C. 1992. Engineering implication of ground motion A/V ratio[J]. Soil Dyn Earthq Eng,11(3):133–144. doi: 10.1016/0267-7261(92)90027-B
Virella J C,Godoy L A,Suárez L E. 2006. Fundamental modes of tank-liquid systems under horizontal motions[J]. Eng Struct,28(10):1450–1461. doi: 10.1016/j.engstruct.2005.12.016
Wu C H,Chen B F. 2009. Sloshing waves and resonance modes of fluid in a 3D tank by a time-independent finite difference method[J]. Ocean Eng,36(6/7):500–510.
Xue M A,Chen Y C,Zheng J H,Qian L,Yuan X L. 2019. Fluid dynamics analysis of sloshing pressure distribution in storage vessels of different shapes[J]. Ocean Eng,192:106582. doi: 10.1016/j.oceaneng.2019.106582
计量
- 文章访问数: 110
- HTML全文浏览量: 20
- PDF下载量: 30
