3-D modeling of velocity structure for the Yuxi basin
-
摘要: 本文以玉溪盆地为例,提出了一种包含数据预处理、模型建立、模型修正和模型检验的建模方法.基于各类数据间不同的可信度,给出了消除各类数据间速度偏差的折减函数.为避免以往模型修正过程中对地震波形数据的依赖以及对地脉动H/V谱进行模拟等复杂问题,本文提出了一种改进的模型修正方法,即根据基阶瑞雷波H/V谱与实测地脉动H/V谱形状变化相似的原则,对模型进行修正.修正依据为:在玉溪盆地中,单个地脉动测点所在位置处的地下速度结构中各沉积层面的深度均增加约15 m时,由该点的地下速度结构得到的基阶瑞雷波H/V谱的波峰周期和波谷周期均增加约0.1 s,且二者分别由盆地内沉积层的深层和浅层的速度结构所控制.由于地脉动数据的获取较方便,因此该模型修正方法具有广泛的适用性,由该方法修正后的玉溪盆地三维速度结构模型经检验具有较高的准确度.Abstract: To achieve ground motion prediction, it is necessary to build a 3-D velocity structure model of sedimentary basin. Taking the Yuxi basin as an example, we provide a modeling procedure, including data preprocessing, model building, model updating and model testing. Based on the different credibility of various data, we propose a function that can eliminate the velocity deviation among various data. In this paper, an improved model updating method is proposed in order to avoid the dependence on the seismic waveform data and the simulation of the microtremors spectral ratio of horizontal to vertical component (H/V spectra). We apply the theory that the H/V spectral shape of the fundamental-mode Rayleigh, including its peak and trough periods, is similar to that of the microtremors to update the 3-D velocity structure model. When the depth of each sedimentary layer in the velocity structure under a single microtremors measuring point is increased by about 15 m, the H/V spectral peak and trough periods of the fundamental-mode Rayleigh wave will both increase about 0.1 s, moreover, the two periods are controlled by deep or shallow velocity structures of the sedimentary layers in basin respectively. Because of the convenient acquisition of the microtremors data, the model updating method has extensive applicability. The 3-D velocity structure model of the Yuxi basin modified by the model updating method has been confirmed a high accuracy after inspection.
-
Keywords:
- ground motion prediction /
- Yuxi basin /
- 3-D velocity structure /
- model updating /
- H/V spectra
-
引言
在日本Kobe地震(Kawase,1996)、美国Northridge地震(Graves et al,1998)和墨西哥Michoacan地震(Reinoso,Ordaz,1999)中均出现了沉积盆地内的震害比盆地周边地区更严重的现象,这是因为盆地内的沉积层对地震动的振幅和持时有明显的放大作用(Olsen et al,1995),加重了对地表结构物的破坏(Olsen,2000;Komatitsch et al,2004;Shani-Kadmiel et al,2011),因此要预测沉积盆地内的地震动,需要建立精确的盆地内沉积层三维速度结构模型(高孟潭等,2002;Kohler et al,2003;Aoi et al,2008).同时由于多数城市均位于沉积盆地内,这也使得沉积盆地三维速度结构建模的研究工作变得尤为重要(Koketsu et al,2009).
沉积盆地三维速度结构模型的准确度主要由建模数据的精度和数量所控制.近几年,一些研究人员基于丰富的钻孔、地震测线、重力勘探、地脉动、强震记录及地震波形等数据,在一些沉积盆地内建立了准确的三维速度结构模型,例如Koketsu等(2009)建立的Kanto盆地速度结构模型和Dhakal等(2011)建立的Tokachi盆地速度结构模型.在各类建模数据中,由于获取地脉动数据比获取其它建模数据更加经济和方便,使得地脉动数据被广泛地应用于地下速度结构的确定(Uebayashi et al,2012).目前采用地脉动数据建立地下速度结构模型的方法可分为两类:利用地脉动台阵法反演浅层剪切波速度(Arai,Tokimatsu,2005;Huang,Wu,2006;何正勤等,2007;王伟君等,2009;Claprood et al,2011;徐佩芬等,2012)和利用单点地脉动的水平向与垂直向H/V谱比反演地下速度结构(Delgado et al,2000;Dolenc,2005;Özalaybey et al,2011),但通过这两种方法得到的速度结构均存在一定的误差(Arai,Tokimatsu,2004;Claprood, Asten,2010),尤其是利用单点地脉动H/V谱反演得到的速度结构误差更大(王伟君等,2011).此外,单点地脉动H/V谱也常被用于评估场地的放大效应(陈棋福等,2008).
随着对地脉动研究的逐渐深入,Rhie和Dreger(2009)提出可通过对比理论地脉动谱与实测地脉动H/V谱的波形来修正沉积盆地的速度结构模型.但无论是基于一维速度结构模型推导出的地脉动H/V谱(Lunedei,Albarello,2010;Sánchez-Sesma et al,2011),还是基于三维速度结构模型模拟出的地脉动H/V谱(Guéguen et al,2007;Barnaba et al,2010),均与实测地脉动H/V谱的振幅存在偏差,所以基于对比地脉动H/V谱的波形变化对模型进行修正的方法并不成熟.此外,在模型修正环节中为避免模拟地脉动H/V谱,Suzuki等(2005)根据地震数据的H/V谱与基阶瑞雷波的H/V谱形状变化相似的原则对模型进行修正. Aoi等(2008)建立的Tokachi盆地三维速度结构模型以及Koketsu等(2009)建立的Kanto盆地三维速度结构模型,均利用了Suzuki等(2005)提出的模型修正方法,并得到了较精确的沉积盆地三维速度结构模型,证明了依据地震数据的H/V谱与基阶瑞雷波H/V谱形状变化相似的原则对模型进行修正的方法是可行的,但该修正方法依赖于丰富的地震数据,对于缺少地震记录的地区并不适用.在模型检验环节,Iwata等(2008)以及Iwaki和Iwata(2010)通过对比模拟的地震动波形与实测的地震动波形来检验沉积盆地模型建立的准确性,并取得了很好的应用效果,但该方法仅适用于强震记录丰富的地区.
鉴于此,为了避免以往建模过程中对地震数据,特别是强震数据的依赖, 本文拟提出一种包含数据预处理、模型建立、模型修正和模型检验的完整的建模方法.基于各类数据间不同的可信度,提出消除不同数据之间速度偏差的折减函数.为了避免以往在模型修正环节中对地震波形数据的依赖以及地脉动H/V谱的模拟等问题,本文提出一种改进的模型修正方法,即根据基阶瑞雷波H/V谱(H/V)R0与实测地脉动H/V谱(H/V)m形状变化相似(郭明珠等,2004;Arai,Tokimatsu,2004)的原则,对模型进行修正.由于地脉动数据的获取比较经济和方便,使得该模型修正方法具有广泛的适用性.本文以玉溪盆地为例进行建模,玉溪盆地位于云贵高原西南部的盆岭分布区,四周群山环绕,盆地内地形平坦,是一个典型的沉积盆地.
1. 建模过程
地震动模拟的关键是基岩面以上沉积层速度结构(Dhakal,Yamanaka,2013),且基岩面以下缺少详细的建模数据,故本文仅建立该盆地内基岩面以上沉积层的三维速度结构模型.
在建立玉溪盆地模型的过程中,可用于盆地速度结构建模的数据有钻孔资料、地震勘探数据和地脉动数据.因为在地质分层较多的情况下,通过地脉动数据反演得到的地下速度结构存在较大误差,所以本文并未将地脉动数据用于初始建模,而仅用于模型修正.玉溪盆地的建模过程为:①数据预处理.依据经验公式(朱广生等,1995)将地震勘探得到的P波速度转换成S波速度.当钻井资料和地震勘探数据之间存在速度偏差时,考虑到各类数据间不同的可信度,对数据进行修正,以消除各类型数据间的速度偏差. ②模型建立.综合考虑钻孔资料和地震勘探数据,以剪切波速度为主要分层依据,同时参考地质序列.将钻孔资料和地震勘探数据作为模型层面的“硬性约束”,采用克里格插值方法,获得各沉积层面(各沉积层之间的分界面)的几何形状和展布形态,得到初步构建的盆地分层模型. ③模型修正.通过对比由盆地初始分层模型计算得到的基阶瑞雷波H/V谱(H/V)R0与实测地脉动H/V谱(H/V)m的波峰周期和波谷周期,对模型进行修正,得到最终模型. ④模型检验.用未参与模型修正的地脉动测点数据对修正后的模型进行检验,检查(H/V)R0与(H/V)m的波峰周期和波谷周期是否一致,进而确定本文所建立的速度结构模型的合理性.若检验结果显示不一致,则进一步修正模型.
2. 玉溪盆地三维速度结构模型
2.1 数据预处理
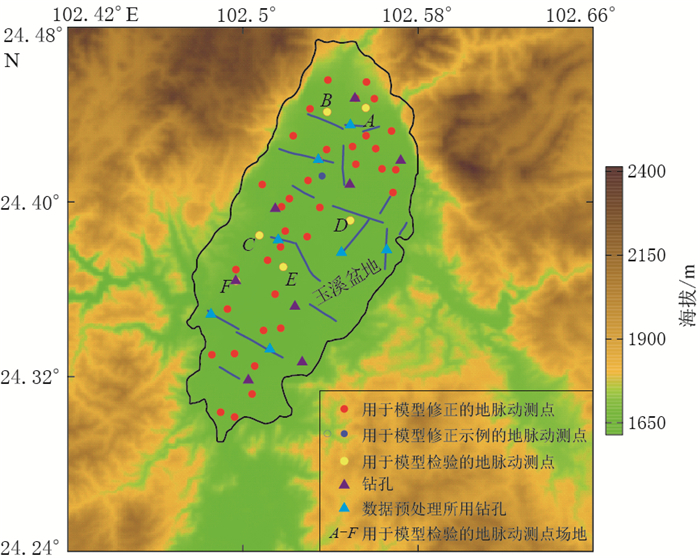
用于玉溪盆地速度结构建模的数据包括钻孔(深度为80 m左右)、地震勘探测线和地脉动,如图 1所示.由于地表附近(深度小于80 m)的钻孔资料与地震勘探数据的精度不同,造成即使在同一位置由地震勘探得到的剪切波速度远大于由钻孔得到的剪切波速度.
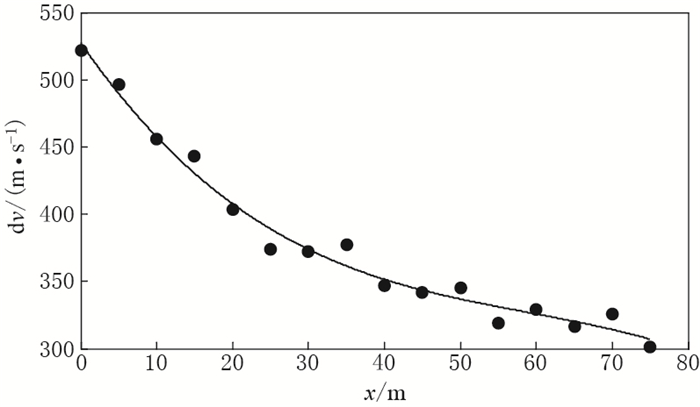
选取图 1中7个青色钻孔及其邻近地震勘探测线上距钻孔最近的7个点,计算这7组地震勘探数据与钻孔资料之间的剪切波速度偏差的平均值dv.使用R语言对剪切波速度偏差dv与深度x作相关性分析,得到两者的相关系数为-0.935,具有很好的负相关性,即地震勘探数据与钻孔数据之间的剪切波速度偏差dv随深度x的增加而递减.产生这种现象的原因是在通过钻孔实测数据获取剪切波速度数据的过程中不确定性因素较少,所以由钻孔实测数据获取剪切波速度的准确度较高.另一方面通过地震勘探数据获取剪切波速度的过程中不确定性因素较多,例如数据采集时外界环境的干扰、反演结果的不唯一性和用于反演的震相射线路径的平均效应等问题,均会造成由地震勘探数据获取剪切波速度的准确度较低;然而随着深度的增加,外界干扰相对降低,由地震勘探数据得到的剪切波速度的精度相对于地表处有所增加.
由于钻孔资料的精度较高,本文将由钻孔资料得到的剪切波速度视为准确的剪切波速度,使用剪切波速度偏差dv修正由地震勘探得到的剪切波速度.用R语言作剪切波速度偏差dv与深度x的多项式拟合(图 2),得到一个速度偏差dv与深度x的三次多项式,即
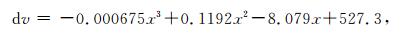
(1) 式(1)中各项系数(从3次项依次到0次项)t检验的概率值p依次为0.02349,0.00173,1.81×10-6和2×10-16,均小于0.025,因此拒绝各项系数为0的假设,即各项系数显著不为0.多项式的可决系数为0.9828,调整可决系数为0.9786.对多项式的整体显著性进行检验,即对残差进行F检验,得到p为7.408×10-11,小于0.01,拒绝速度偏差dv与深度x的精度存在显著性差异的假设,说明该拟合结果是可靠的.同时得到各项系数95%的置信区间分别为(-1.24×10-3, -1.08×10-4),(5.44×10-2, 0.18),(-10.13,-6.03),(510.08,544.44).由式(1)可知,当深度约为131 m时dv减小至0.本文假定当深度大于131 m时由地震勘探得到的剪切波速度是准确的,可直接用于速度建模;当深度在131 m以内时,由地震勘探得到的剪切波速度需使用式(1)进行速度修正后,才可用于速度建模.
2.2 模型建立
何正勤等(2013)综合考虑地震勘探数据、钻孔资料和沉积层的地质序列,提出了玉溪盆地普渡河断裂带及其附近的速度结构模型.由于普渡河断裂带南段穿过玉溪盆地,所以该断裂带及其附近的速度结构能很好地反映盆地内的速度结构.本文在该速度结构模型的基础上,综合考虑已获得的各类数据,以剪切波速度为主要分层依据,初步假定玉溪盆地内存在6个沉积层,各沉积层的剪切波速度分别为400, 600, 800, 1200, 1400和1600 m/s.玉溪盆地的基岩主要为震旦系澄江组及中元古界昆阳群灰岩、砂岩和板岩(何正勤等,2013).本文将基岩面以下地层的剪切波速度定为3000 m/s(Koketsu et al,2009).
各沉积层面的形状变化满足空间自相关关系,因此可使用克里格插值方法对由钻孔资料和地震勘探测线得到的剪切波速度进行插值,得到玉溪盆地模型内各沉积层面的几何形状和展布形态,其中在克里格插值过程中所需的变差函数可通过拟合已知的剪切波速度数据来确定.
2.3 模型修正
目前计算理论地脉动H/V谱的方法仍不成熟,如果通过对比理论地脉动H/V谱与实测地脉动H/V谱的振幅变化的方法来修正模型,会使修正后的模型存在较大误差.本文根据基阶瑞雷波H/V谱(H/V)R0与实测地脉动H/V谱(H/V)m的变化形状相似(波峰周期和波谷周期相同,振幅不同)的原则,对模型进行修正.基阶瑞雷波H/V谱(H/V)R0为(Arai,Tokimatsu,2004)
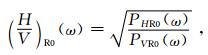
(2) 
(3) 
(4) 式中:D为介质响应因子(Harkrider, 1964, 1970);κ为波数,κ=(2/h)e-4πh,h为地层散射阻尼系数;PHR0(ω)和PVR0(ω)分别为瑞雷波水平向和垂直向功率谱;LV为当地脉动测点到地脉动加载源的距离大于瑞雷波的波长时地表处垂直分量的随机分布;θ为地脉动加载源水平向与垂直向之比,
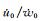
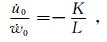
(5) 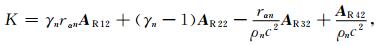
(6) 
(7) 式中: γn=2(βn/c)2,α和β分别为P波和S波的速度,c为瑞雷波的相速度; ρn为第n层土层的密度;AR=an-1an-2…a1,ai为第i层瑞雷波传递矩阵; 当c>αi时,rαi=
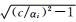
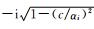
基阶瑞雷波H/V谱的变化形状主要由盆地内各沉积层的厚度和剪切波速度控制,因此,可以通过改变盆地速度模型内各沉积层的厚度或剪切波速度的方式来调整基阶瑞雷波H/V谱(H/V)R0的变化形状,使其与实测地脉动H/V谱(H/V)m的变化形状相同(振幅不同),以达到修正模型的目的.若通过改变剪切波速度的方法修正模型,只是改变了单个地脉动测点所在位置处地下速度结构中某一个或若干个沉积层的剪切波速度,修改后的速度数据无法利用克里格插值得到新的各沉积层面的展布形态和几何形状;而若通过改变沉积层厚度的方法修正模型,则可改变单个地脉动测点所在位置处地下速度结构中某一个或若干个沉积层分界面的埋深,修改后的分界面埋深数据可直接用于克里格插值,从而得到各沉积层面的展布形态和几何形状,因此本文通过改变各沉积层厚度(即各沉积层分界面埋深)的方法进行模型修正.
玉溪盆地内地脉动测点的分布情况如图 1所示.以图 1中蓝色圆点代表的地脉动测点为例,提出该模型修正方法的依据及具体步骤.在初始建立的速度结构模型中,地脉动测点所在位置的地下速度结构显示,剪切波速度分别为400,600,800,1200,1400,1600 m/s的沉积层分界面的埋深分别为63,116,162,502,663,862 m,基岩面埋深为496 m(何正勤等,2013).剪切波速度为1200 m/s的沉积层分界面的埋深(502 m)已超过基岩面的埋深(496 m),所以该点地下沉积层速度结构中剪切波速度的最大值为1200 m/s.面波H/V谱的形状对浅层速度结构的变化比较敏感,即浅部速度结构对面波H/V谱的影响更显著(Arai,Tokimatsu,2004),并且本文只对盆地内沉积层的速度结构进行建模,未考虑基岩面以下地层的速度结构,所以在计算(H/V)R0时,将最深层的沉积层视为无限半空间.在计算地脉动测点所在位置处地下速度结构的(H/V)R0时,将剪切波速度为1200 m/s的沉积层视为无限半空间.
模型修正时只需(H/V)R0与(H/V)m的波峰周期和波谷周期的变化形状相同即可,并不要求振幅相同.基阶瑞雷波H/V谱的公式是依据瑞雷波在水平层状半空间介质中的传播理论推导出来的,使用单点地脉动反演浅层速度结构时,将单个地脉动测点所在位置处的地下速度结构视为水平层状介质,从而反演出单个地脉动测点处的地下速度结构模型.经多次模型修正,提出修正的原则:基阶瑞雷波H/V谱的波峰周期主要由剪切波速度为800,1200和1400 m/s的沉积层分界面的深度所控制,而基阶瑞雷波H/V谱的波谷周期主要由剪切波速度为400和600 m/s的沉积层分界面的深度所控制.若(H/V)R0的波峰周期或波谷周期大于(H/V)m的波峰周期或波谷周期时,各沉积层面的深度要相应减小;反之,各沉积层面的深度则相应增大.一般情况下,玉溪盆地中单个地脉动测点所在位置处的地下速度结构中各沉积层分界面的深度每增加约15 m时,由该点地下速度结构得到的(H/V)R0波峰周期和波谷周期则会增加约0.1 s.
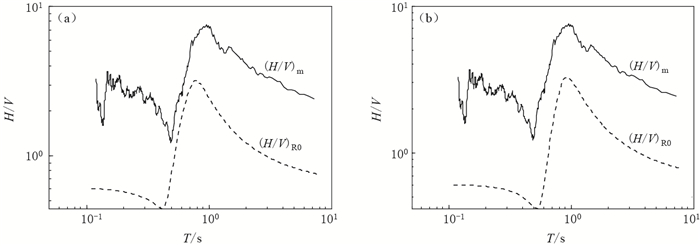
图 3a给出了计算得到的模型修正前地脉动测点(蓝色圆点)的(H/V)R0和(H/V)m。从图中可以看出,初始模型中(H/V)R0的波谷周期比(H/V)m的波谷周期小0.1 s,根据模型修正原则,将剪切波速度为400和600 m/s的沉积层分界面的深度均增加15 m左右,依次修正为75和126 m;(H/V)R0与(H/V)m的波峰周期相差0.2 s,根据模型修正原则,将剪切波速度为800 m/s的沉积层分界面的深度增加30 m左右,修正为192 m.模型修正后的(H/V)R0与(H/V)m如图 3b所示,(H/V)R0与(H/V)m的波峰周期和波谷周期均很一致,证明该地脉动测点所在场地的速度结构模型是合理的.其余各地脉动测点所在场地的速度结构模型也通过该方法进行多次修正,将修正后得到的各地脉动测点的速度结构模型作为新的已知数据,结合钻井资料和地震勘探数据重新进行克里格插值,得到修正后的玉溪盆地速度结构模型.
2.4 模型检验
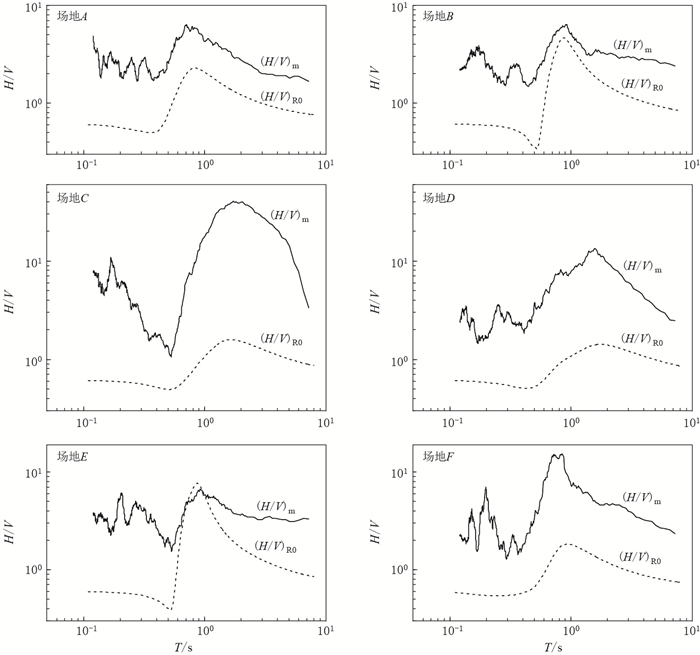
由于玉溪盆地内缺少强震记录,不能通过对比模拟地震动与实测地震动的波形变化来检验模型的准确度; 而本文通过对比(H/V)R0与(H/V)m的形状变化来检验修正后的玉溪盆地的速度结构模型,则可避免对强震数据的依赖.使用图 1中预留的6个未参与模型修正的黄色圆点(A-F)所代表的地脉动测点,检验修正后的盆地速度模型是否准确。6个地脉动测点所在场地的(H/V)R0和(H/V)m如图 4所示。
从图 4可以看出,6个场地的(H/V)R0与(H/V)m的形状变化均很相似.场地C和D的(H/V)R0波峰周期比(H/V)m波峰周期小,主要是因为场地C和D附近用于建模的数据均较少,场地C附近的钻孔和地震勘探数据均比较缺乏, 场地D附近仅有的建模数据均为地震勘探得到的剪切波速度,且地震勘探数据本身存在一定误差,所以在初始建立的模型中,场地C和D处存在一些误差,并且玉溪盆地的中东部地区缺少地脉动数据,使得场地D附近缺少修正模型的数据,导致其速度结构并未被修正.由于场地A,B,E和F附近用于初始建模的钻孔和地震勘探数据比较丰富,所以这4个场地的速度结构模型在初始模型中比较准确.此外,场地A,B,E和F附近用于模型修正的地脉动数据也较充足,因此这4个场地中(H/V)R0与(H/V)m的变化形状很相似.除了场地C和D外,场地A,B,E和F的速度模型均能准确地反映真实的地下速度结构,所以使用本文提出的新的建模方法得到的玉溪盆地速度结构模型的准确度较高,证明该建模方法是可靠的.
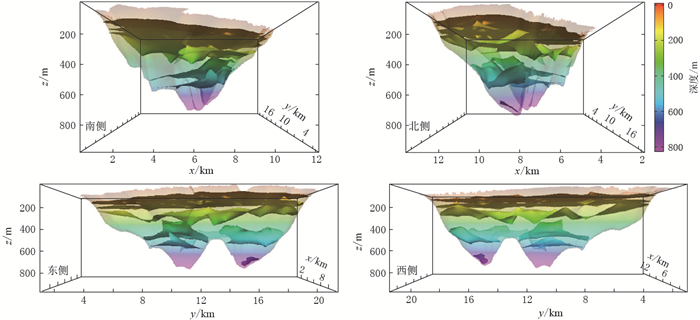
图 5给出了修正后的玉溪盆地内沉积层的三维速度结构模型.图中沉积层分界面的剪切波速度由上到下依次为400,600,800,1200,1400 m/s,基岩面与剪切波速度为1400 m/s的沉积层分界面之间的沉积层的剪切波速度为1600 m/s.
玉溪盆地是发育于普渡河断裂带南端的沉积盆地,沉积层厚度变化较大,盆地边缘沉积层的厚度仅约100 m,而盆地西北部厚度可达800 m,根据岩性差异可分为5—6层(何正勤等,2013).这与本文建立的玉溪盆地速度模型(图 5)相符:该模型中基岩面埋深的变化较大,盆地边缘地区基岩面埋深较浅,由于盆地中部地区太极山的出露使得该地区的基岩埋深较浅;该模型在沉积层厚度较小的区域有4—5个沉积层,在沉积层厚度较大的中部区域有6个沉积层,与玉溪盆地有5—6层不同岩性沉积层的地质情况相符合.
3. 讨论与结论
沉积层对地震动的振幅和持时有显著的放大作用,增大了位于盆地内的城市震害.盆地内的地震动模拟需要建立精确的沉积盆地三维速度结构模型.本文基于已有的数据,提出了一种包括数据预处理、模型建立、模型修正和模型检验的沉积盆地三维速度结构的建模方法.在数据预处理时,依据各类数据间不同的可信度,拟合得到消除速度偏差的折减函数, 然后根据(H/V)R0与(H/V)m的变化形状相似的原则对模型进行修正和检验.该模型修正方法避免了对地震波形数据的依赖, 使得该模型修正方法具有广泛的适用性.使用该模型修正方法得到修正后的玉溪盆地三维速度结构模型,经检验准确度较高,说明了该模型修正方法的合理性.
本文通过地脉动H/V谱的形状变化对比的方法对模型进行修正,未考虑地脉动H/V谱振幅变化的影响,造成修正后的模型还存在一些误差.同时在模型修正时,存在主观因素的干扰,如何避免此类干扰是一个亟需解决的问题.另外,地脉动数据的缺少也极大地限制了模型修正的精度,例如玉溪盆地的中东部地区缺少地脉动数据,造成该地区模型无法进行修正,因此该地区模型的准确度较低.
中国地震局地球物理研究所鲁来玉研究员和陈石研究员分别在面波频散曲线计算和速度结构模型三维可视化展示方面给予了指导,中央级公益性科研院所业务专项(021904)提供了本文所用玉溪盆地的建模数据,作者在此一并表示感谢. -
-
陈棋福, 刘澜波, 王伟君, Rohrbach E. 2008.利用地脉动探测北京城区的地震动场地响应[J].科学通报, 53(18): 2229-2235. doi: 10.3321/j.issn:0023-074X.2008.18.013 Chen Q F, Liu L B, Wang W J, Rohrbach E. 2009. Site effects on earthquake ground motion based on microtremors measurements for metropolitan Beijing[J]. Chinese Science Bulletin, 54(2): 280-287. http://kns.cnki.net/KCMS/detail/detail.aspx?filename=jxtw200902014&dbname=CJFD&dbcode=CJFQ
高孟潭, 俞言祥, 张晓梅, 吴健, 胡平, 丁彦慧. 2002.北京地区地震动的三维有限差分模拟[J].中国地震, 18(4): 356-364. http://kns.cnki.net/KCMS/detail/detail.aspx?filename=zgzd200204004&dbname=CJFD&dbcode=CJFQ Gao M T, Yu Y X, Zhang X M, Wu J, Hu P, Ding Y H. 2002. Three-dimensional finite-difference simulations of ground motions in the Beijing area[J]. Earthquake Research in China, 18(4): 356-364 (in Chinese). http://en.cnki.com.cn/Article_en/CJFDTOTAL-ZGZD200204004.htm
郭明珠, 谢礼立, 凌贤长. 2004.弹性介质面波地脉动单点谱比法研究[J].岩土工程学报, 26(4): 450-453. http://www.oalib.com/paper/4370280 Guo M Z, Xie L L, Ling X Z. 2004. Research on spectral ratio of horizontal to vertical component for elastic model and surface microtremors[J]. Chinese Journal of Geotechnical Engineering, 26(4): 450-453 (in Chinese). http://en.cnki.com.cn/Article_en/CJFDTotal-YTGC200404003.htm
何正勤, 丁志峰, 贾辉, 叶太兰. 2007.用微动中的面波信息探测地壳浅部的速度结构[J].地球物理学报, 50(2): 492-498. http://www.doc88.com/p-9512110300275.html He Z Q, Ding Z F, Jia H, Ye T L. 2007. To determine the velocity structure of shallow crust with surface wave information in microtremors[J]. Chinese Journal of Geophysics, 50(2): 492-498 (in Chinese). http://en.cnki.com.cn/article_en/cjfdtotal-dqwx200702020.htm
何正勤, 安好收, 沈坤, 鲁来玉, 胡刚, 叶太兰. 2013.用地震反射法对玉溪盆地普渡河断裂的探测[J].地震学报, 35(6): 836-847. http://www.dzxb.org/Magazine/Show?id=28891 He Z Q, An H S, Shen K, Lu L Y, Hu G, Ye T L. 2013. Detection of Puduhe fault in Yuxi basin of Yunnan by seismic reflection method[J]. Acta Seismologica Sinica, 35(6): 836-847 (in Chinese). http://www.dzxb.org/Magazine/Show?id=28891
王伟君, 刘澜波, 陈棋福, 张杰. 2009.应用微动H/V谱比法和台阵技术探测场地响应和浅层速度结构[J].地球物理学报, 52(6): 1515-1525. https://es.scribd.com/document/265808204/I Wang W J, Liu L B, Chen Q F, Zhang J. 2009. Applications of microtremor H/V spectral ratio and array techniques in assessing the site effect and near surface velocity structure[J]. Chinese Journal of Geophysics, 52(6): 1515-1525 (in Chinese). http://en.cnki.com.cn/Article_en/CJFDTOTAL-DQWX200906014.htm
王伟君, 陈棋福, 齐诚, 谭毅培, 张项, 周青云. 2011.利用噪声HVSR方法探测近地表结构的可能性和局限性:以保定地区为例[J].地球物理学报, 54(7): 1783-1797. http://d.wanfangdata.com.cn/Periodical_dqwlxb201107012.aspx Wang W J, Chen Q F, Qi C, Tan Y P, Zhang X, Zhou Q Y. 2011. The feasibilities and limitations to explore the near-surface structure with microtremor HVSR method: A case in Baoding area of Hebei Province, China[J]. Chinese Journal of Geophysics, 54(7): 1783-1797 (in Chinese). http://manu39.magtech.com.cn/Geophy/EN/abstract/abstract8060.shtml
徐佩芬, 侍文, 凌苏群, 郭慧丽, 李志华. 2012.二维微动剖面探测"孤石":以深圳地铁7号线为例[J].地球物理学报, 55(6): 2120-2128. doi: 10.6038/j.issn.0001-5733.2012.06.034 Xu P F, Shi W, Ling S Q, Guo H L, Li Z H. 2012. Mapping spherically weathered "Boulders" using 2D microtremor profiling method: A case study along subway line 7 in Shenzhen[J]. Chinese Journal of Geophysics, 55(6): 2120-2128 (in Chinese). http://en.cnki.com.cn/Article_en/CJFDTOTAL-DQWX201206033.htm
朱广生, 桂志先, 熊新斌, 段天友, 唐宗黄, 吴永刚. 1995.密度与纵横波速度关系[J].地球物理学报, 38(增刊1): 260-264. http://www.cqvip.com/QK/94718X/1995A01/1668153.html Zhu G S, Gui Z X, Xiong X B, Duan T Y, Tang Z H, Wu Y G. 1995. Relationship between density and P-wave, S-wave velocities[J]. Acta Geophysica Sinica, 38(S1): 260-264 (in Chinese). http://www.cqvip.com/QK/94718X/1995A01/1668153.html
Aoi S, Honda R, Morikawa N, Sekiguchi H, Suzuki H, Hayakawa Y, Kunugi T, Fujiwara H. 2008. Three-dimensional finite difference simulation of long-period ground motions for the 2003 Tokachi-oki, Japan, earthquake[J]. J Geophys Res Solid Earth, 113(B7): B07302. doi: 10.1029/2007JB005452/citedby
Arai H, Tokimatsu K. 2004. S-wave velocity profiling by inversion of microtremor H/V spectrum[J]. Bull Seismol Soc Am, 94(1): 53-63. doi: 10.1785/0120030028
Arai H, Tokimatsu K. 2005. S-wave velocity profiling by joint inversion of microtremor dispersion curve and horizontal-to-vertical (H/V) spectrum[J]. Bull Seismol Soc Am, 95(5): 1766-1778. doi: 10.1785/0120040243
Barnaba C, Marello L, Vuan A, Palmieri F, Romanelli M, Priolo E, Braitenberg C. 2010. The buried shape of an alpine valley from gravity surveys, seismic and ambient noise analysis[J]. Geophys J Int, 180(2): 715-733. doi: 10.1111/gji.2010.180.issue-2
Claprood M, Asten M W. 2010. Statistical validity control on SPAC microtremor observations recorded with a restricted number of sensors[J]. Bull Seismol Soc Am, 100(2): 776-791. doi: 10.1785/0120090133
Claprood M, Asten M W, Kristek J. 2011. Using the SPAC microtremor method to identify 2D effects and evaluate 1D shear-wave velocity profile in valleys[J]. Bull Seismol Soc Am, 101(2): 826-847. doi: 10.1785/0120090232
Delgado J, Casado C L, Giner J, Estévez A, Cuenca A, Molina S. 2000. Microtremors as a geophysical exploration tool: Applications and limitations[J]. Pure Appl Geophys, 157(9): 1445-1462. doi: 10.1007/PL00001128
Dhakal Y P, Sasatani T, Takai N. 2011. Validation of the deep velocity structure of the Tokachi basin based on 3-D simulation of long-period ground motions[J]. Pure Appl Geophys, 168(10): 1599-1620. doi: 10.1007/s00024-010-0237-3
Dhakal Y P, Yamanaka H. 2013. An evaluation of 3-D velocity models of the Kanto basin for long-period ground motion simulations[J]. J Seismol, 17(3): 1073-1102. doi: 10.1007/s10950-013-9373-4
Dolenc D. 2005. Microseisms observations in the Santa Clara Valley, California[J]. Bull Seismol Soc Am, 95(3): 1137-1149. doi: 10.1785/0120040060
Graves R W, Pitarka A, Somerville P G. 1998. Ground-motion amplification in the Santa Monica area: Effects of shallow basin-edge structure[J]. Bull Seismol Soc Am, 88(5): 1224-1242. https://pubs.geoscienceworld.org/bssa/article-lookup/88/5/1224
Guéguen P, Cornou C, Garambois S, Banton J. 2007. On the limitation of the H/V spectral ratio using seismic noise as an exploration tool: Application to the Grenoble Valley (France), a small apex ratio basin[J]. Pure Appl Geophys, 164(1): 115-134. doi: 10.1007/s00024-006-0151-x
Harkrider D G. 1964. Surface waves in multilayered elastic media. 1. Rayleigh and Love waves from buried sources in a multilayered elastic half-space[J]. Bull Seismol Soc Am, 54(2): 627-679. https://pubs.geoscienceworld.org/bssa/article-lookup/54/2/627
Harkrider D G. 1970. Surface waves in multilayered elastic media. Part Ⅱ. Higher mode spectra and spectral ratios from point sources in plane layered Earth models[J]. Bull Seismol Soc Am, 60(6): 1937-1987. http://authors.library.caltech.edu/60571
Haskell N A. 1953. The dispersion of surface waves on multilayered media[J]. Bull Seismol Soc Am, 43(1): 17-34. doi: 10.1029/SP030p0086/summary
Huang H C, Wu C F. 2006. Estimations of the S-wave velocity structures in Chia-Yi City, Taiwan, using the array records of microtremors[J]. Earth Planets Space, 58(11): 1455-1462. doi: 10.1186/BF03352644
Iwaki A, Iwata T. 2010. Simulation of long-period ground motion in the Osaka sedimentary basin: Performance estimation and the basin structure effects[J]. Geophys J Int, 181(2): 1062-1076. http://adsabs.harvard.edu/abs/2010geoji.181.1062i
Iwata T, Kagawa T, Petukhin A, Ohnishi Y. 2008. Basin and crustal velocity structure models for the simulation of strong ground motions in the Kinki area, Japan[J]. J Seismol, 12(2): 223-234. doi: 10.1007/s10950-007-9086-7
Kawase H. 1996. The cause of the damage belt in Kobe: "The basin-edge effect" constructive interference of the direct S-wave with the basin-induced diffracted/Rayleigh waves[J]. Seismol Res Lett, 67(5): 25-34. doi: 10.1785/gssrl.67.5.25
Kohler M D, Magistrale H, Clayton R W. 2003. Mantle heterogeneities and the SCEC reference three-dimensional seismic velocity model version 3[J]. Bull Seismol Soc Am, 93(2): 757-774. doi: 10.1785/0120020017
Koketsu K, Miyake H, Afnimar, Tanaka Y. 2009. A proposal for a standard procedure of modeling 3-D velocity structures and its application to the Tokyo metropolitan area, Japan[J]. Tectonophysics, 472(1/4): 290-300. http://www.sciencedirect.com/science/article/pii/S0040195108002539
Komatitsch D, Liu Q Y, Tromp J, Süss P, Stidham C, Shaw J H. 2004. Simulations of ground motion in the Los Angeles basin based upon the spectral-element method[J]. Bull Seismol Soc Am, 94(1): 187-206. doi: 10.1785/0120030077
Lunedei E, Albarello D. 2010. Theoretical HVSR curves from full wavefield modelling of ambient vibrations in a weakly dissipative layered Earth[J]. Geophys J Int, 181(2): 1093-1108. doi: 10.1111/j.1365-246X.2010.04560.x/full
Olsen K B, Archuleta R J, Matarese J R. 1995. Three-dimensional simulation of a magnitude 7.75 earthquake on the San Andreas fault[J]. Science, 270(5242): 1628-1632. doi: 10.1126/science.270.5242.1628
Olsen K B. 2000. Site amplification in the Los Angeles basin from three-dimensional modeling of ground motion[J]. Bull Seismol Soc Am, 90(6B): S77-S94. doi: 10.1785/0120000506
Özalaybey S, Zor E, Ergintav S, Tapirdamaz M C. 2011. Investigation of 3-D basin structures in the İzmit Bay area (Turkey) by single-station microtremor and gravimetric methods[J]. Geophys J Int, 186(2): 883-894. doi: 10.1111/j.1365-246X.2011.05085.x
Reinoso E, Ordaz M. 1999. Spectral ratios for Mexico City from free-field recordings[J]. Earthq Spectra, 15(2): 273-295. doi: 10.1193/1.1586041
Rhie J, Dreger D. 2009. A simple method for simulating microseism H/V spectral ratio in 3D structure[J]. Geosci J, 13(4): 401-406. doi: 10.1007/s12303-009-0036-y
Sánchez-Sesma F J, Rodríguez M, Iturrarán-Viveros U, Luzón F, Campillo M, Margerin L, García-Jerez A, Suarez M, Santoyo M A, Rodríguez-Castellanos A. 2011. A theory for microtremor H/V spectral ratio: Application for a layered medium[J]. Geophys J Int, 186(1): 221-225. doi: 10.1111/gji.2011.186.issue-1
Shani-Kadmiel S, Tsesarsky M, Louie J N, Gvirtzman Z. 2011. Simulation of seismic-wave propagation through geome-trically complex basins: The Dead Sea basin[J]. Bull Seismol Soc Am, 102(4): 1729-1739. https://pubs.geoscienceworld.org/ssa/bssa/article-abstract/102/4/1729/325455/simulation-of-seismic-wave-propagation-through?redirectedFrom=fulltext
Suzuki H, Morino M, Iwamoto K, Liu Y, Fujiwara H, Hayakawa Y. 2005. 3D subsurface structural model for strong motion simulation around Lake Biwa, southwest Japan[J]. Zisin, 58: 91-106 (in Japanese). doi: 10.4294/zisin1948.58.2_91
Uebayashi H, Kawabe H, Kamae K. 2012. Reproduction of microseism H/V spectral features using a three-dimensional complex topographical model of the sediment-bedrock interface in the Osaka sedimentary basin[J]. Geophys J Int, 189(2): 1060-1074. doi: 10.1111/gji.2012.189.issue-2
-
期刊类型引用(2)
1. 师黎静,宋健,党鹏飞,刘佳轩. 区域场地近地表速度结构建模研究. 岩土工程学报. 2022(02): 360-367 .  百度学术
百度学术
2. 万远春,于彦彦,丁海平,胡颖平. 考虑不均匀地壳构造的四川盆地地震动模拟研究. 自然灾害学报. 2022(02): 204-214 .  百度学术
百度学术
其他类型引用(3)




 下载:
下载: