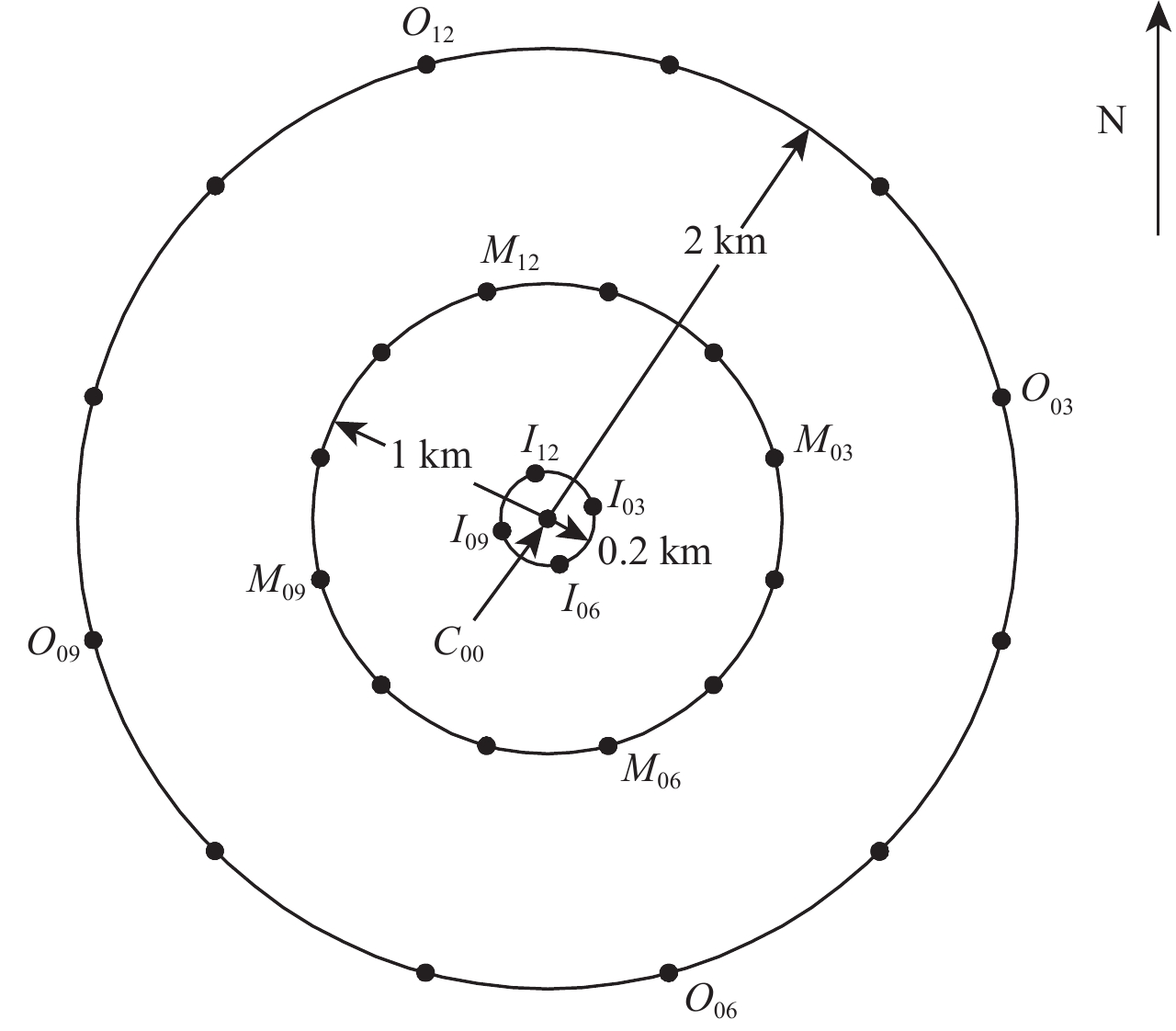
The influence of cut-off frequency on the statistical results of spatial coherency function of seismic ground motion
-
摘要: 以SMART-1台阵第45号地震的南北分量加速度作为研究对象，计算截止频率fcut分别为8，16和24 Hz，台站间距分别为200，1 000和2 000 m这9种工况的相干系数，并进行曲线拟合，再根据9组拟合参数计算相应的相干系数并进行比较。结果表明：① 截止频率fcut的取值对相干函数统计模型参数和相干系数拟合曲线有很大影响；② 根据较小的截止频率fcut得到的拟合参数计算出的相干系数随频率的衰减很快，反之，根据较大的截止频率fcut得到的拟合参数计算出的相干系数随频率的衰减较慢；③ 当使用由确定的截止频率fcut得到的相干系数拟合参数计算超出截止频率fcut范围的相干系数时，将产生很大的误差。Abstract: Based on the north-south component of the 45th seismic record on the SMART-1 station, in this paper, we firstly calculated the lagged coherencies under nine conditions which were combined with the three cut-off frequencies （8, 16, 24 Hz） and three separation distances （200, 1 000, 2 000 m）. Then, we fitted the curves. Finally, the coherencies received according to nine groups of fitting parameters were compared. The conclusion can be drawn as follows: ① The selection of the cut-off frequency fcut has great effect on the parameters of coherence function model and lagged coherency fitting curves. ② The lagged coherency calculated by the fitting parameters which obtained by smaller cut-off frequency fcut decays rapidly with increasing frequency; on the other hand, the lagged coherency calculated by the fitting parameters which obtained by larger cut-off frequency fcut decays slowly with increasing frequency. ③ Great errors will be produced when using fitting parameters of the lagged coherency that got from the determined cut-off frequencyfcut to calculate the lagged coherency beyond that frequency range.
-
引言
夏威夷群岛的火山喷发记录始于1790年，其中的大部分火山事件，都可以定位在群岛的东南方向，也就是地质年代形成最晚的夏威夷大岛（Mooney，2018）。该岛共包含五座盾状火山，其中基拉韦厄火山的持续喷发导致其成为全球火山研究的热点。Nettles和Ekström （2004）重新分析了1975年卡拉帕纳（Kalapana）地震的长周期数据，观察到该地震的震源机制解显示为向陆地方向倾覆的冲断层；Wright和Klein （2006）通过研究夏威夷活火山的岩浆运移通道，认为岩浆通道在30 km以下是垂直的，沿海洋地幔边界是近似水平的，在基拉韦厄顶峰下接近垂直（Wright，Klein，2006）；Benz等（2002）和Lin等（2014）在基拉韦厄火山地区通过反演地震速度结构，发现在基拉韦厄火山埋深4—12 km处孕育了一个8 km宽的复杂高波速管状岩体，岩浆通道近乎垂直，火山东部裂谷带下方8—11 km处存在异常体，具有低速纵波、低速横波和高纵横波速比的特点，认定其为岩浆囊（Lin et al，2014）；Lei和Zhao （2006）将穿过外核的震相用于新的全球三维层析成像，观察到夏威夷的地幔柱成像结果表现为地表到核幔边界间的连续低速异常。
在夏威夷深部构造研究方面，科研人员大多采用地震学手段，然而，大地电磁测深方法在深部构造探测特别是火山研究中具有独特的优势。地下电阻率对介质的温度、含水饱和度和熔融状态非常敏感，而地下电性的变化通常是由岩浆的喷出和补充等循环变化造成的，因此电磁监测对研究低阻岩浆囊的动态变化较为灵敏。Constable和Heinson （2004）在海底布设了7个海洋大地电磁仪器，反演结果显示，在地幔柱顶部150 km处出现一个约为10 Ω·m的低阻体，且地下的导电性是各向异性的，垂直电导率高于水平电导率；汤吉等（1997，2001）观测到在天池火山区12 km深处存在岩浆囊，并且在不同剖面上表现出不同形态，其团队之后在阿尔山活火山区进行大地电磁观测，反演结果显示，新、老两条火山带的岩浆通道在深部可能是同源的（汤吉等，2005）；仇根根等（2014）在天池火山区同一地点进行大地电磁反演，得到了浅部发育的多个岩浆囊的位置及其发展趋势；李世文等（2020）通过大地电磁反演得出东北地区新生代火山区的三维电性结构，认为在高阻岩石圈下，存在多个与新生代火山相对应的局部低阻异常；Aizawa等（2011）在日本樱岛进行连续大地电磁测量，认为岩浆挥发物与地下水之间的混合作用可能会导致电阻率在海平面附近的某个深度发生变化；Díaz等（2012）通过分析大地电磁数据和岩石特征，推断出拉斯卡尔火山和普那火山下存在向南部延伸的大规模高电导率带，安第斯山脉南部存在两个不同深度和组成的岩浆储层；Abdallah等（2020）对库车火山群进行大地电磁测量，得到了该火山群下方的三维电阻率结构以及浅部热液系统与深部岩浆通道之间的联系。这些研究成果充分证明了大地电磁方法在探测低阻火山岩浆囊方面拥有的独特优势。
因此，大地电磁测深方法可用于探测火山深部电性结构，了解地下岩浆囊的构造赋存形态以及岩浆上升通道及深度展布范围，为岩浆活动和火山的危险性预测提供电性数据支持。然而，我们对夏威夷岛的深部构造特别是电性结构认识还很不充分，因此本文拟对夏威夷地区的深部电性结构进行研究，以期为认识夏威夷岛的深部结构提供电性依据。
1. 野外数据处理与分析
1.1 数据来源
文中的大地电磁数据来源于加拿大凤凰公司在夏威夷岛上布设的长期观测站（图1），位置为（19.8°N，155.6°W），测点海拔为1 665 m。台站采集到的自然发生的地磁场和感应地球电流的频率范围为10 400—0.005 5 Hz，通过趋肤深度公式进行粗略计算，可知反演深度可达100 km，100 km深度以下的地层电性信息由于超低频观测数据质量太差而参考价值不高。
![]()
图 1 夏威夷岛的构造背景及测点位置Figure 1. The tectonic background and distribution of the MT station of Hawaii Island1.2 近期地震与火山事件
夏威夷群岛地震及火山活动频发，参考中国地震台网中心国家地震科学数据中心（2021）发布的全球地震动态，可知自2018年5月至2019年4月大约一年的时间里，夏威夷大岛发生的M≥5.0的火山喷发地震高达54次。在大岛上标注出54次地震的震中位置（图2），可以看出地震非常频繁并且集中，震中大多汇聚在基拉韦厄火山附近。表1为部分较大震级的地震群的活动记录，这些震群的地震类型可能包括了火山构造地震、低频地震以及颤动（陈洪洲等，2004）。这些地震事件或发生在裂谷系统下的浅部（深约1—4 km），或沿着火山口下垂直的岩浆通道附近发生，且多以震群形式出现，有空间集中和震级较小的特征（傅征祥，1991）。
![]()
图 2 2018年5月至2019年4月地震的震中位置Figure 2. The epicenters of earthquakes from May 2018 to April 2019表 1 夏威夷岛部分较大震级的地震事件Table 1. Some earthquakes with large magnitude on Hawaii Island发震日期 北纬/° 西经/° 深度/km mb 年-月-日 时:分 2018-05-03 20:30 19.34 155.07 6 5.1 2018-05-04 22:32 19.31 155.00 2 6.9 2018-06-08 12:44 19.41 155.28 −1 5.2 2018-07-02 11:24 19.39 155.27 −1 5.3 2019-03-13 10:55 19.33 155.20 7 5.5 2019-04-14 03:09 19.47 155.79 13 5.3 1.3 数据质量分析
本文中数据的处理及反演使用的是MTPioneer软件。2018年7月15日的原始资料2018715M，2018715N，2018715O的视电阻率曲线如图3所示。其中：12个月份对应记为1—9，A，B，C；日期最后一位字母A-X分别对应了一天中的24个小时。2018年7月15日13时26分，夏威夷岛上发生了mb5.2地震，图3b的2018715N表示地震发生时记录到的视电阻率Rxy，低频段的数据质量很差，不能参与反演，图3a，c中2018715M和2018715O分别表示震前、震后记录到的视电阻率Rxy，其中中频部分的数据质量较好，低频的部分频点出现了飞点现象，高频部分出现整段的上移。由于本文主要关注中深层的电性信息，因此选择了频率小于629 Hz的频点进行反演。反演之前对部分受干扰严重的数据进行了编辑、圆滑、剔除等操作，使其达到反演要求。
![]()
图 3 原始数据中视电阻率Rxy的质量日期最后一位字母A−X分别对应一天中的24时，下同Figure 3. The quality of resistivity Rxy for the original dataThe last letters A-X of the date correspond to 24 hours in a day, the same below（a） 2018715M；（b） 2018715N；（c） 2018715O2. 大地电磁一维反演与讨论
对大地电磁数据进行自适应正则化一维反演，获得了观测台站下方深部电性结构及其长期变化特征。本文反演所用的阻抗数据均为Rxy，反演过程中，设置截断误差为1，最大迭代次数为50，反演初始模型为均匀半空间。
2.1 地震及火山活动前后电性特征分析
许多研究表明，夏威夷的地震活动直接或间接地与火山活动过程有密切关系。图4给出了三种典型火山地震发生前后的地下电性变化曲线，深度范围大约为0.5—130 km。给视电阻率加5%的误差，可以看出地震前后两条曲线基本无重叠部分，证明了视电阻率随时间变化的可行度。由图4可见，随着深度的增加，电阻率从地表向深处开始快速下降，到了2—3 km深度处，电阻率降低到最小值约20 Ω·m，与实测的熔融玄武岩熔岩的电阻率（大约为40 Ω·m）相近（Bartel et al，1983），推测其可能为低阻岩浆囊位置之一。在3 km深度以下，地震前后的电性差异出现明显变化，而且变化趋势相似，即电阻率先升高后降低，到了约100 km深度处逐渐趋于稳定的低阻异常，推测该深度下可能存在复杂的岩浆结构。
![]()
图 4 火山地震发生前后地下电性变化示意图图（b）−（d）线条上的短线为误差棒，误差棒的长短表示误差大小（a） 无地震发生；（b） 2018年5月3日；（c） 2018年5月4日；（d） 2018年6月8日Figure 4. Schematic diagram of underground electrical changes before and after volcanic earthquakesThe short line on the curves of Figs.（b）−（d） is error bar，and the length of the bar represents the error size（a） No earthquake；（b） May 3，2018；（c） May 4，2018；（d） June 8，2018火山活动与深部岩浆运输和物质熔融存在着密不可分的关系，火山喷发和强烈的浅层地震活动诱发了地下电阻率的变化，这是因为裂缝的发育会导致岩石中流体含量及其连通性发生变化，而岩浆和气体上升迁移又会导致岩石破裂。一般来说，熔融或部分熔融后的岩浆的电阻率值很低，地下电性变化通常对应着岩浆物质的喷出、补充等构造变化，但岩浆的上升通道和储存路径等细节却不明确。图4a表示2019年9月1日在没有地震发生的情况下，相近三个小时内的地下电性变化曲线，电阻率有变化但不明显，由此可证实电阻率变化的确与地震相关；图4b表示2018年5月3日地震发生前后深部电性变化，推测可能是由于火山型地震改变了岩浆输送通道，部分岩浆被挤压进入其它通道，导致地震后的电阻率大于地震前的电阻率；图4c表示2018年5月4日基拉韦厄南翼mb6.9地震前后的地下深部电性变化，可见其电性变化特征从浅至深在不同深度上都与2018年5月3日有所差异，随着深度的增加，电性差异逐渐增大，特别到了20 km以下，火山喷发后的电阻率明显高于喷发前的电阻率，可能是由于该层位大量的岩浆被抽送或运移至东南方向，导致了电阻率的升高；图4d表示2018年6月8日地震发生前后的电阻率变化，观测点下方100 km深度处，地震前后的电阻率变化差异不大，可能是此次地震对地下地层结构的影响较小，岩浆的喷出和补充变化不明显或者喷出与补充达到了一种动态平衡。Aizawa等（2011）通过对比在日本樱岛采集的视电阻率时间序列和多种地球物理数据，认为视电阻率的长期变化与潮汐、降水量之间无显著关联，故本文不考虑潮汐和降水对夏威夷岛地下视电阻率变化的影响。
2.2 夏威夷地区深部长期电性变化特征
为分析大岛地区较长时期内的深部电性变化特征，本文选择了连续18个月（2018年4月至2019年9月中每月第4日，或距4日最近一天）采集的大地电磁数据进行反演，选择典型的7条反演曲线进行展示（图5）。Díaz等（2020）认为复杂的岩浆系统具有多层次的储集能力，可能由不同深度和组分的储层组成。夏威夷地区的反演结果显示出两处明显的低阻异常，在观测点下方2—3 km处存在一个薄岩浆层，电阻率大约为20 Ω·m，该层位正对应了大岛裂谷系统中地震频发的深度，这些震群是由于岩浆囊在通道中的体积变化或压强变化引起应力集中的结果；在3—100 km深度范围内，视电阻率曲线随深度增加整体呈现先增大再逐渐降低的特征，大约在30—300 Ω·m之间变化，且在10—100 km深度范围内电性变化较为明显，推测为岩浆通道。该深度范围与Matoza等（2021）探测到的深度在20—50 km之间的横向连续地震活动扩散带相对应。而100 km深度左右电阻率变化范围在20—70 Ω·m，结合Constable和Heinson （2004）的反演认为地幔柱在40—400 km存在一个低电阻体，电阻率约为10 Ω·m。Witze （2013）认为在地下约110—155 km处存在一个暖池，该暖池并非位于夏威夷主岛的正下方，而是在主岛西侧100 km处，推测100 km以下可能存在大型的复杂岩浆储层，为上方岩浆囊提供深部热源（张炯等，2017）。
![]()
图 5 2018年5月至2019年9月测点下方的电阻率变化示意图Figure 5. Schematic diagram of resistivity change under the station from May 2018 to September 2019结合夏威夷岛的地震动态和每月的电性变化特征进行分析，可以看出在地震和火山活动频发的2018年5—7月份，电阻率呈上升趋势（图6）。地震的电阻率效应源于微裂隙内孔隙流体的变化，故推测地震余震期间电阻率的增加趋势是由震后应变释放区应力恢复和孔隙度恢复所致（王立凤等，2017）。
![]()
图 6 2018年4月至8月测点下方的电阻率变化示意图Figure 6. Schematic diagram of resistivity change under the station from April to August，20183. 结论
基于加拿大凤凰公司在夏威夷大岛上布设的长期大地电磁观测台站所采集的数据，对该地区开展了大地电磁反演研究，获取了其深部电性结构，单个台站得到的结果仅对于该测点下方是合理的，可能不能代表远离该测点下方的地下结构特征。结合该地区一年来发生的多次火山地震事件，发现观测点下方约2—3 km处存在明显的低阻岩浆囊，100 km以下可能是热源储层。研究表明，使用长期大地电磁观测台站可以对火山下方的电性结构特征进行长期的动态监测，大地电磁勘探对于认识和掌握火山区深部构造及变化特征具有重要的参考价值。本文仅得到了对夏威夷大岛的单点初步探测结果，要进一步了解大岛的深部电性结构及其动态变化特征，仍需进行更大范围和更长期的观测和研究。
本研究数据来自加拿大凤凰地球物理公司的公开数据，作者在此表示感谢。
-
![]()
图 2 第45号地震在台站C00处的NS向加速度时程及S波时间窗
Figure 2. Acceleration traces of NS and EW components for the 45th event recorded at station C00 and the S wave time window
![]()
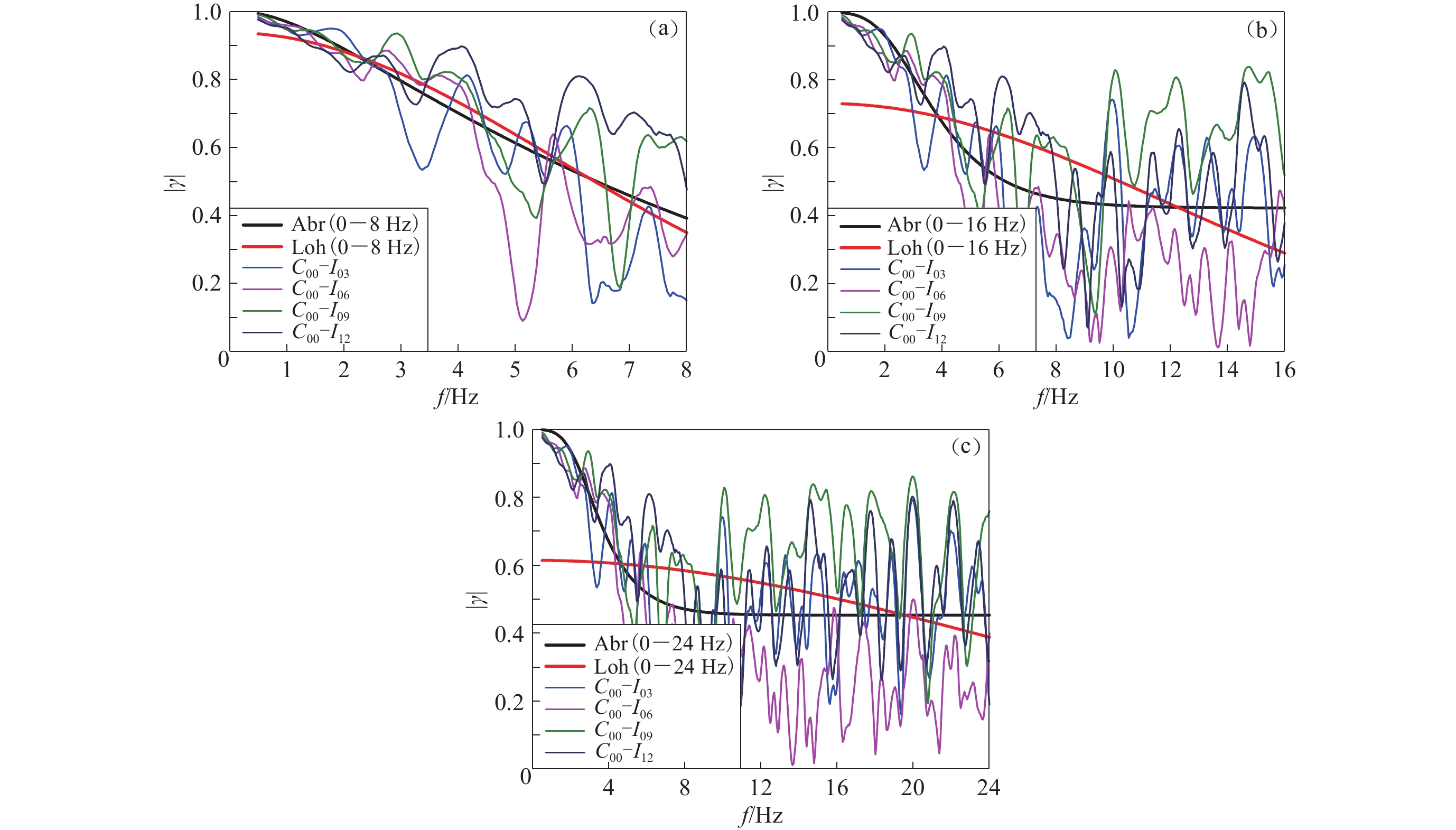
图 5 台站间距d＝2 000 m，截止频率fcut为8 Hz （a），16 Hz （b） 和24 Hz （c） 时的相干系数 |γ| （细实线）及其根据Abrahamson相干函数模型（黑粗线）和Loh相干函数模型（红粗线）拟合所得的拟合曲线
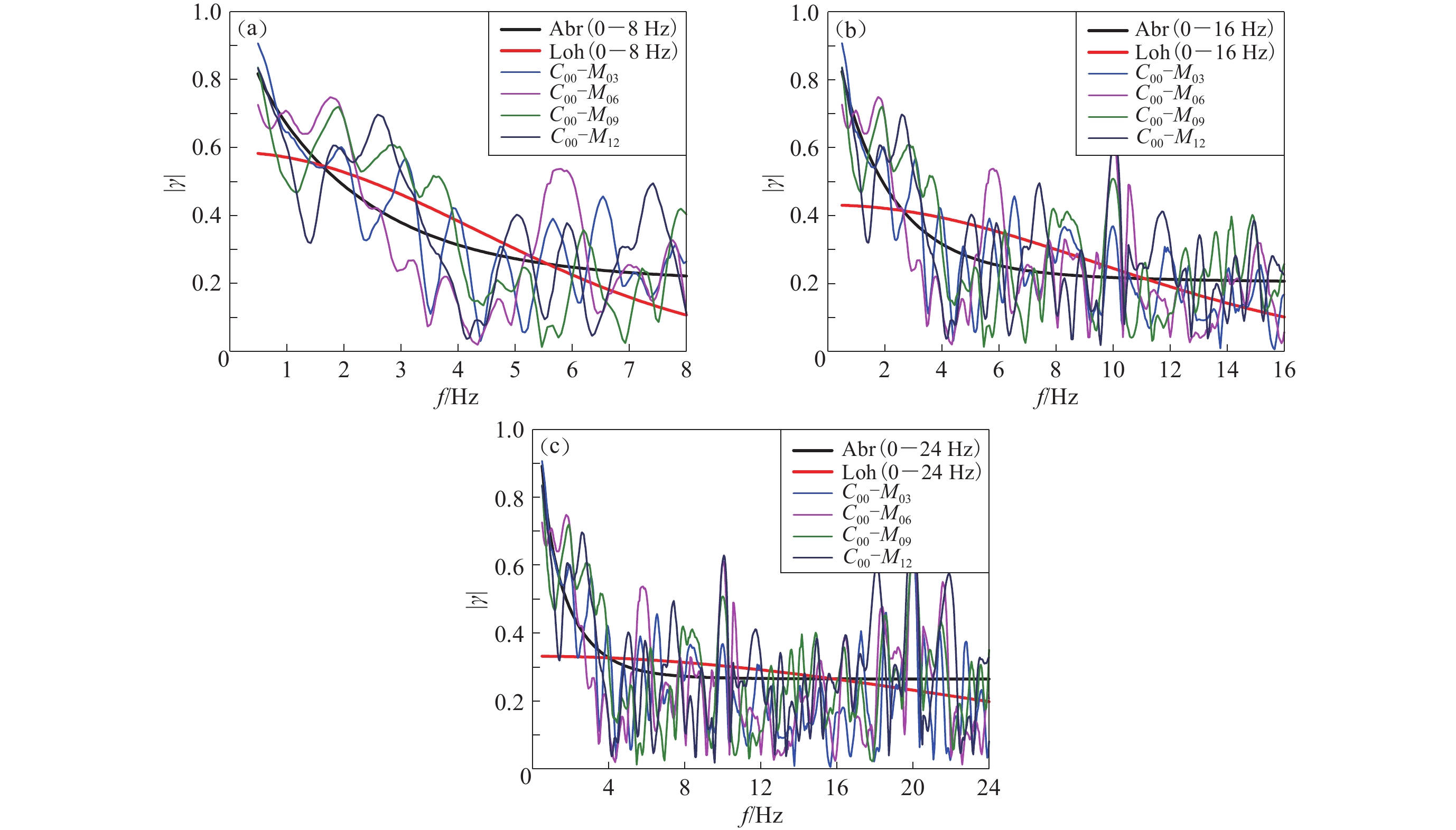
Figure 5. Lagged coherencies |γ| at station spacing d＝2 000 m （thin solid line） and the corresponding fitting curves based on Abrahamson （black rough line） and Loh （red rough line） coherence function model which obtained with different cut-off frequency values of 8 Hz （a），16 Hz （b） and 24 Hz （c）
![]()
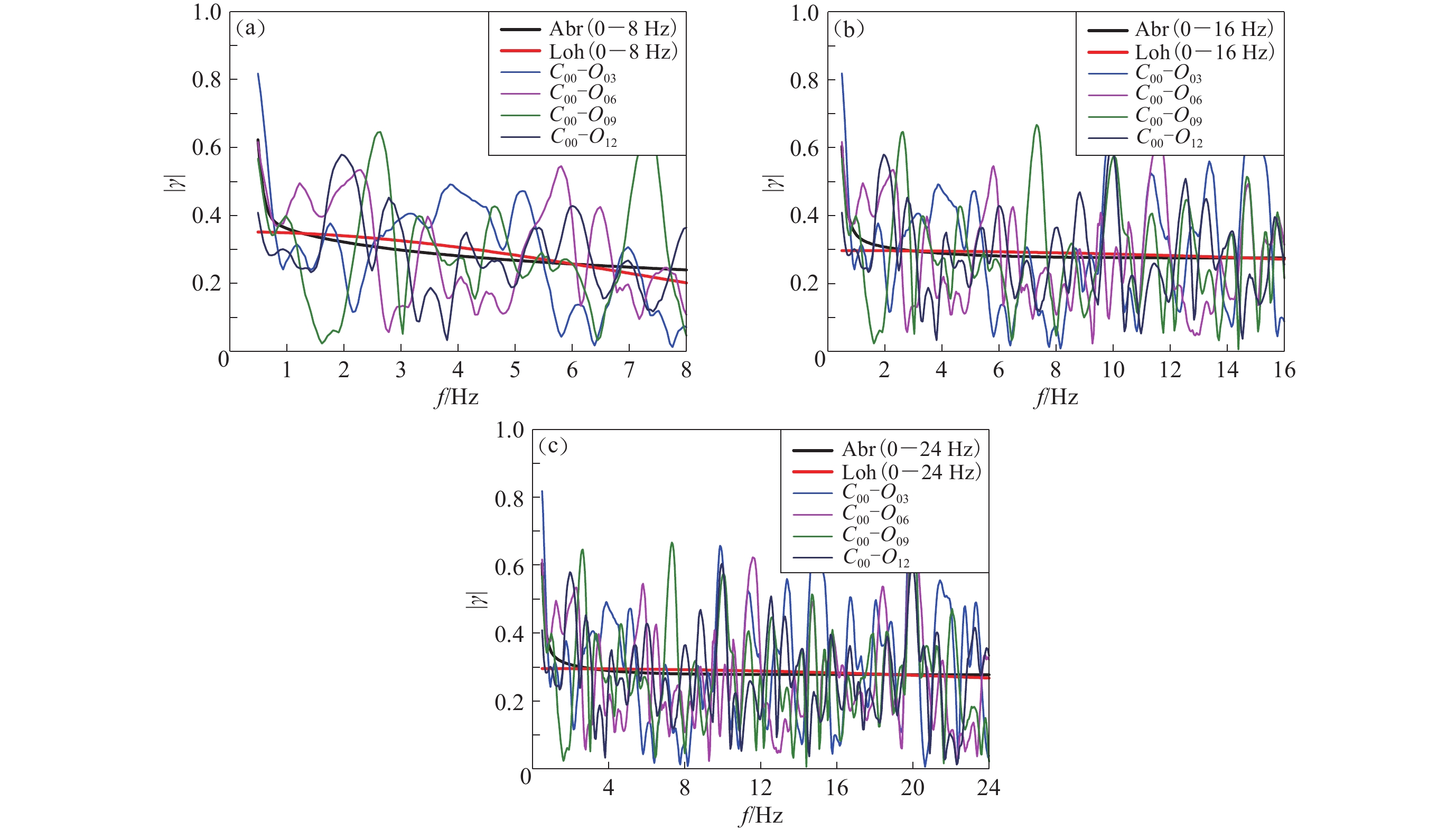
图 3 台站间距d＝200 m，截止频率fcut为8 Hz （a），16 Hz （b） 和24 Hz （c）时的相干系数 |γ| （细实线）及其根据Abrahamson相干函数模型（黑粗线）和Loh相干函数模型（红粗线） 拟合所得的拟合曲线
Figure 3. Lagged coherencies |γ| at station spacing d＝200 m （thin solid line） and the corresponding fitting curves based on Abrahamson （black rough line） and Loh （red rough line） coherence function model which obtained with different cut-off frequency values of 8 Hz （a），16 Hz （b） and 24 Hz （c）
![]()
图 4 台站间距d＝1 000 m，截止频率fcut为8 Hz （a），16 Hz （b） 和24 Hz （c） 时的相干系数 |γ| （细实线）及其根据Abrahamson相干函数模型（黑粗线）和Loh相干函数模型（红粗线）拟合所得的拟合曲线
Figure 4. Lagged coherencies |γ| at station spacing d＝1 000 m （thin solid line） and the corresponding fitting curves based on Abrahamson （black rough line） and Loh （red rough line） coherence function model which obtained with different cut-off frequency values of 8 Hz （a），16 Hz （b） and 24 Hz （c）
![]()
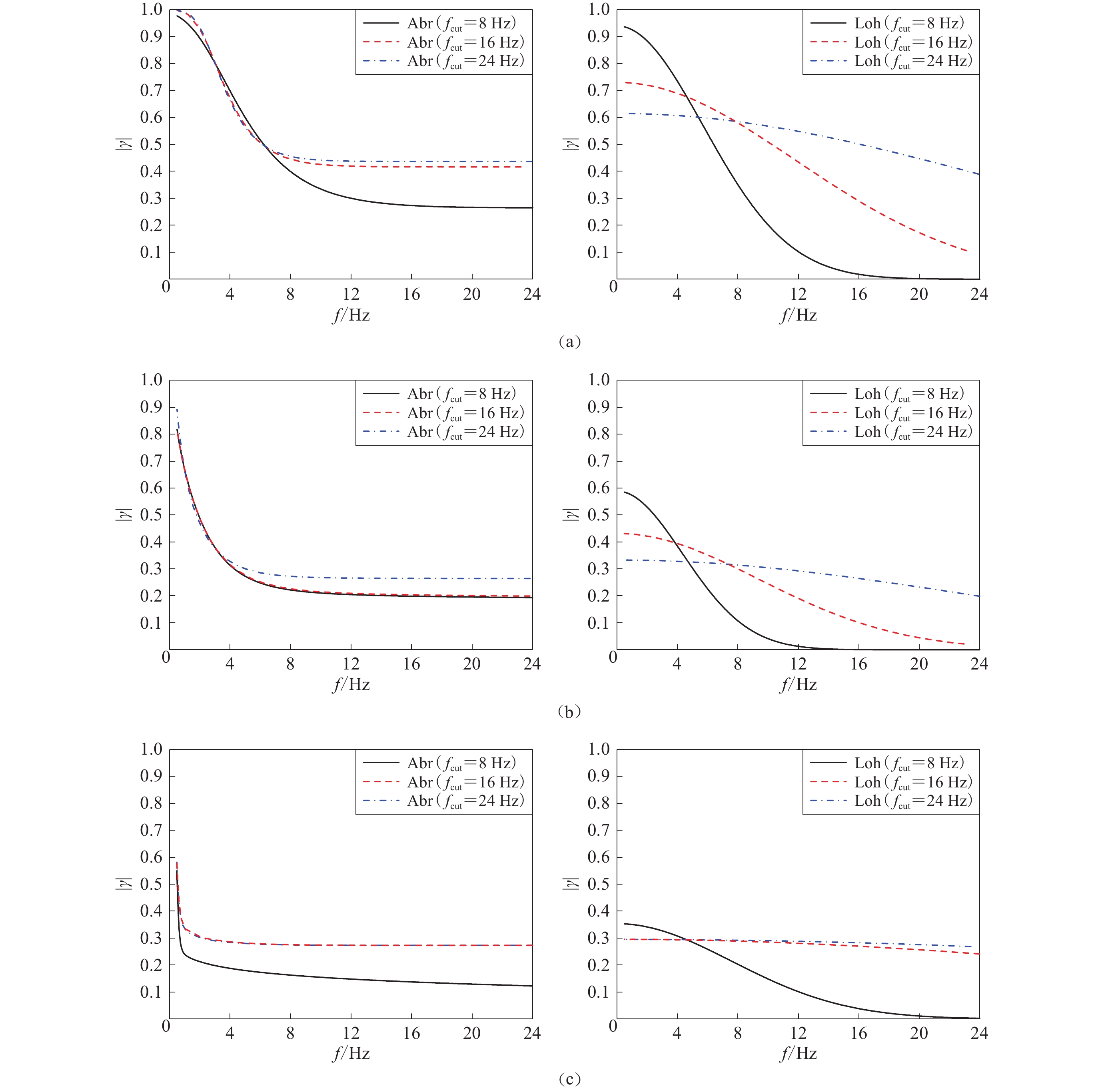
图 6 根据不同截止频率fcut所得的拟合参数计算得到的台站间距d＝200 m （a），1 000 m （b） 和2 000 m （c） 时的相干系数|γ|
左侧对应于Abr相干函数模型的结果，右侧对应于Loh相干函数模型的结果
Figure 6. Coherencies at separation distance d＝200 m （a），1 000 m （b），2 000 m （c） based on fitting parameters which obtained from different cut-off frequency values
The graphs on the left correspond to the result of the Abrahamson，and the graphs on the right correspond to the result of the Loh
表 2 基于Loh相干函数模型得到的拟合参数
Table 2 Fitting parameters based on Loh coherence function model
台站间距
d/m截止频率
fcut/Hz系数 a b 200 8 0.32 1.95×10−3 16 1.57 4.57×10−3 24 2.43 1.01×10−4 1 000 8 0.53 6.73×10−4 16 0.84 1.44×10−4 24 1.10 2.27×10−5 2 000 8 0.52 1.10×10−4 16 0.61 4.46×10−6 24 0.61 2.18×10−6 
下载: 导出CSV
表 1 基于Abrahamson相干函数模型得到的拟合参数
Table 1 Fitting parameters based on Abrahamson coherence function model
台站间距
d/m截止频率
fcut/Hz系数 a1 a2 b1 b2 c n 200 8 −0.65 0.013 2.31 −1.34×10−2 −1.604 0.33 16 2.36 1.17×10−3 1.29 −9.39×10−3 −1.148 0.39 24 −0.16 9.74×10−3 −0.24 −1.71×10−3 −0.587 0.36 1 000 8 0.95 −2.70×10−4 0.62 1.16×10−3 −0.942 0.19 16 1.05 −3.76×10−4 −0.54 6.04×10−6 −0.988 0.20 24 0.62 −1.62×10−5 0.59 1.20×10−3 −1.778 0.27 2 000 8 −0.91 5.78×10−4 −0.02 −2.31×10−5 −1.570 0.10 16 1.06 −4.96×10−4 −3.10 1.53×10−3 −3.970 0.24 24 2.66 −1.27×10−3 −0.052 2.23×10−5 −3.203 0.18
下载: 导出CSV
表 3 根据不同截止频率fcut拟合得到的相干系数在0—8 Hz频段内的变化
Table 3 Changes of the lagged coherencies obtained with different fcut in the 0—8 Hz frequency band
台站间距
d/m0—8 Hz内的相干系数 Abr相干函数模型 Loh相干函数模型 fcut＝8 Hz fcut＝16 Hz fcut＝24 Hz fcut＝8 Hz fcut＝16 Hz fcut＝24 Hz 200 0.99—0.39 0.99—0.45 0.99—0.47 0.93—0.33 0.73—0.58 0.61—0.58 1 000 0.78—0.22 0.78—0.23 0.79—0.24 0.58—0.11 0.43—0.29 0.33—0.31 2 000 0.62—0.24 0.60—0.28 0.60—0.28 0.35—0.20 0.29—0.28 0.30—0.29
下载: 导出CSV
-
阿布都瓦里斯,俞瑞芳,俞言祥. 2013. 基于汶川地震加速度记录的地震动相干函数变化特性[J]. 振动与冲击,32(16):70–75 Abuduwalisi,Yu R F,Yu Y X. 2013. Spatial variation of ground motions based on Wenchuan acceleration records[J]. Journal of Vibration and Shock,32(16):70–75 (in Chinese)
陈厚群. 1997. 当前我国水工抗震中的主要问题和发展动态[J]. 振动工程学报,10(3):253–257 Chen H Q. 1997. The main problems and developments in seismic studies on hydraulic structures in China[J]. Journal of Vibration Engineering,10(3):253–257 (in Chinese)
丁海平,刘启方,金星,袁一凡. 2004. 基岩地震动的一个相干函数模型:走滑断层情形[J]. 地震学报,26(1):62–67 Ding H P,Liu Q F,Jin X,Yuan Y F. 2004. A coherency function model of ground motion at base rock corresponding to strike-slip fault[J]. Acta Seismologica Sinica,26(1):62–67 (in Chinese)
冯启民,胡聿贤. 1981. 空间相关地面运动的数学模型[J]. 地震工程与工程振动,1(2):1–8 Feng Q M,Hu Y X. 1981. Mathematic model of spatial correlative ground motion[J]. Earthquake Engineering and Engineering Dynamics,1(2):1–8 (in Chinese)
李英民,吴哲骞,陈辉国. 2013. 地震动的空间变化特性分析与修正相干模型[J]. 振动与冲击,32(2):164–170 Li Y M,Wu Z Q,Chen H G. 2013. Analysis and modeling for characteristics of spatially varying ground motion[J]. Journal of Vibration and Shock,32(2):164–170 (in Chinese)
刘先明,叶继红,李爱群. 2004. 竖向地震动场的空间相干函数模型[J]. 工程力学,21(2):140–144 Liu X M,Ye J H,Li A Q. 2004. Space coherency function model of vertical ground motion[J]. Engineering Mechanics,21(2):140–144 (in Chinese)
屈铁军,王君杰,王前信. 1996. 空间变化的地震动功率谱的实用模型[J]. 地震学报,18(1):55–62 Qu T J,Wang J J,Wang Q X. 1996. A practical model for the power spectrum of spatially variant ground motion[J]. Acta Seismologica Sinica,18(1):69–79 (in Chinese)
王君杰,王前信,江近仁. 1995. 大跨拱桥在空间变化地震动下的响应[J]. 振动工程学报,8(2):119–126 Wang J J,Wang Q X,Jiang J R. 1995. Random response of long-span arch bridge under spatially variable seismic excitations[J]. Journal of Vibration Engineering,8(2):119–126 (in Chinese)
Abrahamson N A,Bolt B A,Darragh R B,Penzien J,Tsai Y B. 1987. The SMART-1 accelerograph array (1980−1987):A review[J]. Earthquake Spect,3(2):263–287
Abrahamson N A,Schneider J F,Stepp J C. 1991. Empirical spatial coherency functions for applications to soil-structure interaction analyses[J]. Earthquake Spect,7(1):1–27
Ghobarah A,Aziz T S,EI-Attar M. 1996. Response of transmission lines to multiple support excitation[J]. Eng Struct,18(12):936–946
Hao H. 1989. Effects of Spatial Variation of Ground Motions on Large Multiply-Supported Structures[R]. Berkeley: University of California: 18–27.
Hao H,Oliveira C S,Penzien J. 1989. Multiple-station ground motion processing and simulation based on SMART-1 array data[J]. Nucl Eng Des,111(3):293–310
Hao H. 1993. Arch responses to correlated multiple excitations[J]. Int J Earthq Eng Struct Dynam,22(5):389–404
Harichandran R S,Vanmarcke E H. 1986. Stochastic variation of earthquake ground motion in space and time[J]. J Eng Mechan,112(2):154–174
Loh C H,Lin S G. 1990. Directionality and simulation in spatial variation of seismic waves[J]. Eng Struct,12(2):134–143
Matsushima Y. 1975. Spectra of spatially variant ground motions and associated transfer functions of soil-foundation system[J]. Transaltions of AIJ,232:63–69
Oliveira C S. 1985. Variability of strong ground motion characteristics obtained in SMART-1 Array[C]//Proceeding of 12th Regional Seminar on Earthquake Engineering. Halkidiki, Greece.
Zerva A. 2009. Spatial Variation of Seismic Ground Motions[M]. Boca Raton: Taylor and Francis Group: 10–58.
Zerva A,Harada T. 1997. Effect of surface layer stochasticity on seismic ground motion coherence and strain estimates[J]. Soil Dynam Earthquake Eng,16(7/8):445–457
-
期刊类型引用(0)
其他类型引用(2)
计量
- 文章访问数: 1428
- HTML全文浏览量: 608
- PDF下载量: 35
- 被引次数: 2
