Determining the threshold value in upper limit of wavenumber integration by reflection-transmission coefficient in theoretical seismograms calculation
-
摘要: 本文尝试基于理论分析来求解积分限阈值kc，即通过反透射系数来确定kc。根据计算理论地震图的广义反透射系数法，在反透射系数中进行求逆运算的矩阵行列式的零点将会使得被积函数产生较大的变化，通过具体实例显示出自由界面处的反射系数中含有的零点可以作为合适的kc。多种情况的实例显示，通过反射系数来确定kc具有较好的普适性。通过与经验公式的计算结果进行比对，表明根据本文方案所确定的kc在保证准确性的同时可以有效地提高计算效率。Abstract: In multi-layered half-space, the displacement field is expressed as the integration of wavenumber k using reflection-transmission (R/T) coefficient when the seismogram is calculated in numerical ways in the frequency domain. Therefore, some special methods were introduced to solve those kinds of integration as to accelerate computation. For example, when the depth of source is equal or close to that of receiver, the peak-trough averaging method (PTAM) is applied. To apply PTAM, however, one must determine the threshold value called kc after which the integration oscillates regularly. In previous studies, kc was estimated empirically without theoretical support. In this study, a scheme based on theoretical analysis to determine kc is proposed, and kc is related to the R/T coefficients. According to the generalized R/T coefficient method, violent variation of integrand will occur when the determinant of relevant R/T coefficient vanishes. We show by examples that for a given frequency, root of the determinant of the reflection coefficient on the free surface is a proper value for kc. Compared with the method calculated with empirical formula, the new method to determine kc will be more efficient for a given precision to prove the validity.
-
-
![]()
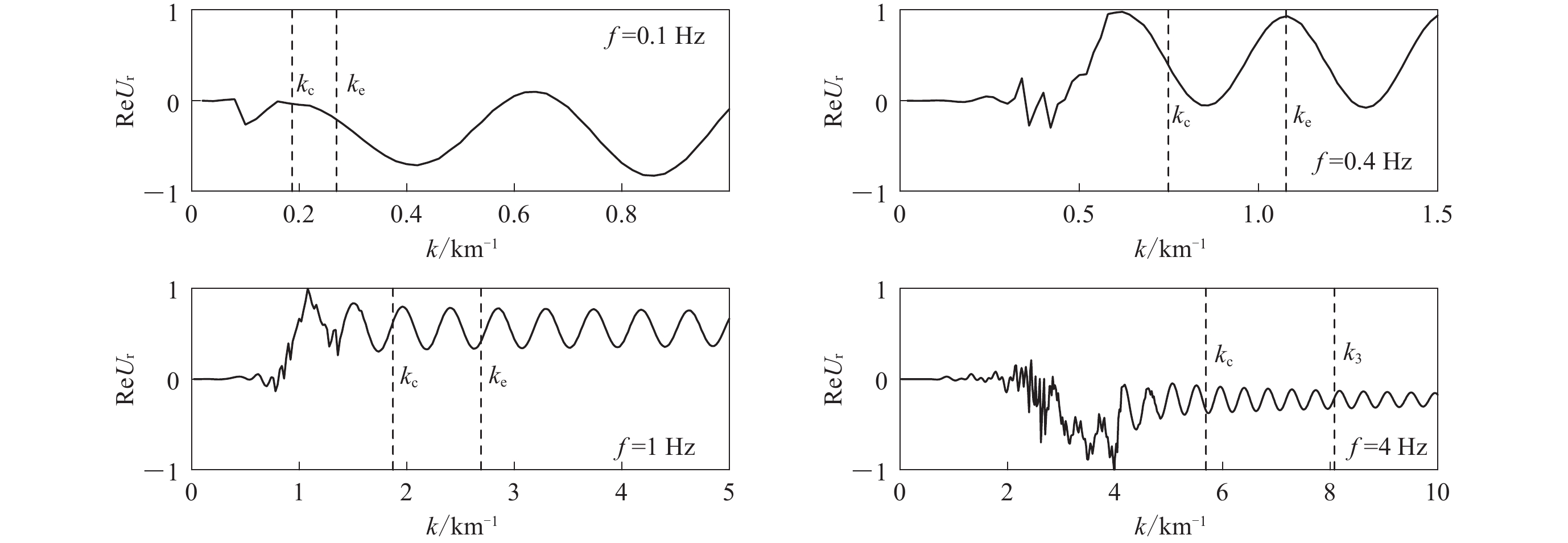
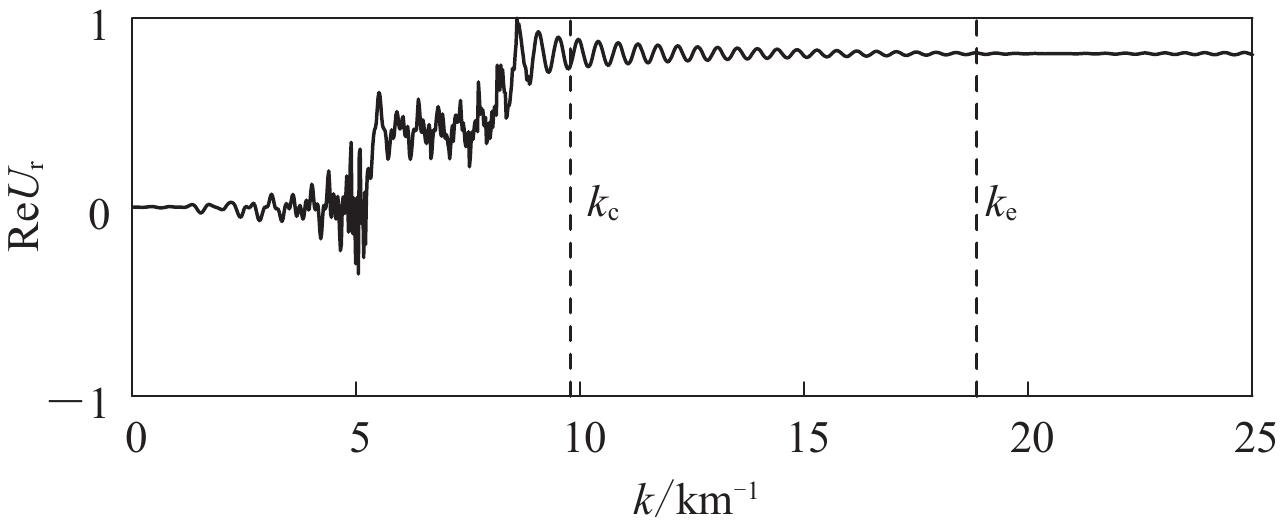
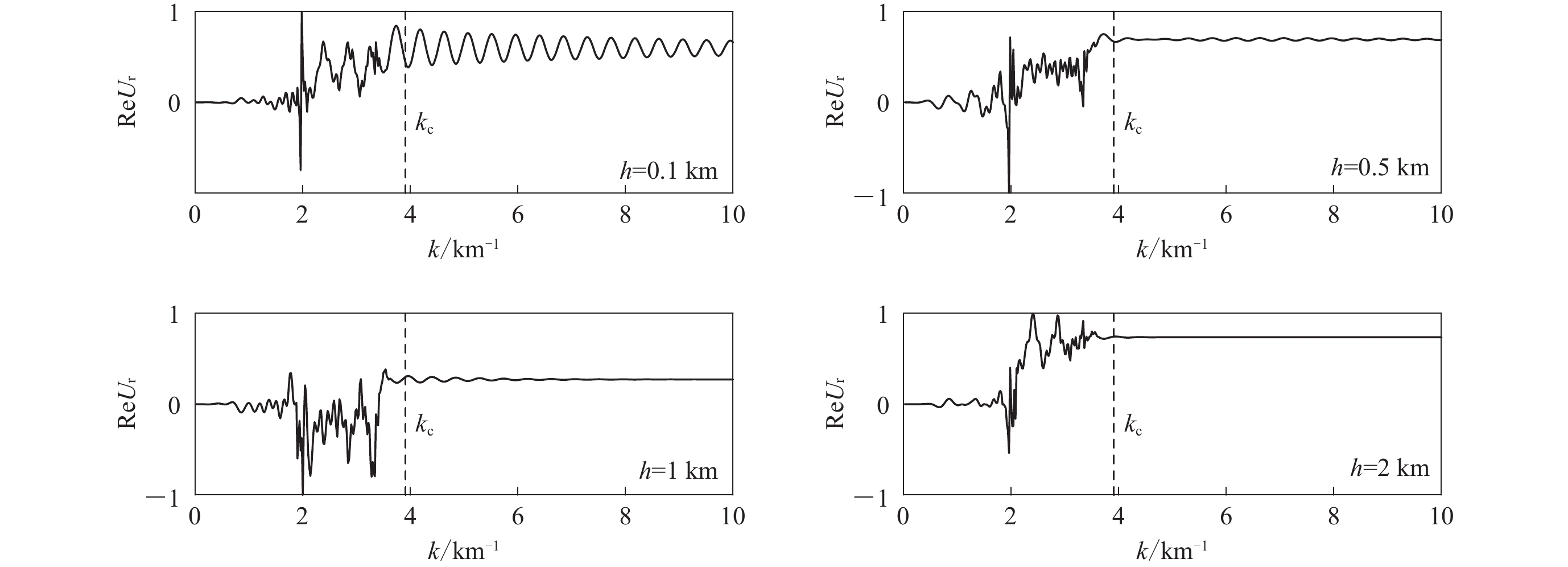
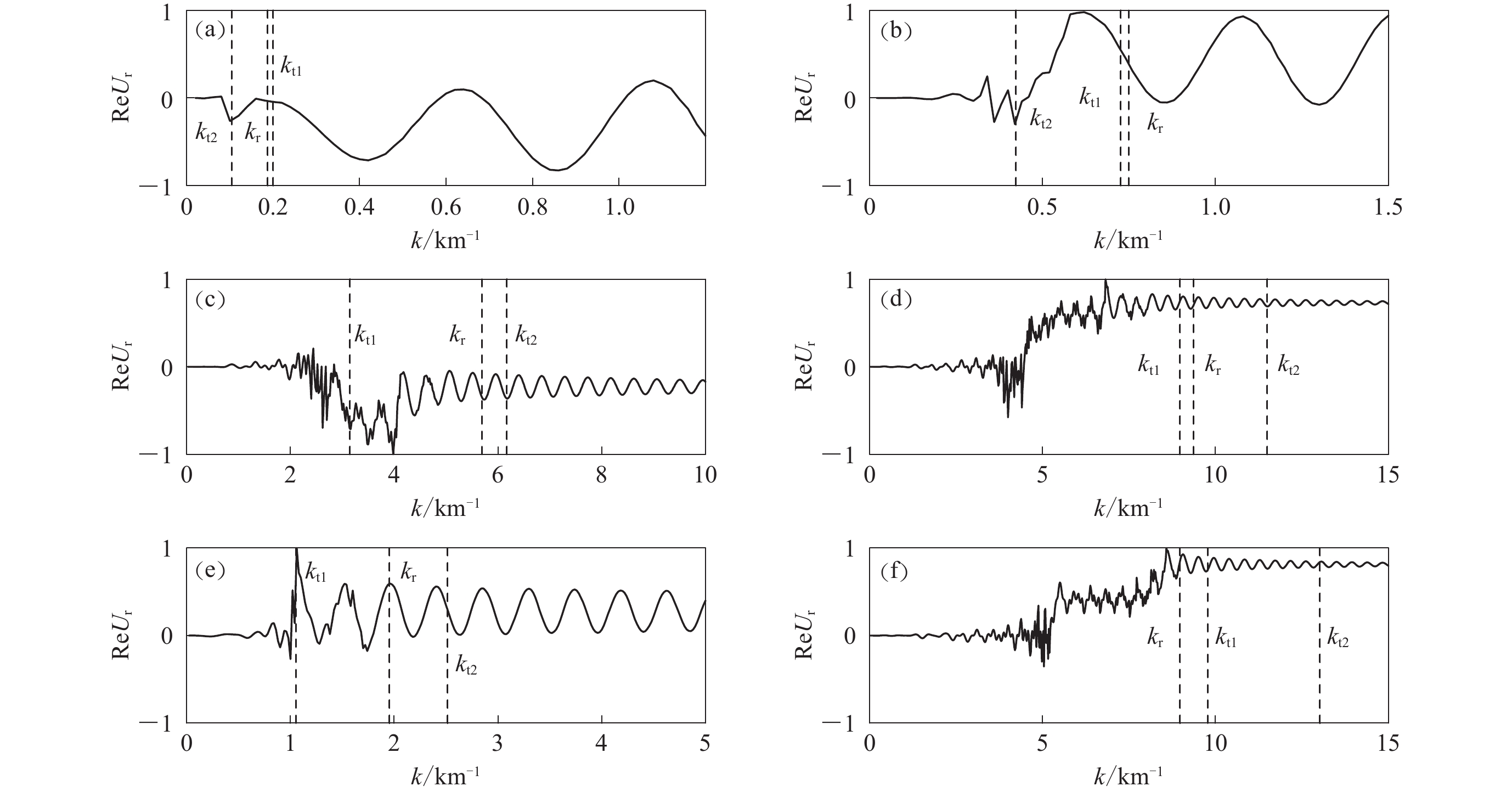
图 1 不同频率下反透射系数的零点与位移的关系（位移Ur为归一化后的结果）
图（a）−（d）分别对应f＝0.1，0.4，3，5 Hz的不含低速层的三层地壳模型；图（e）和（f）分别对应f＝1，5 Hz的含低速层的五层地壳模型
Figure 1. The relationship between zeros of R/T coefficient and the displacement in different frequencies with the normalized displacement Ur
Figs. （a） to （d） correspond to the three-layer crust model without low-velocity-layers when f＝0.1，0.4，3 and 5 Hz；Figs. （e） and （f） correspond to the five-layer crust model with low-velocity-layers when f＝1 Hz and 5 Hz
![]()
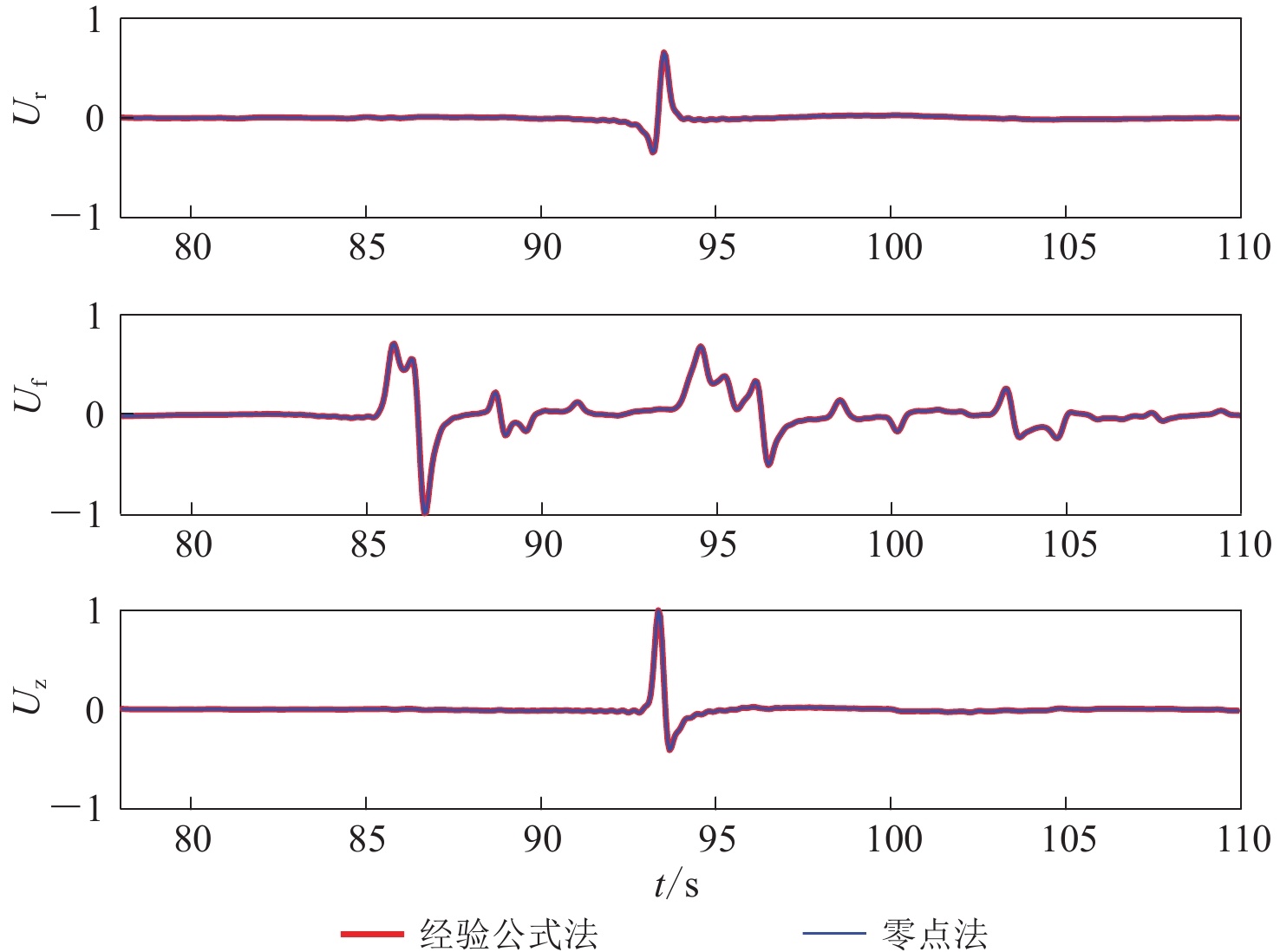
图 2 f＝2 Hz时波速递增的三层地壳模型下位移三分量的实部、虚部图（位移U为归一化后的结果）
图（a），（b）分别为径向位移Ur的实部和虚部；图（c），（d）分别为切向位移Uf的实部和虚部；图（e），（f）分别为垂向位移Uz的实部和虚部
Figure 2. The real part and image part of three components of displacement in the three-layer crust model without low-velocity-layers with the normalized displacement U when f＝2 Hz
Figs. （a） and （b） are the real part and image part of the radial direction displacement （Ur），respectively；Figs. （c） and （d） are the real part and image part of the tangential displacement （Uf），respectively；Figs. （e） and （f） are the real part and image part of the vertical displacement （Uz），respectively
![]()
图 3 波速递增的三层地壳模型在不同频率下ke与kc的关系（位移Ur为归一化后的结果）
Figure 3. The relationship between kc and ke at different frequencies in the three-layer crust model without low-velocity-layers with the normalized displacement Ur
![]()
图 4 f＝5 Hz时含低速层的五层地壳模型下ke与kc的关系（位移Ur为归一化后的结果）
Figure 4. The relationship between ke and kc in the five-layer crust model with low-velocity-layers while the displace-ment has been normalized when f＝5 Hz
![]()
图 5 利用求零点法和经验公式法分别获得的理论地震图径向位移Ur （a）、切向位移Uf （b）和垂向位移Uz （c）三分量归一化图
Figure 5. Seismogram calculated by for-zero method and empirical-formula method respectively to obtain the radial direction displacement Ur，the tangential displacement Uf，and the vertical displace-ment Uz，which are normalized
![]()
图 6 波速递增的三层地壳模型下位移在不同震源与接收点相对深度h时的变化图（位移Ur为归一化后的结果）
Figure 6. Relative depth between source and receiver in the three-layer crust model without low-velocity-layers while the displacements Ur have been normalized
表 1 不含低速层的三层地壳模型参数 （Chen，1993）
Table 1 Parameters of the three-layer crust model without low-velocity-layers（after Chen，1993）
层序 层底深度/km ρ/（g·cm−3） vS/（km·s−1） vP/（km·s−1） 1 20 2.8 3.50 6.0 2 30 2.9 3.65 6.3 3 45 3.1 3.90 6.7 4 ∞ 3.3 4.70 8.2 
下载: 导出CSV
表 2 含低速层的五层地壳模型的参数（何耀锋等，2006）
Table 2 Parameters of the five-layer crust model with low-velocity-layers（after He et al, 2006）
层序 层底深度/km ρ/（g·cm−3） vS/（km·s−1） vP/（km·s−1） 1 20 2.8 3.50 6.0 2 30 2.9 3.65 6.3 3 45 3.5 2.50 4.9 4 70 4.0 3.80 6.5 5 90 3.2 4.50 7.6 6 ∞ 2.4 5.60 9.3
下载: 导出CSV
-
何耀锋,陈蔚天,陈晓非. 2006. 利用广义反射-透射系数方法求解含低速层水平层状介质模型中面波频散曲线问题[J]. 地球物理学报,49(4):1074–1081. doi: 10.3321/j.issn:0001-5733.2006.04.020 He Y F,Chen W T,Chen X F. 2006. Normal mode computation by the generalized reflection-transmission coefficient method in planar layered half space[J]. Chinese Journal of Geophysics,49(4):1074–1081 (in Chinese).
张海明,陈晓非,张似洪. 2001. 峰谷平均法及其在计算浅源合成地震图中的应用[J]. 地球物理学报,44(6):805–813. doi: 10.3321/j.issn:0001-5733.2001.06.010 Zhang H M,Chen X F,Zhang S H. 2001. Peak-trough averaging method and its application to calculation of synthetic seismograms with shallow focuses[J]. Chinese Journal of Geophysics,44(6):805–813 (in Chinese).
Apsel R J,Luco J E. 1983. On the Green’s functions for a layered half-space. Part II[J]. Bull Seismol Soc Am,73(4):931–951.
Capdeville Y,Chaljub E,Montagner J P. 2003. Coupling the spectral element method with a modal solution for elastic wave propagation in global earth models[J]. Geophys J Int,152(1):34–67. doi: 10.1046/j.1365-246X.2003.01808.x
Chen X F. 1993. A systematic and efficient method of computing normal modes for multilayered half-space[J]. Geophys J Int,115(2):391–409. doi: 10.1111/gji.1993.115.issue-2
Chen X F. 1999. Seismogram synthesis in multi-layered half-space Part I. Theoretical formulations[J]. Earthquake Research in China,13(2):149–174.
Fuchs K,Müller G. 1971. Computation of synthetic seismograms with the reflectivity method and comparison with observations[J]. Geophys J Int,23(4):417–433. doi: 10.1111/j.1365-246X.1971.tb01834.x
Graves R W. 1996. Simulating seismic wave propagation in 3D elastic media using staggered-grid finite differences[J]. Bull Seismol Soc Am,86(4):1091–1106.
Helmberger D V. 1973. On the structure of the low velocity zone[J]. Geophys J Int,34(2):251–263. doi: 10.1111/j.1365-246X.1973.tb02395.x
Helmberger D V. 1974. Generalized ray theory for shear dislocations[J]. Bull Seismol Soc Am,64(1):45–64.
Hisada Y. 1994. An efficient method for computing Green’s functions for a layered half-space with sources and receivers at close depths[J]. Bull Seismol Soc Am,84(5):1457–1472.
Hisada Y. 1995. An efficient method for computing Green’s functions for a layered half-space with sources and receivers at close depths (Part 2)[J]. Bull Seismol Soc Am,85(4):1080–1093.
Johnson L R. 1974. Green’s function for Lamb’s problem[J]. Geophys J Int,37(1):99–131. doi: 10.1111/j.1365-246X.1974.tb02446.x
Kennett B L N,Kerry N J. 1979. Seismic waves in a stratified half space[J]. Geophys J Int,57(3):557–583. doi: 10.1111/gji.1979.57.issue-3
Komatitsch D,Martin R,Tromp J,Taylor M A,Wingate B A. 2001. Wave propagation in 2-D elastic media using a spectral element method with triangles and quadrangles[J]. J Comput Acoust,9(2):703–718. doi: 10.1142/S0218396X01000796
Komatitsch D,Tromp J. 2003. A perfectly matched layer absorbing boundary condition for the second-order seismic wave equa-tion[J]. Geophys J Int,154(1):146–153. doi: 10.1046/j.1365-246X.2003.01950.x
Lamb H. 1904. On the propagation of tremors over the surface of an elastic solid[J]. Philos Trans Roy Soc A,203(359/371):1–42.
Lapwood E R. 1948. Convection of a fluid in a porous medium[J]. Math Proc Cambridge Philos Soc,44(4):508–521. doi: 10.1017/S030500410002452X
Levander A R. 1988. Fourth-order finite-difference P-SV seismograms[J]. Geophysics,53(11):1425–1436. doi: 10.1190/1.1442422
Liu T S,Feng X,Zhang H M. 2016. On Rayleigh wave in half-space:An asymptotic approach to study the Rayleigh function and its relation to the Rayleigh wave[J]. Geophys J Int,206(2):1179–1193. doi: 10.1093/gji/ggw189
Luco J E,Apsel R J. 1983. On the Green’s functions for a layered half-space. Part I[J]. Bull Seismol Soc Am,73(4):909–929.
Madariaga R. 1976. Dynamics of an expanding circular fault[J]. Bull Seismol Soc Am,66(3):639–666.
Pekeris C L. 1955. The seismic surface pulse[J]. Proc Natl Acad Sci USA,41(7):469–480. doi: 10.1073/pnas.41.7.469
Priolo E, Seriani G. 1991. A numerical investigation of Chebyshev spectral element method for acoustic wave propa-gation[C]//Proceedings of the 13th IMACS World Congress on Computational and Applied Mathematics. Dublin: Criterion Press: 551–556.
Tromp J,Komattisch D,Liu Q. 2008. Spectral-element and adjoint methods in seismology[J]. Commun Comput Phys,3(1):1–32.
Yao Z X,Harkrider D G. 1983. A generalized reflection-transmission coefficient matrix and discrete wavenumber method for syn-thetic seismograms[J]. Bull Seismol Soc Am,73(6A):1685–1699.
Zhang H M,Chen X F. 2001. Self-adaptive filon’s integration method and its application to computing synthetic seismograms[J]. Chinese Phys Lett,18(3):313–315. doi: 10.1088/0256-307X/18/3/301
Zhang H M,Chen X F,Chang S. 2003. An efficient numerical method for computing synthetic seismograms for a layered half-space with sources and receivers at close or same depths[J]. Pure Appl Geophys,160(3/4):467–486.
Zhang W,Chen X F. 2006. Traction image method for irregular free surface boundaries in finite difference seismic wave simul-ation[J]. Geophys J Int,167(1):337–353. doi: 10.1111/gji.2006.167.issue-1
计量
- 文章访问数: 1722
- HTML全文浏览量: 937
- PDF下载量: 52
