Spatio-temporal characteristics of b value in the northern margin of Ordos block and its earthquake prediction efficiency
-
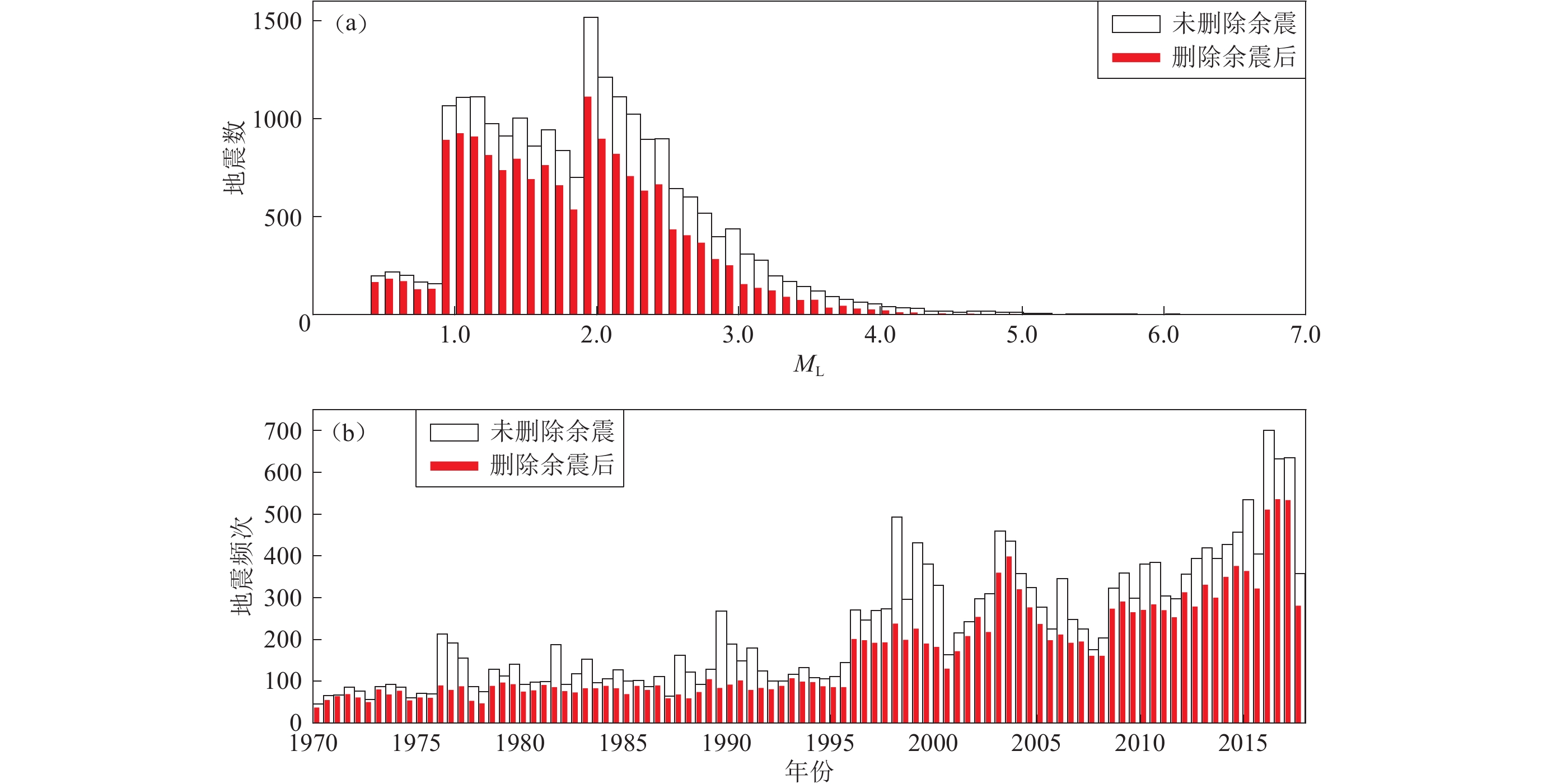
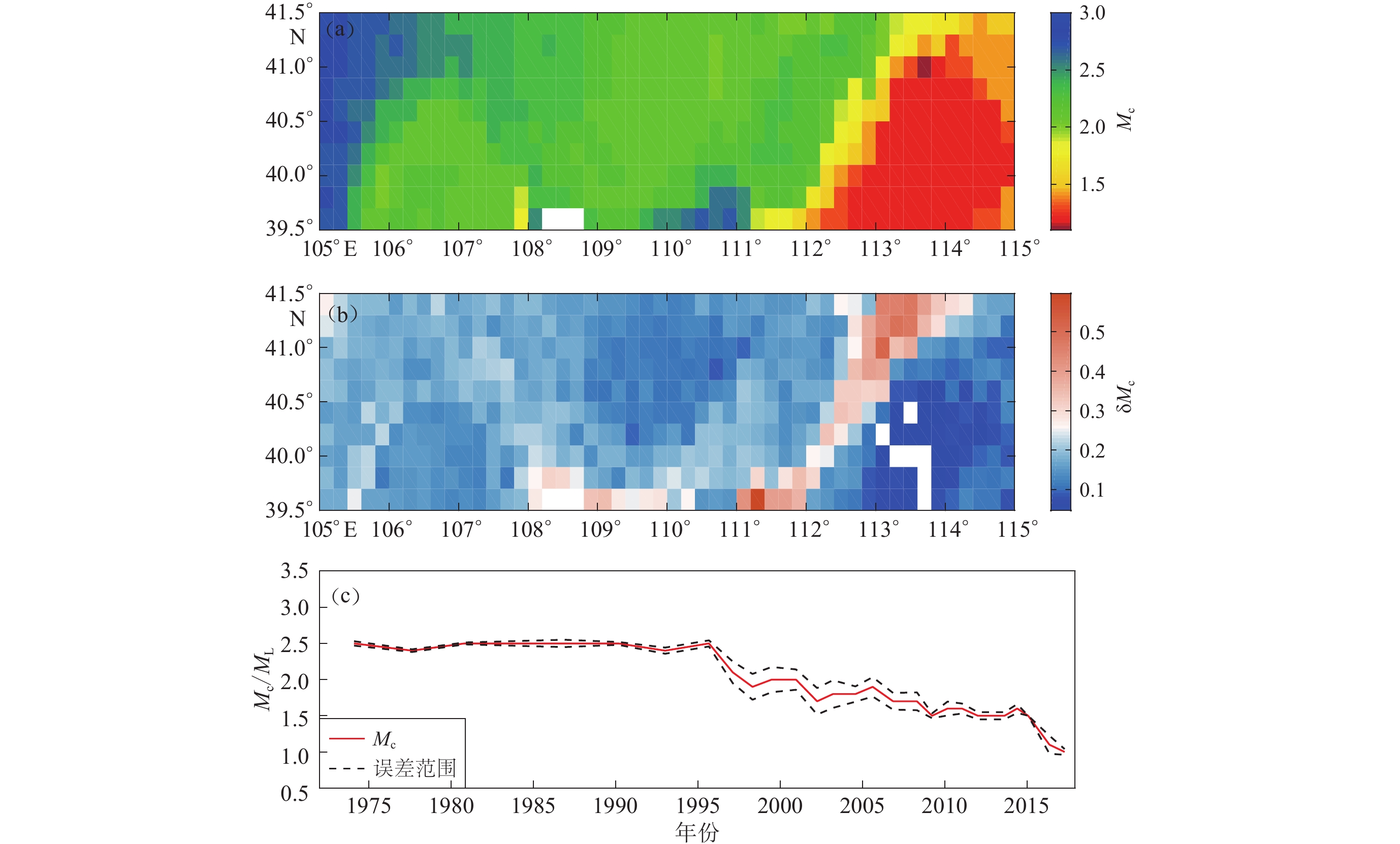
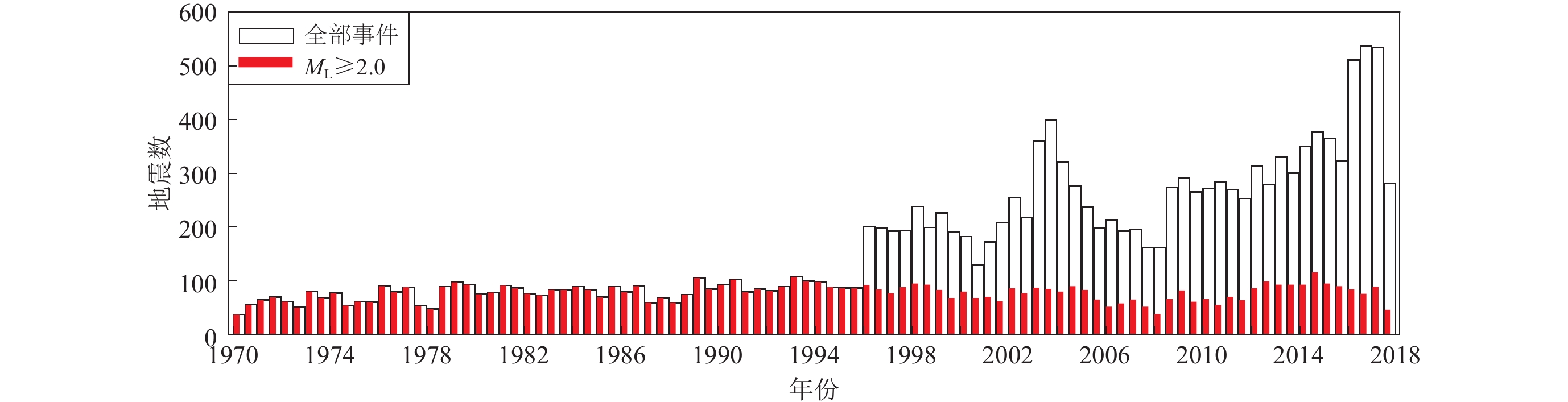
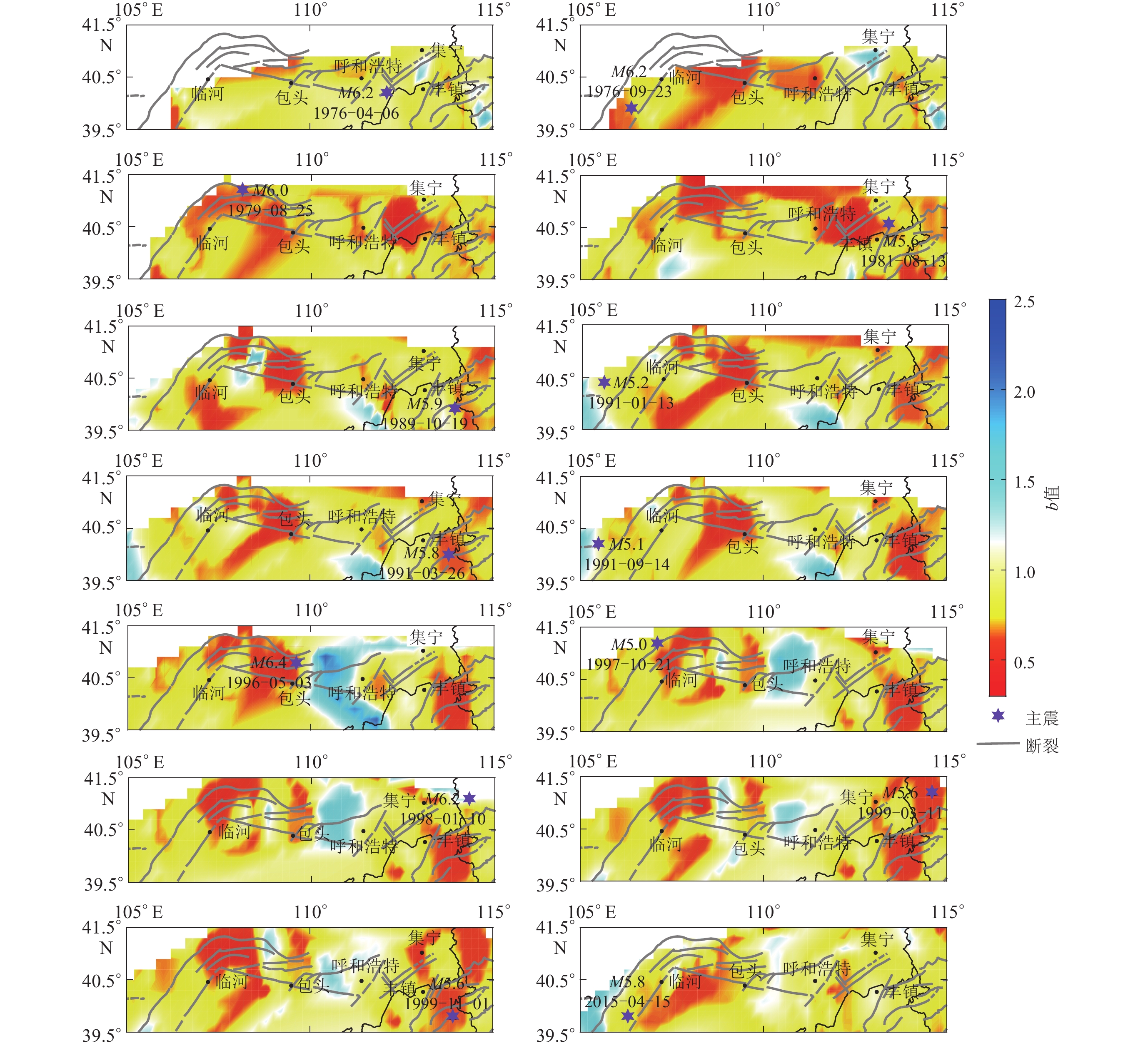
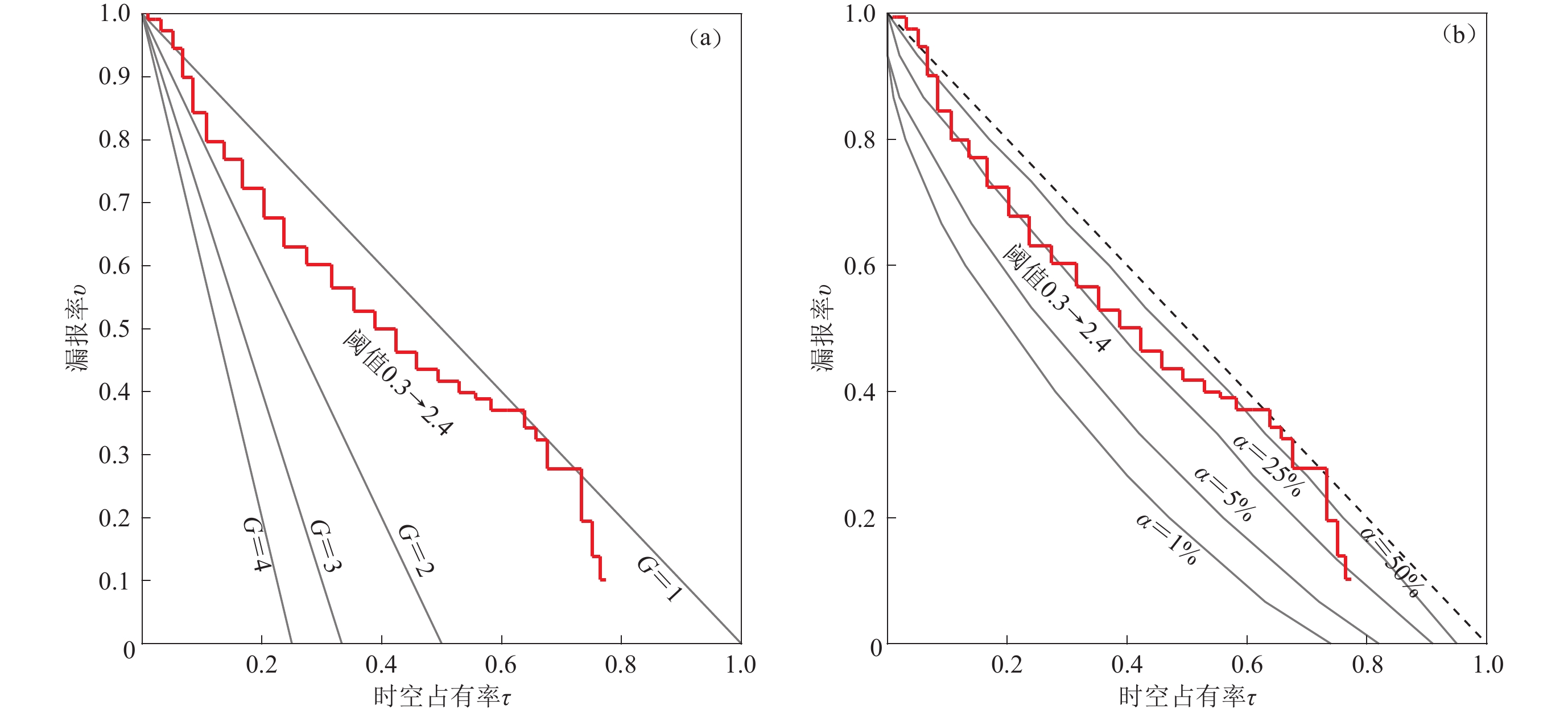
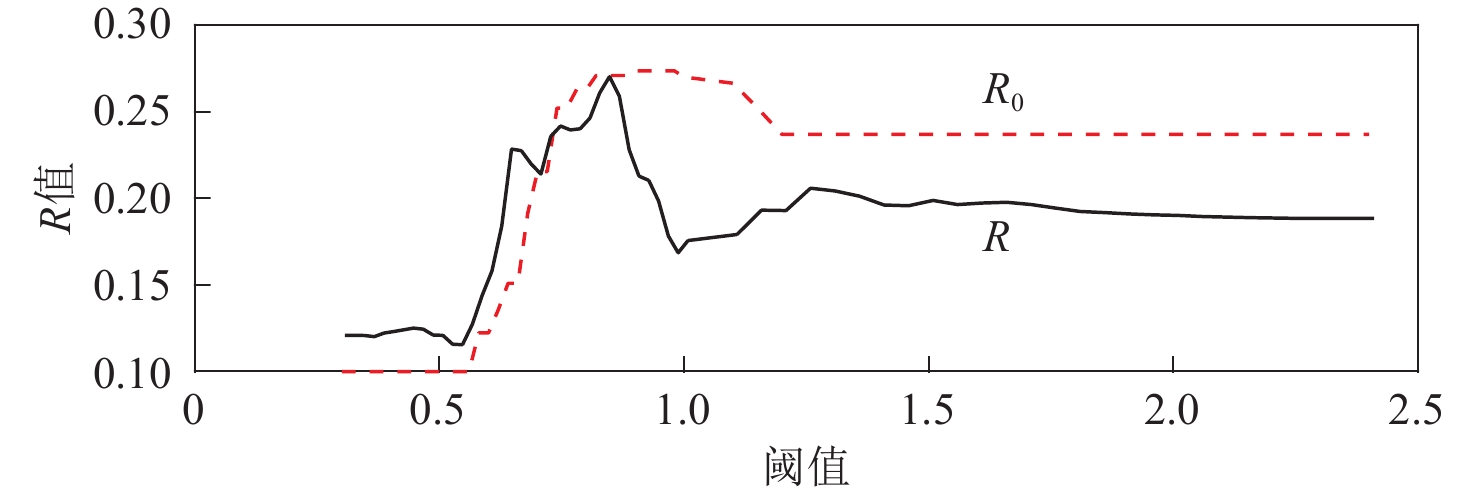
摘要: 使用中国地震台网1970—2017年地震目录，在评估目录完整性和删除余震的基础上，使用最大似然法对鄂尔多斯地块北缘进行b值时空扫描. 扫描结果显示，鄂尔多斯地块北缘的低b值区域主要分布于阿拉善和晋冀蒙地区，低b值区域的空间分布随时间呈现一定变化. 低b值区域与中强地震存在一定的对应关系，对14次震例的回溯性检验显示，其中10次地震发生在低b值区域及其边缘. 使用Molchan图表法和R值评分法对b值时空扫描结果进行预测效能检验，以低于阈值的区域为预测区域，计算不同阈值的漏报率、报准率和时空占有率。Molchan图表法检验结果显示，本文所使用的b值时空扫描方法的预测效果优于泊松分布，最优结果的概率增益达到2，显著性水平达到0.25. R值曲线显示，当阈值为0.8时，达到最高R值0.28，并达到97.5%置信水平.
-
关键词:
- b值 /
- 时空扫描 /
- 预测效能 /
- Molchan图表法 /
- 鄂尔多斯地块北缘
Abstract: Using earthquake catalogue of China Earthquake Networks Center from 1970 to 2017, the b values of the north edge of Ordos block were scanned by the maximum likelihood method after evaluating completeness and deleting aftershocks. The results show that the low b value region of the north margin of Ordos block is mainly distributed in the Alxa area and the Shanxi-Hebei-Inner Mongolia area, and the spatial distribution of low b value region changes with time to a certain extent. Meanwhile, there is a certain corresponding between the low b value region and the location of moderate-strong earthquakes. The retrospective test of 14 earthquake cases shows that ten earthquakes occurred in the low b value regions and their edges, and four earthquakes occurred beyond the low b value region. And then Molchan chart method and R value scoring method were used to test the prediction efficiency of b value spatio-temporal scanning. Taking the area below the threshold as the predicted area, the missing rate, the hit rate and the spatio-temporal occupation rate of different thresholds were calculated. The result of Molchan chart test shows that the prediction efficiency of spatio-temporal scanning is better than that of Poisson distribution, the probability gain of the optimal result reached 2, and the significance level reached 0.25. The R value curve shows that the highest R value is 0.28 at the threshold of 0.8 with 97.5% confidence level. -
引言
对于地震学而言，高频全球导航卫星系统（global navigation satellite system，简写为GNSS）是能够记录到地震波传播信息的一种新手段，其在强震中可直接提供时变位移场观测信息，弥补了传统观测手段在强震观测时出现诸如震级饱和、漂移等现象的不足。但由于高频GNSS数据存在信噪比低、信号稳定性差等问题，在预警中需要与测/强震观测手段联合使用。地震波信号往往是一种非线性、非平稳信号，故研究其频率的局部特性显得至关重要，这就需要用时频分析方法将一维时域信号转换到二维的时频平面。而傅里叶变换和反变换虽然能从时间域和频率域对平稳信号进行分析，却不能同时保留时间和频率信息。故此，本文拟以2008年汶川地震时获取的高频GNSS同震信号为例，通过对其进行广义S变换，系统分析变换后同震信号的时间域和频率域，以期能够更加准确地判断P波和S波到时。同时，广义S变换的使用也为高频GNSS在地震学中的应用提供了一条从多层面提取地震波形到时的新途径。
2. S变换
美国地球物理学家Stockwell等（1996）综合了小波变换和短时傅里叶变换的特点，提出了一种分析非平稳信号的新方法—S变换。其时频随着频率的不同而变化，具有多分辨率、逆变换唯一等特点，同时获得的二维时频谱也是基于傅里叶变换的基本理论。该方法已经在地震勘探、电能信号分析、故障检测等多个领域得到了广泛的应用（齐春燕等，2010；周竹生，陈友良，2011；郑成龙，王宝善，2015）。
S变换的表示形式为
$$ {{S}}(\tau {\text{，}}f) {\text{＝}} \int _{ {\text{－}} \infty }^{ {\text{＋}} \infty }h(t)\left\{ {\frac{{|f|}}{{\sqrt {2\pi } }}\exp \left[\frac{{ {\text{－}} {f^2}{{(t {\text{－}} \tau )}^2}}}{2}\right]\exp ( {\text{－}} {\rm i}2\pi ft)} \right\}{\rm d}t{\text{，}} $$ (1) 式中：τ为窗函数的中心点，控制窗函数在时间轴上的位置；f为采样频率；h（t）为原始信号。S变换使用的高斯窗函数gf （t）和基本小波ωf （t）分别定义为
$$ {{g}_f}(t) {\text{＝}} \frac{{|f|}}{{\sqrt {2\pi } }}\exp \left({\text{－}} \frac{{{t^2}{f^2}}}{2}\right){\text{，}} $$ (2) $$ {\omega _f}(t) {\text{＝}} \frac{{|f|}}{{\sqrt {2\pi } }}\exp \left( {\text{－}} \frac{{{t^2}{f^2}}}{2} {\text{－}} {\rm i}2\pi ft\right) {\text{＝}} {g_f}(t)\exp ( {\text{－}} {\rm i}2\pi ft){\text{，}} $$ (3) 从上式可以看到，S变换在分析高频信号或低频信号时具有良好的特性：对于高频信号，设计窗口比较窄，可满足高时间分辨率的要求；对于低频信号，设计窗口比较宽，可满足高频率分辨率的要求。
相比于单分辨率的短时傅里叶变换，S变换属于多分辨率时频分析方法，可以更加有效地对复杂的地震波信号进行分析。同时由于信号x（t）可以由S变换S（τ，f ）通过逆变换进行重构，S（τ，f ）的S逆变换为
$$ {\rm{x}}(t) {\text{＝}} \int_{ {\text{－}} \infty }^{ {\text{＋}} \infty } {\left[ {\int_{ {\text{－}} \infty }^{ {\text{＋}} \infty } {S(\tau{\text{，}} f\;)\rm d\tau } } \right]} \exp ({\rm i}2\pi ft){\rm {d}}f{\text{，}} $$ (4) S变换所具有的无损逆变换的特性，使得信号在经过时频域处理后可逆变换为时间信号。
由于S变换的基本高斯窗函数固定，因此在处理复杂的地震信号时，不能根据需求对频率域和时间域的分辨率进行调节，缺乏必要的灵活性。随着后期的不断发展，多位研究人员对S变换中的高斯窗函数和基本小波进行改变，构建出了广义的S变换（Mansinha等，1997；McFadden et al，1999；高静怀等，2003；Pinnegar，Mansinha ，2003；陈学华等，2006；齐春燕等，2010；周竹生，陈友良，2011）。
本文使用的广义S变换是通过两个调节因子对高斯窗进行改造（陈学华等，2006），以便根据实际要求对高斯窗作出调整，从而更好地分析复杂频率的地震波形，其表达式为
$$ {{S}}(\tau {\text{，}} f) {\text{＝}} \int\nolimits_{ {\text{－}} \infty }^\infty {x(t)\frac{{|{\lambda _{\rm a}}||f{|^p}}}{{2\pi }}} {{\rm exp}({\frac{{ {\text{－}} {\lambda ^2_{\rm{a}}}{f^{2p}}{{(t {\text{－}} \tau )}^2}}}{2}}}){{\rm exp}({ {\text{－}} {\rm i}2\pi ft}}){\rm d}t{\text{，}} $$ (5) 式中λa和p均为调节因子。选定p值后，当λa＞1时，时窗宽度随频率呈反比变化的速度加快；当λa＜1时，时窗宽度随频率呈反比变化的速度减慢；选定λa之后，通过调节p值可以对频率域的分辨率进行调整。引入λa和p两个参数之后，可以根据实际信号的频率分布特点和时频分析的侧重点，改变高斯窗函数随频率变化的规律，对时频分辨率进行调整，但由于其运算过程与标准的S变换相似，因此并不需要额外增加运算量。当两个调节因子λa和p均设置为1时，则为标准S变换。
3. 到时分析中的S变换
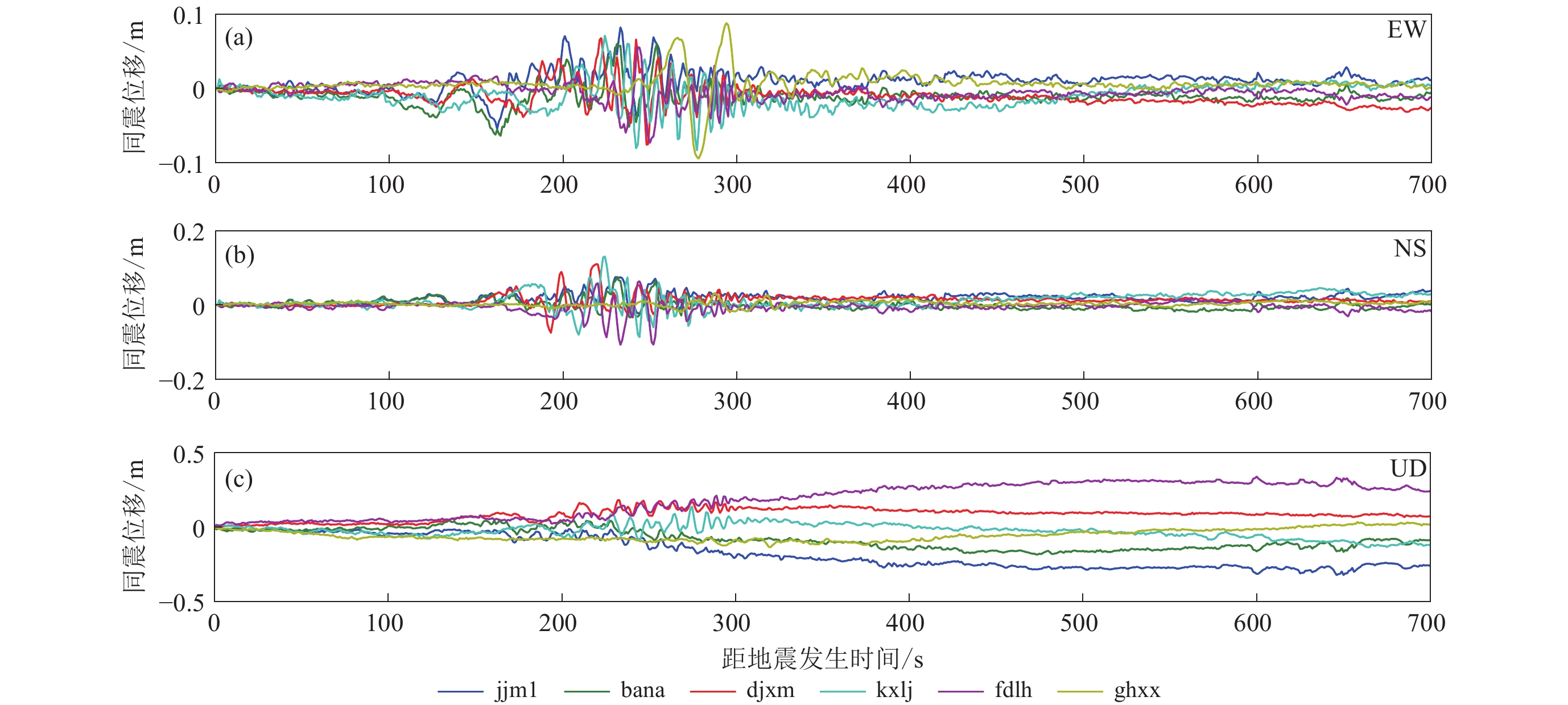
本文收集了2008年汶川MS8.0地震中重庆台网和昆明台网共28个站点的高频GNSS信号（采样率为1 Hz），采用GAMIT/GLOBK软件包（Bock，2000）中的高频GNSS处理模块TRACK （Chen，1998）对附近站点的高频GNSS数据进行解算。由于所选取数据按小时文件进行解算，时间最长为1小时，故未考虑昼夜变化等周期性较长的噪音。按照GNSS站点的位置及分布情况，选取15个站点的数据使用广义S变换进行分析，并挑选其中6个站点的同震波形进行展示，如图1所示。
根据距离震中位置的远近选取了bana （距震中约346 km）、djxm （距震中381 km）、fdlh （距震中477 km）和ghxx （距震中661 km）等4个站点的同震波形，对其进行广义S变换后识别出P波、S波到时并进行展示（图1），在计算P波和S波的理论到时中使用了频率-波数法（Zhu，2002），计算到时的速度模型为一维速度模型（吴建平等，2009；殷海涛等，2013；邓文哲等，2014；王小娜等，2015；杨彦明等，2016）。
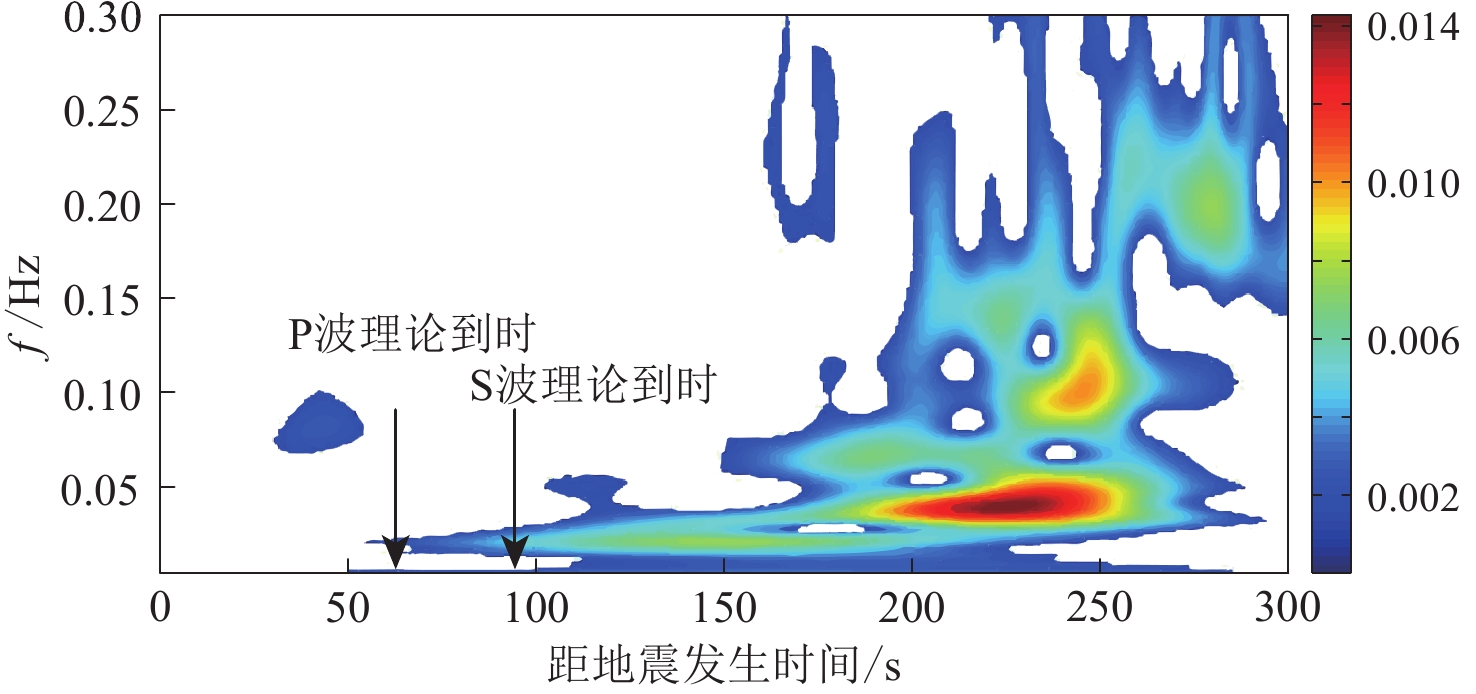
bana站点距离震中346 km，该站点记录高频GNSS信号的频率为1 Hz，根据奈奎斯特（Nyquist）采样定理，其可记录到的地震波信号的最大频率为0.5 Hz。bana站点东西向同震信号广义S变换的结果表明（图2），地震信号的能量主要集中于0—0.3 Hz，所研究各站点的P波和S波理论到时均在300 s以内。为了更加直观地显示所研究的到时区域，在后续分析中，按照S变换后信号频率为0—0.3 Hz，取地震发生后的300 s进行分析。结果显示，bana站点的P波理论到时为56.7 s，S波理论到时为96.1 s。如图1所示，经过广义S变换后的图像显示，在P波和S波理论到时（图2）的位置，均有着较明显的能量汇集。在本文分析的地震中，P波和S波周期均较长、频率均相近，故P波的识别周期为20—50 s左右的初至拾取、高于背景噪声的能量汇集，S波能量为P波能量的2.5倍以上（水平向）。
![]()
图 2 bana站点东西向同震信号的S变换结果Figure 2. S transform of coseismic signal in the EW directionin at the bana station在使用广义S变换进行频率分析时，通过对两个调节因子λa和p进行调整，以获得最优的分辨率。以bana站东西向为例进行分析，结果如图3所示。
![]()
图 3 通过微调调节因子λa和p所产生的同震信号广义S变换结果展示Figure 3. S transform results of the same coseismic signal with different adjustment factor λa and p（a） λa＝1，p＝1.1；（b） λa＝1，p＝1.2；（c） λa＝1.05，p＝1.05；（d） λa＝1.05，p＝1.05在λa不变的情况下，提高因子p值，可以增加时间域的分辨率，详见图2和图3a。但是p值过高，会导致频率域的分辨率增加，时间域的分辨率降低，从而降低了对初至波的识别能力（图3b）。当调节因子λa＝1.05，p＝1.05时，对于P波和S波的识别均较为清晰。图3d将图3c进行三维展示，更加直观地展示了震后随着时间的变化，不同周期的波形所汇聚的能量大小。
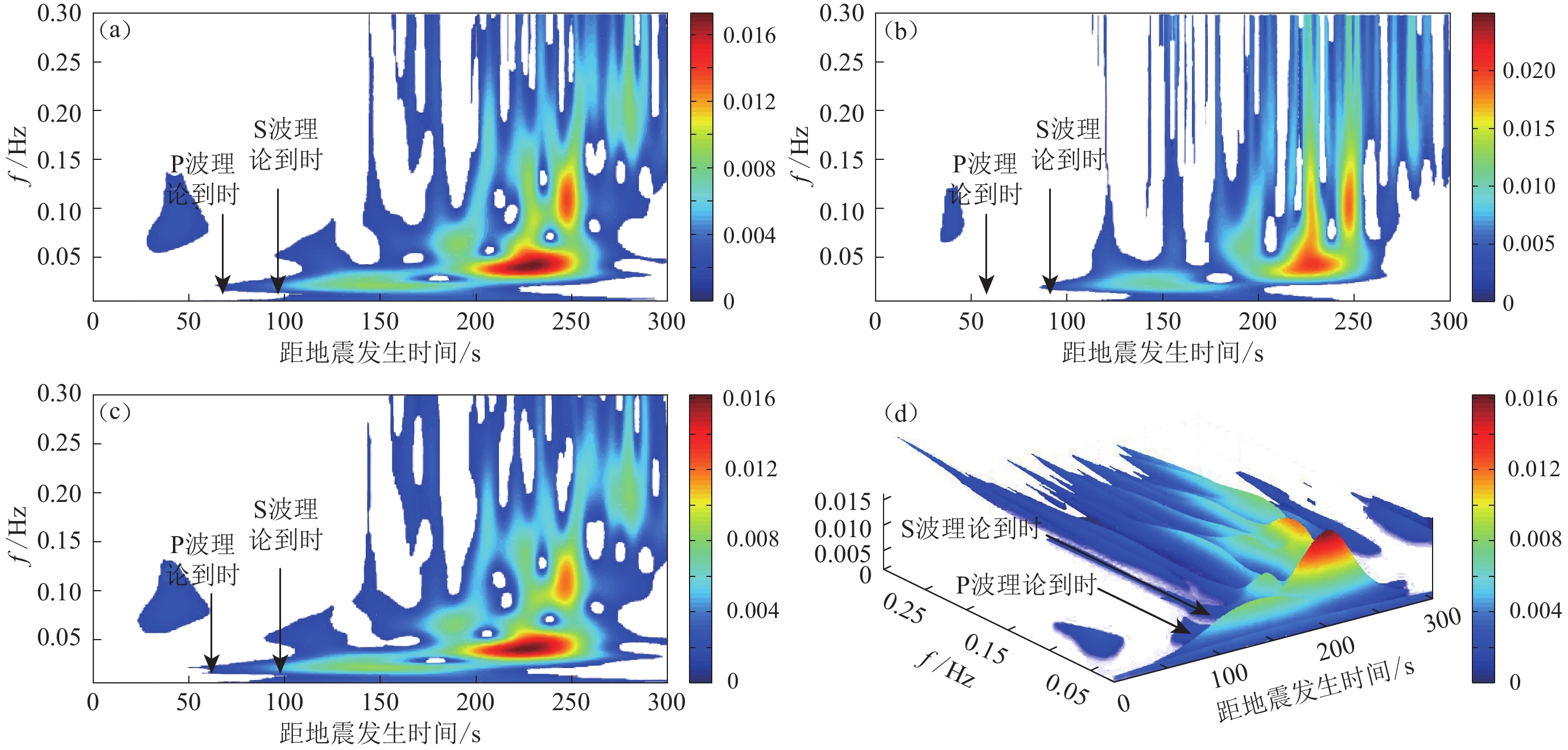
对于震中距为381 km的djxm站点，其P波理论到时为62.5 s，S波理论到时为105.9 s。将P波和S波理论到时分别标注于S变换结果上（选用的调节因子为λa＝1.05，p＝1.05），可以看出，在同震信号中，djxm站记录的到时要比理论到时延迟约4—5 s （图4a），可能与本文使用的速度结构有一定关系，而djxm站在垂向上很难识别S波到时（图4b）。在原始波形记录中，无论垂直向还是水平向均已很难判定P波和S波到时。这表明通过微调广义S变换的两个调节因子λa和p，能够得到较好的到时分辨率。鉴于目前GNSS预警中的触发方式为阈值触发（Allen，Ziv，2011；Melgar et al，2012），很难通过反演的方式进行精确的震源定位。而相比于人工或者阈值判断，通过对地震信号进行广义S变换，能够对到时作出更为精准的判断。
![]()
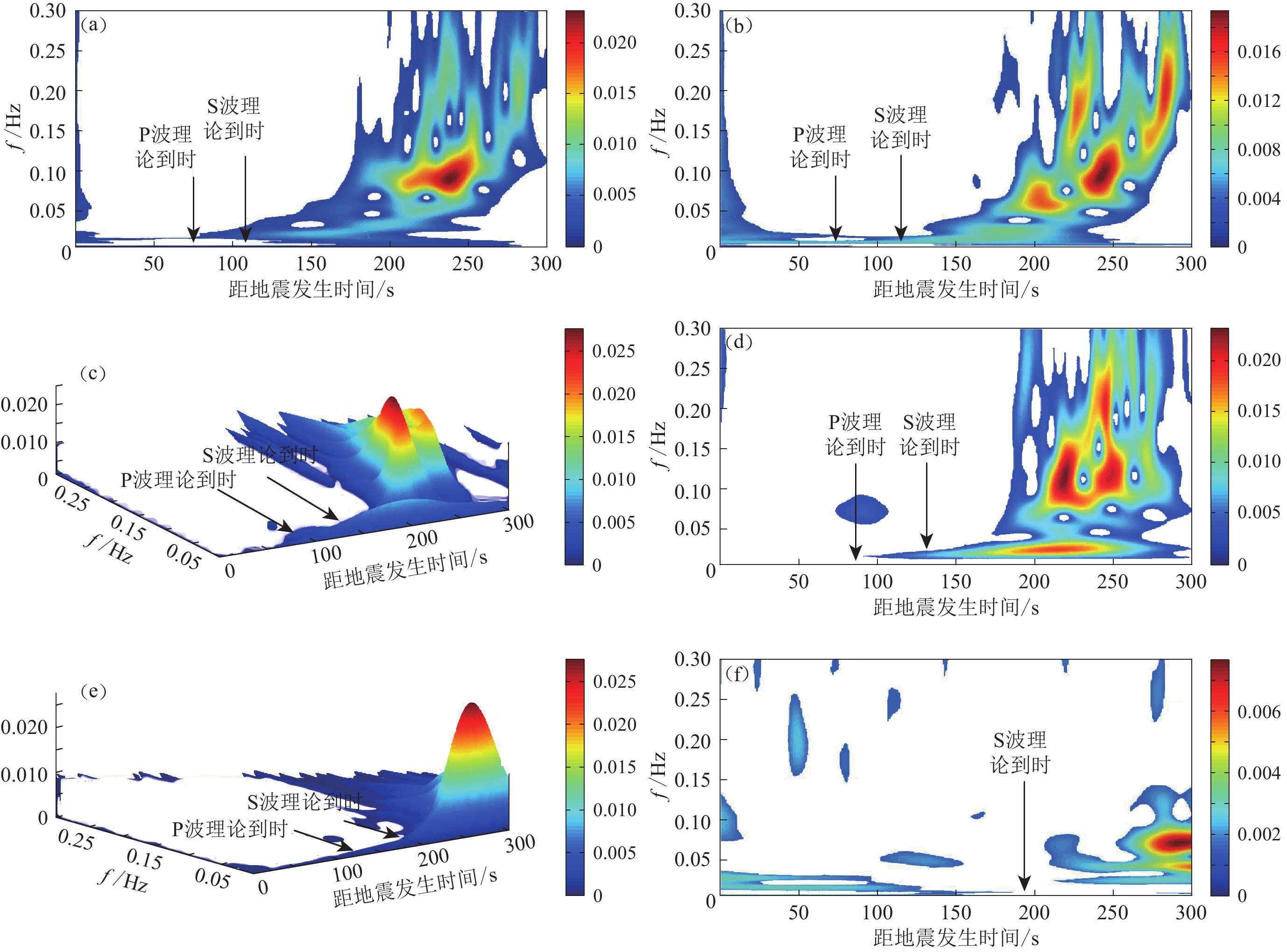
图 4 各高频GNSS站点同震信号S变换结果展示（a） djxm站点EW向同震信号S变换；（b） djxm站点UD向同震信号S变换；（c） fdlh站点EW向同震信号S变换；（d） fdlh站点UD向同震信号S变换；（e） ghxx站点EW向同震信号S变换；（f） ghxx站点UD向同震信号S变换Figure 4. S treansform of coseismic signals for several stations（a） S transform of EW coseismic signal in djxm；（b） S transform of UD coseismic signal in djxm；（c） S transform of the EW coseismic signal in fdlh ；（d） S transform of the UD coseismic signal in fdlh；（e） S transform of the EW coseismic signal in ghxx；（f） S transform of the UD coseismic signal in ghxx对于震中距为477 km的fdlh站，P波和S波理论到时分别为79 s和133 s。东西向同震信号的广义S变换（选用的调节因子为λa＝1.05，p＝1.05）波形识别基本与理论到时一致，相差2—3 s （图4c）。fdlh站垂直向上的分辨率也可以满足波形识别的需求（图4d）。其结果较好，可能与fdlh站的背景噪声较低有关。三维图像中，面波的识别更加清晰（图4c）。
对于震中距为661 km的ghxx站，在同震信号中很难看到P波到时和S波到时。经过计算，其P波理论到时为108.3 s，S波理论到时为183 s。在图4e中可以看到，通过广义S变换后对S波的识别较好，对P波的识别稍显模糊。而对于垂直向（图4f），通过广义S变换之后，P波已经无法识别，S波的识别时间较理论地震波形要晚将近20 s。这可能与GNSS背景噪声较高，且垂直向的噪音水平高于水平向2—3倍有关。而同震信号的分析中，P波和S波的到时基本均无法分辨。这说明通过广义S变换，即使是震中距大于600 km，也能有效地提取到地震波到时。
4. 讨论与结论
对于实时解算的高频GNSS数据，其背景噪声相对较大，使用广义S变换，可以更加准确地得到P波到时。即使远距离的站点记录，在时频域中也可以很好地指示出P波初至到时。这为高频GNSS数据在地震学中的应用和分析提供了一种新的途径。其优势在于：
1） 广义S变换集合了短时傅里叶变换和小波变换的优点，在地球物理领域及电能分析领域取得了很多成果，但依旧存在很多应用前景。本文通过对高频GNSS同震信号进行S变换，从以往的只针对单独的时间域或频率域分析高频GNSS信号，转变为从时间域和频率域同时进行分析。从频率域的角度看，P波和S波的能量均汇聚于0.025 Hz左右，面波的能量汇聚于0.04—0.25 Hz并能够明显地看出面波是分为多个频率区间；从时间域的角度看，P波和S波的理论到时均有明显的能量汇集，而面波到时则更加明显。通过对不同频率的信号进行分析，认为近震范围内P波和S波实际到时与理论到时的分辨率相差仅为1—2 s，中远震其分辨率相差3—5 s，该方法可以作为高频GNSS信号的分析及各类波形到时的提取手段。
2） 通过对高频GNSS信号使用S变换可见，有效信号与噪音信号的分离较为明显，说明S变换在降噪去噪方面具有独特的优势。鉴于信号可以进行无损S变换和S逆变换，因此可以使用该方法提高高频GNSS信号的信噪比，尤其是在压制噪音方面具有很大的优势，下一步研究中将根据各波形的到时按时间提取波形并用于专门性的分析。
感谢两位审稿专家对本文提出的意见和建议。
-
![]()
图 1 鄂尔多斯地块北缘的构造背景和地震分布
Figure 1. Tectonic settings and earthquake distribution in the northern margin of Ordos block
![]()
图 2 余震删除前后的地震频次时序统计（a）和震级频次统计（b）
Figure 2. Seismic frequency time series statistics （a） and magnitude frequency statistics （b） before and after aftershock deletion
![]()
图 3 鄂尔多斯地块北缘的最小完整性震级计算结果
（a） Mc空间分布；（b） δMc空间分布；（c） Mc时序分布
Figure 3. Minimum completeness magnitude of the northern margin of Ordos block
（a） Mc spatial distribution；（b） δMc spatial distribution；（c） Mc time-series distribution
![]()
图 4 参与计算的地震目录（ML≥2.0）与全部地震目录的频次对比
Figure 4. Comparison of frequencies of earthquakes used in calculation （ML≥2.0） with those of all earthquakes
![]()
图 5 鄂尔多斯地块北缘b值 （a）和δb （b）的时空扫描结果
Figure 5. Spatio-temporal scanning results of b value （a） and δb （b） in the northern margin of Ordos block
![]()
图 6 鄂尔多斯地块北缘14次震例的b值回溯检验
Figure 6. Retrospective test for b values of the fourteen earthquake examples in the northern margin of Ordos block
![]()
图 7 Molchan检验
（a） 不同概率增益G下的τ - υ曲线；（b） 不同显著性水平α下的τ - υ曲线；
Figure 7. Molchan test
（a） τ - υ curve with different probabilistic gain G；（b） τ - υ curve with different significant level α
表 1 鄂尔多斯地块北缘14次震例的回溯情况
Table 1 Retrospective test for the fourteen earthquake examples in the northern margin of Ordos block
编号 年-月-日 北纬/° 东经/° MS 地点 低b值 1 1976-04-06 40.20 112.10 6.2 和林格尔 × 2 1976-09-23 39.90 106.40 6.2 巴音木仁 √ 3 1979-08-25 41.20 108.10 6.0 五原 √ 4 1981-08-13 40.58 113.41 5.6 丰镇 √ 5 1989-10-19 39.92 113.91 5.9 山西大同 √ 6 1991-01-13 40.40 105.60 5.2 阿拉善左旗 × 7 1991-03-26 40.00 113.80 5.8 山西大同 √ 8 1991-09-14 40.20 105.50 5.1 阿拉善左旗 × 9 1996-05-03 40.80 109.60 6.4 包头 √ 10 1997-10-21 41.20 107.10 5.0 乌拉特后旗 √ 11 1998-01-10 41.10 114.30 6.2 河北张北 × 12 1999-03-11 41.20 114.60 5.6 河北张北 √ 13 1999-11-01 39.80 113.90 5.6 山西浑源 √ 14 2015-04-15 39.80 106.30 5.8 阿拉善左旗 √ 
下载: 导出CSV
-
曹刚. 2001. 内蒙古地震研究[M]. 北京: 地震出版社: 5–6. Cao G. 2001. Seismological Research of Inner Mongolia[M]. Beijing: Seismological Press: 5–6 （in Chinese）.
邓起东, 程绍平, 闵伟, 杨桂枝, 任殿卫. 1999. 鄂尔多斯块体新生代构造活动和动力学的讨论[J]. 地质力学学报, 5（3）: 13-21. Deng Q D, Cheng S P, Min W, Yang G Z, Ren D W.1999.Discussion on Cenozoic tectonics and dynamics of ordos block[J]. Journal of Geomechanics, 5（3）: 13-21（in Chinese）
韩晓明, 张文韬, 王树波, 赵星, 包金哲, 李娟. 2016. 河套地震带的b值时空变化特征分析[J]. 中国地震, 32（3）: 522-532. Han X M, Zhang W T, Wang S B, Zhao X, Bao J Z, Li J. 2016. Analysis of spatial and temporal variation characteristics of b-value in the Hetao seismic belt[J]. Earthquake Research in China, 32（3）: 522-532 （in Chinese）.
蒋长胜, 张浪平, 韩立波, 来贵娟. 2011. 中长期地震危险性概率预测中的统计检验方法Ⅰ: Molchan图表法[J]. 地震, 31（2）: 106-113. Jiang C S, Zhang L P, Han L B, Lai G J. 2011. Probabilistic forecasting method of long-term and intermediate-term seismic hazard I: Molchan error diagram[J]. Earthquake, 31（2）: 106-113 （in Chinese）.
李全林, 陈锦标, 于渌, 郝柏林. 1978. b值时空扫描: 监视破坏性地震孕育过程的一种手段[J]. 地球物理学报, 21（2）: 101-125. Li Q L, Chen J B, Yu L, Hao B L. 1978. Time and space scanning of the b-value: A method for monitoring the development of catastrophic earthquakes[J]. Chinese Journal of GeophysicsSinica, 21（2）: 101-125 （in Chinese）.
李正芳, 周本刚. 2014. 利用断裂带上的低b值识别凹凸体方法的探讨:以龙门山断裂带和鲜水河断裂带为例[J]. 2014. 震灾防御技术, 9（2）: 213-225. Li Z F, Zhou B G. 2014. Asperity identification based on low b-value: Application to the Longmenshan and Xianshuihe fault zone[J]. Technology for Earthquake Disaster Prevention, 9（2）: 213-225 （in Chinese）.
马鸿庆. 1978. 华北地区几次大震前的b值异常变化[J]. 地球物理学报, 21（2）: 126-141. Ma H Q. 1978. Variations of the b-values before several large earthquakes occurred in north china[J]. Chinese Journal of Geophysics, 21（2）: 126-141 （in Chinese）.
梅世蓉. 1993. 中国地震预报概论[M]. 北京: 地震出版社: 65–69. Mei S R. 1993. Introduction to Earthquake Prediction in China[M]. Beijing: Seismological Press: 65–69 （in Chinese）.
孙加林. 1985. 鄂尔多斯块体北部边缘发生强震的危险性[J]. 西北地震学报, 7（增刊1）: 13-23. Sun J L. 1985. Strong earthquake assessment in the northern edge of Ordos[J]. Northwestern Seismological Journal, 7（S1）: 13-23 （in Chinese）.
孙丽娜, 齐玉妍, 温超, 张合. 2012. 中长期地震预测中的PI算法改进研究及应用[J]. 地震, 32（4）: 44-52. Sun L N, Qin Y Y, Chao Wen CW, Zhang H. 2012. Application of improved PI algorithm in medium to long-term earthquake prediction[J]. Earthquake, 32（4）: 44-52 （in Chinese）.
王熠熙, 张辉, 刘双庆, 曹井泉, 李赫, 陈嵩, 高也. 2015. 河北平原地震带b值时空变化特征[J]. 地震工程学报, 37（1）: 188-195. Wang Y X, Zhang H, Liu S Q, Cao J Q, Li H, Chen S, Gao Y. 2015. Spatial and temporal variations of b-value in the Hebei plain seismic belt[J]. China Earthquake Engineering Journal, 37（1）: 188-195 （in Chinese）.
许绍燮. 1989. 地震预报能力评分[G]//地震预报方法实用化研究文集: 地震学专辑. 北京: 学术书刊出版社: 586–590. Xu S X. 1989. Earthquake prediction ability score[G]//Earthquake Prediction Method Practical Research Anthology: Seismology Album. Beijing: Academic Book Press: 586–590 （in Chinese）.
徐锡伟, 程国良, 马杏垣, 孙宇航, 韩竹君. 1994. 华北及其邻区块体转动模式和动力来源[J]. 地球科学: 中国地质大学学报, 19（2）: 129-138. Xu X W, Cheng G L, Ma X Y, Sun Y H, Han Z J. 1994. Rotation model and dynamics of blocks in North China and its adjacent areas[J]. Earth Science: Journal of China University of Geosciences, 19（2）: 129-138 （in Chinese）.
薛艳, 陈琳荣, 黎明晓, 刘双庆. 2017. 华北地区震群活动与强震[J]. 华北地震科学, 35（2）: 1-9. Xue Y, Chen L R, Li M X, Liu S Q. 2017. Relationship between earthquake swarms and the strong earthquake in North China[J]. North China Earthquake Sciences, 35（2）: 1-9 （in Chinese）.
易桂喜, 闻学泽, 张致伟, 龙锋, 阮祥, 杜方. 2010. 川南马边地区强震危险性分析[J]. 地震地质, 32（2）: 282-293. Yi G X, Wen X Z, Zhang Z W, Long F, Ruan X, Du F. 2010. Study on potential strong earthquake risks inAround the Mabian area, Southern Sichuan[J]. Seismology and Geology, 32（2）: 282-293 （in Chinese）.
易桂喜, 闻学泽, 辛华, 乔慧珍, 龙锋, 王思维. 2011. 2008年汶川MS8.0地震前龙门山—岷山构造带的地震活动性参数与地震视应力分布[J]. 地球物理学报, 54（6）: 1490-1500. Yi G X, Wen X Z, Xin H, Qiao XH Z, Long F, Wang S W. 2011. Distributions of seismicity parameters and seismic apparent stresses on the Longmenshan-Minshan tectonic zone before the 2008 M（S）8.0 Wenchuan earthquake[J]. Chinese Journal of Geophysics, 54（6）: 1490-1500 （in Chinese）.
张琳琳, 唐兰兰. 2015. 新疆天山地区b值时空变化特征[J]. 地震, 35（3）: 93-101. Zhang L L, Tang L L. 2015. Temporal and spatial variations of b-value in Tianshan, Xinjiang[J]. Earthquake, 35（3）: 93-101 （in Chinese）.
郑兆苾, 刘东旺, 沈小七,汪雪泉. 2001. 华北b值全时空扫描结果的可靠性及与地震的相关性[J]. 地震, 21(3):8-14. Zheng Z B, Liu D W, Shen X Q, Wang X Q. 2001. Reliability of time-space scanning results of b value and correlativity between b vales and earthquakes with M≥5.0 in North China[J]. Earthquake, 21(3):8-14 （in Chinese）.
Aki K. 1984. Asperities, barriers, characteristic earthquakes and strong motion prediction[J]. J Geophys Res, 89（B7）: 5867-5872. doi: 10.1029/JB089iB07p05867
Amitrano D. 2003. Brittle-ductile transition and associated seismicity: Experimental and numerical studies and relationship with the b value[J]. J Geophys Res, 108（B1）: 2044.
Bak P, Tang C. 1989. Earthquakes as a self-organized critical phenomenon[J]. J Geophys Res, 94（B11）: 15635-15637. doi: 10.1029/JB094iB11p15635
Chen S, Jiang C S, Zhuang J C. 2016. Statistical evaluation of efficiency and possibility of earthquake predictions with gravity field variation and its analytic signal in western China[J]. Pure Appl Geophys, 173（1）: 305-319. doi: 10.1007/s00024-015-1114-x
Gutenberg B, Richter C F. 1954. Seismicity of the Earth and Associated Phenomena[M]. 2nd ed. Princeton: Princeton University Press: 1–310.
Ito K, Matsuzaki M. 1990. Earthquakes as self-organized critical phenomena[J]. J Geophys Res, 95（B5）: 6853-6860. doi: 10.1029/JB095iB05p06853
Kamer Y, Hiemer S. 2015. Data-driven spatial b value estimation with applications to California seismicity: to b or not to b[J]. J Geophys Res, 120（7）: 5191-5214. doi: 10.1002/2014JB011510
Molchan G M. 1997. Earthquake prediction as a decision-making problem[J]. Pure Appl Geophys, 149（1）: 233-247. doi: 10.1007/BF00945169
Mori J, Abercrombie R E. 1997. Depth dependence of earthquake frequency-magnitude distributions in California: implications for rupture initiation[J]. J Geophys Res, 102（B7）: 15081–15090. doi: 10.1029/97JB01356
Reasenberg P. 1985. Second‐order moment of central California seismicity, 1969–1982[J]. J Geophys Res, 90（B7）: 5479-5495. doi: 10.1029/JB090iB07p05479
Scholz C H. 1968. The frequency-magnitude relation of microfracturing in rock and its relation to earthquakes[J]. Bull Seismol Soc Am, 58（1）: 399-415.
Schorlemmer D, Wiemer S, Wyss M. 2005. Variations in earthquake-size distribution across different stress regimes[J]. Nature, 437（7058）: 539-542. doi: 10.1038/nature04094
Urbancic T I, Trifu C I, Long J M, Young R P. 1992. Space-time correlations of b values with stress release[J]. Pure Appl Geophys, 139（3/4）: 449-462.
Utsu T. 1978. Estimation of parameters in formulas for frequency-magnitude relation of earthquake occurrence: in cases involving a parameter c for the maximum magnitude[J]. Zisin, 31（4）: 367-382. doi: 10.4294/zisin1948.31.4_367
Wiemer S, Wyss M. 1997. Mapping the frequency‐magnitude distribution in asperities: An improved technique to calculate recurrence times[J]. J Geophys Res, 102（B7）: 15115-15128. doi: 10.1029/97JB00726
Wiemer S, Schorlemmer D. 2007. ALM: An asperity-based likelihood model for California[J]. Seismol Res Lett, 78（1）: 134-140. doi: 10.1785/gssrl.78.1.134
Woessner J, Wiemer S. 2005. Assessing the quality of earthquake catalogues: estimating the magnitude of completeness and its uncertainty[J]. Bull Seismol Soc Am, 95（2）: 684-698. doi: 10.1785/0120040007
Wyss M. 1973. Towards a physical understanding of the earthquake frequency distribution[J]. Geophys J Int, 31（4）: 341-359. doi: 10.1111/j.1365-246X.1973.tb06506.x
Wyss M, McNutt S R. 1998. Temporal and three-dimensional spatial analyses of the frequency-magnitude distribution near Long Valley Caldera, California[J]. Geophys J Int, 134（2）: 409-421. doi: 10.1046/j.1365-246x.1998.00561.x
Wyss M, Wiemer S. 2000. Change in the probability for earthquakes in southern California due to the Landers magnitude 7.3 earthquake[J]. Science, 290（5495）: 1334-1338. doi: 10.1126/science.290.5495.1334
Wyss M. 2004. Fractal dimension and b-value on creeping and locked patches of the san Andreas fault near Parkfield, California[J]. Bull Seismol Soc Am, 94（2）: 410-421. doi: 10.1785/0120030054
Zechar J D, Jordan T H. 2008. Testing alarm-based earthquake predictions[J]. Geophys J Int, 172（2）: 715-724. doi: 10.1111/gji.2008.172.issue-2
-
期刊类型引用(5)
1. 周依，曹筠，马旭东，单连君. 唐山老震区现今地震活动特征. 地震研究. 2024(03): 430-440 . 
百度学术
2. Jinxin Hou，Yunpeng Zhang，Liwei Wang，Zhirong Zhao. Earthquake relocation using a 3D velocity model and implications on seismogenic faults in the Beijing-Tianjin-Hebei region. Earthquake Research Advances. 2024(02): 55-64 . 必应学术
3. 周依，马旭东，王想. 唐山地区地震视应力时空变化特征. 大地测量与地球动力学. 2024(10): 1059-1063 . 百度学术
4. 曹筠，周依，高晨，刘书峰，陈安，张素欣，冯向东，吴鹏，陈肇东. 2020年7月12日河北唐山5.1级地震发震断层判定及其区域构造意义. 地震地质. 2024(05): 993-1011 . 百度学术
5. 陈婷，余海琳，章阳，孙丽娜. 唐山地区三维b值分布特征研究. 华北地震科学. 2022(01): 34-40 . 百度学术
其他类型引用(1)
计量
- 文章访问数: 1341
- HTML全文浏览量: 833
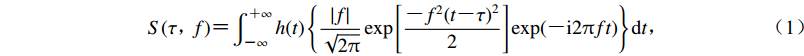
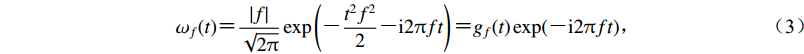
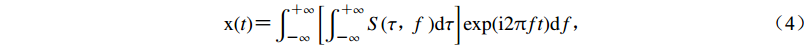
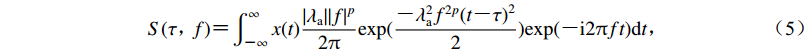
- PDF下载量: 115
- 被引次数: 6
