Spatial domain division based on superposition pattern of seismic waves and spatial variation of motion：Case of SV wave incidence
-
摘要: 针对地震动空间差异问题，以半无限空间内平面SV波入射为例，采用波动理论和叠加原理相结合的方法研究地震动的空间特征。首先，分析入射波和反射波在空间内形成质点运动的叠加模式差异，并依此对空间域进行划分；其次，针对不同泊松比和SV波入射角情形研究叠加区与分离区的分界线控制情况；最后，对比分析不同空间域内的质点运动在峰值、持时等方面的特征。研究结果显示：当SV波入射时，叠加区与分离区的分界线通常由z3 （反射P波与反射SV波的分离线）控制；同时存在两种特殊情况，当SV波垂直入射时，分界线由z1 （入射SV波与反射SV波的分离线）控制，当反射SV波幅值为零时，分界线由z2 （入射SV波与反射P波的分离线）控制。在入射波和反射波的影响下，质点运动时程的形状具有水平不变性。三波贡献时段只出现在深度小于z1的质点的运动时程中，且持时随着深度的增加线性减少；双波贡献时段出现在位于叠加区内的质点的运动时程中，持时沿深度先增加后减少；单波贡献时段随着深度的增加而逐渐加长，在分离区达到最大值。质点运动总持时随深度逐渐增加，在分离线z2和z3深度处存在两个拐点。在质点运动峰值方面，靠近自由面的叠加区质点运动峰值变化较大，深度较大的叠加区和分离区的质点运动峰值一般不变。Abstract: For the investigation of spatial variation of seismic motion, the wave theory and superposition principle are used to illustrate the spatial variation of motions caused by seismic waves. And plane SV wave’s incidence in semi-infinite space is used as a case in this paper. Firstly the difference in superposition pattern of waves in different position is investigated and the space region is divided into different domains according to superposition pattern. Then the border line between superposition domain and separation domain with different Poisson’s ratio and SV wave’s incident angle is discussed. Finally the difference in peak value and duration time of particle motion in different domains is investigated. The result shows that, the border line is usually controlled by z3 (separation line generated by reflected P wave and reflected SV wave). Particularly, there exist two special cases, one is the vertical incidence of SV wave in which the border line is controlled by z1 (separation line generated by incident SV wave and reflected SV wave), the other is the zero amplitude of reflected SV wave in which the border line is controlled by z2 (separation line generated by incident SV wave and reflected P wave). The time history of different particle motion in one depth has the same shape with the influence of incident and reflected waves. In time history of particle motion, the period contributed by three waves only exists in depth lower than z1 and the duration time decreases with depth. The period contributed by two waves exists in all superposition domain and the duration time increases firstly and then decreases with depth. The period contributed by one single wave increases with depth and reaches its maximum in separation domain. The increase of particle motion duration has two slope change in depths of z2 and z3. The peak value of particle motion changes dramatically in superposition domain near free surface and remains constant in deep superposition domain and separation domain.
-
引言
我国位于世界两大地震带之间，是强震发生频率较高的国家之一。自2008年汶川MS8.0大地震之后，陆续发生了一系列MS≥7.0强震，主要有2010年青海玉树MS7.1地震、2013年四川芦山MS7.0地震、2014年新疆和田MS7.3地震以及2017年四川九寨沟MS7.0地震。这些强震不仅损害着人员安全和经济发展，也严重威胁着高坝、地下结构、桥梁等大跨度结构的安全（陈厚群等，2008；张楚汉等，2016；林皋，2017）。
对大跨度结构进行地震响应分析时，须考虑一定空间范围内的地震动输入。地震台阵监测资料显示，不同空间位置的地面运动具有不可忽略的差异性（Zerva，2009）。地震动空间差异通常被归因为行波效应、衰减效应、相干效应和局部场地效应等（钟万勰等，2000；缪惠全，李杰，2018）。目前工程学领域通常采用相干函数模型来描述地震动的空间差异，相干函数模型可分为依实测台阵记录总结得到的经验模型和结合实测台阵记录与理论分析而得到的半经验模型（Luco，Wong，1986；Loh，Yeh，1988；Hao et al，1989；Zerva，Harada，1997）。相干函数模型为描述地面运动空间差异提供了可行途径，然而受计算复杂性的影响，通过相干函数模型获得非一致地震动场在结构地震响应分析中的应用尚不多见。在研究对象方面，相干函数模型通常侧重于地面运动，而大坝、地下结构等的地震响应分析所需地震动输入模型通常不仅注重地面运动，还注重地面以下的运动；在模型机制方面，相干函数模型属于唯象模型，侧重地震动空间差异的经验性描述，而未就物理过程考察地震动场的特征（金星，1993）。因此，从地震动形成机制出发研究地震动的空间差异，从而构建空间差异地震动场，成为地震动输入模型的发展方向。从形成机制方面描述地震动场的具体方法有金星和廖振鹏（1994）提出的随机地震动场模型、张翠然等（2011）提出的随机有限断层法以及贺春晖等（2017）通过建立震源—传播介质—坝址峡谷场地数值模型生成坝址区三维地震动场的方法。
研究地震动空间域特征，探索地震动空间差异的形成机理，是描述地震动场和获得准确地震动输入模型的前提。地震动场是由震源产生的地震波经传播介质到达场址后，入射波、反射波叠加而形成的运动场。因此，从波动理论和叠加原理出发开展研究是揭示地震动空间差异形成机理的可行思路。目前，基于波动理论的地震动空间差异研究主要体现在面波研究中，如瑞雷波和勒夫波的质点运动沿深度的变化（Shearer，2009）。然而，以波动理论解释地震运动空间差异的研究仍不多见，且通常未探讨多波叠加情况。为此，本文拟以半无限介质中SV波在临界角以内的入射为例，研究空间内由地震波传播效应和叠加效应形成的质点运动空间特征，从而为研究地震动空间差异的形成机理和非一致地震动场的构建提供依据。首先依据质点运动叠加模式对空间域进行划分，然后以无量纲的叠加区范围系数为基础探讨不同介质内不同入射角情形下空间域分界线的控制情况，最后对不同空间域内的质点运动在持时、峰值和时程形状等方面的差异进行对比分析。
1. 地震波叠加模式与质点运动空间差异
地震波在介质内的传播引起质点运动，如平面P波引起的质点运动方向与传播方向平行，平面S波引起的质点运动方向与传播方向垂直，瑞雷波引起的质点运动轨迹通常为椭圆。当多条地震波在相同空间区域传播时，质点运动由多条地震波运动叠加形成。由于地震波在波阵面形状、波型类别、传播方向、初至时间等方面可能存在差异，不同空间位置的质点运动所对应的地震波叠加方式通常存在差异。
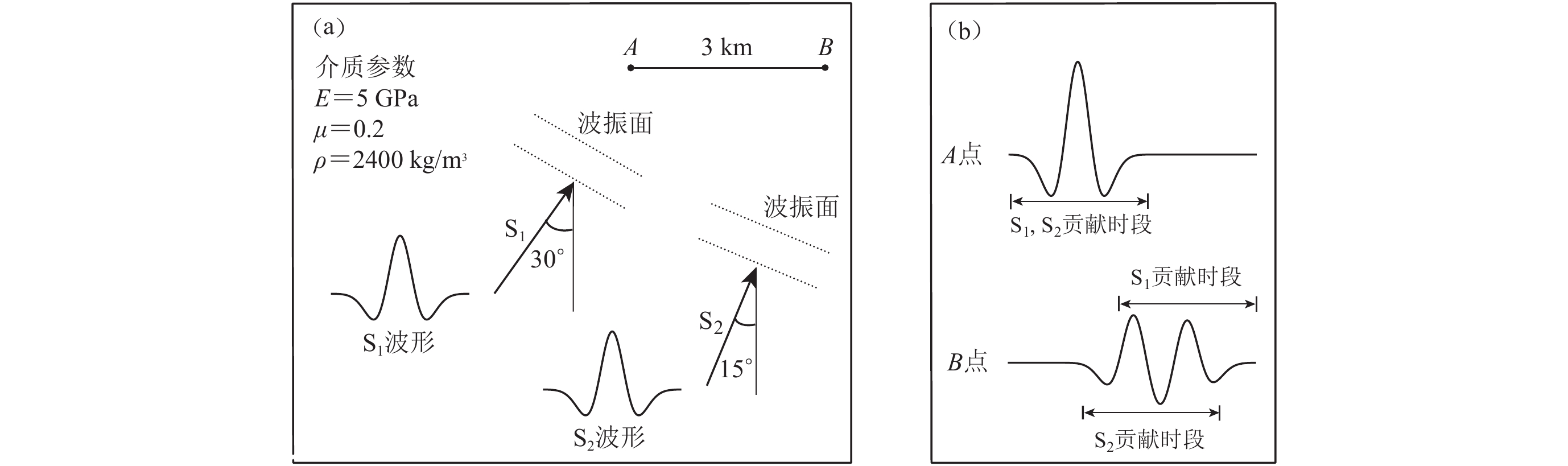
如图1a所示，在均匀介质中S1和S2为两条倾斜向上传播的SV波，分别以30°和15°入射，波前同时到达A点。依据叠加原理，可得A点和B点的水平向运动时程如图1b所示。在A点，S1和S2对质点运动的贡献时段相同，质点运动为两条地震波运动的直接叠加；在B点，S1和S2对质点运动的贡献时段存在差异，在叠加模式影响下，B点运动在幅值、持时和时程形状等方面与A点均相差较大。因此，研究地震波传播效应和叠加模式产生的运动空间差异，对解释地震动空间差异的形成机理具有重要意义。
![]()
图 1 地震波叠加模式与质点运动空间差异示意图（a） 问题说明；（b） A点和B点的水平向运动Figure 1. Superposition pattern of seismic waves and spatial variation of particle motion at different point（a） Description of problem；（b） Horizontal motion at points A and B2. 平面SV波倾斜入射时质点运动叠加模式及空间域划分
2.1 SV波倾斜入射时的反射系数
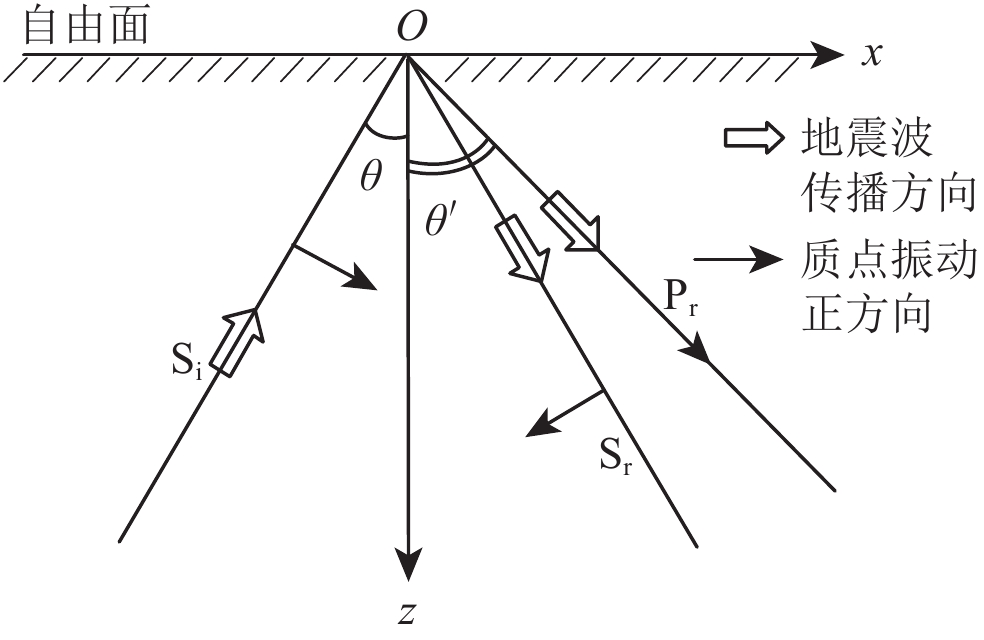
SV波倾斜入射到自由面时，受到自由面边界条件的限制，通常会产生反射P波和反射SV波两类波型。将入射SV波记为Si，反射P波记为Pr，反射SV波记为Sr。SV波倾斜入射情形下半无限介质内的地震波情况，如图2所示，半无限空间内通常存在Si，Pr和Sr共3条地震波。
依据自由面应力和位移边界条件，反射P波和反射SV波的幅值系数为
$ {A_{\rm{P}}} {\text{＝}} \frac{{2{v_{\rm{S}}}{v_{\rm{P}}}\sin 2\theta \cos 2\theta }}{{v_{\rm{S}}^2\sin 2\theta \sin 2\theta{\text{′}}\!\!\!\!\!\!{\text{＋}} v_{\rm{P}}^2{{\cos }^2}2\theta }}{\text{，}} $
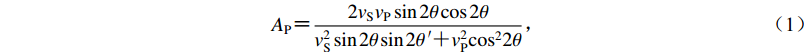
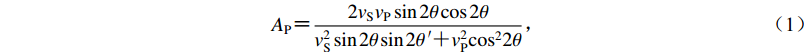
(1) $ {A_{\rm{S}}} {\text{＝}} \frac{{v_{\rm{S}}^2\sin 2\theta \sin 2\theta{\text{′}}\!\!\!\!\!\!{\text{－}} v_{\rm{P}}^2{{\cos }^2}2\theta }}{{v_{\rm{S}}^2\sin 2\theta \sin 2\theta {\text{′}}\!\!\!\!\!\!{\text{＋}} v_{\rm{P}}^2{{\cos }^2}2\theta }}{\text{，}} $
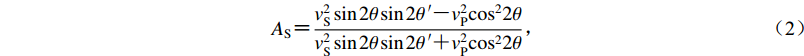
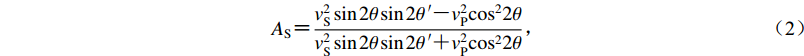
(2) This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
$ {v_{\rm{P}}} {\text{＝}} \sqrt {\frac{{E(1 {\text{－}}\mu)}}{{\rho (1 {\text{＋}} \mu)(1{\text{－}} 2\mu)}}}{\text{，}} $
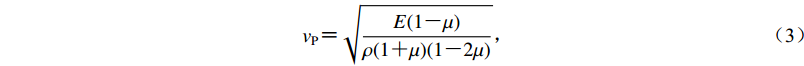
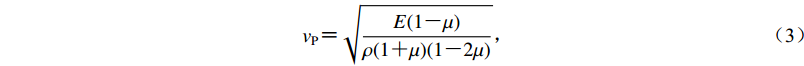
(3) $ {v_{\rm{S}}} {\text{＝}}\sqrt {\frac{E}{{2\rho (1 {\text{＋}} \mu)}}}{\text{，}} $
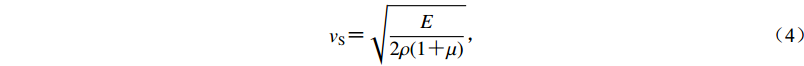
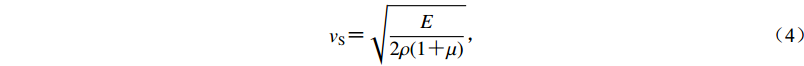
(4) 式中，E，μ和ρ分别为半无限空间内介质的弹性模量、泊松比和密度。
2.2 空间内任意质点的地震波到时和离时
平面SV波倾斜入射时，半无限空间介质中通常存在3条地震波，即Si，Pr和Sr。当单一地震波在空间内传播时，对于任意的空间质点，波前到达时质点开始运动，波后离开时质点停止运动。将质点开始运动和停止运动的时间称为地震波的到时和离时。
This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
$ t_{{\rm{S}}_{\rm{i}}}^{\rm{a}} {\text{＝}} \frac{{x\sin \theta {\text{－}} {\textit{z}}\cos \theta }}{{{v_{\rm{S}}}}}{\text{，}} $
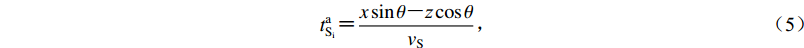
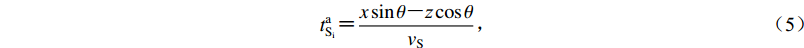
(5) $ t_{{\rm{P}}_{\rm{r}}}^{\rm{a}} {\text{＝}} \frac{{x\sin \theta {\text{′}}\!\!\!\!\!\! {\text{＋}} {\textit{z}}\cos \theta {\text{′}}\!\!\!\!\!\!}}{{{v_{\rm{P}}}}}{\text{，}} $
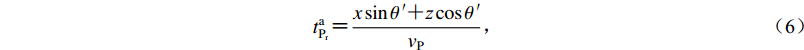
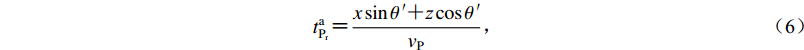
(6) $ t_{{\rm{S}}_{\rm{r}}}^{\rm{a}} {\text{＝}} \frac{{x\sin \theta {\text{＋}} {\textit{z}}\cos \theta }}{{{v_{\rm{S}}}}}{\text{.}} $
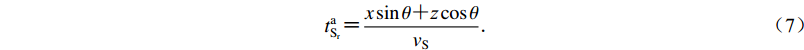
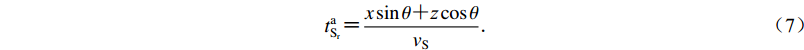
(7) 假设入射波的持时为T，则反射波的持时也为T，Si，Pr和Sr波在同一质点的离时可表示为
$ t_{{\rm{S}}_{\rm{i}}}^{\rm{l}} {\text{＝}} \frac{{x\sin \theta {\text{－}}{\textit{z}}\cos \theta }}{{{v_{\rm{S}}}}} {\text{＋}} T{\text{，}} $
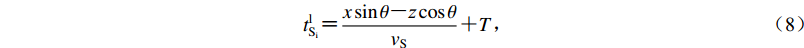
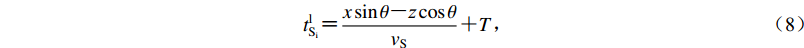
(8) $ t_{{\rm{P}}_{\rm{r}}}^{\rm{l}} {\text{＝}} \frac{{x\sin \theta {\text{′}}\!\!\!\!\!\! {\text{＋}} {\textit{z}}\cos \theta {\text{′}}\!\!\!\!\!\!}}{{{v_{\rm{P}}}}} {\text{＋}} T{\text{，}} $
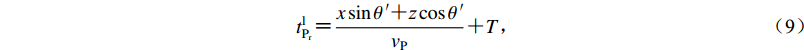
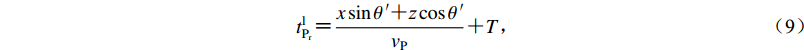
(9) $ t_{{\rm{S}}_{\rm{r}}}^{\rm{l}} {\text{＝}} \frac{{x\sin \theta {\text{＋}} {\textit{z}}\cos \theta }}{{{v_{\rm{S}}}}} {\text{＋}} T{\text{.}} $
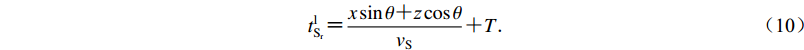
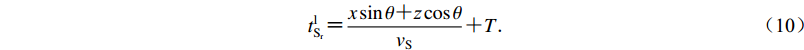
(10) This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
2.3 空间区域的分离线和分界线
This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
$ {{\textit{z}}_1} {\text{＝}} \frac{{{v_{\rm{S}}}T}}{{2\cos \theta }}{\text{.}} $
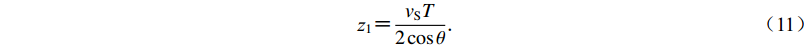
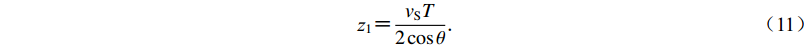
(11) 当介质和入射波信息已知时，z1为确定数值，被称为分离线。依据地震波传播规律，分离线的物理意义为两条地震波对质点运动贡献时段开始分离的空间位置。当质点坐标大于分离线z1时，Si和Sr对质点运动的贡献时段在时域内是分离的；当质点坐标小于分离线z1时，Si和Sr对质点运动的贡献时段存在重叠。同样可得到Si与Pr、Pr与Sr的分离线z2和z3分别为
$ {{\textit{z}}_2} {\text{＝}} \frac{{{v_{\rm{P}}}{v_{\rm{S}}}T}}{{{v_{\rm{P}}}\cos \theta {\text{＋}} {v_{\rm{S}}}\cos \theta {\text{′}}\!\!\!\!\!\!}}{\text{，}} $
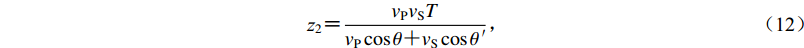
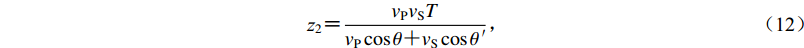
(12) $ {{\textit{z}}_3} {\text{＝}} \frac{{{v_{\rm{P}}}{v_{\rm{S}}}T}}{{{v_{\rm{P}}}\cos \theta{\text{－}} {v_{\rm{S}}}\cos \theta {\text{′}}\!\!\!\!\!\!}}{\text{.}} $
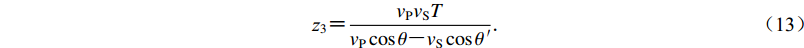
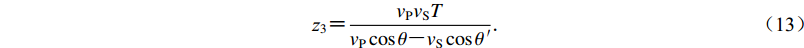
(13) 平面SV波倾斜入射的情形下，质点运动通常由3条地震波共同贡献而形成，将质点运动中3条地震波贡献时段均不出现重叠的空间区域定义为分离区，将质点运动存在于任意地震波重叠贡献时段的空间区域定义为叠加区，将分离区与叠加区的界线称为分界线，分界线由z1，z2，z3中的最大值控制。
3. 平面SV波倾斜入射下叠加区范围系数及分界线控制
3.1 叠加区范围系数
依据斯奈尔（Snell）定律（Shearer，2009）
$ \frac{{{v_{\rm{P}}}}}{{{v_{\rm{S}}}}} {\text{＝}} \frac{{\sin \theta{\text{′}}\!\!\!\!\!\!}}{{\sin \theta }}{\text{，}} $
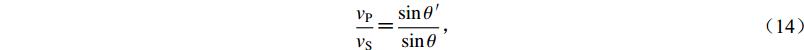
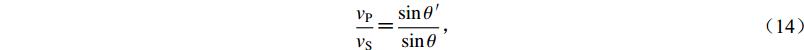
(14) $ {{\textit{z}}_2} {\text{＝}} \frac{{{v_{\rm{S}}}T}}{{\cos \theta {\text{＋}}\displaystyle\frac{\sin \theta \cos \theta {\text{′}}\!\!\!\!\!\!}{\sin \theta {\text{′}}\!\!\!\!\!\!}}}{\text{，}} $
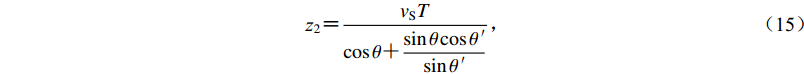
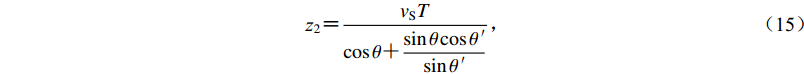
(15) $ {{\textit{z}}_3} {\text{＝}} \frac{{{v_{\rm{S}}}T}}{\cos \theta {\text{－}} \displaystyle\frac{\sin \theta \cos \theta {\text{′}}\!\!\!\!\!\!}{\sin \theta {\text{′}}\!\!\!\!\!\!}}{\text{.}} $
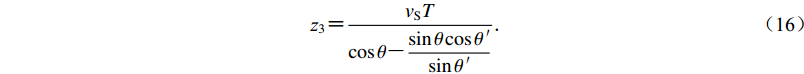
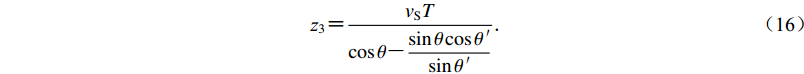
(16) 对比式（11）、（15）和（16），z1，z2和z3的表达式中除波速vS和持时T外均为无量纲量，将这些无量纲部分定义为叠加区范围系数，得到反映分离线位置的3个叠加区范围系数a1，a2和a3分别为
$ {a_1} {\text{＝}} \frac{1}{{2\cos \theta }}{\text{，}} $
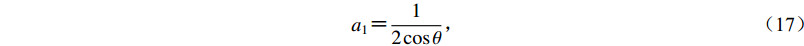
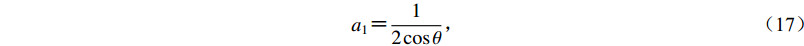
(17) $ {a_2} {\text{＝}} \frac{{\sin \theta{\text{′}}\!\!\!\!\!\!}}{{\cos \theta \sin \theta {\text{′}}\!\!\!\!\!\!}{\text{＋}}\sin \theta \cos \theta {\text{′}}\!\!\!\!\!\!}{\text{，}} $
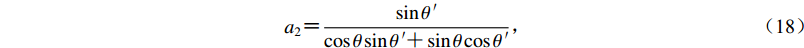
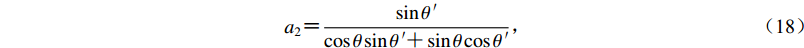
(18) $ {a_3}{\text{＝}} \frac{{\sin \theta{\text{′}}\!\!\!\!\!\!}}{{\cos \theta \sin \theta{\text{′}}\!\!\!\!\!\!} {\text{－}} \sin \theta \cos \theta{\text{′}}\!\!\!\!\!\!}{\text{.}} $
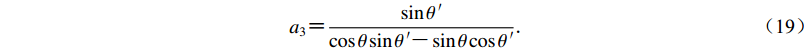
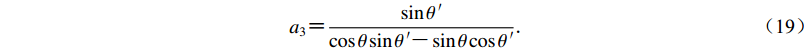
(19) 由于本文研究的是平面SV波入射角小于临界角的情况，因此以上公式须加限制条件
$ \theta {\text{＜}} \arcsin \left(\frac{{{v_{\rm{S}}}}}{{{v_{\rm{P}}}}}\right){\text{＝}}\arcsin \sqrt {\frac{{1 {\text{－}} 2\mu }}{{2(1 {\text{－}} \mu)}}}{\text{.}} $
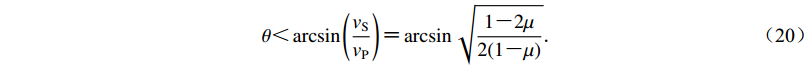
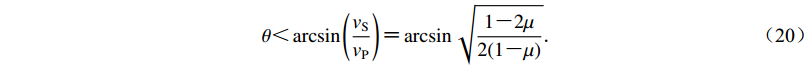
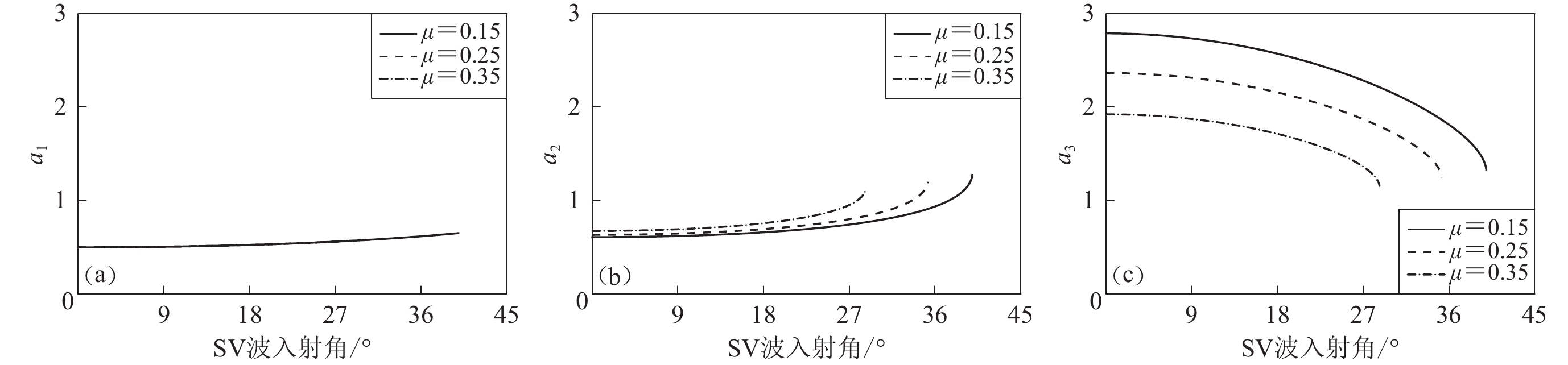
(20) 由式（17）—（19）可见，3个叠加区范围系数中，a1仅与SV波的入射角度有关，a2和a3受入射角和反射角的共同影响。当入射角已知时，反射角可由泊松比μ确定，因此叠加区范围系数仅受入射角和泊松比的影响。考虑到实际介质的泊松比通常在0.15—0.35之间，分别选取泊松比为0.15，0.25和0.35时研究叠加区范围系数随入射角度的变化情况，结果如图3所示。图中仅显示临界角以下的叠加区范围系数情况，泊松比为0.15，0.25和0.35时3种介质所对应的临界角分别为39.9°，35.3°和28.7°。
![]()
图 3 不同泊松比时叠加区范围系数a1 （a），a2 （b）和a3 （c）随平面SV波入射角的变化Figure 3. Variation of superposition range coefficient a1 （a），a2 （b） and a3 （c） with incident angle of SV wavea1在不同泊松比情况下的曲线重合（图3a），代表Si与Sr的分离线仅受SV波入射角的影响，而与介质信息无关；a2表现出随泊松比增加而逐渐增大的规律（图3b）；a3表现出随泊松比增加而逐渐减小的规律（图3c），说明随着介质变软，Pr与Sr波的叠加区域逐渐减小。
如图3所示：a1和a2均表现出随入射角的增加而逐渐增大的规律，说明随着入射角的增加，Si与Sr、Si与Pr对应的分离线逐渐变深，叠加区范围逐渐增大；a3表现出随入射角度增加而逐渐减小的规律，说明随着入射角的增加，Pr与Sr对应的分离线逐渐变浅，叠加区范围逐渐减小。
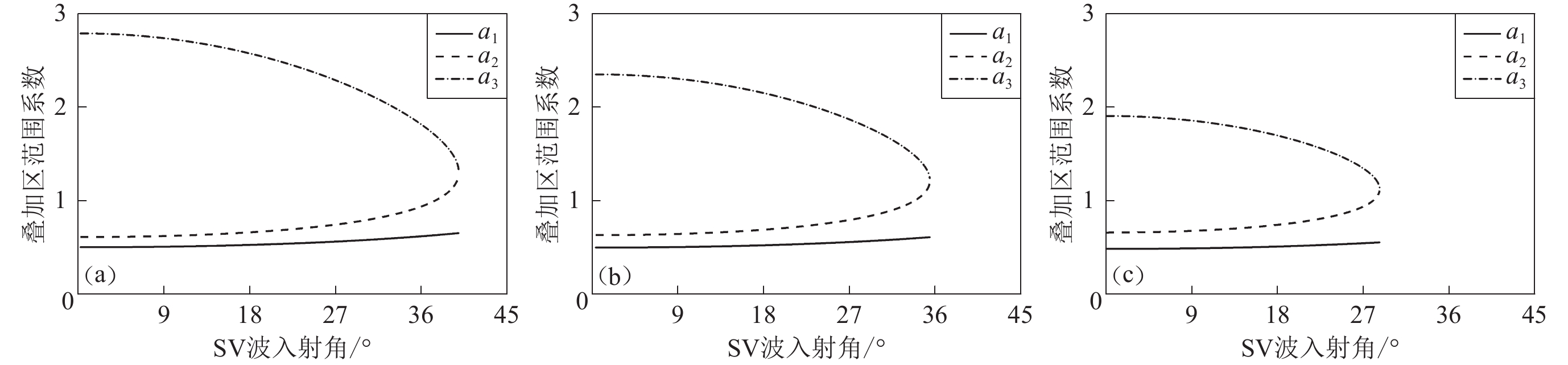
3.2 分界线控制情况
叠加区范围系数同时影响了Pi，Pr和Sr在空间域内的叠加模式，因此有必要研究叠加区范围系数之间的大小关系。图4给出了泊松比分别为0.15，0.25和0.35时，3个叠加区范围系数的对比曲线，可以看出，不同泊松比和入射角条件下均满足a3＞a2＞a1，说明平面SV波倾斜入射情形下，叠加区与分离区的分界线通常由Pr与Sr的分离线控制。
![]()
图 4 泊松比为0.15 （a），0.25 （b）和0.35 （c）时叠加区范围系数对比Figure 4. Comparison of superposition range coefficient with Poisson’s ratio of 0.15 （a），0.25 （b） and 0.35 （c）3.3 特殊情况下的分界线
上文讨论了空间内存在3条地震波时的分界线控制情况，此外，平面SV波入射时空间内可能只有2条地震波，分别为SV波垂直入射和反射SV波幅值为0两类特殊情况。
当平面SV波垂直入射时，空间内只存在Si和Sr地震波，分界线由Si与Sr的分离线控制，此时分离线对应的叠加区范围系数为a1，且a1＝0.5。
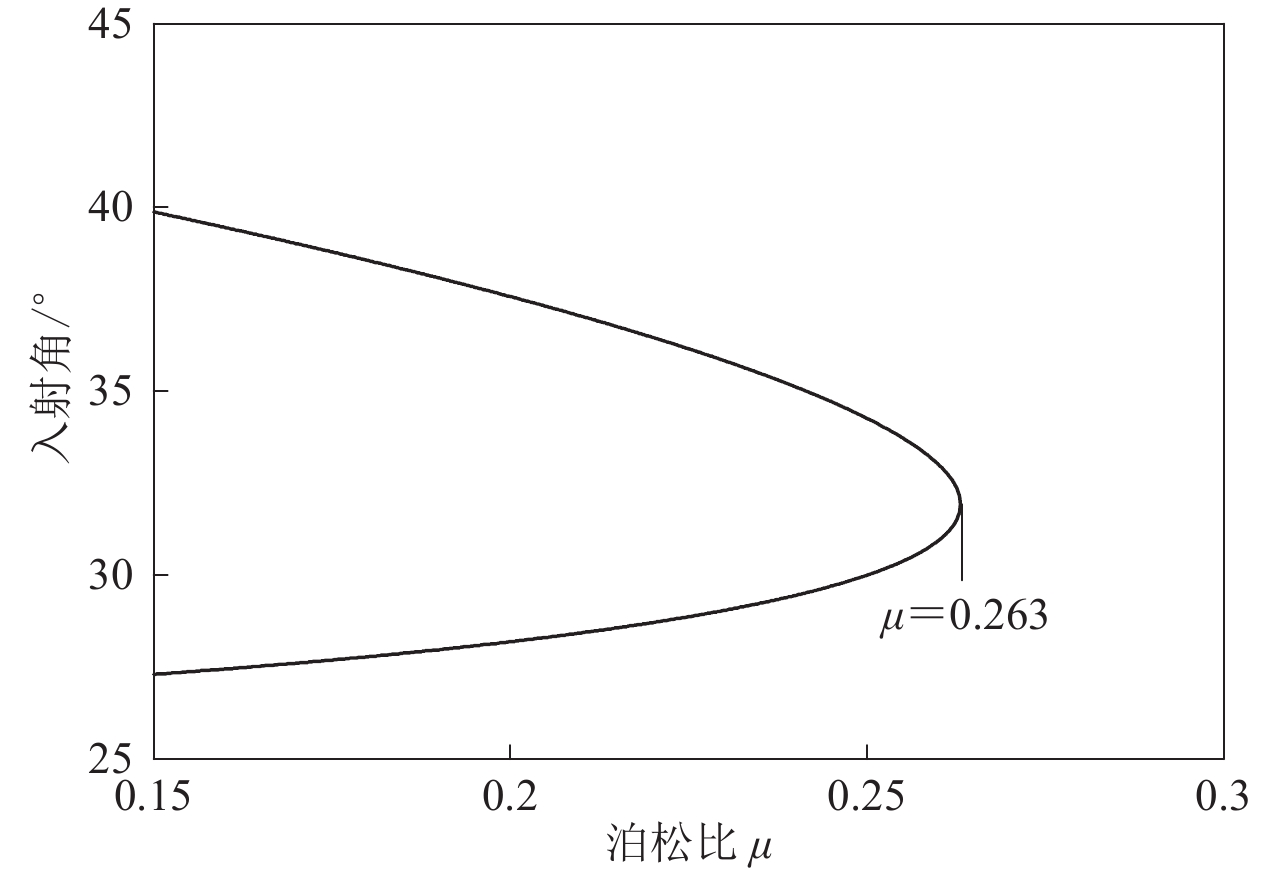
当SV波幅值系数为0时，空间内只存在Si和Pr地震波，分界线由Si与Pr的分离线控制，此时分离线对应的叠加区范围系数为a2。由Sr幅值系数AS＝0得到分离线由系数a2控制时，入射角、反射角和泊松比须满足
$ \frac{{\sin 2\theta \sin 2\theta{\text{′}\!\!\!}}}{{{{\cos }^2}2\theta }}{\text{－}}\frac{{2(1{\text{－}} \mu)}}{{1 {\text{－}} 2\mu }} {\text{＝}} 0{\text{.}} $
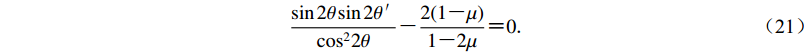
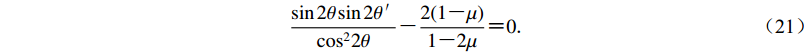
(21) 由于反射角由入射角θ和泊松比μ共同确定，因此式（21）仅包含入射角和泊松比两个未知量。当介质泊松比已知时，可依据该式求得满足条件的入射角。通过matlab程序求解式（21），得到不同泊松比介质分界线由叠加区范围系数a2控制对应的入射角，如图5所示，可以看出分界线由系数a2控制的入射角数量的规律为：当μ＜0.263时，存在2个满足条件的入射角；当μ＝0.263时，存在1个满足条件的入射角；当μ＞0.263时，不存在满足条件的入射角。
![]()
图 5 分界线由Si与Pr分离线控制的对应情况Figure 5. Border controlled by separation line generated by Si and Pr4. 平面SV波倾斜入射空间内质点运动的水平不变性
4.1 质点运动表达式
半无限空间内，平面SV波的波函数可表示为
$ f(t {\text{，}}\!\! x {\text{，}}\!\! {\textit{z}}) {\text{＝}}f\left(t - \frac{{x\sin \theta {\text{－}} {\textit{z}}\cos \theta }}{{{v_{\rm{S}}}}}\right){\text{，}} $
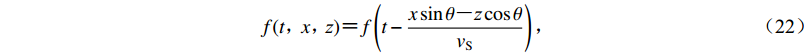
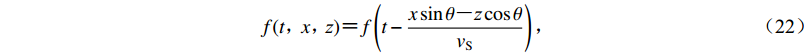
(22) 反射P波和反射SV波的波函数可表示为
$ {f_{\rm{P}}}(t {\text{，}}\!\! x {\text{，}}\!\! {\textit{z}}) {\text{＝}} {A_{\rm{P}}}f\left(t {\text{－}} \frac{{x\sin \theta{\text{′}}\!\!\!\!\!\! {\text{＋}} {\textit{z}}\cos \theta{\text{′}}\!\!\!\!\!\!}}{{{v_{\rm{P}}}}}\right) {\text{，}} $
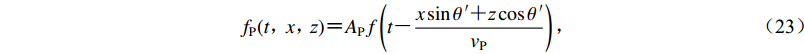
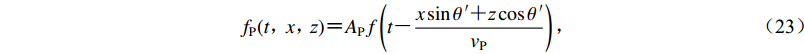
(23) $ {f_{\rm{S}}}(t {\text{，}}\!\!x {\text{，}}\!\! {\textit{z}}) {\text{＝}} {A_{\rm{S}}}f\left(t{\text{－}}\frac{{x\sin \theta {\text{＋}}{\textit{z}}\cos \theta }}{{{v_{\rm{S}}}}}\right){\text{.}} $
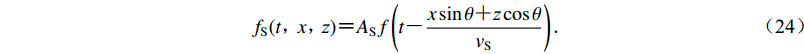
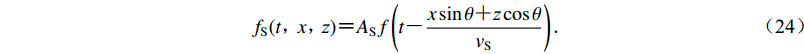
(24) 半无限介质内质点运动的水平分量和竖直分量可依据3条地震波的波函数表示为
$ h(t {\text{，}}\!\!x {\text{，}}\!\! {\textit{z}}) {\text{＝}} f(t {\text{，}}\!\! x {\text{，}}\!\!\!\! {\textit{z}})\cos \theta {\text{＋}} {f_{\rm{P}}}(t{\text{，}}\!\! x{\text{，}} \!\!{\textit{z}})\sin \theta {\text{′}}\!\!\!\!\!\! {\text{－}} {f_{\rm{S}}}(t {\text{，}}\!\! x {\text{，}}\!\! {\textit{z}})\cos \theta {\text{，}} $
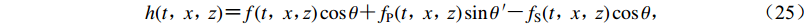
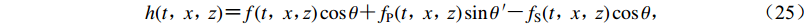
(25) $ v(t{\text{，}}\!\! x{\text{，}}\!\! {\textit{z}}) {\text{＝}} f(t{\text{，}}\!\! x{\text{，}}\!\! {\textit{z}})\sin \theta {\text{＋}}{f_{\rm{P}}}(t{\text{，}}\!\!x{\text{，}}\!\!{\textit{z}})\cos \theta {\text{′}}\!\!\!\!\!\! {\text{＋}} {f_{\rm{S}}}(t{\text{，}}\!\! x{\text{，}}\!\! {\textit{z}})\sin \theta{\text{.}} $
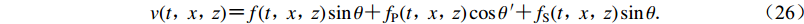
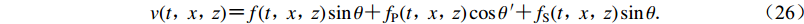
(26) 4.2 质点运动时程形状的水平不变性
This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
$ h(t{\text{，}}x {\text{＋}} \Delta x{\text{，}}{\textit{z}}) {\text{＝}} h\left(t {\text{－}} \frac{{\Delta x\sin \theta }}{{{v_{\rm{S}}}}}{\text{，}}x{\text{，}} {\textit{z}}\right){\text{，}} $
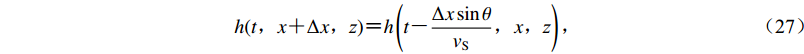
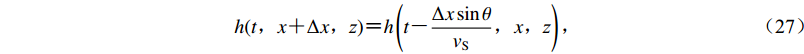
(27) $ v(t{\text{，}}\!\! x {\text{＋}} \Delta x{\text{，}}\!\! {\textit{z}}){\text{＝}} v\left(t {\text{－}} \frac{{\Delta x\sin \theta }}{{{v_{\rm{S}}}}}{\text{，}}\!\! x{\text{，}}\!\! {\textit{z}}\right){\text{.}} $
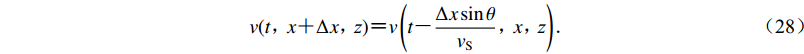
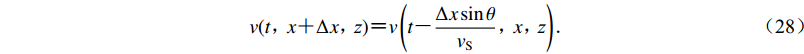
(28) 式（27）和（28）可直接解释为：在半无限介质内SV波倾斜入射情况下，（x＋Δx，z）处质点在t时刻的运动与（x，z）处质点在t－Δxsinθ/vS时刻的运动相同，其物理意义可简单描述为质点运动的时程形状不随水平坐标变化，或称为时程形状的水平不变性。
5. 质点运动空间差异
5.1 介质特性与入射地震波选取
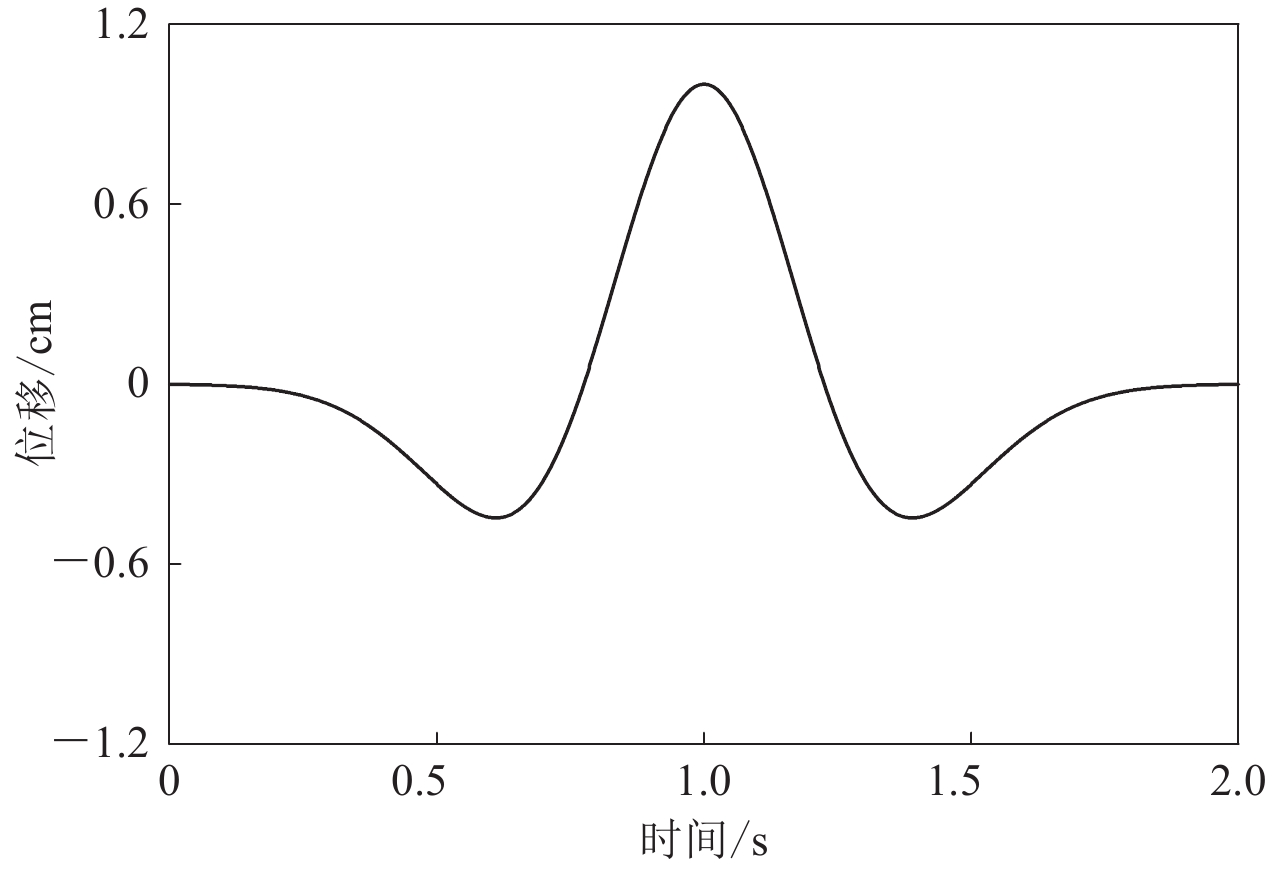
采用简单算例对不同空间域的质点运动特征进行对比分析。假定半无限介质的弹性模量为15 GPa，泊松比为0.22，密度为2 400 kg/m3。若平面SV波以20°倾斜入射，入射地震波选为持时为2 s，位移峰值为1 cm的雷克子波，其运动时程如图6所示。
依据给定参数，可得到3个分离线深度分别为z1＝1.70 km，z2＝2.24 km，z3＝7.15 km。给定介质参数和地震波持时下，Si和Sr在深度1.70 km处开始分离，Si和Pr在深度2.24 km处开始分离，Pr和Sr在深度7.15 km开始分离。叠加区与分离区的分界线深度为7.15 km。
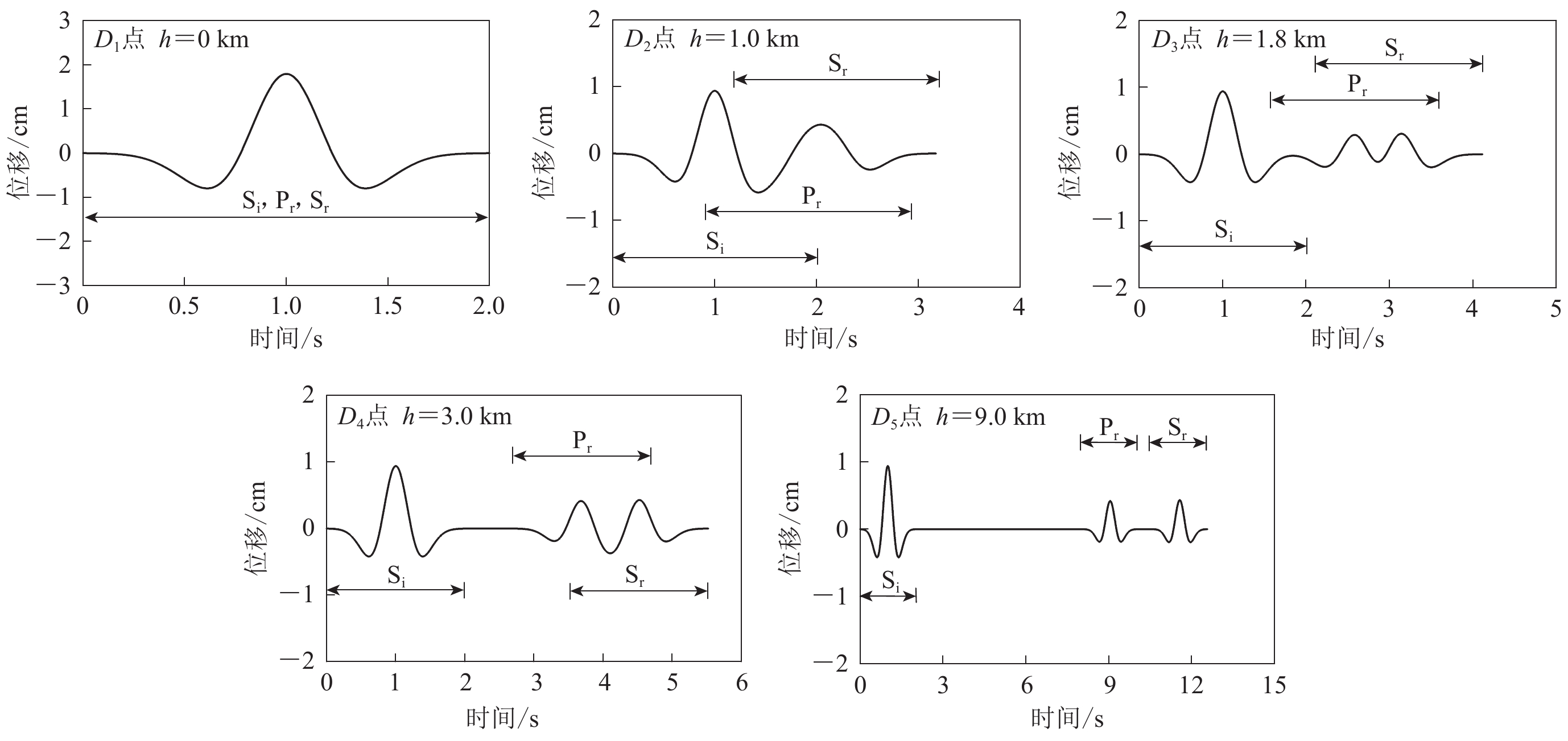
5.2 不同空间域质点运动时程
分别在自由面、叠加区和分离区选择特征点，研究其运动时程特征。自由面选取深度为0 km的质点D1；叠加区分别选取深度为1 km的质点D2；深度为1.8 km的质点D3；深度为3 km的质点D4；分离区选取深度9 km的质点D5。5个特征质点的水平向运动时程如图7所示，为便于显示，图中时间零点选为第1个贡献地震波的到时。每个质点的运动时程都标识了3条地震波对其运动时程的贡献时段，可以看出：在叠加区质点运动时程中，存在不同程度的地震波重叠贡献时段；在分离区质点运动时程中，3条地震波的贡献时段是分离的。
![]()
图 7 不同深度h下特征位置质点的水平向运动时程Figure 7. Time history of horizontal particle motions in different depth h对于质点D1和D2，深度小于分离线z1，Si、Pr和Sr中任意两条地震波均存在叠加贡献时段；对于质点D3，深度大于z1且小于z2和z3，Si和Sr开始分离，Si和Pr、Pr和Sr仍然存在叠加贡献时段；对于质点D4，深度大于z1和z2，小于z3，Si和Sr、Si和Pr在时域内呈现分离状态，Pr和Sr仍存在叠加贡献时段；对于质点D5，深度大于z3，3条地震波在时域内均呈现分离状态。
5.3 地震波贡献时段
为研究不同质点运动时程中地震波的贡献情况，在表1中列出了5个质点运动时程中单波、双波和三波贡献时段的起止时间。可以看出：质点D1的运动时程中，3条地震波的贡献时段完全重叠，仅存在三波贡献时段，持时为2 s；质点D2的运动时程中，存在单波、双波和三波贡献时段；质点D3和D4的运动时程中，存在单波和双波贡献时段；质点D5的运动时程中，仅存在单波贡献时段，Si、Pr和Sr的贡献时段在时域分离。
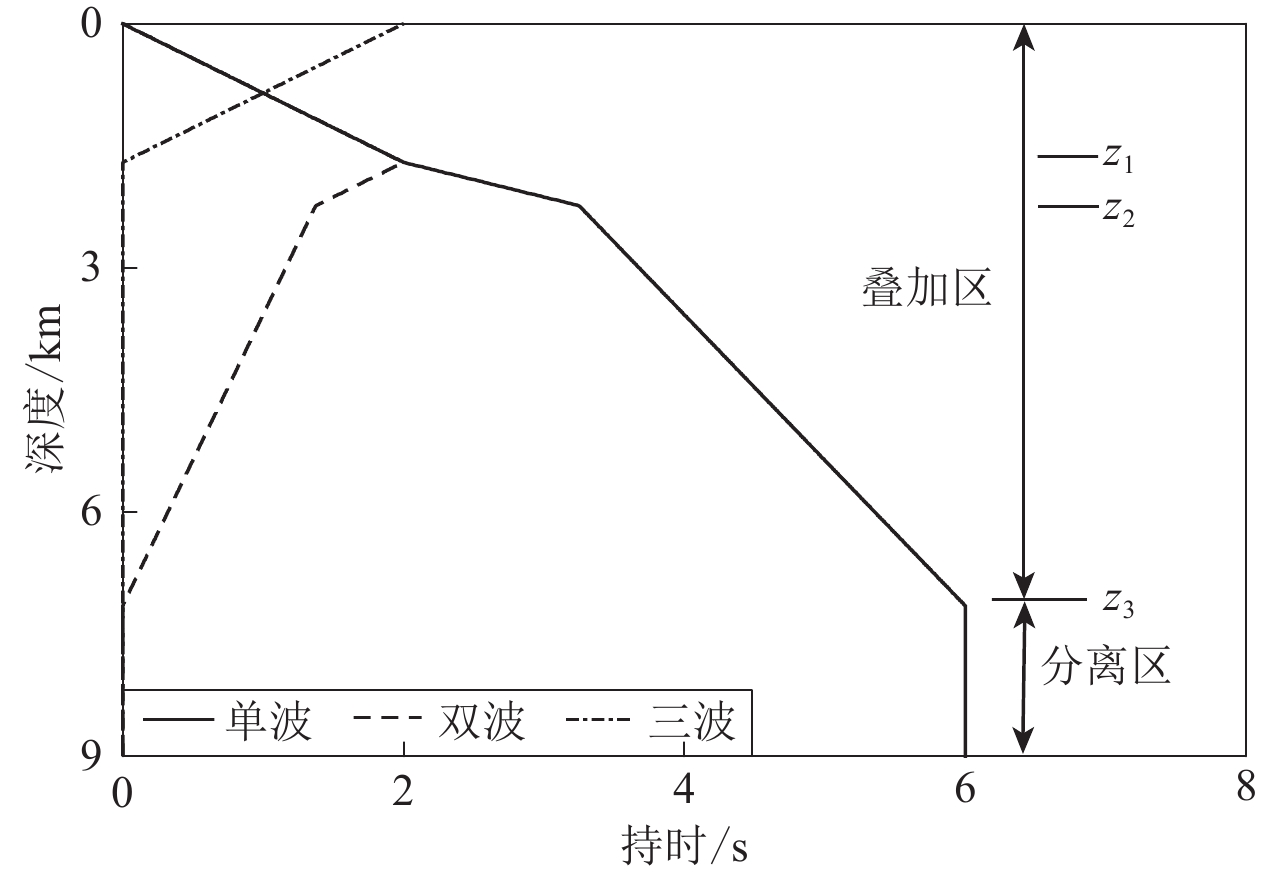
表 1 不同深度质点地震波贡献时段Table 1. Contribution time interval of waves on particle motion at different depth质点 深度/km 单波贡献时段/s 双波贡献时段/s 三波贡献时段/s Si Pr Sr （Si，Pr） （Pr，Sr） （Si，Pr，Sr） D1 0 0—2.00 D2 1.0 0—0.89 2.89—3.17 0.89—1.17 2.00—2.89 1.17—2.00 D3 1.8 0—1.61 2.00—2.11 3.61—4.11 1.61—2.00 2.11—3.61 D4 3.0 0—2.00 2.68—3.52 4.68—5.52 3.52—4.68 D5 9.0 0—2.00 8.05—10.05 10.57—12.57 在深度9 km以内，质点运动时程中单波、双波和三波贡献时段的持时随深度的变化，如图8所示。可见：三波贡献时段只在深度小于z1区域出现，且三波贡献持时随着深度的增加而线性减少；双波贡献时段在整个叠加区域出现，在深度小于z1的坐标区域随深度线性增加，在深度大于z1的坐标区域随深度的增加而逐渐减少；单波贡献持时随着深度的增加而逐渐增多，在达到分离区时单波贡献持时达到最大值，即3倍入射波持时。
![]()
图 8 不同地震波叠加类型下持时沿深度分布Figure 8. Duration of different superposition pattern of particle motion in different depth5.4 介质参数对强叠加区质点运动空间差异的影响
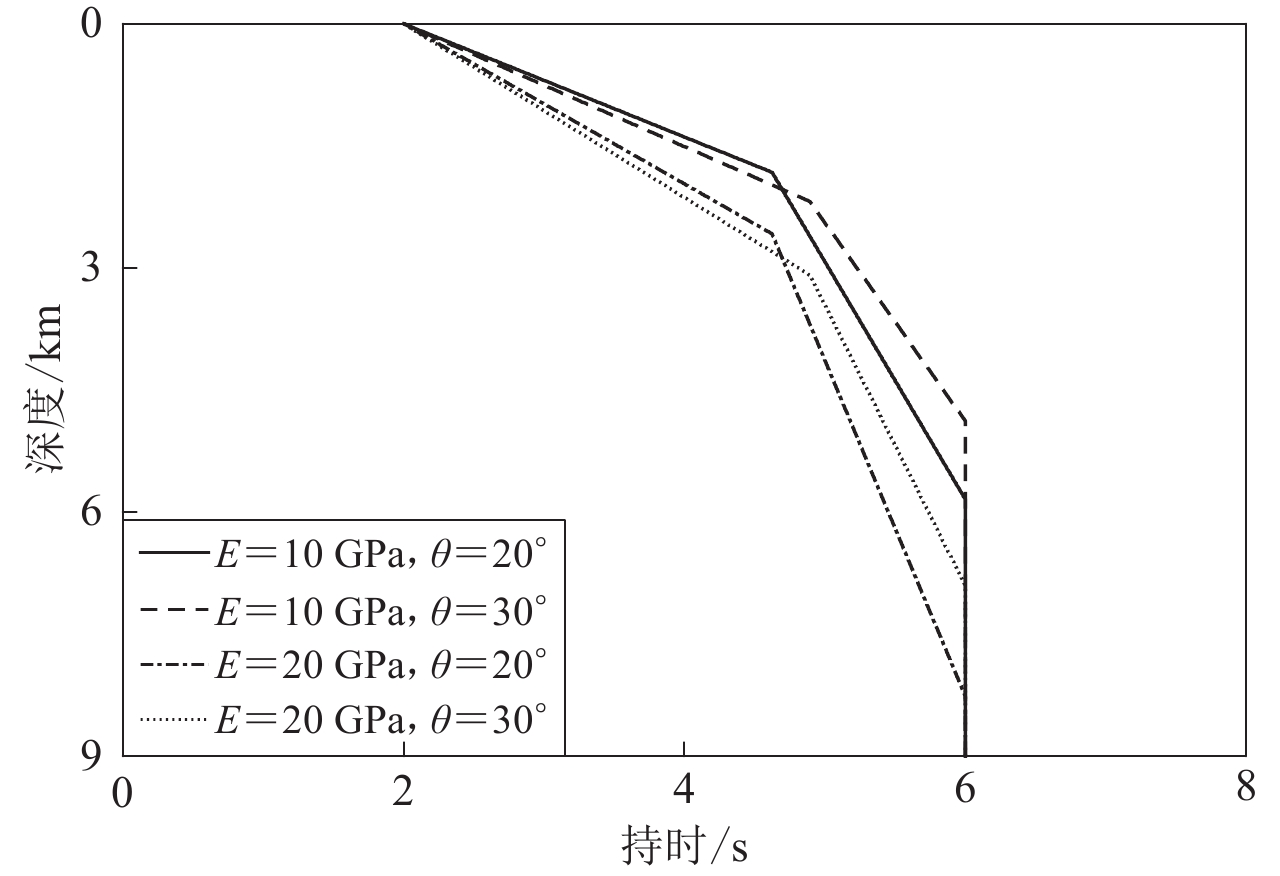
介质参数对地震波传播速度产生影响，从而对质点运动空间差异产生影响。为研究不同计算条件下质点运动持时、峰值随深度的变化，计算工况中半无限介质密度取2 400 kg/m3，泊松比取0.22，弹性模量分别取10 GPa和20 GPa，SV波入射角取20°和30°，通过组合得到4种计算工况。
4种工况的质点运动持时随深度的变化如图9所示。可以看出：受叠加模式的影响，质点运动持时随深度逐渐增加；运动持时沿深度增加的过程中出现了两个拐点，第1个拐点在z2深度处，第2个拐点在z3深度处。这两处出现拐点的主要原因是分别出现了无地震波贡献时段：从z2深度开始，在Si波与Pr波之间出现无地震波贡献时段；从z3深度开始，在Pr波与Sr波之间出现无地震波贡献时段。不同工况下，持时沿深度增加的规律存在差异，一方面体现在拐点出现的深度上，即分离线深度的不同，另一方面则体现在持时变化速率的差异上。
质点运动位移峰值沿深度的分布如图10所示，可以看出：在靠近自由面时，位移峰值变化较大；当深度增加到一定程度后，水平向和垂直向位移峰值均不再变化。从范围上看，峰值变化区域的范围小于叠加区范围，主要原因是在深度较大的叠加区，对质点运动产生贡献的地震波虽然存在重叠时段，但并未影响到峰值。因此可将叠加区内峰值受到地震波叠加模式影响的区域称为强叠加区，将峰值不受地震波叠加模式影响的区域称为弱叠加区。
![]()
图 10 质点运动水平向（a）、垂直向（b）峰值沿深度变化Figure 10. Peak displacement of particle motion in horizontal （a） and vertical （b） displacement along depth6. 讨论与结论
本文以波动理论和叠加原理为基础，针对平面SV波在临界角以下的入射情况，研究半无限介质内空间域划分及质点运动的空间差异。与平面P波情况类似（何卫平等，2019），平面SV波倾斜入射时，空间域可依据地震波叠加模式划分为叠加区和分离区，但平面SV波入射情况下分界线的控制情况较为简单。在质点运动空间差异方面，平面SV波倾斜入射时由入射波和反射波叠加形成的质点运动空间差异主要体现在沿深度方向的变化。沿深度方向的质点运动不仅具有峰值差异，在运动持时和时程形状方面也存在差异。本文研究在揭示场址区域地震动空间差异的形成机理方面具有基础作用，对非一致地震响应分析中地震动场的构建具有借鉴意义。
研究基于平面SV波在临界角以下的倾斜入射，采用的地震波为雷克子波，考虑因素较简单。实际地震发生时，受震源破裂机制和传播路径的复杂性的影响，场址的入射地震波数量可能更多、波型构成和波函数可能更复杂。总结实际地震在场址形成的入射地震波规律，研究复杂入射地震波影响下场址地震动的空间特征，是可以继续推进的研究工作。
-
![]()
图 1 地震波叠加模式与质点运动空间差异示意图
（a） 问题说明；（b） A点和B点的水平向运动
Figure 1. Superposition pattern of seismic waves and spatial variation of particle motion at different point
（a） Description of problem；（b） Horizontal motion at points A and B
![]()
图 3 不同泊松比时叠加区范围系数a1 （a），a2 （b）和a3 （c）随平面SV波入射角的变化
Figure 3. Variation of superposition range coefficient a1 （a），a2 （b） and a3 （c） with incident angle of SV wave
![]()
图 4 泊松比为0.15 （a），0.25 （b）和0.35 （c）时叠加区范围系数对比
Figure 4. Comparison of superposition range coefficient with Poisson’s ratio of 0.15 （a），0.25 （b） and 0.35 （c）
![]()
图 5 分界线由Si与Pr分离线控制的对应情况
Figure 5. Border controlled by separation line generated by Si and Pr
![]()
图 7 不同深度h下特征位置质点的水平向运动时程
Figure 7. Time history of horizontal particle motions in different depth h
![]()
图 8 不同地震波叠加类型下持时沿深度分布
Figure 8. Duration of different superposition pattern of particle motion in different depth
![]()
图 10 质点运动水平向（a）、垂直向（b）峰值沿深度变化
Figure 10. Peak displacement of particle motion in horizontal （a） and vertical （b） displacement along depth
表 1 不同深度质点地震波贡献时段
Table 1 Contribution time interval of waves on particle motion at different depth
质点 深度/km 单波贡献时段/s 双波贡献时段/s 三波贡献时段/s Si Pr Sr （Si，Pr） （Pr，Sr） （Si，Pr，Sr） D1 0 0—2.00 D2 1.0 0—0.89 2.89—3.17 0.89—1.17 2.00—2.89 1.17—2.00 D3 1.8 0—1.61 2.00—2.11 3.61—4.11 1.61—2.00 2.11—3.61 D4 3.0 0—2.00 2.68—3.52 4.68—5.52 3.52—4.68 D5 9.0 0—2.00 8.05—10.05 10.57—12.57 
下载: 导出CSV
-
陈厚群,徐泽平,李敏. 2008. 汶川大地震和大坝抗震安全[J]. 水利学报,39(10):1158–1167. doi: 10.3321/j.issn:0559-9350.2008.10.002 Chen H Q,Xu Z P,Li M. 2008. Wenchuan earthquake and seismic safety of large dams[J]. Journal of Hydraulic Engineering,39(10):1158–1167 (in Chinese).
贺春晖,王进廷,张楚汉. 2017. 基于震源—河谷波场数值模拟的坝址地震动参数确定方法[J]. 地球物理学报,60(2):585–592. He C H,Wang J T,Zhang C H. 2017. Determination of seismic parameters for dam sites by numerical simulation of the rupture-canyon wave field[J]. Chinese Journal of Geophysics,60(2):585–592 (in Chinese).
何卫平,周宜红,赵春菊. 2019. 平面P波入射半无限介质空间域划分及运动偏振特征[J]. 岩石力学与工程学报,38(2):321–331. He W P,Zhou Y H,Zhao C J. 2019. Spatial division of semi-infinite space and polarization characteristics of particle motion for incident plane primary wave[J]. Chinese Journal of Rock Mechanics and Engineering,38(2):321–331 (in Chinese).
金星. 1993. 地震动场研究状况简介[J]. 世界地震工程,(3):19–22,52. Jin X. 1993. Introduction of research on earthquake motion field[J]. World Earthquake Engineering,(3):19–22,52 (in Chinese).
金星,廖振鹏. 1994. 地震动随机场的物理模拟[J]. 地震工程与工程振动,14(3):11–19. Jin X,Liao Z P. 1994. Physical simulation on the random field of seismic motion[J]. Earthquake Engineering and Engineering Vibration,14(3):11–19 (in Chinese).
林皋. 2017. 地下结构地震响应的计算模型[J]. 力学学报,49(3):528–542. Lin G. 2017. A computational model for seismic response analysis of underground structures[J]. Chinese Journal of Theoretical and Applied Mechanics,49(3):528–542 (in Chinese).
缪惠全,李杰. 2018. 基于物理机制的工程场地地震动相干函数模型[J]. 中国科学:技术科学,48(2):209–216. Miao H Q,Li J. 2018. The coherence function model of ground motion in engineering sites based on physical mechanism[J]. Scientia Sinica:Technologica,48(2):209–216 (in Chinese).
张楚汉,金峰,王进廷,徐艳杰,潘坚文. 2016. 高混凝土坝抗震安全评价的关键问题与研究进展[J]. 水利学报,47(3):253–264. Zhang C H,Jin F,Wang J T,Xu Y J,Pan J W. 2016. Key issues and developments on seismic safety evaluation of high concrete dams[J]. Journal of Hydraulic Engineering,47(3):253–264 (in Chinese).
张翠然,陈厚群,李敏. 2011. 采用随机有限断层法生成最大可信地震[J]. 水利学报,42(6):721–728. Zhang C R,Chen H Q,Li M. 2011. Generation of the maximum credible earthquake by using the stochastic finite fault method[J]. Journal of Hydraulic Engineering,42(6):721–728 (in Chinese).
钟万勰,林家浩,吴志刚,孙东科,张亚辉. 2000. 大跨度桥梁分析方法的一些进展[J]. 大连理工大学学报,40(2):127–135. doi: 10.3321/j.issn:1000-8608.2000.02.001 Zhong W X,Lin J H,Wu Z G,Sun D K,Zhang Y H. 2000. Development of analytical method for long-span bridges[J]. Journal of Dalian University of Technology,40(2):127–135 (in Chinese).
Hao H,Oliveira C S,Penzien J. 1989. Multiple-station ground motion processing and simulation based on SMART-1 array data[J]. Nucl Eng Des,111(3):293–310. doi: 10.1016/0029-5493(89)90241-0
Loh C H,Yeh Y T. 1988. Spatial variation and stochastic modelling of seismic differential ground movement[J]. Earthq Eng Struct Dyn,16(4):583–596. doi: 10.1002/eqe.4290160409
Luco J E,Wong H L. 1986. Response of a rigid foundation to a spatially random ground motion[J]. Earthq Eng Struct Dyn,14(6):891–908. doi: 10.1002/eqe.4290140606
Shearer P M. 2009. Introduction to Seismology[M]. 2nd ed. Cambridge: Cambridge University Press: 215−240.
Zerva A. 2009. Spatial Variation of Seismic Ground Motions: Modeling and Engineering Applications[M]. Boca Raton: CRC Press: 1–64.
Zerva A,Harada T. 1997. Effect of surface layer stochasticity on seismic ground motion coherence and strain estimates[J]. Soil Dyn Earthq Eng,16(7/8):445–457.
-
期刊类型引用(2)
1. 何卫平，李小军，杜修力，姚惠芹. P波入射分界面叠加区质点运动形成机制与峰值规律. 振动与冲击. 2023(18): 81-87+163 . 
百度学术
2. 王婷，延军平，李双双，万佳，张玉凤. 帕米尔高原Mw≥6.6级地震时间韵律特征. 高原地震. 2020(04): 6-16 . 百度学术
其他类型引用(1)
计量
- 文章访问数: 1547
- HTML全文浏览量: 952
- PDF下载量: 60
- 被引次数: 3
