Numerical parametric study of seismic dynamic response and amplification effects of slope topography
-
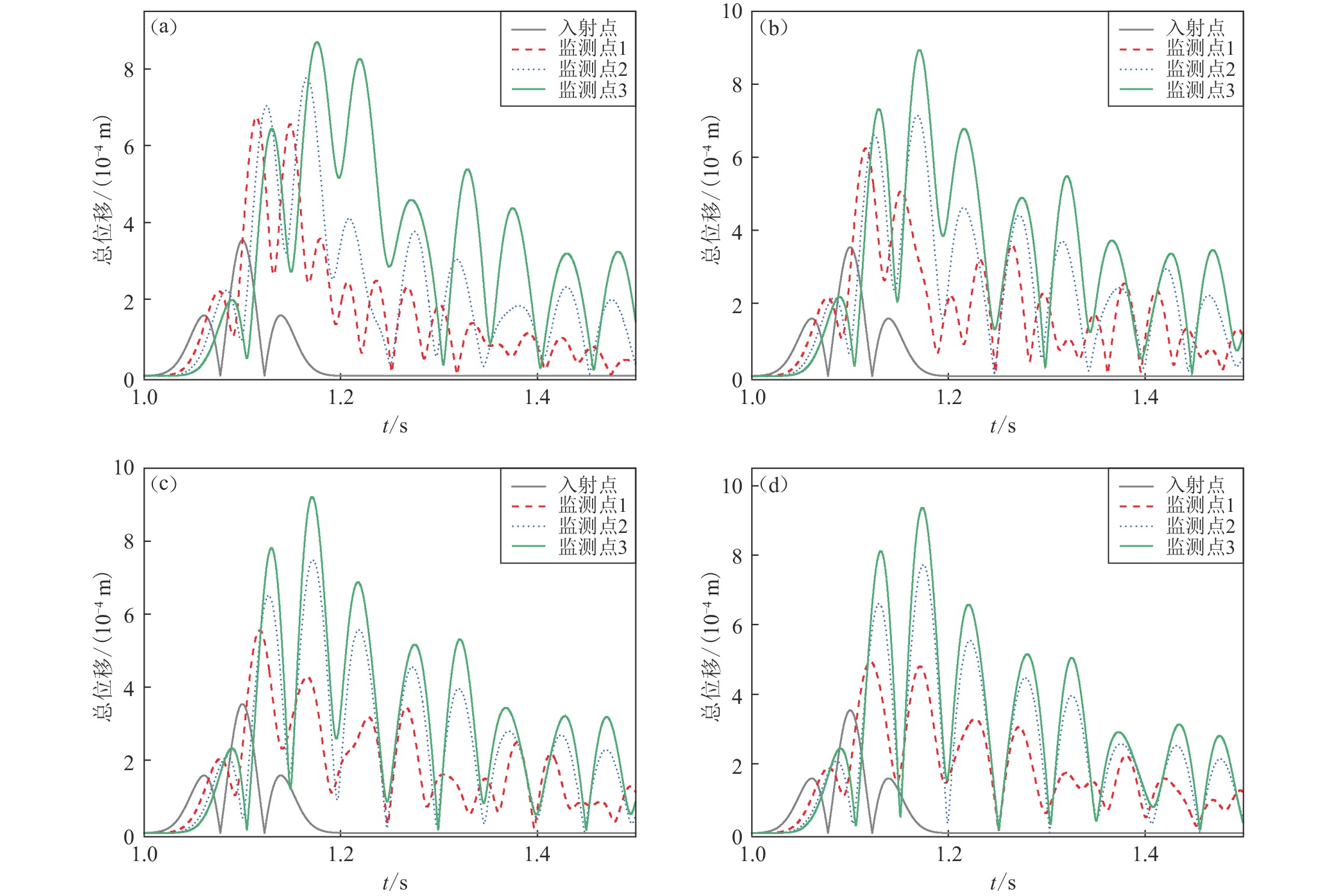
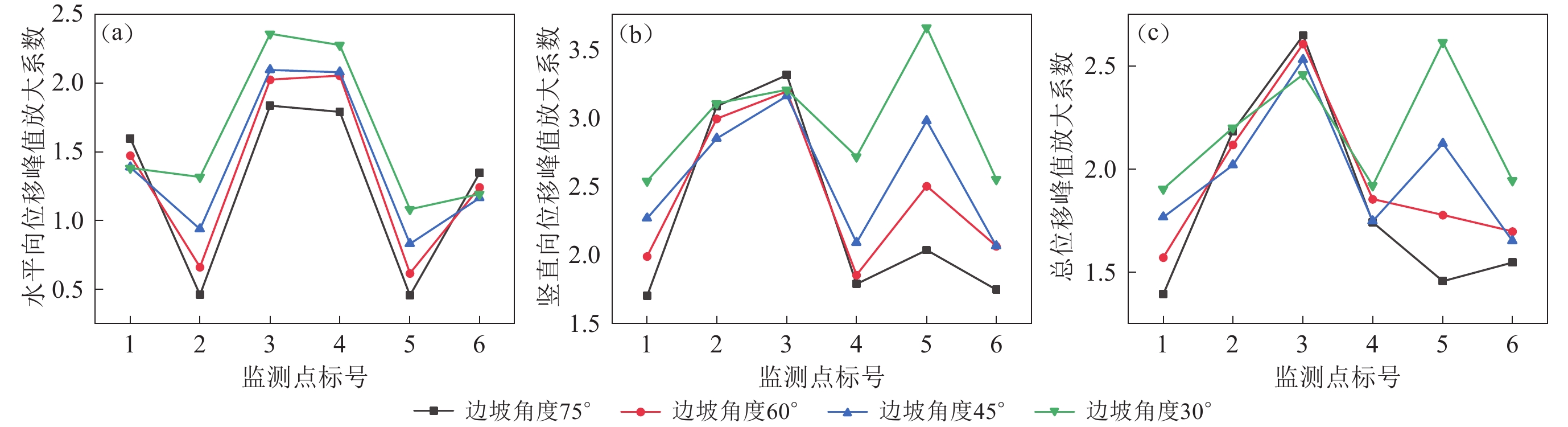
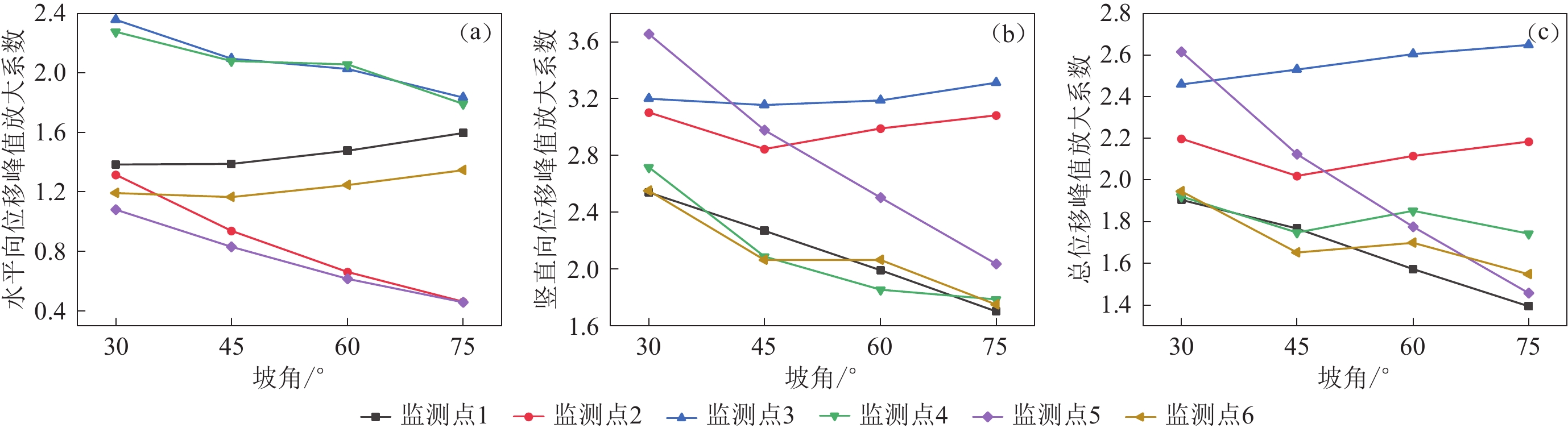
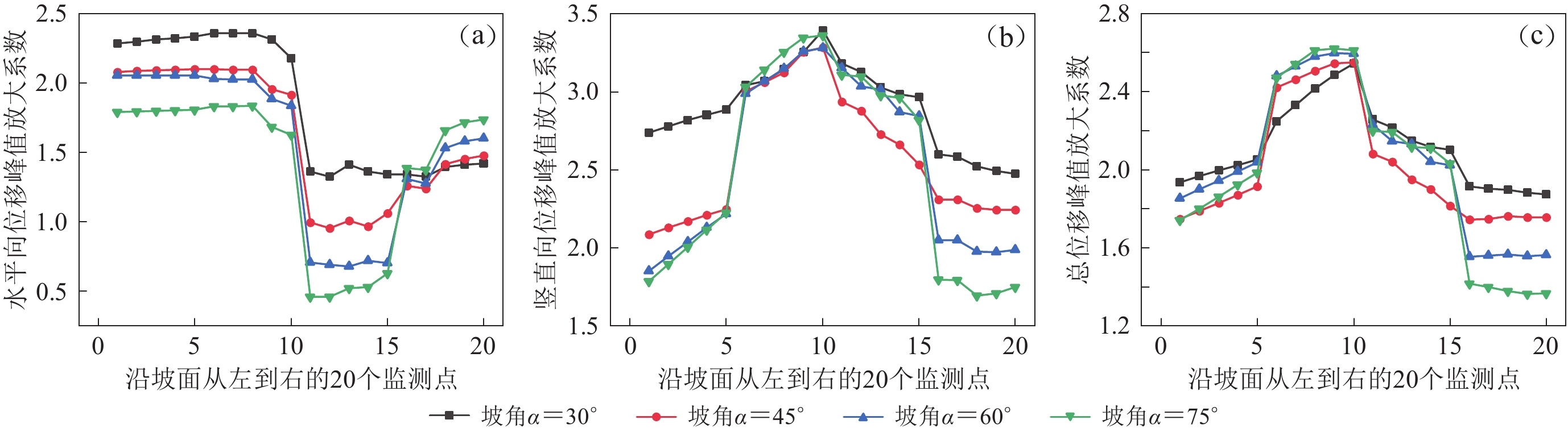
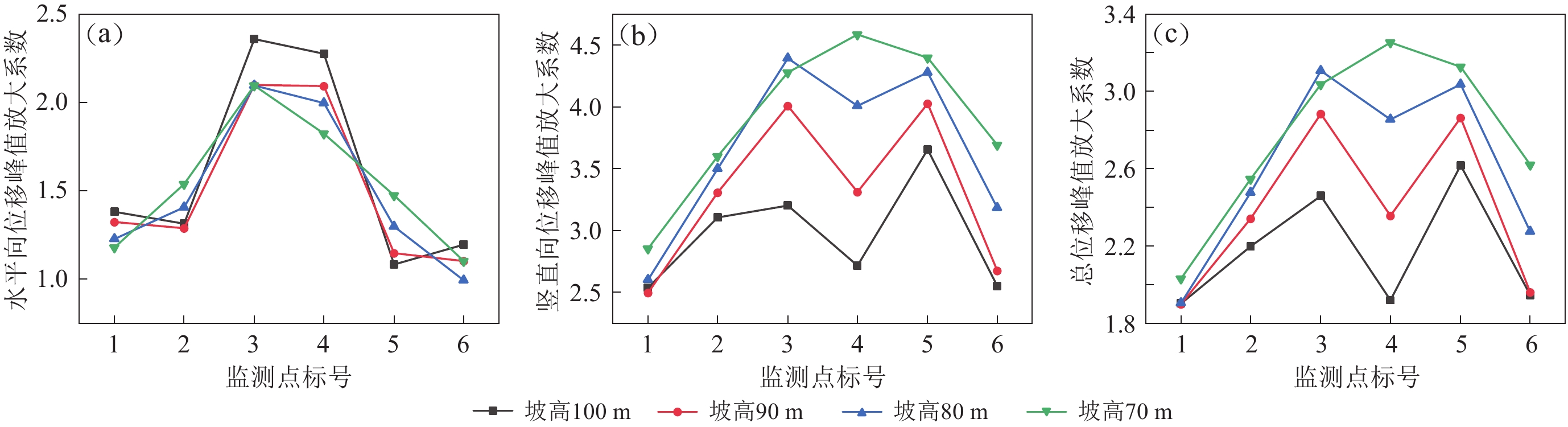
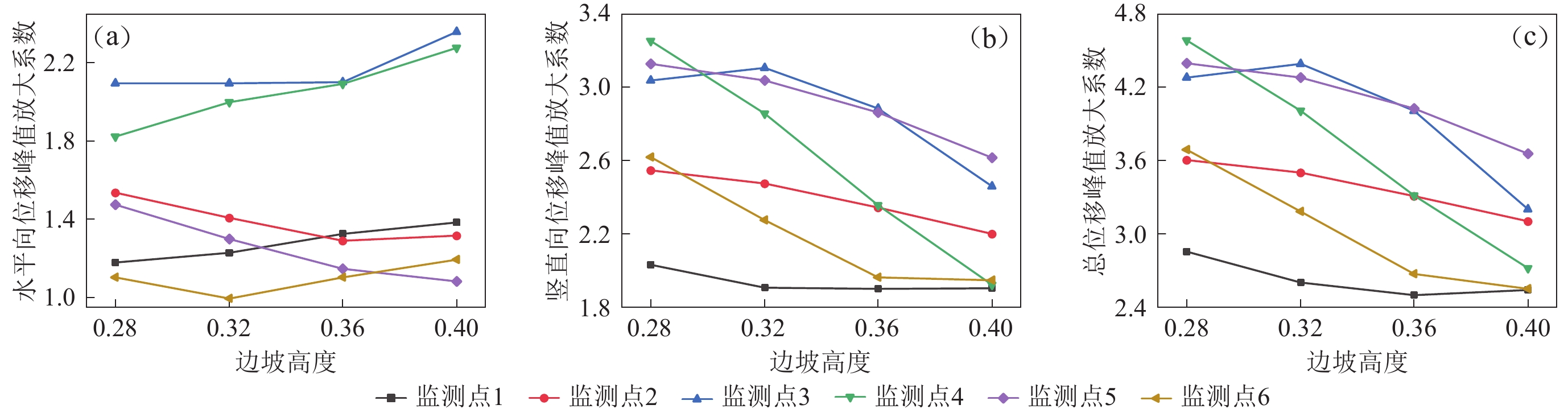
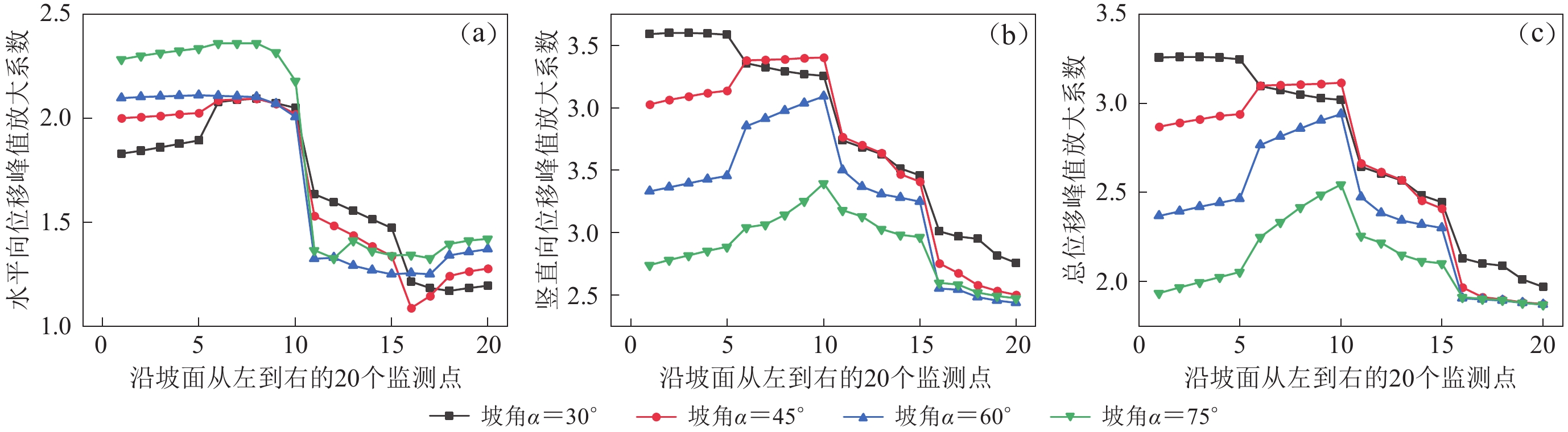
摘要: 本文采用隐式动力有限单元法研究了不同的边坡角度和边坡高度对地形放大效应的影响，并以位移峰值放大系数为衡量地震动放大效应的标准，计算了不同边坡角度和边坡高度条件下的地震响应，在此基础上对模型关键监测点的输出波形以及位移峰值放大系数的变化趋势进行了分析，获得了不同监测点处的地震动时程曲线，揭示了坡角和坡高对单体边坡地震动放大效应的定量作用规律。数值结果表明，相同高度处坡面监测点的水平向位移峰值放大系数大于坡内监测点的，地形放大效应在水平方向具有趋表效应。由于坡面存在入射波和反射波的叠加，因此竖直向位移峰值放大系数的最大值出现在坡体内部。Abstract: In this paper, the implicit dynamic finite element method was applied to analyze the seismic dynamic response and amplification effects of slope topography with different slope angles and heights. With the peak displacement amplification factor （PDA） as the measurement of the amplification effects of seismic waves, the seismic dynamic response based on the PDA under the different conditions was investigated. And then the topographical amplification effects of seismic waves were investigated by analyzing the output waveforms of key monitoring points in the numerical model as well as the variation trend of PDA. Consequently, the time history curves of ground motion at the different monitoring points were obtained. The peak displacement amplification effects induced by different slope angles and heights were quantitatively analyzed and discussed. The numerical results showed that, when the monitoring points were at the same height, the PDAs at the slope surface were larger than those inside the slope. The PDA had the surface effect in horizontal direction. Namely, the more close to the surface was, the larger PDA was. Due to the superposition of the incident seismic waves and the reflected waves induced by the slope surface, the maximum amplification factor of vertical peak displacement appeared inside the slope. The numerical results can provide some guidance in predicting and evaluating the vibration intensity and disaster degree of slopes to some extent.
-
引言
由于城市交通的立体化发展，地下轨道交通和城市立交桥交叉修建。地下结构和邻近地面桥梁相互作用，在地震过程中，高烈度地区的地下和地面结构之间存在复杂的动力相互作用，这种地下和地面结构的耦联破坏作用及其破坏机理已成为城市岩土地震工程及防震减灾领域重要的研究课题之一。
针对地下结构与地面结构的地震相互作用已有相关研究。杨书燕等（2007）对矩形隧道下穿地表框架结构和紧邻地表框架结构两个二维有限元模型进行了数值模拟，结果表明：地表建筑紧邻地下结构时地震响应最大；傅玉勇等（2009）对并行圆形隧道与地表框架结构动力相互作用体系进行了二维有限元数值模拟表明：输入水平向地震动时，地下隧道会使邻近地表建筑顶层水平位移放大30%，但输入竖向地震动时影响较小；何伟和陈健云（2012）通过采用二维有限元数值模拟方法研究了不同场地条件下矩形地下结构对地表建筑地震响应的影响，认为地下结构会使得上部区域的地震动减小而使远处区域增大，并且场地土体刚度越小地下结构对地表建筑地震响应的影响越大；郭靖等将地表建筑简化为弯剪梁模型并采用层间位移谱分析方法计算地表建筑的地震响应，分别采用二维有限元数值模型计算矩形地下结构和圆形隧道存在时的地面运动，并以此作为地表建筑弯剪梁模型底部的地震激励分析了矩形地下结构对地表建筑地震响应的影响，其结果表明，地下结构对地表建筑地震响应的影响与地表建筑的自振周期相关（郭靖，2013；郭靖，陈健云，2013；郭靖等，2017）；Wang等（2013）通过对ANSYS二次开发使其能在频域内求解，利用该方法对地下结构与地表建筑的三维有限元模型进行了求解，分析出矩形地下结构对邻近低矮结构地震响应的影响更加明显。
上述研究采用的数值模型大多未考虑或仅考虑了部分非线性特征，难以给出实际的结构地震破坏过程，因此尚无法合理给出地下结构与地面结构耦联灾变机理。近些年来，由于数值计算技术的发展，杜修力等（2017，2018）首次利用三维动力有限元数值模型模拟了大开车站塌毁过程。董瑞（2020）采用非线性数值模型精细地研究了矩形地下结构的地震灾变过程，得到了矩形地下结构的渐进式破坏模式，并指出结构形式（顶板和侧墙的线刚度比）对其地震响应有较大的影响。Xu等（2020）模拟了地下框架结构的破坏过程，认为中柱由于受到高轴压作用而发生完全脆性破坏。
精细的非线性有限元数值模型可以实现对结构地震破坏过程的高度仿真，但针对地下结构与地面结构的耦联灾变过程的模拟鲜有研究。为了研究地铁车站与横跨桥梁耦联破坏机理，本文拟采用非线性动力时程数值模拟方法，对软土场地的地铁车站与上部交叉桥梁模型进行数值模拟分析。模拟出一种潜在的地铁车站−桥梁耦联灾变模式；并对比弹性工况和弹塑性工况下单一车站模型和车站−桥梁模型的模拟结果，以期分析出地铁车站−桥梁耦联灾变机理，为地下结构与地面结构的选址及抗震设计提供科学依据。
1. 计算模型及模拟工况
1.1 计算模型
本文以两层三跨形式地铁车站及其上部横跨的高架桥结构-土-结构相互作用体系为研究对象，其横断面尺寸如图1所示。地铁车站的横断面（宽×高）为21.00 m×12.5 m；侧墙厚度为0.70 m （纵筋配筋率为0.8%），顶板厚度为0.70 m （纵筋配筋率为1.0%），底板厚度为0.8 m （纵筋配筋率为1.0%），中柱直径为0.8 m，中心距为5.0 m，（纵筋配筋率为6.0%，箍筋为直径9 mm；钢筋间距为350 mm）。上部横跨桥梁的桥面宽度为16 m；桥柱高度为8 m，截面尺寸为0.8 m×0.6 m；基础形式为桩基础，每个桥柱下面一根直径为0.6 m的混凝土圆形桩，桩长为11.5 m，承台厚度为0.8 m。
![]()
图 1 两层三跨地铁车站（a）及高架桥（b）示意图Figure 1. Diagram of two-story and three-span subway station （a） and viaduct （b）根据广州某工程场地勘探资料选取场地土层，土层参数列于表1。土层从上至下分别为素填土层、粉质黏土层、粉细砂层、粉质黏性土层、全风化混合花岗岩层和中风化混合花岗岩层，厚度分别为4.4，3.5，3.4，8.7，8.1和5.9 m。地铁车站与桥梁相互作用模型剖面如图2所示。车站埋深为5.1 m，邻近车站的桩基础距离车站侧边净距为1.2 m。
表 1 场地土层参数Table 1. Soil parameters of site土层序号 土层类别 密度/（103kg·m−3） 剪切波速/（m·s−1） 弹性模量/kPa 泊松比 黏聚力/kPa 摩擦角/° 厚度/m 1 素填土 1.80 24.39 3000 0.4 10 10 4.4 2 粉质黏土 1.87 27.59 3700 0.3 18 14 3.5 3 粉细砂 1.85 32.12 5000 0.31 0 28 3.4 4 粉质黏性土 1.91 34.37 6000 0.33 25 21 8.7 5 全风化混合花岗岩 1.93 38.37 7500 0.32 30 23 8.1 6 中风化混合花岗岩 2.50 742.42 3500000 0.27 300 35 5.9 在Abaqus中建立两层三跨车站与桥梁交叉的有限元模型，如图3所示。土体和混凝土均采用8结点6面体缩减积分单元进行离散；钢筋采用2结点杆单元进行离散；采用Abaqus中的嵌固模型模拟钢筋和混凝土间的协同工作。根据廖振鹏（2002）给出的离散网格中的波传播条件，选择土体单元尺寸（长×宽×深）为2 m×2 m×2 m，并在地下结构附近进行适当加密处理。
根据董瑞（2020）研究结果，选取模拟人工边界条件为：底部采用黏性边界，侧向边界采用自由度绑定边界（tied degrees of freedom，缩写为TDOF）（Doltsinis，1989），即分别在x和y向两组侧边界处设置位移约束条件使对应节点的位移协调；为保证计算精度，车站-桥梁模型土体计算范围为147 m×30 m×34 m。在Abaqus/Explicit模块中，黏性边界采用无限元（CIN3D8），绑定边界采用多点约束（multi-point constraints，缩写为MPC）方式实现。混凝土结构和周围土体间的摩擦滑移通过Abaqus中的接触面模型模拟，法向采用“硬接触”，切向采用摩擦接触（
$\; \mu {\text{＝}}0.4 $ ）。1.2 本构模型
土体本构模型采用基于Montáns （2000）模型提出的改进边界面本构模型（Dong et al，2020），这一模型已经被二次开发并可以用于通用有限元软件Abaqus。该模型在归一化偏平面内建立屈服函数；并采用土体材料模型与围压的经验公式描述围压改变引起土体模量变化；根据偏应力不变量建立应力等效关系，将一维滞回本构（Pyke）模型拓展到三维应力空间并推导塑性模量。归一化偏平面如图4所示，边界面（即Mises强度面）在归一化偏平面中的投影为一个圆，其方程可以表示为：
![]()
图 4 归一化偏平面内边界面及投影法则Figure 4. Bounding surface and mapping law in normalized deviatoric plane$$ F{\text{＝}}\frac{3}{2}{\boldsymbol{m}}_{{\rm{p}}{\text{，}}ij}{\boldsymbol{m}}_{{\rm{p}}{\text{，}}ij}{\text{－}}{m}_{\mathrm{c}}^{2}{\text{＝}}0 {\text{，}} $$ (1) 式中，
$ {\boldsymbol{m}}_{{\rm{p}}{\text{，}}ij} $ 表示投影点在一般应力空间内的i，j两个方向上的应力张量（i，j＝1，2，3表示空间内x，y，z方向），${m}_{\mathrm{c}}{\text{＝}}6\mathrm{s}\mathrm{i}\mathrm{n}\phi /（3{\text{－}}\mathrm{s}\mathrm{i}\mathrm{n}\phi ）$ 为边界面的半径。图4中的mi为与mij对应的主应力空间内的归一化应力张量，其中${\boldsymbol{m}}_{i}{\text{＝}}{\boldsymbol{s}}_{i}/\bar{{p}}$ ，$\bar{{p}}{\text{＝}}{p}{\text{＋}}c/\mathrm{t}\mathrm{a}\mathrm{n}\phi$ 为等效围压，p为围压，$ c $ 为黏聚力，$ \phi $ 为内摩擦角。基于第二偏应力不变量的应力状态等效关系如图5所示。根据应力状态参量
$ \eta $ 可将一维滞回本构模型拓展到三维应力空间。增量形式的一维滞回本构模型可以表示为![]()
图 5 应力等效关系。图中q为偏应力，$\bar {q}$ 为等效偏应力Figure 5. Equivalent relation of stress。 q is deviatoric stress， and$\bar {q}$ is equivalent deviatoric stress$$ {\rm{d}}\tau {\text{＝}}G{\Bigg(1{\text{－}}\frac{{\tau }_{{\rm{r}}}{\text{－}}\tau }{{\tau }_{{\rm{p}}}{\text{＋}}{\tau }_{{\rm{r}}}}\Bigg)}^{2}{\rm{d}}\gamma {\text{，}} $$ (2) 式中：
$ \tau $ 表示剪应力，$ {\tau }_{{\rm{p}}} $ 和$ {\tau }_{{\rm{r}}} $ 分别为投影点和反向点的剪应力；$ \mathrm{d}\gamma $ 为工程剪应变增量；初始剪切模量$ G $ 采用经验公式计算$$ G{\text{＝}}k{\mathrm{P}}_{\mathrm{a}}{\left(\frac{p}{{\mathrm{P}}_{\mathrm{a}}}\right)^{n}} {\text{，}} $$ (3) 式中，
$ G $ 表示最大剪切模量，$ {\mathrm{P}}_{{\rm{a}}} $ 表示一个标准大气压强，k和$ n $ 为与土性相关的材料常数。根据塑性模量和偏应力增量可以表示为（Dong et al，2020）：
$$ {K}_{\mathrm{p}}{\text{＝}}12G{（{m}_{{\rm{c}}}{\bar{{p}}}_{{\rm{p}}}）}^{2}\frac{{（1{\text{－}}\eta ）}^{2}}{1{\text{－}}{（1{\text{－}}\eta ）}^{2}} {\text{，}} $$ (4) $$ \mathrm{d}{\boldsymbol{s}}_{{\rm{c}}，ij}{\text{＝}}2G\mathrm{d}{\boldsymbol{e}}_{ij}{\text{－}}\frac{3G\left[1{\text{－}}{（1{\text{－}}\eta ）}^{2}\right]}{{m}_{{\rm{c}}}^{2}}（{\boldsymbol{m}}_{{\rm{p}}，{{kl}}}{\rm{d}}{\boldsymbol{e}}_{kl}）{\boldsymbol{m}}_{{\rm{p}}，{{ij}}} {\text{.}} $$ (5) 式中：e表示应变张量；k和l取值为1，2，3，表示空间内x，y，z方向。
地铁车站混凝土材料采用Abaqus材料库中的混凝土塑形损伤（concrete damaged plasticity，缩写为CDP）模型进行模拟，钢筋采用理想弹塑性材料模拟；混凝土强度等级为C30，钢筋强度等级为HRB335。CDP模型的计算参数列于表2，混凝土材料屈服强度及损伤因子随非弹性应变的变化关系如图6所示；假定桥梁已经按照规范进行了完善的抗震设计，因此可以忽略桥梁桩基础、桥柱和梁板的强度破坏，模拟时桥梁采用弹性模型并施加阻尼比为5%的质量相关阻尼模拟材料耗能特性，桥梁材料密度为2 500 kg/m3，弹性模量为30 GPa，泊松比为0.2；桥梁支座和梁板间设置接触模拟支座，法向采用“硬接触”，切向采用摩擦接触，桥梁支座和梁板间的摩擦系数取为0.4。
表 2 CDP模型材料参数Table 2. Parameter of CDP model弹性模量/GPa 泊松比 剪胀角/° 偏心率 双轴与单轴抗压强度比值 屈服面形态的参数K 黏性系数 损伤发展 30 0.2 30 0.1 1.16 0.666 7 0.000 5 见图6 ![]()
图 6 混凝土拉伸（a）和压缩（b）损伤发展Figure 6. Development of tensile damage （a） and compression damage （b） for concrete1.3 模拟工况
选择1995年阪神地震的Kobe波的南北分量和竖直分量（图7）作为输入地震波地震；分别选取有限元模型中x向（地铁车站横断面方向）和z向（竖直方向）作为水平地震动和竖直地震动输入方向，将水平方向分量和竖直方向分量调幅为0.4g和0.3g，以此模拟在强震作用下地铁车站的地震响应。
![]()
图 7 阪神地震中神户海洋气象台记录Figure 7. Ground motion record at the Kobe marine observatory during the Hanshin earthquake针对单一车站模型和车站−桥梁模型，分别进行车站为弹性模型和弹塑性模型情况下的动力时程反应分析，并依据上述两组模型模拟结果对比研究桥梁和地铁车站间的相互作用机理及耦联破坏机制。弹塑性工况P-1模拟了桥梁和地铁车站耦联破坏的震害现象，并与单一车站（P-2）的破坏模式进行对比给出桥梁和地铁车站间的耦联破坏机制。弹性工况E-1和E-2用于分析在未发生破坏的条件下，邻近车站的桥梁基础与车站的相互作用，进一步解释桥梁−车站耦联破坏机制。数值模拟工况如表3所示。
表 3 数值模拟工况Table 3. simulated Working condition工况 计算模型 地铁车站
本构模型输入地震动 工况 计算模型 地铁车站
本构模型输入地震动 水平/g 竖向/g 水平/g 竖向/g P-1 车站−桥梁 CDP 0.4 0.3 E-1 车站−桥梁 黏弹性 0.4 0.3 P-2 单一车站 CDP 0.4 0.3 E-2 单一车站 黏弹性 0.4 0.3 2. 有限元模拟结果分析
2.1 地铁车站−桥梁耦联灾变模拟结果
车站−桥梁模型（P-1工况）4个关键时刻的水平位移与车站混凝土压损伤如图8所示，可以看出：当t＝0—7 s 时，地铁车站中柱混凝土在双向地震动作用下损伤逐渐发育，但地铁车站和桥梁均未发生破坏；当t＝7—8 s 时，地铁车站中柱混凝土损伤进一步发育，下层左侧柱子首先发生屈曲破坏；当t＝8—11 s 时，地铁车站下层左侧中柱失效后，顶板和楼板失去中柱约束发生破坏，顶板和楼板失效后，侧墙无法承受两侧土压力的作用而向车站内侧坍塌；当t＝11—15 s时，地铁车站破坏后，邻近桥梁基础随土层向车站方向产生较大变形，进而产生了“落梁”破坏。
![]()
图 8 车站−桥梁模型4个关键时刻的水平位移及车站混凝土压损伤因子云图Figure 8. Nephogram of horizontal displacement and compression damage of station concrete for station-bridge model at four key moments2.2 地铁车站−桥梁耦联破坏机理
地震荷载作用下地下结构受到周围土体的约束而发生剪切变形，地下结构的层间位移可以反应地下结构受到的地震荷载作用大小。图9给出弹性工况（E-1和E-2）和弹塑性工况（P-1和P-2）在结构破坏前（3—7 s）地铁车站上下两层在左侧、中部和右侧的层间相对位移。单一车站模型和车站−桥梁模型计算得到的车站层间位移基本一致。由此可知，在地铁车站未破坏时，桥梁对车站的地震响应影响很小。
![]()
图 9 地铁车站层间相对位移（a） 弹性工况；（b） 弹塑性工况（3—7 s）Figure 9. Relative displacement of subway station（a） Elastic condition；（b） Elastic-plastic condition （3−7 s）车站−桥梁模型（P-1工况）和单一车站模型（P-2工况）地铁车站破坏后引起邻近区域土层永久变形（变形放大比例为1），如图9所示。图中显示了车站楼板（D＝−11.3 m）位置水平位移等值线，单一车站模型左右两侧水平位移等值线与水平方向夹角分别为38.26°和42.94°，车站−桥梁模型左右两侧水平位移等值线与水平方向夹角分别为42.90°和43.00°。
两模型在地表（D＝0 m）、车站顶板（D＝−5.1 m）和车站楼板（D＝−11.3 m）位置的土层永久变形分布曲线，如图11所示。车站−桥梁模型得到的车站邻近区域土层永久位移大于单一车站模型，桥梁会加剧车站破坏引起的地基变形以及其影响范围。结合图10和图11的结果可知，桥梁不会改变地铁车站的破坏形式；但是由于桥梁的基础对土层存在一定的加固效果使得车站两侧土体整体向车站移动，进而加剧了车站−桥梁系统的震害。
![]()
图 10 车站邻近区域土层永久水平位移云图（a） 车站-桥梁模型 ；（b） 单一车站模型Figure 10. Nephogram of soil permanent horizontal displacement near subway station（a） Station-bridge model；（b） Single station model![]()
图 11 地表、车站顶板和车站楼板位置处的土层永久位移Figure 11. Permanent horizontal displacement of soil at the depths of soil surface，station roof and station floor3. 讨论与结论
本文通过对软土场地的单一两层三跨车站以及其与邻近桥梁耦联相互作用模型进行非线性动力时程反应分析，模拟了一种可能的地铁车站-桥梁模型耦联破坏模式。并通过对比分析桥梁对弹性地铁车站模型（E-1工况和E-2工况）和弹塑性地铁车站模型（P-1工况和P-2工况）地震响应的影响，对地铁车站与桥梁的耦联破坏机制进行了分析，得出如下结论：
1） 依托Abaqus强大的大变形模拟计算能力，并采用本文给出的土体非线性本构模型，可以实现对地下结构与地面结构复杂耦联破坏过程的仿真模拟，地铁车站发生破坏后会引起邻近区域土层发生较大的水平和竖向变形，地铁车站的破坏会引起邻近区域地基失效并使桥梁发生“落梁”破坏。
2） 分别根据弹性模型和弹塑性模型（未破坏阶段）模拟结果，对比单一地铁车站和地铁车站-桥梁模型得到车站响应，可知桥梁对车站的地震响应影响较小，车站发生破坏主要取决于地震作用以及其本身的强度。
3） 地铁车站-桥梁耦联破坏主要表现为地铁车站破坏后桥梁基础失效形式的“次生”灾害， 在车站破坏后，桥梁基础会带动临近区域土层整体向车站方向移动，增大周围土层永久变形的范围，加剧车站坍塌震害。
需要说明的是，对地下结构地震破坏过程的模拟是一个高度非线性的问题，此类问题的非线性主要表现为几何非线性和材料非线性两个方面，选取合适的数值模型描述上述两种非线性是仿真模拟破坏过程的关键。针对几何非线性问题，本文采用的Abaqus能够实现对单元网格大变形的模拟；针对材料非线性问题，本文采用基于边界面理论建立的土体弹塑性本构模型，在该模型中引入了模量随围压变化的经验公式，可以更合理的描述地下结构两侧土体围压改变引起的土体非线性响应。
综上所述，本研究利用了一种可以模拟地下结构和地面结构耦联灾变过程的方法，并通过对地铁车站-桥梁相互作用模型地震灾变过程的仿真模拟结果，得出了地铁车站和邻近桥梁的耦联灾变机理，研究成果对于城市地下结构选址及抗震设计具有一定的参考意义。
-
![]()
图 3 本模型输入的雷克子波的位移（a）和加速度（b）时程曲线
Figure 3. Displacement （a） and acceleration （b） time-history curves of input Ricker wavelet
![]()
图 4 入射点沿x向（a）和y向（b）的入射波形以及总入射波形（c）
Figure 4. Input waveform in x （a） and y （b） directions and total incident waveform （c）
![]()
图 5 不同坡角α条件下关键监测点的总位移输出
Figure 5. Total displacement output of key monitoring points under different slope angles α
（a） α＝30°；（b） α＝45°；（c） α＝60°；（d） α＝75°
![]()
图 6 不同坡角α时各监测点的位移峰值放大系数
（a） 水平向位移峰值放大系数；（b） 竖直向位移峰值放大系数；（c） 总位移峰值放大系数
Figure 6. Peak displacement amplification factor （PDA） of the monitoring points under different slope angle α
（a） Horizontal PDA；（b） Vertical PDA；（c） Total PDA
![]()
图 7 各监测点位移峰值放大系数随坡角α的变化
（a） 水平向位移峰值放大系数；（b） 竖直向位移峰值放大系数；（c） 总位移峰值放大系数
Figure 7. Variation of peak displacement amplification factor of monitoring points with slope angle α
（a） Horizontal PDA；（b） Vertical PDA；（c） Total PDA
![]()
图 8 不同坡角工况下坡表面监测点位移放大系数
（a） 水平向位移峰值放大系数；（b） 竖直向位移峰值放大系数；（c） 总位移峰值放大系数
Figure 8. PDA of observation points under different slope angle α
（a） Horizontal PDA；（b） Vertical PDA；（c） Total PDA
![]()
图 9 不同位置监测点的放大效应规律
（a） 水平向位移峰值放大系数；（b） 竖直向位移峰值放大系数；（c） 总位移峰值放大系数
Figure 9. Amplification effect of the monitoring points at different locations
（a） Horizontal PDA；（b） Vertical PDA；（c） Total PDA
![]()
图 10 边坡高度对各监测点放大效应的影响
（a） 水平向位移峰值放大系数；（b） 竖直向位移峰值放大系数；（c） 总位移峰值放大系数
Figure 10. Amplification effect of the monitoring points under different slope height
（a） Horizontal PDA；（b） Vertical PDA；（c） Total PDA
![]()
图 11 不同坡高工况下坡表面监测点的位移峰值放大系数
（a） 水平向位移峰值放大系数；（b） 竖直向位移峰值放大系数；（c） 总位移峰值放大系数
Figure 11. PDA of the observation points under different slope height
（a） Horizontal PDA；（b） Vertical PDA；（c） Total PDA
表 1 本文模型的材料参数
Table 1 Material parameters of the model in this study
材料 密度/（kg·m−3） 弹性模量/GPa 泊松比 岩体 2 400 50 0.25 节理 2 320 5 0.30 
下载: 导出CSV
-
陈宝魁,王东升,成虎. 2016. 粘弹性人工边界在地震工程中应用研究综述[J]. 地震研究,<bold>39</bold>(1):137–142. doi: 10.3969/j.issn.1000-0666.2016.01.023 Chen B K,Wang D S,Cheng H. 2016. Research review on the application of viscous-spring artificial boundary in earthquake engineering[J]. <italic>Journal of Seismological Research</italic>,<bold>39</bold>(1):137–142 (in Chinese).
戴嘉伟. 2016. 利用地脉动和强震动观测数据分析汶川地震的场地效应[D]. 哈尔滨: 中国地震局工程力学研究所: 15−22. Dai J W. 2016. Site Effects Analysis of Wenchuan Earthquake by Using Microtremor and Strong Motion Observation Data[D]. Harbin: Institute of Engineering Mechanics, China Earthquake Administration: 15−22 （in Chinese）.
丁梓涵,赵其华,彭社琴,陈继彬,喻豪俊. 2015. 岩层倾角对顺倾向边坡地震效应的影响[J]. 地震工程学报,<bold>37</bold>(4):956–962. doi: 10.3969/j.issn.1000-0844.2015.04.0956 Ding Z H,Zhao Q H,Peng S Q,Chen J B,Yu H J. 2015. Impact of dip angle of rock stratum on seismic response of consequent slope[J]. <italic>China Earthquake Engineering Journal</italic>,<bold>37</bold>(4):956–962 (in Chinese).
冯永,谢飞亚,李旭光. 2017. 地震荷载下边坡抗滑桩桩土机理的三维模拟分析[J]. 地震工程学报,<bold>39</bold>(1):32–38. doi: 10.3969/j.issn.1000-0844.2017.01.0032 Feng Y,Xie F Y,Li X G. 2017. Three dimensional simulation of pile-soil interaction mechanism of anti-slide piles under seismic loads[J]. <italic>China Earthquake Engineering Journal</italic>,<bold>39</bold>(1):32–38 (in Chinese).
高玉峰. 2019. 河谷场地地震波传播解析模型及放大效应[J]. 岩土工程学报,<bold>41</bold>(1):1–25. Gao Y F. 2019. Analytical models and amplification effects of seismic wave propagation in canyon sites[J]. <italic>Chinese Journal of Geotechnical Engineering</italic>,<bold>41</bold>(1):1–25 (in Chinese).
蒋锋云,朱良玉,李玉江. 2018. 基于三维粘弹性有限元研究汶川地震对川滇地区的震后影响[J]. 地震研究,<bold>41</bold>(2):233–243. doi: 10.3969/j.issn.1000-0666.2018.02.011 Jiang F Y,Zhu L Y,Li Y J. 2018. Study of the co-seismic and post-seismic deformation deduced by the Wenchuan earthquake in the Sichuan-Yunnan region by three dimensional viscoelastic finite element model[J]. <italic>Journal of Seismological Research</italic>,<bold>41</bold>(2):233–243 (in Chinese).
李白基,秦嘉政,罗娣华,叶建庆,陈敏恭,蔡绍平,刘学军. 1999. 云南丽江峡谷的场地放大[J]. 地震学报,<bold>21</bold>(2):175–179. doi: 10.3321/j.issn:0253-3782.1999.02.008 Li B J,Qin J Z,Luo D H,Ye J Q,Chen M G,Cai S P,Liu X J. 1999. Site amplification in the valley of Lijiang,Yunnan[J]. <italic>Acta Seismologica Sinica</italic>,<bold>21</bold>(2):175–179 (in Chinese).
李天斌. 2008. 汶川特大地震中山岭隧道变形破坏特征及影响因素分析[J]. 工程地质学报,<bold>16</bold>(6):742–750. doi: 10.3969/j.issn.1004-9665.2008.06.003 Li T B. 2008. Failure characteristics and influence factor analysis of mountain tunnels at epicenter zones of great Wenchuan earthquake[J]. <italic>Journal of Engineering Geology</italic>,<bold>16</bold>(6):742–750 (in Chinese).
梁建文,陈健琦,巴振宁. 2012. 弹性层状半空间中无限长洞室对斜入射平面SH波的三维散射( Ⅰ ):方法及验证[J]. 地震学报,<bold>34</bold>(6):785–792. doi: 10.3969/j.issn.0253-3782.2012.06.005 Liang J W,Chen J Q,Ba Z N. 2012. 3D scattering of obliquely incident SH waves by a cylindrical cavity in layered elastic half-space ( Ⅰ ):Methodology and verification[J]. <italic>Acta Seismologica Sinica</italic>,<bold>34</bold>(6):785–792 (in Chinese).
刘汉龙,费康,高玉峰. 2003. 边坡地震稳定性时程分析方法[J]. 岩土力学,<bold>24</bold>(4):553–556. doi: 10.3969/j.issn.1000-7598.2003.04.013 Liu H L,Fei K,Gao Y F. 2003. Time history analysis method of slope seismic stability[J]. <italic>Rock and Soil Mechanics</italic>,<bold>24</bold>(4):553–556 (in Chinese).
刘晶波,谷音,杜义欣. 2006. 一致粘弹性人工边界及粘弹性边界单元[J]. 岩土工程学报,<bold>28</bold>(9):1070–1075. doi: 10.3321/j.issn:1000-4548.2006.09.004 Liu J B,Gu Y,Du Y X. 2006. Consistent viscous-spring artificial boundaries and viscous-spring boundary elements[J]. <italic>Chinese Journal of Geotechnical Engineering</italic>,<bold>28</bold>(9):1070–1075 (in Chinese).
刘新荣,邓志云,刘永权,刘树林,路雨明. 2019. 地震作用下水平层状岩质边坡累积损伤与破坏模式研究[J]. 岩土力学,<bold>40</bold>(7):2507–2516. Liu X R,Deng Z Y,Liu Y Q,Liu S L,Lu Y M. 2019. Study of cumulative damage and failure mode of horizontal layered rock slope subjected to seismic loads[J]. <italic>Rock and Soil Mechanics</italic>,<bold>40</bold>(7):2507–2516 (in Chinese).
孙强强,薄景山,刘红帅,景立平. 2019. 隧道对地表地震动放大作用的研究[J]. 现代隧道技术,<bold>56</bold>(3):65–71. Sun Q Q,Bao J S,Liu H S,Jing L P. 2019. Effects of a tunnel on ground motion amplification[J]. <italic>Modern Tunnelling Technology</italic>,<bold>56</bold>(3):65–71 (in Chinese).
王猛,梁庆国,王丽丽,边磊. 2019. 黄土隧道边仰坡动力响应的大型振动台模型试验研究[J]. 地震工程与工程振动,<bold>39</bold>(2):141–150. Wang M,Liang Q G,Wang L L,Bian L. 2019. Large-scale shaking table model test study on dynamic response of loess tunnel side slope[J]. <italic>Earthquake Engineering and Engineering Dynamics</italic>,<bold>39</bold>(2):141–150 (in Chinese).
詹志发,祁生文,何乃武,郑博文,葛传峰. 2019. 强震作用下均质岩质边坡动力响应的振动台模型试验研究[J]. 工程地质学报,<bold>27</bold>(5):946–954. Zhan Z F,Qi S W,He N W,Zheng B W,Ge C F. 2019. Shaking table test study of homogeneous rock slope model under strong earthquake[J]. <italic>Journal of Engineering Geology</italic>,<bold>27</bold>(5):946–954 (in Chinese).
张伯艳,李德玉. 2014. 白鹤滩水电站左岸边坡抗震分析[J]. 工程力学,<bold>31</bold>(增刊1):149–154. Zhang B Y,Li D Y. 2014. Dynamic stability analyses of Bai He Tan hydropower-station left slope[J]. <italic>Engineering Mechanics</italic>,<bold>31</bold>(S1):149–154 (in Chinese).
张泽林,吴树仁,王涛,唐辉明,梁昌玉. 2018. 地震波振幅对黄土-泥岩边坡动力响应规律的影响[J]. 岩土力学,<bold>39</bold>(7):2403–2412. Zhang Z L,Wu S R,Wang T,Tang H M,Liang C Y. 2018. Influence of seismic wave amplitude on dynamic response of loess-mudstone slope[J]. <italic>Rock and Soil Mechanics</italic>,<bold>39</bold>(7):2403–2412 (in Chinese).
郑颖人,叶海林,黄润秋. 2009. 地震边坡破坏机制及其破裂面的分析探讨[J]. 岩石力学与工程学报,<bold>28</bold>(8):1714–1723. doi: 10.3321/j.issn:1000-6915.2009.08.024 Zheng Y R,Ye H L,Huang R Q. 2009. Analysis and discussion of failure mechanism and fracture surface of slope under earthquake[J]. <italic>Chinese Journal of Rock Mechanics and Engineering</italic>,<bold>28</bold>(8):1714–1723 (in Chinese).
Boore D M. 1972. A note on the effect of simple topography on seismic SH waves[J]. <italic>Bull Seismol Soc Am</italic>,<bold>62</bold>(1):275–284.
Bouchon M. 1973. Effect of topography on surface motion[J]. <italic>Bull Seismol Soc Am</italic>,<bold>63</bold>(3):615–632.
Çelebi M. 1987. Topographical and geological amplifications determined from strong-motion and aftershock records of the 3 March 1985 Chile earthquake[J]. <italic>Bull Seismol Soc Am</italic>,<bold>77</bold>(4):1147–1167.
Çelebi M. 1991. Topographical and geological amplification:Case studies and engineering implications[J]. <italic>Struct Saf</italic>,<bold>10</bold>(1/3):199–217.
Chang K H,Tsaur D H,Wang J H. 2015. Response of a shallow asymmetric V-shaped canyon to antiplane elastic waves[J]. <italic>Proc Roy Soc A</italic>:<italic>Math Phy Eng Sci</italic>,<bold>471</bold>(2174):20140215. doi: 10.1098/rspa.2014.0215
Erzin Y,Cetin T. 2012. The use of neural networks for the prediction of the critical factor of safety of an artificial slope subjected to earthquake forces[J]. <italic>Sci Iran</italic>,<bold>19</bold>(2):188–194. doi: 10.1016/j.scient.2012.02.008
Fukushima T, Matsushita K, Usami H, Wakai A. 2013. Finite element simulation for an earthquake-induced landslide considering strain-softening characteristics of sensitive clayey loam[G]//Earthquake-Induced Landslides. Berlin Heidelberg: Springer: 671–678.
Lin M L, Huang C Y. 2017. Numerical simulation of run-out behavior of earthquake-induced landslides[G]//Geotechnical Hazards From Large Earthquakes and Heavy Rainfalls. Tokyo, Japan: Springer: 193–202.
Massa M,Ferretti G,Cevasco A,Isella L,Eva C. 2004. Analysis of site amplification phenomena:An application in Ripabottoni for the 2002 Molise,Italy,earthquake[J]. <italic>Earthq Spectra</italic>,<bold>20</bold>(S1):S107–S118.
Poursartip B,Fathi A,Kallivokas L F. 2017. Seismic wave amplification by topographic features:A parametric study[J]. <italic>Soil Dyn Earthq Eng</italic>,<bold>92</bold>:503–527. doi: 10.1016/j.soildyn.2016.10.031
Sánchez-Sesma F J,Rosenblueth E. 1979. Ground motion at canyons of arbitrary shape under incident SH waves[J]. <italic>Earthq Eng Struct Dyn</italic>,<bold>7</bold>(5):441–450. doi: 10.1002/eqe.4290070505
Sánchez-Sesma F J,Herrera I,Avilés J. 1982. A boundary method for elastic wave diffraction:Application to scattering of SH waves by surface irregularities[J]. <italic>Bull Seismol Soc Am</italic>,<bold>72</bold>(2):473–490.
Sánchez-Sesma F J. 1985. Diffraction of elastic SH waves by wedges[J]. <italic>Bull Seismol Soc Am</italic>,<bold>75</bold>(5):1435–1446.
Sills L B. 1978. Scattering of horizontally-polarized shear waves by surface irregularities[J]. <italic>Geophys J R astr Soc</italic>,<bold>54</bold>(2):319–348. doi: 10.1111/j.1365-246X.1978.tb04263.x
Smith W D. 1975. The application of finite element analysis to body wave propagation problems[J]. <italic>Geophys J Int</italic>,<bold>42</bold>(2):747–768.
Trifunac M D. 2010. Scattering of plane SH waves by a semi-cylindrical canyon[J]. <italic>Earthq Eng Struct Dyn</italic>,<bold>1</bold>(3):267–281.
Wang G,Du C Y,Huang D R,Jin F,Koo R C H,Kwan J S H. 2018. Parametric models for 3D topographic amplification of ground motions considering subsurface soils[J]. <italic>Soil Dyn Earthq Eng</italic>,<bold>115</bold>:41–54. doi: 10.1016/j.soildyn.2018.07.018
Wong H L,Jennings P C. 1975. Effects of canyon topography on strong ground motion[J]. <italic>Bull Seismol Soc Am</italic>,<bold>65</bold>(5):1239–1257.
Zhang W,Zhang Z G,Chen X F. 2012. Three-dimensional elastic wave numerical modelling in the presence of surface topography by a collocated-grid finite-difference method on curvilinear grids[J]. <italic>Geophys J Int</italic>,<bold>190</bold>(1):358–378. doi: 10.1111/j.1365-246X.2012.05472.x
计量
- 文章访问数: 886
- HTML全文浏览量: 406
- PDF下载量: 63
