Improved half-wavelength method for determining the velocity structure of site soil layer
-
摘要: 地球物理反演浅地表土层波速剖面通常基于初始速度结构模型，为避免对地勘资料产生较多依赖，充分利用地震和背景噪声等被动源观测记录，快速简便地构建出土层反演的初始模型，本文基于场地土层的频散曲线和水平与竖向谱比提出了一种改进半波长法。该方法首先通过水平与竖向谱比确定场地土层的卓越频率，获取场地覆盖层厚度，确定反演所需的频带范围；其次，利用瑞雷波相速度对剪切波速的偏导数随土层深度的变化规律反演出场地的初始速度结构，并结合工程场地勘探中的半波长法，对常见的三类典型土层模型和日本Kushiro场地实测模型进行了实例分析；最后，将反演得到的速度结构与理论或实测速度结构进行误差对比分析。结果表明：改进半波长法获得的初始速度结构相对于理论或实测速度结构的最大误差不超过35%，可为利用地球物理方法反演工程场地波速剖面构造一个较小的搜索模型空间，进而提高反演计算的速度和结果的可靠性。Abstract: The initial velocity structure model is generally necessary in the study of geophysical inversion for shallow surface soil wave velocity profile. In order to avoid excessive reliance on the surveying data instead, and to make full use of observation records of passive sources such as earthquakes and ambient noise to quickly and easily construct the initial model of soil inversion, this paper proposes an improved half-wavelength method based on the dispersion curve and the horizontal-to-vertical spectral ratio （HVSR） of the soil layer. Firstly, the method deter-mines the predominant frequency of the soil layer by HVSR, and then obtains the depth of overlying soil, and determines the frequency band range required for the inversion. Next, invert initial velocity structure of the site by using the variation of the partial derivative of the Rayleigh wave velocity to the shear wave velocity with the depth of the soil layer. Combined with the half-wavelength method in engineering site exploration, three types of soil layer models frequently encountered and the measured model of Kushiro site in Japan were analyzed, and the error analysis of the velocity structure was carried out and compared with the theoretical or measured velocity structures. The research shows that the initial velocity structure obtained by the improved half-wavelength method has a maximum error not more than 35%, which can be used to reconstruct a small searching model space in inversion for the wave velocity profile of engineering site by using the geophysical method, so as to improve the speed of inversion calculations and the reliability of result.
-
引言
浅地表剪切波速度结构是进行工程勘察和工程抗震的重要参数，了解地下的剪切波速度结构对地质灾害预测和防御具有重要意义（Strobbia，Cassiani，2011）。获取工程场地剪切波速结构剖面的常规方法分为跨（单）孔法和面波勘探法。跨（单）孔法虽然能获得较准确的速度值，但该方法经济耗费高、效率低，且对环境具有一定的破坏作用。面波勘探法分为人工面波和天然源面波勘探法，其中：人工源面波勘探法受场地条件限制较多，且勘探深度有限（Park et al，1999）；而天然源面波勘探法具有受场地条件限制小、可探测的深度范围大、对所测环境不产生破坏、高效、经济、适合人口密集地区等优点，因而受到越来越多的关注。天然源面波勘探法需从多点记录中提取面波频散曲线，提取面波频散曲线的常用方法主要有空间自相关法（spatial autocorrelation method，缩写为SPAC）（Aki，1957；Okada et al，1990）、频率-波数法（frequency-wavenumber spectral method，缩写为f-k）（Capon，1969；Wathelet et al，2008）。对于提取的频散曲线进行反演分析，则可获得试验场地速度结构。近年来，国内外很多学者利用更为简便的单台记录噪声水平与竖向谱比（noise horizontal to vertical spectral ratio，缩写为NHVSR）进行土层结构反演（王伟君等，2009；Bignardi et al，2016；García-Jerez et al，2016），Rong等（2017）和荣棉水等（2018）在利用测震或强震动观测数据反演浅地表土层速度结构方面也开展了研究，发展了全局优化反演实用方法。
This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
基于以上原因，本文借鉴崔建文等（1994）简化剥层法的思路，对半波长法进行改进，利用场地土层的频散曲线及水平与竖向谱比（horizontal-to-vertical spectral ratio，简写为HVSR）提出一种改进半波长反演方法。通过对理论和实测场地算例分析，得到改进半波长法对土层速度结构的反演误差范围，为采用其它地球物理手段进一步确定土层速度结构提供合理的参数约束区间。
1. 改进半波长法
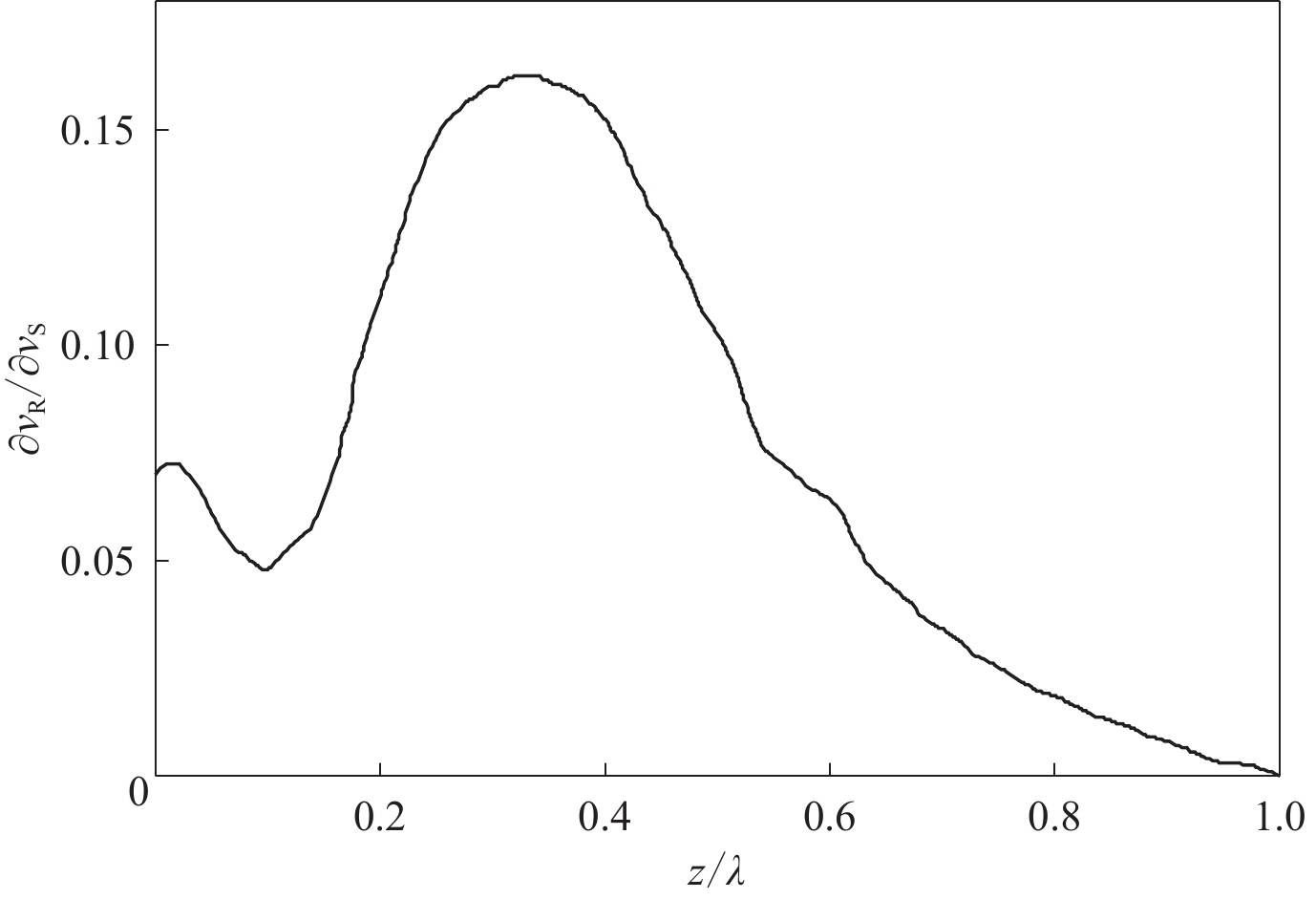
利用面波频散资料反演土层波速的半波长法，是根据均匀半空间中面波相速度近似等于介质剪切波速，在成层弹性半空间中的面波相速度主要受控于一个波长深度的剪切波速，将与波长对应的等效相速度近似折算为相应深度处介质的剪切波速。由于该方法采用了过于简单的近似假定，其计算结果精度较低。而本文提出的改进半波长法侧重于反演工程场地的剪切波速剖面，首先通过场地土层的HVSR确定场地的卓越频率，结合频散曲线获取场地土层厚度；再根据半波长理论确定场地土层反演所需的频带范围，以提高实际资料反演结果的精度和可靠性；最后利用瑞雷面波相速度对剪切波速的偏导数随土层深度的变化规律得出权重，用加权法建立相速度与土层剪切波速的近似关系，从而反演出场地的初始速度结构。以下分别对实际反演过程中改进半波长法的两个步骤进行阐述。
1.1 基岩埋深及频带范围的确定
苏经宇和王广军（1985）通过对典型土层剖面的地震反应分析，指出不同场地的地震效应除受地震参数本身影响外，主要影响因素是场地基岩埋深、场地剖面深度以及土层刚度。由此可以看出，明确基岩埋深不仅可以确定场地土层反演所需的频带范围，而且对评估场地效应也必不可少。
依靠传统钻探技术获取土层厚度等参数由于花费高昂而受限制，充分利用地震和背景噪声等被动源观测记录推断场地土层参数受到了越来越多研究人员的关注。目前场地卓越频率的测定方法主要有依据数值计算的波速数值法和根据场地地震或背景噪声记录的直接测定法。近年来，国内外学者基于观测记录证实了运用HVSR可以准确地确定场地土层卓越频率（Wen et al，2006；王伟君等，2009，2011；Nagashima et al，2014；荣棉水等，2016）。理论HVSR的计算公式系Kawase等（2011）基于散射场理论提出，具体如下：
${\mathop{\rm HVSR}\nolimits} {\text{＝}}\sqrt {\frac{{2\alpha }}{\beta }} \frac{{\left| {{{{\mathop{\rm TF}\nolimits} }_{\mathop{\rm S}\nolimits} }\!\!\!{\text{（}}f{\text{）}}\!\!\!} \right|}}{{\left| {{{{\mathop{\rm TF}\nolimits} }_{\mathop{\rm P}\nolimits} }\!\!\!{\text{（}}f{\text{）}}\!\!\!} \right|}} {\text{，}}$
(1) This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
${f_0} {\text{＝}} \frac{{{v_{\rm S}}}}{{4h}} {\text{，}}$
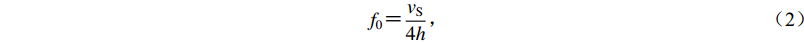
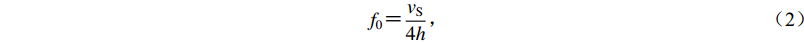
(2) 式中，h为盖土层厚度，vS为等效剪切波波速，f0为土层卓越频率。
当采用被动源台阵获取场地土层面波频散时，由单台三分量记录可额外获取场地的HVSR。基于此，本文提出一种简单、快速的基岩埋深获取方法。首先，根据HVSR方法与波速数值法获得的土层卓越频率相同，结合频散曲线可得出该卓越频率的相速度；然后，根据半波长理论，将该相速度等同于覆盖土层深度内介质的相速度，依此计算出覆盖土层厚度
$h {\text{＝}} \frac{{{v_{\rm S}}}}{{4{f_0}}} {\text{，}}$
(3) 式中，h是由观测记录所得的覆盖土层厚度，vS是由等效相速度折算而来的等效剪切波速。
由vR-f频散曲线可知，频率越高，波长越小，勘探深度越小。因此对于场地土层所需的频带范围，频率上限可取观测所得的最大值，频率下限则根据半波长理论确定为
${h_{\max }} {\text{＝}} \frac{{{\lambda _{\max }}}}{2} {\text{＝}}\frac{v_{\text{R}}\!\!{{{\!{\text{（}}\!f}_{\min } \!{\text{）}}}}}{{2{f_{\min }}}} {\text{，}}$
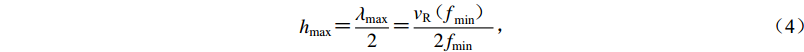
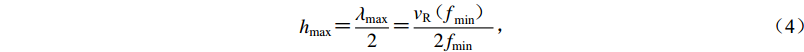
(4) This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
1.2 场地土层剪切波速剖面的反演
This page contains the following errors:
error on line 1 at column 77: Extra content at the end of the documentBelow is a rendering of the page up to the first error.
崔建文等（1994）![]()
图 1This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
Figure 1.This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
在均匀弹性半空间中，剪切波速与瑞雷波相速度之间有如下近似关系（Harker，1988）：
${v_{\rm R}} {\text{≈}} \frac{{0.87 {\text{＋}} 1.12\mu }}{{1 {\text{＋}} \mu }}{v_{\rm S}} {\text{，}}$
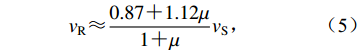
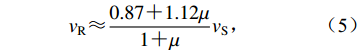
(5) This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
具体反演过程归纳如下：
1） 由实测瑞雷波频散曲线计算波长和等效剪切波速为
${\lambda _j} {\text{＝}} \frac{{{v_{{\mathop{\rm R}\nolimits} j}}}}{{{f_j}}} {\text{，}}$
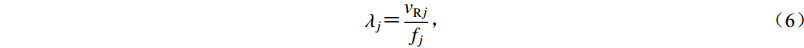
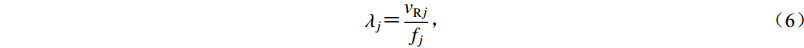
(6) ${\overline v_{{\mathop{\rm S}\nolimits} j}} {\text{＝}} \frac{{1 {\text{＋}} \mu }}{{0.87 {\text{＋}} 1.12\mu }}{v_{{\mathop{\rm R}\nolimits} j}} {\text{，}}$
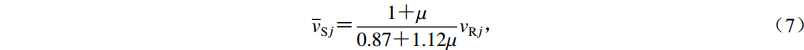
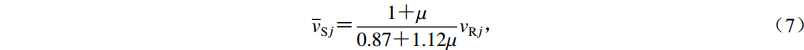
(7) This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
${v_{{\mathop{\rm S}\nolimits} j}} {\text{＝}} \frac{{{{\overline v}_{{\mathop{\rm S}\nolimits} j}} {\text{－}} {P_{j {\text{－}} 1}}{{\overline v}_{j{\rm{ {\text{－}} 1}}}}}}{{{\rm{1 {\text{－}} }}{P_{j {\text{－}} 1}}}} {\text{，}}j{\text{＝}}1 {\text{，}}2 {\text{，}}3 {\text{，}}\cdots {\text{，}}n{\text{.}}$
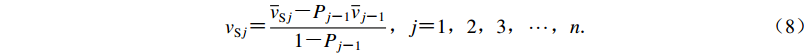
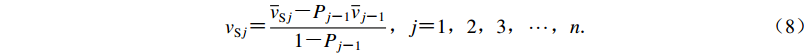
(8) 由图1，式中权系数Pj按
${P_j} {\text{＝}} \dfrac{{\displaystyle \int_0^{{{{H_j}}}/{{{\lambda _{j + 1}}}}} {R\!\!\!\!{\text{（}}\!{\textit{z}}\!{\text{）}}\!\!\!\!} \rm d\xi }}{{\displaystyle \int_0^1 {R\!\!\!\!{\text{（}}\!{\textit{z}}\!{\text{）}}\!\!\!\!} \rm d\xi }} {\text{}}$
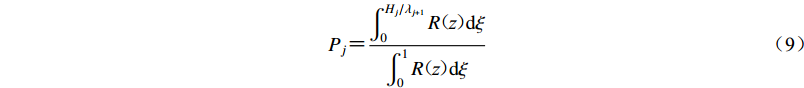
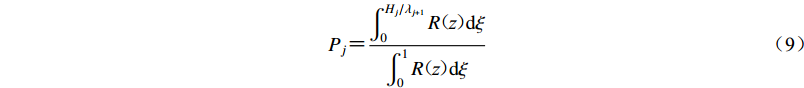
(9) This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
${H_j} {\text{＝}} \left\{ {\begin{array}{*{20}{l}} {{h_1}{\text{，}}}&{j {\text{＝}} 1 {\text{，}}}\\ {{h_1} {\text{＋}} {h_2}{\text{，}}}&{j {\text{＝}} 2 {\text{，}}}\\ {\; \;\;\;\; \vdots}&{\;\;\;\vdots}\\ {{h_1} {\text{＋}} {h_2} {\text{＋}} \cdots {\text{＋}} {h_n}{\text{，}}}&{j {\text{＝}} n {\text{，}}} \end{array}} \right. $
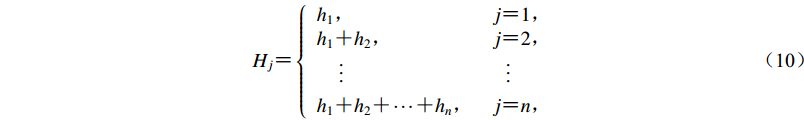
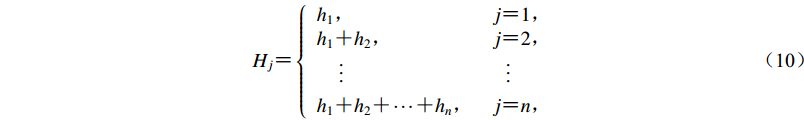
(10) ${\overline v_j} {\text{＝}} \left\{ {\begin{array}{*{20}{l}} {{v_{\rm S1}}{\text{，}} }&{j {\text{＝}} 1{\text{，}}}\\ {\dfrac{{{h_1}{v_{\rm S1}} {\text{＋}} {h_2}{v_{\rm S2}}}}{{{h_1} {\text{＋}} {h_2}}}{\text{，}} }&{j {\text{＝}} 2{\text{，}}}\\ {\;\;\;\;\;\;\;\;\;\;\;\vdots}&{\;\;\;\vdots}\\ {\dfrac{{{h_1}{v_{\rm S1}} {\text{＋}} {h_2}{v_{\rm S2}} {\text{＋}} \cdots {\text{＋}} {h_{n {\text{－}} 1}}{v_{{\rm S}{\!\!\!\!{\text{（}}\!n {\text{－}} {\rm 1\!{\text{）}}\!\!\!\!}}}}}}{{{h_1} {\text{＋}} {h_2} {\text{＋}} ... {\text{＋}} {h_{n {\text{－}} 1}}}}{\text{，}} }&{j {\text{＝}} n {\text{－}} 1{\text{.}}} \end{array}} \right. $
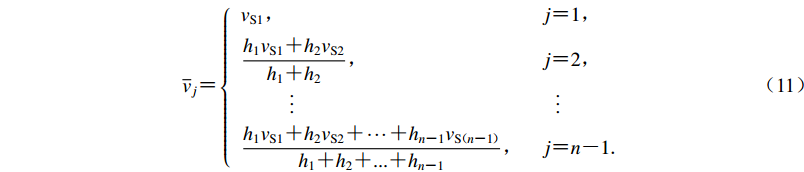
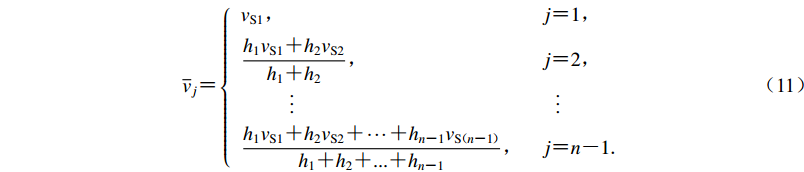
(11) 2. 三类典型土层模型的数值试验及误差分析
2.1 三种典型的土层模型
为了验证改进半波长法的有效性，本文根据大量实际钻孔模型总结了三类典型土层模型对改进半波长法的计算程序进行验证。第一类为递增层模型，第二类为低速夹层模型，第三类为高速夹层模型，如表1所示。工程上一般将剪切波速vS＞500 m/s的层位定义为软基岩，本文将表1中理论模型的底层剪切波速取为600 m/s。
表 1 三类模型的具体属性参数Table 1. Specific attribute parameters of the three types of models模型 层数 层厚/m vP/（m·s−1） vS/（m·s−1） 密度/（g·cm−3） ${Q}_{\rm P}$ 
${Q}_{\rm S}$ 
泊松比μ 递增层 1 5 600 300 1.80 48 24 2 10 700 350 1.90 56 28 3 10 800 400 2.00 64 32 4 10 1000 500 2.10 80 40 无限半空间 ∞ 1200 600 2.20 96 48 低速夹层 1 5 600 300 1.80 48 24 2 10 900 450 1.90 72 36 3 10 700 350 2.00 56 28 0.33 4 10 1000 500 2.10 80 40 无限半空间 ∞ 1200 600 2.20 96 48 高速夹层 1 5 600 300 1.80 48 24 2 10 700 350 1.90 56 28 3 10 1100 550 2.00 88 44 4 10 1000 500 2.10 80 40 无限半空间 ∞ 1200 600 2.20 96 48 This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
2.2 模型分层反演
基于频散曲线求取深度，需计算相速度对波长的偏导数，利用偏导数曲线判断介质的层界面，进而确定层数，并得到该界面深度对应的波长值（张恒山，王庆海，1998）。工程勘察中大多采用波长深度转换系数法，该方法认为，频散曲线的拐点对应于介质的层界面，从而可根据频散曲线的拐点反演出地层的层数，为土层分层提供参考（吴燕清，杨天春，2008）。半波长法就是波长深度转换系数取为0.5的一种半定量的波长解释法。
This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
![]()
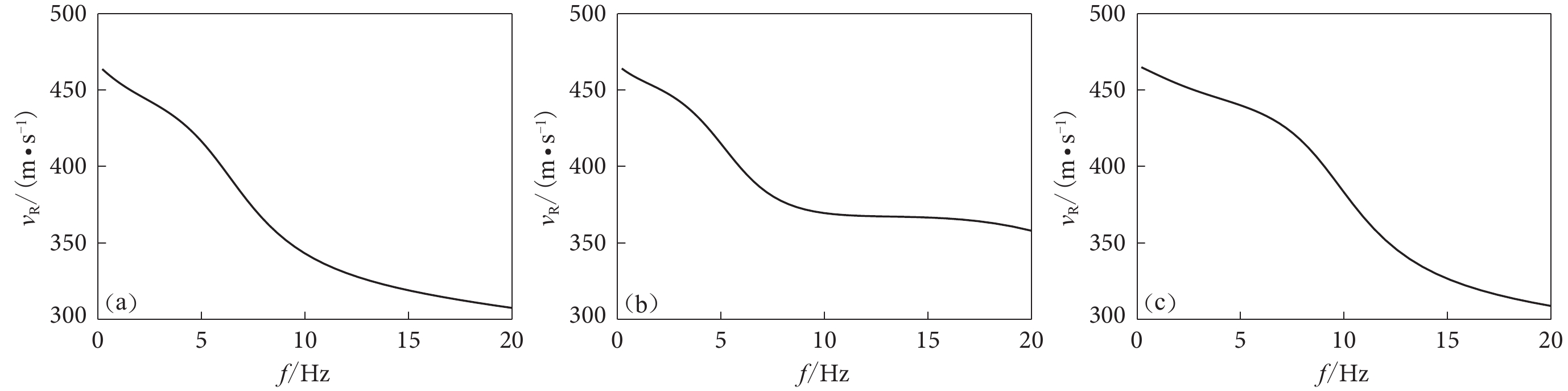
图 2 三类模型的理论频散曲线（a） 递增层模型；（b） 低速夹层模型；（c） 高速夹层模型Figure 2. Theoretical dispersion curves of three types of models（a） The stratified media with S-wave velocity increasing from the top to the bottom；（b） The stratified media with the low-velocity layer in between；（c） The stratified media with the high-velocity layer in between![]()
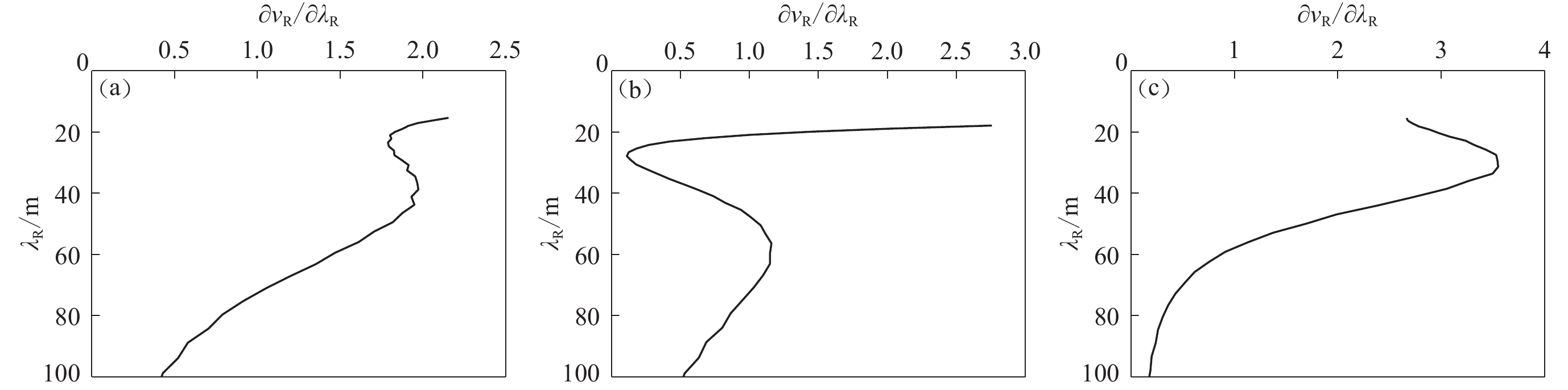
图 3This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
（a） 递增层模型；（b） 低速夹层模型；（c） 高速夹层模型Figure 3.This page contains the following errors:
error on line 1 at column 286: Extra content at the end of the documentBelow is a rendering of the page up to the first error.
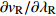（a） The stratified media with S-wave velocity increasing from the top to the bottom；（b） The stratified media with the low-velocity layer in between；（c） The stratified media with the high-velocity layer in between2.3 模型波速反演及其误差分析
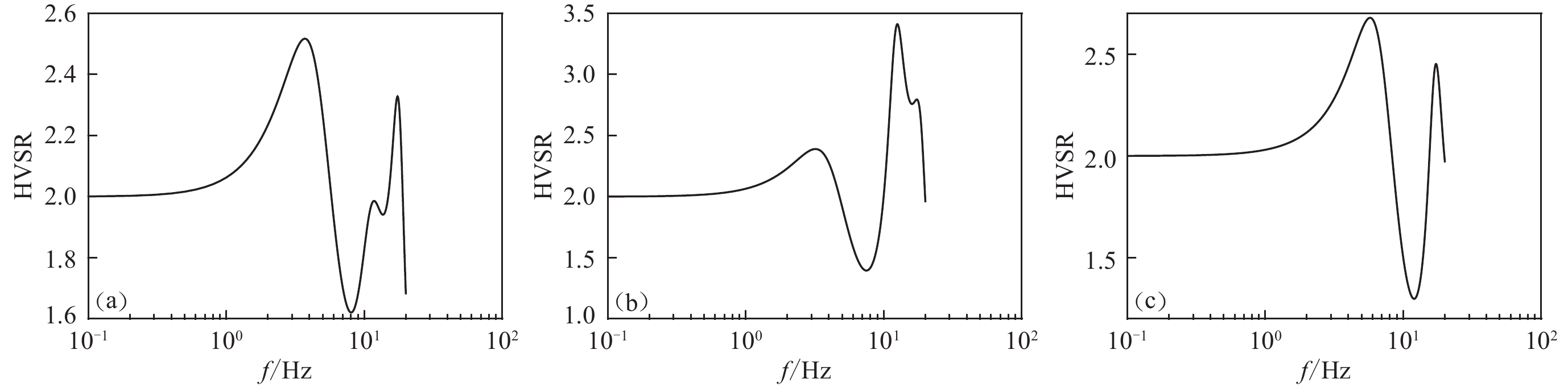
对于表1中三个典型土层模型，由式（1）计算得到三类模型的理论水平与竖向谱比HVSR曲线，如图4所示。根据HVSR曲线得出三类模型的卓越频率，结合三类模型的频散曲线，从而确定出三类模型的覆盖土层厚度，结果如表2所示。
表 2 三类模型的土层厚度Table 2. Soil thickness of three types of models模型名称 卓越频率/Hz 土层厚度/m 递增层模型 3.6 32 低速夹层模型 3.1 38 高速夹层模型 5.6 21 ![]()
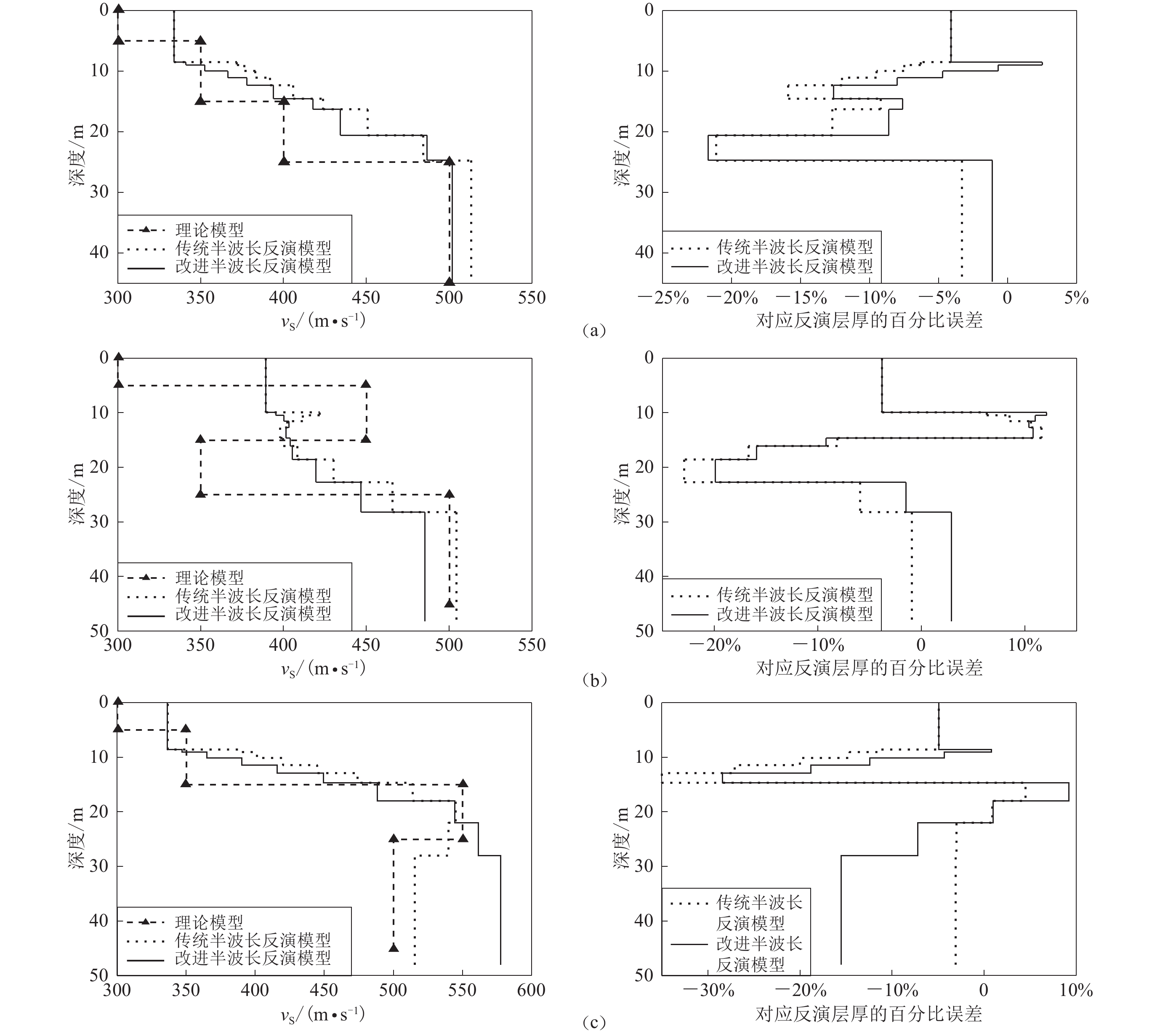
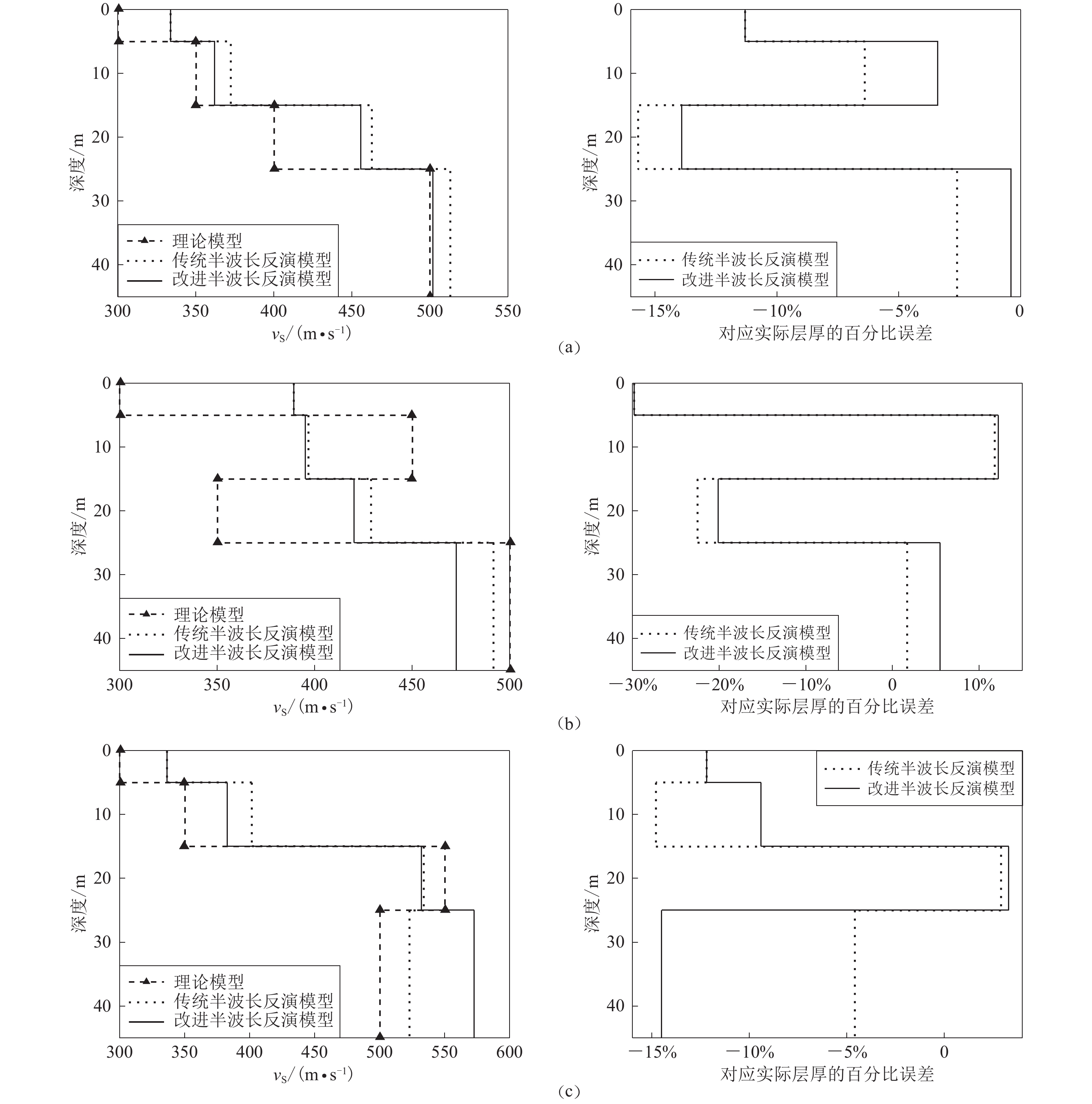
图 4 三类模型的理论水平与竖向谱比HVSR曲线（a） 递增层模型；（b） 低速夹层模型；（c） 高速夹层模型Figure 4. Theoretical HVSR curves for three types of models（a） The stratified media with S-wave velocity increasing from the top to the bottom；（b） The stratified media with the low-velocity layer in between；（c） The stratified media with the high-velocity layer in between基于以上研究结果，根据递增层模型计算所得的32 m覆盖土层厚度，对频散曲线进行计算，频率上限取该场地频散曲线的最大值20 Hz，然后根据覆盖土层厚度，利用半波长法确定所需频散曲线的下限值为6 Hz，即当频散曲线选取在6—20 Hz时反演深度可达33 m。对其它两模型进行相同处理可知，低速夹层模型频散曲线选取在5.5—20 Hz时反演深度达到37.4 m，高速夹层模型频散曲线选取在9.5—20 Hz时反演深度达到20.6 m。对于高速夹层模型，由于深度在15—25 m之间有一层软基岩（vS＝550 m/s），运用卓越频率确定土层厚度时，则将此夹层作为该场地的基岩层。为了对本文方法反演剪切波速剖面进行合理的误差分析，在对该频散曲线进行计算时，覆盖土层厚度取为模型的厚度（35 m），则频率取值为6.2—20 Hz。对于三类模型分别运用传统半波长法以及改进半波长法对相应的频散曲线段进行反演，得到3个剪切波速度剖面，图5左显示出了三种理论模型及两种方法的计算结果。图5右是三种模型下两种方法计算结果相对于实际模型的百分比误差e随深度的变化，其中e计算如下：
![]()
图 5 与反演厚度相关的三种模型的速度剖面（左）及传统、改进半波长法的相对百分比误差（右）（a） 递增层模型；（b） 低速夹层模型；（c） 高速夹层模型Figure 5. The velocity profiles of the three models related to inversion thickness （left） and the relative percentage error variation by the traditional and improved half-wavelength methods with depth （right）（a） The stratified media with S-wave velocity increasing from the top to the bottom；（b） The stratified media with the low-velocity layer in between；（c） The stratified media with the high-velocity layer in between${{e}} {\text{＝}} \frac{{{v_{\rm S}} {\text{－}} v_{\rm S}^*}}{{{v_{\rm S}}}} {\text{×}} {{100 {\text{%}}}}{\text{，}}$
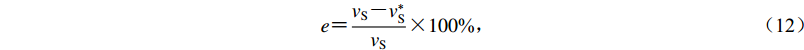
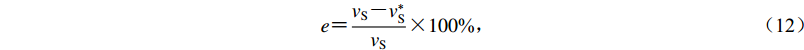
(12) This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
为了定量地分析反演模型与实际模型的剪切波速度误差，本文采用控制变量法，将三类模型反演所得的剪切波速按照实际模型的层厚分别计算出相应的层内平均剪切波速，得到3个剪切波速剖面，图6左为实际的模型和两种方法对应层厚的计算结果。图6右是两种方法计算结果相对于实际模型的百分比误差e随深度的变化。
![]()
图 6 与实际厚度相关的三种模型的速度剖面（左）及传统、改进半波长法的相对百分比误差（右）（a） 递增层模型；（b） 低速夹层模型；（c） 高速夹层模型Figure 6. The velocity profiles of the three models related to actual thickness （left） and the relative percentage error variation by the traditional and improved half-wavelength methods with depth （right）（a） The stratified media with S-wave velocity increasing from the top to the bottom；（b） The stratified media with the low-velocity layer in between；（c） The stratified media with the high-velocity layer in betweenThis page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
${\overline v_{\rm{S}}} {\text{＝}} \frac{{\displaystyle \sum {{v_{\rm S {\textit{i}}}}{h_i}} }}{{\displaystyle \sum {{h_i}} }} {\text{，}}$
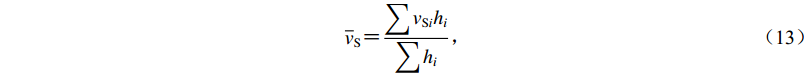
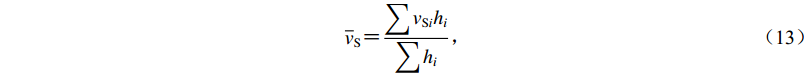
(13) This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
${\overline v_{\rm{S}}^{*}} {\text{＝}} \frac{{\displaystyle \sum {{v_{\rm S{\textit{i}}}^*}h_{i}^{*}} }}{{\displaystyle \sum {h_{i}^{*}} }} {\text{，}}$
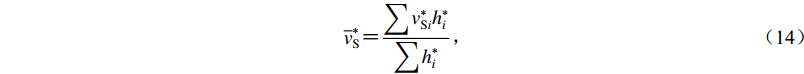
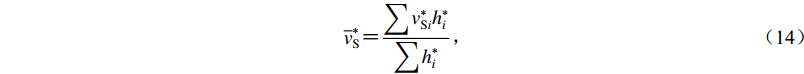
(14) This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
${{{e}}^*} {\text{＝}} \frac{{\overline v_{\rm{S}} {\text{－}} {{\overline{v}}_{\rm S}^{*}}}}{{{\overline v_{\rm{S}}} }} {\text{×}} 100{{\text{%}}} {\text{，}} $
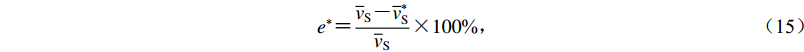
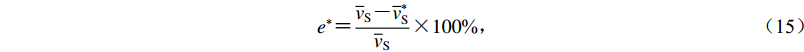
(15) 反演平均波速与模型平均波速的相对误差列于表3。
表 3 理论模型与反演模型的平均波速及相对误差对比Table 3. Comparison of average wave velocity and relative error between theoretical model and inversion model反演方法 递增层模型 低速夹层模型 高速夹层模型 平均波速/（m·s−1） 相对误差 平均波速/（m·s−1） 相对误差 平均波速/（m·s−1） 相对误差 理论模型 400.0 414.3 442.9 传统半波长 429.4 −7.4% 437.1 −5.5% 464.6 −4.9% 改进半波长 421.3 −5.3% 427.9 −3.3% 472.4 −6.7% 对比以上计算结果，可得：
1） 两种反演方法所得平均速度比理论模型偏大，传统半波长法所得速度反演误差不超过7.4%，改进半波长法的速度反演误差不超过6.7%。
2） 两种反演方法的分层是人为给定的，是一种随深度渐变的结果，不代表实际分层，而利用拐点法分层也只能确定基岩界面和部分层面。
3） 传统半波长法在非递增层模型反演中获得的软基岩波速比改进半波长法更接近理论模型，但综合平均波速相对误差，计算结果相对于实际模型的误差，改进半波长法更具优势。
4） 在三个模型中：递增层模型的计算效果最好，两种方法的误差不超过21.7%；高速夹层模型的计算效果最差，两种反演方法的最大误差为35%。
3. 基于实际观测数据的反演算例
This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
![]()
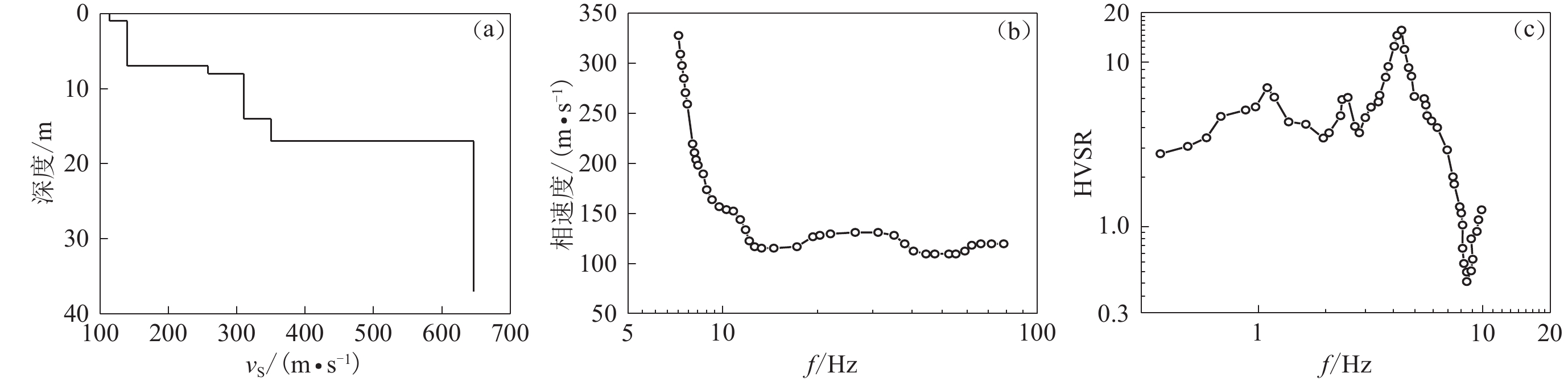
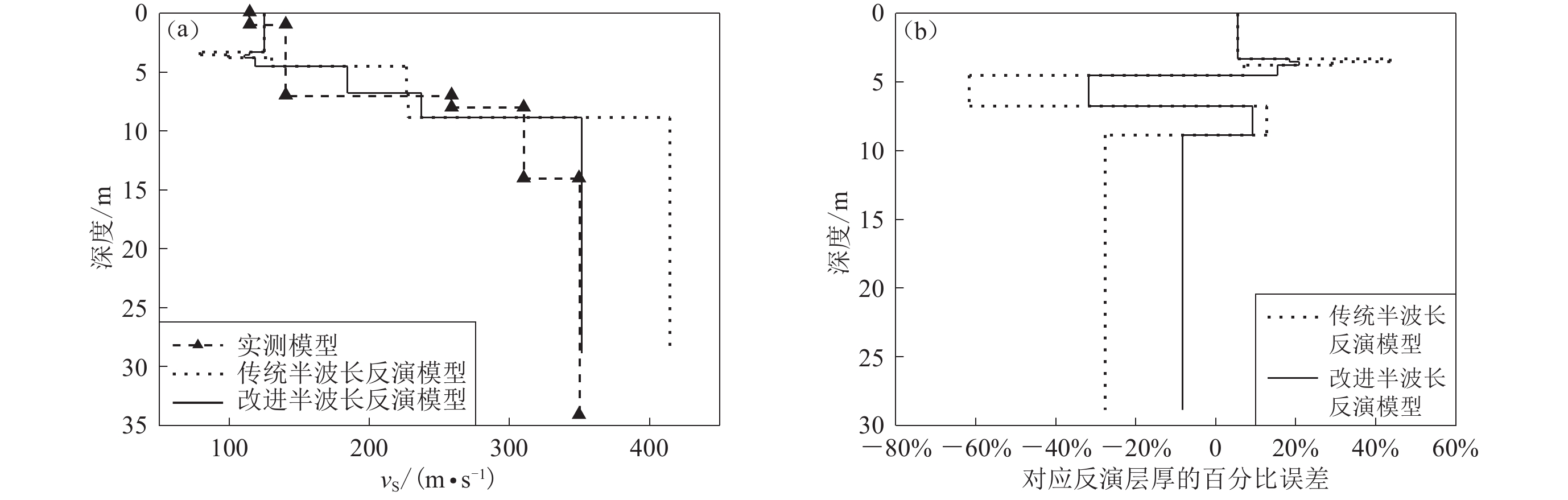
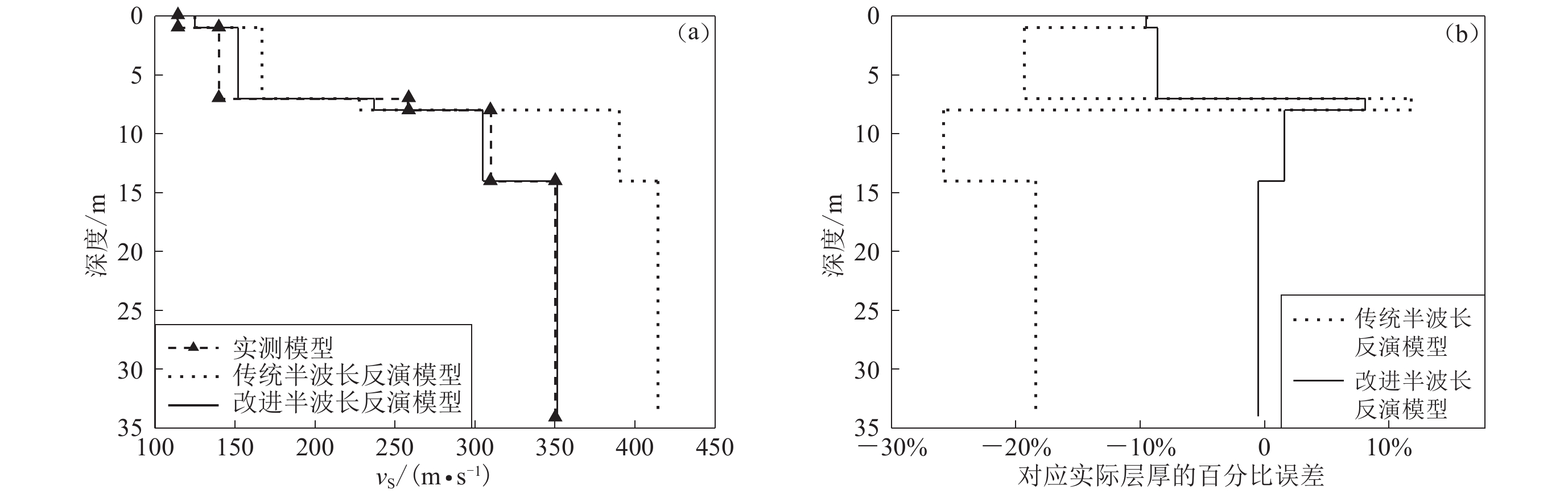
图 7 日本钏路场地剪切波速度剖面、频散曲线与水平与竖向谱比（引自Arai，Tokimatsu，2005）（a） 由钻孔法所得剪切波速度剖面；（b） 由观测数据采用f−k法所得频散曲线；（c） 由三分量传感器观测所得HVSR曲线Figure 7. Shear wave velocity profile，dispersion curve and horizontal-to-vertical spectral ratio HVSR of Kushiro site in Japan （after Arai，Tokimatsu，2005）（a） Shear wave velocity profile obtained from drilling method；（b） The dispersion curve obtained by the f−k method from the observed data；（c） Observed HVSR curve from three-component sensor此外，A场地的钻孔延伸至工程基岩，其剪切波速为600—800 m/s，由钻孔法所得A场地剪切波速度剖面如图7a所示，可知A场地土层厚度为17 m。由此认为Arai和Tokimatsu（2005）对该场地观测台阵采用高分辨率f−k方法获得的频散曲线反演17 m深度内的土层速度结构是合理的。本文根据此频散曲线进行了简单计算，频率下限取该场地频散曲线最小值7.5 Hz，频率上限取为20 Hz，即当频散曲线选取在7.5—20 Hz时反演深度达到19 m，反演获得了基岩以上的土层场地初始速度结构，如图8a所示。从图中可以得出两种反演方法在平均波速方面，其反演误差不超过7.4%，与模型试验所得结果一致。实测模型反演中，在对应反演厚度所得的剪切波速剖面（图8a），改进半波长法所得剪切波速相较于钻孔模型，其相对误差不超过32%，而传统半波长法则误差范围很广，最大可达60%。在对应实测模型厚度所得的剪切波速剖面（图9a），改进半波长法的反演效果很好，与钻孔模型相比，其相对误差不超过10%，传统半波长法则不超过26%。
![]()
图 8 与反演厚度相关的实测模型的速度剖面（a）及传统、改进半波长法的相对百分比误差（b）Figure 8. The velocity profiles of the measured models related to inversion thickness （a） and the relative percentage error variation by the traditional and improved half-wavelength methods with depth （b）![]()
图 9 与实际厚度相关的实测模型的速度剖面（a）及传统、改进半波长法的相对百分比误差（b）Figure 9. The velocity profiles of the measured models related to actual thickness （a） and the relative percentage error variation by the traditional and improved half-wavelength methods with depth （b）4. 结论
This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
中国地震局地壳应力研究所研究生王璞在软件编写中提供了帮助，审稿专家对本文提出了宝贵建议，本文理论频散曲线的正演计算采用了Geopsy软件，作者在此一并表示感谢。
-
![]()
图 1
This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
Figure 1.
This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
![]()
图 2 三类模型的理论频散曲线
（a） 递增层模型；（b） 低速夹层模型；（c） 高速夹层模型
Figure 2. Theoretical dispersion curves of three types of models
（a） The stratified media with S-wave velocity increasing from the top to the bottom；（b） The stratified media with the low-velocity layer in between；（c） The stratified media with the high-velocity layer in between
![]()
图 3
This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
（a） 递增层模型；（b） 低速夹层模型；（c） 高速夹层模型
Figure 3.
This page contains the following errors:
error on line 1 at column 286: Extra content at the end of the documentBelow is a rendering of the page up to the first error.
$\partial {v_{\rm{R}}} / \partial {\lambda _{\rm{R}}}$（a） The stratified media with S-wave velocity increasing from the top to the bottom；（b） The stratified media with the low-velocity layer in between；（c） The stratified media with the high-velocity layer in between
![]()
图 4 三类模型的理论水平与竖向谱比HVSR曲线
（a） 递增层模型；（b） 低速夹层模型；（c） 高速夹层模型
Figure 4. Theoretical HVSR curves for three types of models
（a） The stratified media with S-wave velocity increasing from the top to the bottom；（b） The stratified media with the low-velocity layer in between；（c） The stratified media with the high-velocity layer in between
![]()
图 5 与反演厚度相关的三种模型的速度剖面（左）及传统、改进半波长法的相对百分比误差（右）
（a） 递增层模型；（b） 低速夹层模型；（c） 高速夹层模型
Figure 5. The velocity profiles of the three models related to inversion thickness （left） and the relative percentage error variation by the traditional and improved half-wavelength methods with depth （right）
（a） The stratified media with S-wave velocity increasing from the top to the bottom；（b） The stratified media with the low-velocity layer in between；（c） The stratified media with the high-velocity layer in between
![]()
图 6 与实际厚度相关的三种模型的速度剖面（左）及传统、改进半波长法的相对百分比误差（右）
（a） 递增层模型；（b） 低速夹层模型；（c） 高速夹层模型
Figure 6. The velocity profiles of the three models related to actual thickness （left） and the relative percentage error variation by the traditional and improved half-wavelength methods with depth （right）
（a） The stratified media with S-wave velocity increasing from the top to the bottom；（b） The stratified media with the low-velocity layer in between；（c） The stratified media with the high-velocity layer in between
![]()
图 7 日本钏路场地剪切波速度剖面、频散曲线与水平与竖向谱比（引自Arai，Tokimatsu，2005）
（a） 由钻孔法所得剪切波速度剖面；（b） 由观测数据采用f−k法所得频散曲线；（c） 由三分量传感器观测所得HVSR曲线
Figure 7. Shear wave velocity profile，dispersion curve and horizontal-to-vertical spectral ratio HVSR of Kushiro site in Japan （after Arai，Tokimatsu，2005）
（a） Shear wave velocity profile obtained from drilling method；（b） The dispersion curve obtained by the f−k method from the observed data；（c） Observed HVSR curve from three-component sensor
![]()
图 8 与反演厚度相关的实测模型的速度剖面（a）及传统、改进半波长法的相对百分比误差（b）
Figure 8. The velocity profiles of the measured models related to inversion thickness （a） and the relative percentage error variation by the traditional and improved half-wavelength methods with depth （b）
![]()
图 9 与实际厚度相关的实测模型的速度剖面（a）及传统、改进半波长法的相对百分比误差（b）
Figure 9. The velocity profiles of the measured models related to actual thickness （a） and the relative percentage error variation by the traditional and improved half-wavelength methods with depth （b）
表 1 三类模型的具体属性参数
Table 1 Specific attribute parameters of the three types of models
模型 层数 层厚/m vP/（m·s−1） vS/（m·s−1） 密度/（g·cm−3） ${Q}_{\rm P}$ ${Q}_{\rm S}$ 泊松比μ 递增层 1 5 600 300 1.80 48 24 2 10 700 350 1.90 56 28 3 10 800 400 2.00 64 32 4 10 1000 500 2.10 80 40 无限半空间 ∞ 1200 600 2.20 96 48 低速夹层 1 5 600 300 1.80 48 24 2 10 900 450 1.90 72 36 3 10 700 350 2.00 56 28 0.33 4 10 1000 500 2.10 80 40 无限半空间 ∞ 1200 600 2.20 96 48 高速夹层 1 5 600 300 1.80 48 24 2 10 700 350 1.90 56 28 3 10 1100 550 2.00 88 44 4 10 1000 500 2.10 80 40 无限半空间 ∞ 1200 600 2.20 96 48 
下载: 导出CSV
表 2 三类模型的土层厚度
Table 2 Soil thickness of three types of models
模型名称 卓越频率/Hz 土层厚度/m 递增层模型 3.6 32 低速夹层模型 3.1 38 高速夹层模型 5.6 21
下载: 导出CSV
表 3 理论模型与反演模型的平均波速及相对误差对比
Table 3 Comparison of average wave velocity and relative error between theoretical model and inversion model
反演方法 递增层模型 低速夹层模型 高速夹层模型 平均波速/（m·s−1） 相对误差 平均波速/（m·s−1） 相对误差 平均波速/（m·s−1） 相对误差 理论模型 400.0 414.3 442.9 传统半波长 429.4 −7.4% 437.1 −5.5% 464.6 −4.9% 改进半波长 421.3 −5.3% 427.9 −3.3% 472.4 −6.7%
下载: 导出CSV
-
崔建文,廖振鹏,黄振平. 1994. 反演工程场地剪切波速剖面的简化剥层法[J]. 土木工程学报,<bold>27</bold>(3):50–58. Cui J W,Liao Z P,Huang Z P. 1994. Simplified stripping method for inversing shear wave velocities in site[J]. <italic>China Civil Engineering Journal</italic>,<bold>27</bold>(3):50–58 (in Chinese).
崔建文. 1998. 利用面波资料反演波速剖面的全局优化方法[D]. 哈尔滨: 中国地震局工程力学研究所: 46–53. Cui J W. 1998. The Global Optimization Method for Inverting Velocity Profile With Rayleigh Surface Wave Data[D]. Harbin: Institute of Engineering Mechanics, China Earthquake Administration: 46–53 （in Chinese）.
崔建文. 2004. 一种改进的全局优化算法及其在面波频散曲线反演中的应用[J]. 地球物理学报,<bold>47</bold>(3):521–527. doi: 10.3321/j.issn:0001-5733.2004.03.024 Cui J W. 2004. An improved global optimization method and its application to the inversion of surface wave dispersion curves[J]. <italic>Chinese Journal of Geophysics</italic>,<bold>47</bold>(3):521–527 (in Chinese).
何正勤,丁志峰,贾辉,叶太兰. 2007. 用微动中的面波信息探测地壳浅部的速度结构[J]. 地球物理学报,<bold>50</bold>(2):492–498. doi: 10.3321/j.issn:0001-5733.2007.02.021 He Z Q,Ding Z F,Jia H,Ye T L. 2007. To determine the velocity structure of shallow crust with surface wave information in microtremors[J]. <italic>Chinese Journal of Geophysics</italic>,<bold>50</bold>(2):492–498 (in Chinese).
黄嘉正,周鸿秋,关小平. 1991. 工程地质中瑞利波法勘探的理论初探[J]. 物探与化探,<bold>15</bold>(4):268–277. Huang J Z,Zhou H Q,Guan X P. 1991. Theoretical study of the Rayleigh-wave technique in engineering geology[J]. <italic>Geophysical and Geochemical Exploration</italic>,<bold>15</bold>(4):268–277 (in Chinese).
李瑞山,袁晓铭,吴晓阳. 2018. 抗震规范等效剪切波速简化算法适用性研究[J]. 地震工程与工程振动,<bold>8</bold>(3):30–36. Li R S,Yuan X M,Wu X Y. 2018. Study on the applicability of simplified equivalent shear wave velocity calculation method in seismic design code[J]. <italic>Earthquake Engineering and Engineering Dynamics</italic>,<bold>8</bold>(3):30–36 (in Chinese).
李细兵,范小平. 2014. 基于微动技术探测盆地浅部地层速度结构[J]. 震灾防御技术,<bold>9</bold>(4):821–828. Li X B,Fan X P. 2014. Detection of shallow basin velocity structure based on the microseism technology[J]. <italic>Technology for Earthquake Disaster Prevention</italic>,<bold>9</bold>(4):821–828 (in Chinese).
李细兵,赵启光,宋浩,戴波,张帅帅. 2015. 用台阵微振动方法探测城区地壳浅部介质结构[J]. 防灾减灾工程学报,<bold>35</bold>(4):529–535. Li X B,Zhao Q G,Song H,Dai B,Zhang S S. 2015. Application of microtremor array method to detect the structure of shallow crust in urban area[J]. <italic>Journal of Disaster Prevention and Mitigation Engineering</italic>,<bold>35</bold>(4):529–535 (in Chinese).
李小军,荣棉水,喻烟. 2020. 场地土层模型参数的地震动记录反演方法[J]. 地球物理学报,<bold>63</bold>(1):236–246. doi: 10.6038/cjg2020M0491 Li X J,Rong M S,Yu Y. 2020. Inversion for velocity structure of soil layers by seismic acceleration records[J]. <italic>Chinese Journal of Geophysics</italic>,<bold>63</bold>(1):236–246 (in Chinese).
荣棉水,李小军,王振明,吕悦军. 2016. HVSR方法用于地震作用下场地效应分析的适用性研究[J]. 地球物理学报,<bold>59</bold>(8):2878–2891. Rong M S,Li X J,Wang Z M,Lü Y J. 2016. Applicability of HVSR in analysis of site-effects caused by earthquakes[J]. <italic>Chinese Journal of Geophysics</italic>,<bold>59</bold>(8):2878–2891 (in Chinese).
荣棉水,符力耘,李小军. 2018. 基于单台加速度记录的混合全局优化HVSR反演场地浅层速度结构[J]. 地球物理学报,<bold>61</bold>(3):938–947. doi: 10.6038/cjg2018L0171 Rong M S,Fu L Y,Li X J. 2018. Inversion of site velocity structure using a hybrid optimization algorithm based on HVSRs of accelerograms recorded by a single station[J]. <italic>Chinese Journal of Geophysics</italic>,<bold>61</bold>(3):938–947 (in Chinese).
苏经宇,王广军. 1985. 典型土层剖面的地震反应分析[J]. 工程抗震,(4):16–20. Su J Y,Wang G J. 1985. Seismic response analysis of typical soil layer profiles[J]. <italic>Earthquake Resistant of Engineering</italic>,(4):16–20 (in Chinese).
苏永帅. 2015. 基于地脉动反演硬夹层场地剪切波速[D]. 哈尔滨: 哈尔滨工业大学: 1–36. Su Y S. 2015. Microtremor Survey for Shear Wave Velocity in Site With Hard Interlayer[D]. Harbin: Harbin Institute of Technology: 1–36 （in Chinese）.
王家映. 2002. 地球物理反演理论[M]. 第2版. 北京: 高等教育出版社: 1–181. Wang J Y. 2002. Geophysical Inversion Theory[M]. 2ed. Beijing: Higher Education Press: 1–181 （in Chinese）.
王伟君,刘澜波,陈棋福,张杰. 2009. 应用微动<italic>H</italic>/<italic>V</italic>谱比法和台阵技术探测场地响应和浅层速度结构[J]. 地球物理学报,<bold>52</bold>(6):1515–1525. doi: 10.3969/j.issn.0001-5733.2009.06.013 Wang W J,Liu L B,Chen Q F,Zhang J. 2009. Applications of microtremor <italic>H</italic>/<italic>V</italic> spectral ratio and array techniques in assessing the site effect and near surface velocity structure[J]. <italic>Chinese Journal of Geophysics</italic>,<bold>52</bold>(6):1515–1525 (in Chinese).
王伟君,陈棋福,齐诚,谭毅培,张项,周青云. 2011. 利用噪声HVSR方法探测近地表结构的可能性和局限性:以保定地区为例[J]. 地球物理学报,<bold>54</bold>(7):1783–1797. doi: 10.3969/j.issn.0001-5733.2011.07.012 Wang W J,Chen Q F,Qi C,Tan Y P,Zhang X,Zhou Q Y. 2011. The feasibilities and limitations to explore the near-surface structure with microtremor HVSR method: A case in Baoding area of Hebei Province,China[J]. <italic>Chinese Journal of Geophysics</italic>,<bold>54</bold>(7):1783–1797 (in Chinese).
王振东. 2006. 面波勘探技术要点与最新进展[J]. 物探与化探,<bold>30</bold>(1):1–6. doi: 10.3969/j.issn.1000-8918.2006.01.001 Wang Z D. 2006. Essentials and recent advances of the surface wave exploration technique[J]. <italic>Geophysical and Geochemical Exploration</italic>,<bold>30</bold>(1):1–6 (in Chinese).
吴燕清,杨天春. 2008. 瑞利波频散曲线的反演[J]. 煤炭学报,<bold>33</bold>(10):1097–1101. doi: 10.3321/j.issn:0253-9993.2008.10.004 Wang Y Q,Yang T C. 2008. Inversion of Rayleigh waves dispersion curves[J]. <italic>Journal of China Coal Society</italic>,<bold>33</bold>(10):1097–1101 (in Chinese).
杨成林. 1993. 瑞雷波勘探[M]. 北京: 地质出版社: 1–126. Yang C L. 1993. Rayleigh Wave Exploration[M]. Beijing: Geological Publishing House: 1–126 （in Chinese）.
张恒山,王庆海. 1998. 瑞利波勘探的波长解释法新探[J]. 物探与化探,<bold>22</bold>(4):279–283. Zhang H S,Wang Q H. 1998. A new investigation into interpretation of wavelength in Rayleigh wave exploration[J]. <italic>Geophysicaland Geochemical Exploration</italic>,<bold>22</bold>(4):279–283 (in Chinese).
Aki K. 1957. Space and time spectra of stationary stochastic waves,with special reference to microtremors[J]. <italic>Bull Earthquake Res Inst</italic>,<bold>35</bold>:415–456.
Arai H,Tokimatsu K. 2005. S-wave velocity profiling by joint inversion of microtremor dispersion curve and horizontal-to-vertical (<italic>H</italic>/<italic>V</italic>) spectrum[J]. <italic>Bull Seismol Soc Am</italic>,<bold>95</bold>(5):1766–1778. doi: 10.1785/0120040243
Ballard R F, Chang F K. 1973. Rapid Subsurface Exploration Report 1: Review of Selected Geophysical Techniques[R]. Vicksburg: Soils and Pavements Laboratory, US Army Engineer Waterways Experiment Station: 25.
Bignardi S,Mantovani A,Zeid N A. 2016. Open HVSR:Imaging the subsurface 2D/3D elastic properties through multiple HVSR modeling and inversion[J]. <italic>Comput Geosci</italic>,<bold>93</bold>:103–113. doi: 10.1016/j.cageo.2016.05.009
Capon J. 1969. High-resolution frequency-wavenumber spectrum analysis[J]. <italic>Proc IEEE</italic>,<bold>57</bold>(8):1408–1418. doi: 10.1109/PROC.1969.7278
Constable S C,Parker R L,Constable C G. 1987. Occam’s inversion:A practical algorithm for generating smooth models from electromagnetic sounding data[J]. <italic>Geophysics</italic>,<bold>52</bold>(3):289–300. doi: 10.1190/1.1442303
Dorman J,Ewing M. 1962. Numerical inversion of seismic surface wave dispersion data and crust-mantle structure in the New York-Pennsylvania area[J]. <italic>J Geophys Res</italic>,<bold>67</bold>(13):5227–5241. doi: 10.1029/JZ067i013p05227
García-Jerez A,Piña-Flores J,Sánchez-Sesma F J,Luzón F,Perton M. 2016. A computer code for forward calculation and inversion of the <italic>H</italic>/<italic>V</italic> spectral ratio under the diffuse field assumption[J]. <italic>Comput Geosci</italic>,<bold>97</bold>:67–78. doi: 10.1016/j.cageo.2016.06.016
Harker A H. 1988. Elastic Waves in Solids With Application to Nondestructive Testing of Pipelines[M]. Bristol: Taylor & Francis: 230.
Herak M. 2008. Model HVSR: A Matlab<sup>®</sup> tool to model horizontal-to-vertical spectral ratio of ambient noise[J]. <italic>Comput Geosci</italic>,<bold>34</bold>(11):1514–1526. doi: 10.1016/j.cageo.2007.07.009
Kawase H,Sanchez-Sesma F J,Matsushima S. 2011. The optimal use of horizontal-to-vertical spectral ratios of earthquake motions for velocity inversions based on diffuse-field theory for plane waves[J]. <italic>Bull Seismol Soc Am</italic>,<bold>101</bold>(5):2001–2004. doi: 10.1785/0120100263
Levenberg K. 1944. A method for the solution of certain nonlinear problems in least squares[J]. <italic>Quart Appl Math</italic>,<bold>2</bold>(2):164–168.
Marquardt. 1963. An algorithm for least squares estimation of nonlinear parameters[J]. <italic>J Soc Indus Appl Math</italic>,<bold>11</bold>(2):431–441.
Nagashima F,Matsushima S,Kawase H,Sánchez-Sesma F J,Hayakawa T,Satoh T,Oshima M. 2014. Application of horizontal-to-vertical spectral ratios of earthquake ground motions to identify subsurface structures at and around the K-NET site in Tohoku,Japan[J]. <italic>Bull Seismol Soc Am</italic>,<bold>104</bold>(5):2288–2302. doi: 10.1785/0120130219
Okada H,Matsushima T,Moriya T,Sasatani T. 1990. An exploration technique using long-period microtremors for determination of deep geological structures under urbanized areas[J]. <italic><italic> Butsuri-Tansa</italic> </italic>(<italic><italic> Geophy Explor</italic></italic>)<italic></italic>,<bold>43</bold>:402–417 (in Japanese).
Okada H. 2003. The Microtremor Survey Method[M]. Suto K trans. Tulsa: Society of Exploration Geophysicists: 1−135.
Park C B,Miller R D,Xia J H. 1999. Multichannel analysis of surface waves[J]. <italic>Geophysics</italic>,<bold>64</bold>(3):800–808. doi: 10.1190/1.1444590
Pei D H,Louie J N,Pullammanappallil S K. 2007. Application of simulated annealing inversion on high-frequency fundamental-mode Rayleigh wave dispersion curves[J]. <italic>Geophysics</italic>,<bold>72</bold>(5):R77–R85. doi: 10.1190/1.2752529
Rong M S,Fu L Y,Wang Z M,Li X J,Carpenter N S,Woolery E W,Lyu Y. 2017. On the amplitude discrepancy of HVSR and site amplification from strong-motion observations[J]. <italic>Bull Seismol Soc Am</italic>,<bold>107</bold>(6):2873–2884. doi: 10.1785/0120170118
Sambridge M. 1999. Geophysical inversion with a neighbourhood algorithm: I. Searching a parameter space[J]. <italic>Geophys J Int</italic>,<bold>138</bold>(2):479–494. doi: 10.1046/j.1365-246X.1999.00876.x
Strobbia C,Cassiani G. 2011. Refraction microtremors:Data analysis and diagnostics of key hypotheses[J]. <italic>Geophysics</italic>,<bold>76</bold>(3):MA11–MA20. doi: 10.1190/1.3560246
Tsai N C. 1970. A note on the steady-state response of an elastic half-space[J]. <italic>Bull Seismol Soc Am</italic>,<bold>60</bold>(3):795–808.
Wang Z,Street R,Woolery E,Harris J. 1994. <italic>Q</italic><sub>S</sub> estimation for unconsolidated sediments using first-arrival SH wave critical refractions[J]. <italic>J Geophys Res</italic>:<italic>Solid Earth</italic>,<bold>99</bold>(B7):13543–13551. doi: 10.1029/94JB00499
Wathelet M. 2007. GEOPSY: Geophysical signal database for noise array processing[DB/OL]. [2019−07−05]. http://www.geopsy.org/.
Wathelet M,Jongmans D,Ohrnberger M,Bonnefoy-Claudet S. 2008. Array performances for ambient vibrations on a shallow structure and consequences over <italic>V</italic><sub>S</sub> inversion[J]. <italic>J Seismol</italic>,<bold>12</bold>(1):1–19. doi: 10.1007/s10950-007-9067-x
Wen K L,Chang T M,Lin C M,Chiang H J. 2006. Identification of nonlinear site response using the <italic>H</italic>/<italic>V</italic> spectral ratio method[J]. <italic>Terr Atmos Ocean Sci</italic>,<bold>17</bold>(3):533–546. doi: 10.3319/TAO.2006.17.3.533(T)
Xia J H,Miller R D,Park C B. 1999. Estimation of near-surface shear-wave velocity by inversion of Rayleigh wave[J]. <italic>Geophysics</italic>,<bold>64</bold>(3):691–700. doi: 10.1190/1.1444578
Yamanaka H,Ishida H. 1996. Application of genetic algorithms to an inversion of surface-wave dispersion data[J]. <italic>Bull Seismol Soc Am</italic>,<bold>86</bold>(2):436–444.
-
期刊类型引用(1)
1. 王继鑫，荣棉水，符力耘，傅磊. 用微动台阵记录联合反演场地浅层速度结构——以唐山响嘡台3~#场地为例. 地震地质. 2020(06): 1335-1353 . 
百度学术
其他类型引用(0)
计量
- 文章访问数: 814
- HTML全文浏览量: 357
- PDF下载量: 60
- 被引次数: 1
