IBEM simulation of seismic wave scattering by valley topography with fluid layer
-
摘要: 结合单相介质动力格林函数和流体域格林函数，将间接边界元方法拓展到含流体层河谷对地震波散射的求解，并结合具体算例进行大量参数分析。研究结果表明，含流体层河谷地形对平面P波、SV波入射时的地震响应受控于入射波频率、入射波角度及流体深度等多种因素。总体来看：① 在低频域内，含流体河谷底部及附近地表的频谱特性与不含流体的河谷反应基本一致；② P波入射时在水层体系共振频率处，河谷底部位移缩小效应显著，而此频率处流体表面位移达到最大；③ 流体层具有吸收地震波能量的作用，流体深度越大，河谷表面及附近地表的地震动位移越小。研究成果可在一定程度上为河谷地形附近地震动效应的评估及防震减灾工作提供理论依据。Abstract: Combined with the dynamic Green’s function of single-phase medium and the Green’s function in the fluid domain, the indirect boundary element method is developed to solve the scattering of valley topography with fluid on plane P and SV waves, and the parameter analysis is carried out in combination with specific examples. The results show that the seismic response of valley topography with fluid layer to plane P and SV waves is controlled by many factors, such as incident wave frequency, incident wave angle and the depth of fluid. Generally speaking: ① In the low frequency domain, the frequency spectrum characteristics of the valley bottom and the nearby surface are basically the same in the valley with or without water. ② When P wave incidents, at the resonance frequency of this aquifer system, the displacement at the bottom of the valley decreases significantly, but the displacement of fluid surface reaches the maximum. ③ The fluid layer has the function of absorbing seismic wave energy. The larger the fluid depth is, the smaller the ground motion displacement of the valley surface and nearby ground is. The results can provide a theoretical basis for the evaluation of the ground motion effect near the valley terrain and the work of earthquake prevention and mitigation.
-
引言
我国江河密布，许多重要建筑、桥梁、大坝等坐落在河谷附近。多次震害调查表明，河谷场地对地震动具有显著放大效应。国内外很多专家针对不含流体河谷对地震波的散射问题进行了研究（周国良等，2012；陈少林等，2014；Liu et al，2016；梁建文等，2017；刘中宪等，2017；张宁等，2017；Zhang et al，2017），然而河谷中通常包含一定深度的流体，地震波在流体/固体交界面上会发生复杂的透射与反射现象，从而影响水下地层的地震反应规律，研究流体层对河谷场地地震动的影响意义重大。
王进廷等（2003，2004）研究了P波、SV波入射时弹性半空间上理想流体层的动力响应，随后给出了流体-固体-多孔介质耦合场地中动压力的解析解（Wang et al，2004，2009）；李伟华（2010）采用有限元方法研究了考虑水-饱和土场地-结构耦合时的沉管隧道地震反应；Carbajal-Romero等（2013）采用间接边界元法（indirect boundary element method，缩写为IBEM）研究了Scholte波在流体-固体界面处的扩散；Alejandro等（2014）采用边界元法分析了理论地震事件发生时海洋水体的动态响应；杜修力等（2015）根据地震与波浪作用的动水压力解析解，研究了SV波斜入射时地震和波浪联合作用下自由场海水的动水压力反应问题；张奎等（2018）采用解析解方法得到了水下地层表面位移的表达式，并分析了含水层场地模型中土的刚度、饱和度及平面P波、SV波的入射角等因素变化时流体深度对场地位移响应的影响。
需注意的是以上研究均是针对含水平成层流体的场地动态响应问题，而考虑地震波散射效应对含流体层河谷地形的地震动影响的研究仍非常有限。李伟华和赵成刚（2006）利用波函数展开法，首次在频率域内给出了具有饱和土沉积层的圆弧形充水河谷对平面P波、SV波散射问题的解析解，继而给出了瑞雷波的解析解（赵成刚等，2008）。解析方法中波场构造需严格满足波动方程和边界条件，对于求解复杂的偏微分算子及边界条件难度较大。
在各类数值方法中，边界元法具有降低问题求解维数、自动满足无限远辐射条件且无高频数值弥散的优点，因而在地震波动研究中获得广泛应用。本文尝试将间接边界元法（IBEM）拓展到含流体层局部场地对地震波的散射求解：结合流体域格林函数，将河谷中的流体层模拟为无黏性、可压缩流体，考虑固体/液体交界面上地震波的透射与反射问题，研究了含流体层河谷场地在平面P波、SV波入射时的地震响应。通过与现有文献结果作对比，验证了该方法的精度和数值稳定性。进而结合具体算例，给出了入射波的频率、角度、流体深度等因素对河谷底部及附近地表位移放大系数的影响，为实际情况中含水河谷地震动的确定提供了定性和定量的参考依据。
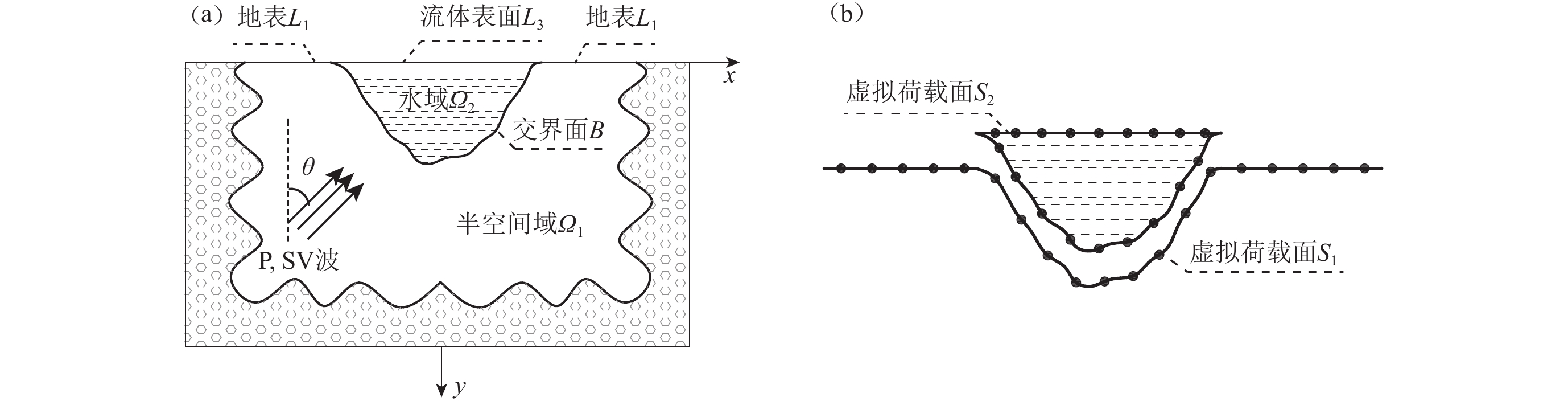
1. 计算模型
图1给出了计算模型。假设半空间河谷中为各向同性弹性介质，Ω1表示半空间域，Ω2表示水域；B表示水域与半空间域的交界面；与空气直接接触的表面用L表示，L1表示两侧水平地表，L3表示流体表面；θ为P波或SV波入射方向与竖直向的夹角；S1表示半空间域的虚拟荷载面，S2表示水域的虚拟荷载面。假设P波、SV波从外部半空间入射，待求问题即为含流体层河谷场地对地震波的散射。
![]()
图 1 含流体层沉积河谷对地震波的散射计算模型（a） 含流体河谷计算模型；（b） 单元离散Figure 1. Calculation model of seismic wave scattering by sedimentary valley with fluid layer（a） Calculation model of the valley with fluid layer；（b） Element discretization2. 间接边界元法的求解
2.1 半空间波场模拟
半空间Ω1内的总位移场和总应力场可表示为
$u_i^{}(x{\text{，}}\omega) {\text{＝}} u_i^{\rm{s}}(x{\text{，}}\omega) {\text{＋}} u_i^{\rm{f}}(x{\text{，}}\omega){\text{，}}x \in {\varOmega _1}{\text{，}}$
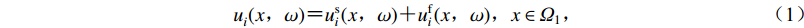
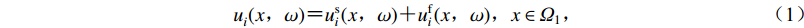
(1) $t_i^{}(x{\text{，}}\omega) {\text{＝}} t_i^{\rm{s}}(x{\text{，}}\omega) {\text{＋}} t_i^{\rm{f}}(x{\text{，}} \omega){\text{，}}x \in {\varOmega _1}{\text{，}}$
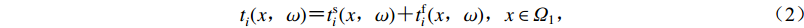
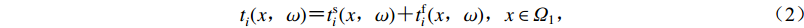
(2) This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
$u_i^{\rm{s}}(x{\text{，}}\omega) {\text{＝}} \int_{{S_1}} {\phi _j^{\rm{s}}(\xi{\text{，}}\omega)G_{ij}^{\rm{s}}(x{\text{，}}\xi){\rm{d}}{S_\xi }}{\text{，}}$
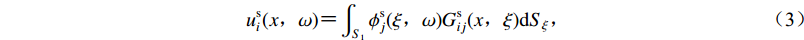
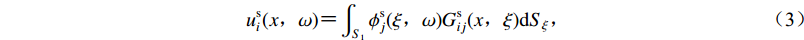
(3) $t_i^{\rm{s}}(x{\text{，}}\omega) {\text{＝}} {c_2}\phi _j^{\rm{s}}(x{\text{，}}\omega) {\text{＋}} \int_{{S_1}} {\phi _j^{\rm{s}}(\xi{\text{，}}\omega)T_{ij}^{\rm{s}}(x{\text{，}}\xi){\rm{d}}{S_\xi }}{\text{，}}$
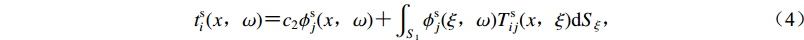
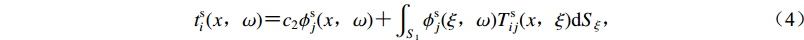
(4) This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
2.2 流体层中的波场模拟
This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
${w_r}(x{\text{，}}\omega) {\text{＝}} {c_1}\psi (x{\text{，}}\omega) {\text{＋}} \frac{1}{{\rho {\omega ^2}}}\int_{{S_2}} \psi (\xi{\text{，}}\omega)\frac{{\partial {G^{\rm{w}}}(x{\text{，}}\xi)}}{{\partial r}}{\rm{d}}{S_\xi }{\text{，}}$
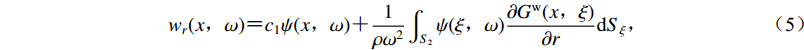
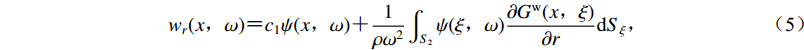
(5) $p(x{\text{，}}\omega){\text{＝}} \int_{{S_2}} \psi (\xi{\text{，}}\omega){G^{\rm{w}}}(x{\text{，}}\xi){\rm{d}}{S_\xi }{\text{，}}$
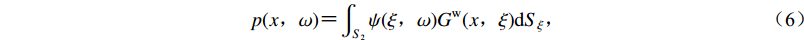
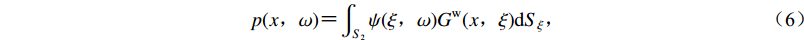
(6) This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
${G}^{\rm{w}}(x{\text{，}}\xi){\text{＝}}\frac{\rho {\omega }^{2}}{4\rm{i}} \cdot {{\rm{H}}}_{0}^{(2)} \cdot \frac{\omega d}{{c}^{\rm{w}}},$
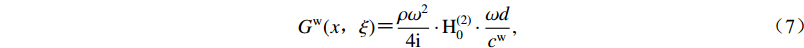
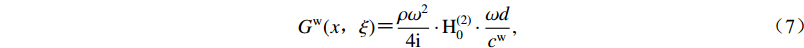
(7) This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
2.3 边界条件及求解
边界条件主要分为三部分：一部分是直接与空气接触的地表L1，满足地表零应力条件；一部分是与空气接触的水体表面L3，满足流体层表面孔压为零；另一部分是半空间与流体域的交界面B处，满足位移连续条件和应力连续条件，包括流量位移和孔压连续及固体位移和应力连续。可分别表示为
$t_\tau ^{\rm{s}}(x{\text{，}}\omega) {\text{＋}} t_\tau ^{\rm{f}}(x{\text{，}}\omega) {\text{＝}} 0{\text{，}}x \in {L_1}{\text{，}}$
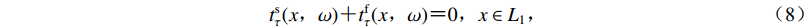
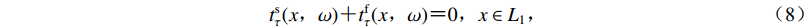
(8) $t_r^{\rm{s}}(x{\text{，}}\omega) {\text{＋}} t_r^{\rm{f}}(x{\text{，}}\omega) {\text{＝}} 0{\text{，}}x \in {L_1}{\text{，}}$
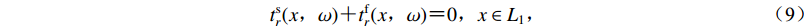
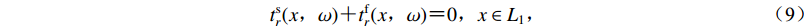
(9) $p(x{\text{，}}\omega) {\text{＝}} 0{\text{，}}x \in {L_3}{\text{，}}$
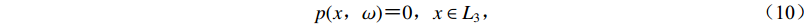
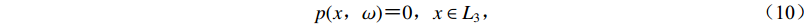
(10) $t_\tau ^{\rm{s}}(x{\text{，}}\omega) {\text{＋}} t_{\rm{\tau }}^{\rm{f}}(x{\text{，}}\omega) {\text{＝}} 0{\text{，}}x \in B{\text{，}}$
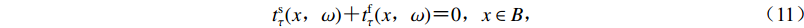
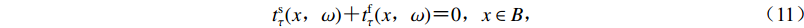
(11) $t_r^{\rm{s}}(x{\text{，}}\omega) {\text{＋}} t_r^{\rm{f}}(x{\text{，}}\omega) {\text{＝－}} p{\text{，}}x \in B{\text{，}}$
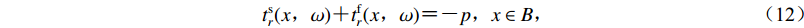
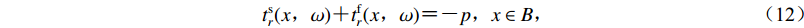
(12) $u_r^{\rm{s}}(x{\text{，}}\omega) {\text{＋}} u_r^{\rm{f}}(x{\text{，}}\omega) {\text{＝}} {w_r}(x{\text{，}}\omega){\text{，}}x \in B{\text{，}}$
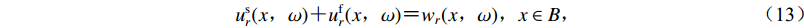
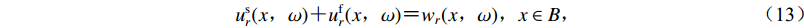
(13) ${c_2}\phi _j^{\rm{s}}(x{\text{，}}\omega) {\text{＋}} \int_{{S_1}} {\phi _j^{\rm{s}}(\xi{\text{，}}\omega)T_{\tau j}^{\rm{s}}(x{\text{，}}\xi){\rm{d}}{S_\xi }} {\text{＝－}}t_\tau ^{\rm{f}}(x{\text{，}}\omega){\text{，}}x \in {L_1}{\text{，}}$
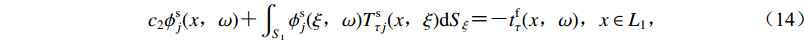
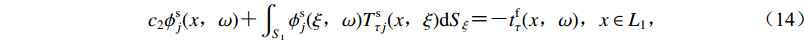
(14) ${c_2}\phi _j^{\rm{s}}(x{\text{，}}\omega) {\text{＋}} \int_{{S_1}} {\phi _j^{\rm{s}}(\xi{\text{，}}\omega)T_{rj}^{\rm{s}}(x{\text{，}}\xi){\rm{d}}{S_\xi }} {\text{＝－}} t_r^{\rm{f}}(x{\text{，}}\omega){\text{，}}x \in {L_1}{\text{，}}$
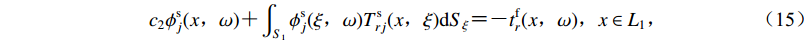
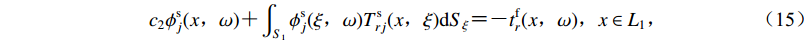
(15) $\int_{{S_2}} \psi (\xi{\text{，}}\omega){G^{\rm{f}}}(x{\text{，}}\xi){\rm{d}}{S_\xi } {\text{＝}} 0{\text{，}}x \in {L_3}{\text{，}}$
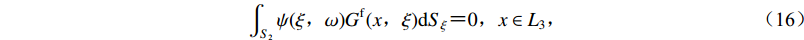
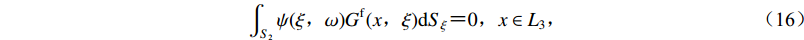
(16) ${c_2}\phi _j^{\rm{s}}(x{\text{，}}\omega) {\text{＋}} \int_{{S_1}} {\phi _j^{\rm{s}}(\xi{\text{，}}\omega)T_{\tau j}^{\rm{s}}(x{\text{，}}\xi){\rm{d}}{S_\xi }} {\text{＝－}} t_\tau ^{\rm{f}}(x{\text{，}}\omega){\text{，}}x \in B{\text{，}}$
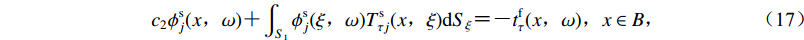
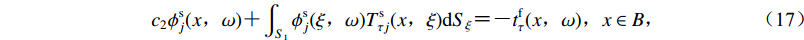
(17) ${c_2}\phi _j^{\rm{s}}(x{\text{，}}\omega) {\text{＋}} \int_{{S_1}} {\phi _j^{\rm{s}}(\xi{\text{，}}\omega)T_{rj}^{\rm{s}}(x{\text{，}}\xi){\rm{d}}{S_\xi }} {\text{＋}} \int_{{S_2}} \psi (\xi{\text{，}}\omega){G^{\rm{f}}}(x{\text{，}}\xi){\rm{d}}{S_\xi } {\text{＝－}} t_r^{\rm{f}}(x{\text{，}}\omega){\text{，}}x \in B{\text{，}}$
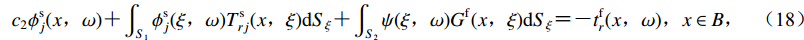
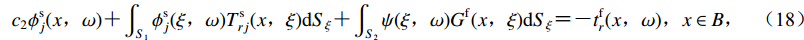
(18) $\int_{{S_1}} {\phi _j^{\rm{s}}(\xi{\text{，}}\omega)G_{rj}^{\rm{s}}(x{\text{，}}\xi){\rm{d}}{S_\xi }} - {c_1}\psi (x{\text{，}}\omega) - \frac{1}{{\rho {\omega ^2}}}\int_{{S_2}} \psi (\xi{\text{，}}\omega)\frac{{\partial {G^{\rm{f}}}(x{\text{，}}\xi)}}{{\partial r}}{\rm{d}}{S_\xi } {\text{＝－}} u_r^{\rm{f}}(x{\text{，}}\omega){\text{，}}x \in B{\text{，}}$
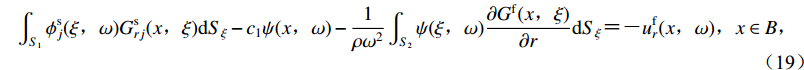
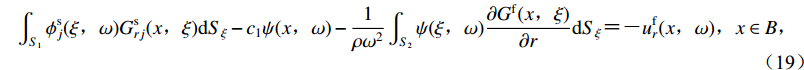
(19) 存在线性方程组
$\sum\limits_{l {\text{＝}} 1}^N {\left[ {\sum\limits_{j {\text{＝}} 1}^2 {\phi _j^{\rm{s}}({\xi _l}{\text{，}}\omega)t_{\tau j}^{\rm{s}}({x_n}{\text{，}}{\xi _l})} } \right]} {\text{＝－}} t_\tau ^{\rm{f}}({x_n}{\text{，}}\omega){\text{，}}{x_n} \in {L_1}{\text{，}}$
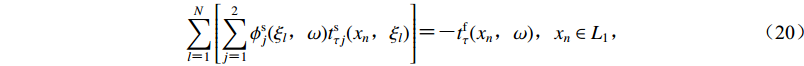
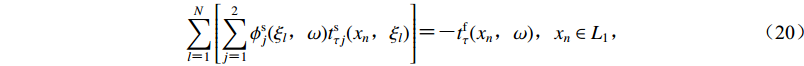
(20) $\sum\limits_{l {\text{＝}} 1}^N {\left[ {\sum\limits_{j {\text{＝}} 1}^2 {\phi _j^{\rm{s}}({\xi _l}{\text{，}}\omega)t_{rj}^{\rm{s}}(x{\text{，}}{\xi _l})} } \right]} {\text{＝－}} t_r^{\rm{f}}({x_n}{\text{，}}\omega){\text{，}}{x_n} \in {L_1}{\text{，}}$
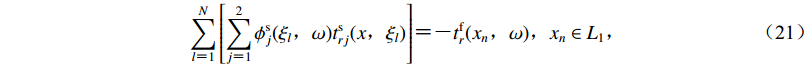
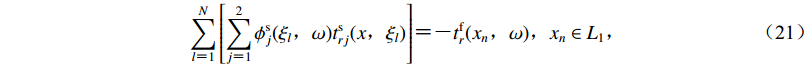
(21) $\sum\limits_{l {\text{＝}} 1}^N {\left[ {\sum\limits_{j {\text{＝}} 1}^2 {\psi ({\xi _l}{\text{，}}\omega){g^{\rm{f}}}({x_n}{\text{，}}{\xi _l})} } \right]} {\text{＝}} 0{\text{，}}{x_n} \in {L_3}{\text{，}}$
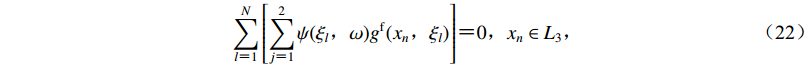
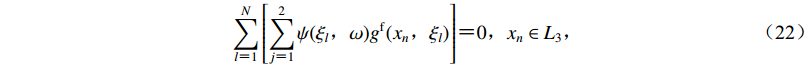
(22) $\sum\limits_{l {\text{＝}} 1}^N {\left[ {\sum\limits_{j {\text{＝}} 1}^2 {\phi _j^{\rm{s}}({\xi _l}{\text{，}}\omega)t_{\tau j}^{\rm{s}}({x_n}{\text{，}}{\xi _l})} } \right]} {\text{＝－}} t_\tau ^{\rm{f}}({x_n}{\text{，}}\omega){\text{，}}{x_n} \in B{\text{，}}$
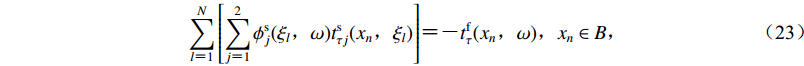
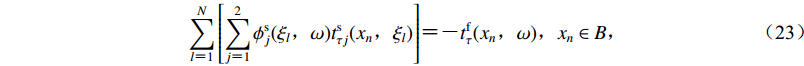
(23) $\sum\limits_{l {\text{＝}} 1}^N {\left[ {\sum\limits_{j {\text{＝}} 1}^2 {\phi _j^{\rm{s}}({\xi _l}{\text{，}}\omega)t_{rj}^{\rm{s}}({x_n}{\text{，}}{\xi _l})} } \right]} {\text{＋}} \sum\limits_{l {\text{＝}} 1}^N {\left[ {\sum\limits_{j {\text{＝}} 1}^2 {\psi ({\xi _l}{\text{，}}\omega)g_1^{\rm{f}}({x_n}{\text{，}}{\xi _l})} } \right]} {\text{＝－}} t_r^{\rm{f}}({x_n}{\text{，}}\omega){\text{，}}{x_n} \in B{\text{，}}$
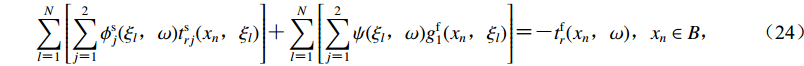
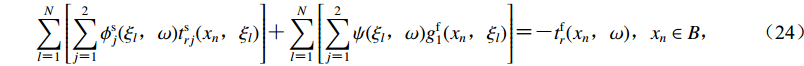
(24) $\sum\limits_{l {\text{＝}}1}^N {\left[ {\sum\limits_{j {\text{＝}} 1}^2 {\phi _j^{\rm{s}}({\xi _l}{\text{，}}\omega)g_{rj}^{\rm{s}}({x_n}{\text{，}}{\xi _l})} } \right]} {\text{－}} \sum\limits_{l {\text{＝}} 1}^N {\left[ {\sum\limits_{j {\text{＝}}1}^2 {\psi ({\xi _l}{\text{，}}\omega)g_2^{\rm{f}}({x_n}{\text{，}}{\xi _l})} } \right]} {\text{＝－}} u_r^{\rm{f}}({x_n}{\text{，}}\omega){\text{，}}{x_n} \in B{\text{，}}$
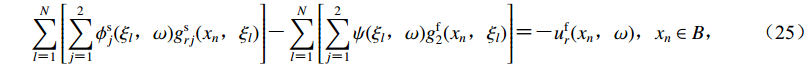
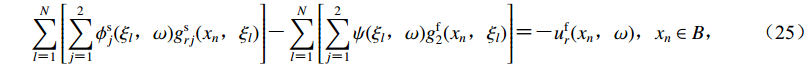
(25) 式中，
$t_{\tau j}^{\rm{s}}({x_n}{\text{，}}{\xi _l}){\text{＝}}{c_{\rm{2}}}{\delta _{ij}}{\delta _{nl}} {\text{＋}} \int_{{S_1}} {T_{\tau j}^{\rm{s}}({x_n}{\text{，}}{\xi _l}){\rm{d}}{S_\xi }}{\text{，}}$
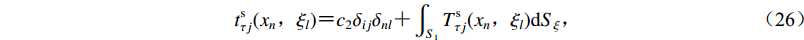
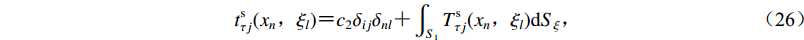
(26) $t_{rj}^{\rm{s}}({x_n}{\text{，}}{\xi _l}){\text{＝}}{c_{\rm{2}}}{\delta _{ij}}{\delta _{nl}} {\text{＋}} \int_{{S_1}} {T_{rj}^{\rm{s}}({x_n}{\text{，}}{\xi _l}){\rm{d}}{S_\xi }}{\text{，}}$
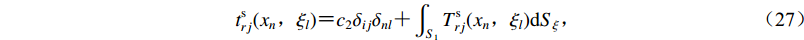
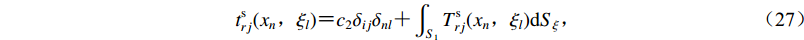
(27) $g_1^{\rm{f}}({x_n}{\text{，}}{\xi _l}){\text{＝}}\int_{{S_2}} {{G^{\rm{f}}}({x_n}{\text{，}}{\xi _l}){\rm{d}}{S_\xi }}{\text{，}}$
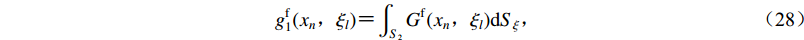
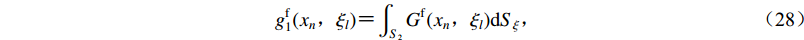
(28) $g_{rj}^{\rm{s}}({x_n}{\text{，}}{\xi _l}) {\text{＝}} \int_{{S_1}} {G_{rj}^{\rm{s}}({x_n}{\text{，}}{\xi _l}){\rm{d}}{S_\xi }}{\text{，}}$
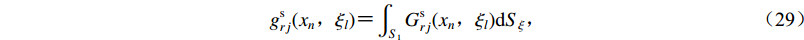
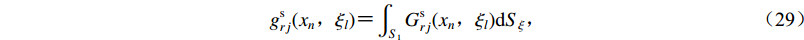
(29) $g_2^{\rm{f}}({x_n}{\text{，}}{\xi _l}){\text{＝}}{c_{\rm{1}}}{\delta _{ij}}{\delta _{nl}} {\text{＋}} \frac{1}{{\rho {\omega ^2}}}\int_{{S_2}} {\frac{{\partial {G^{\rm{f}}}({x_n}{\text{，}}{\xi _l})}}{{\partial r}}{\rm{d}}{S_\xi }}{\text{，}}$
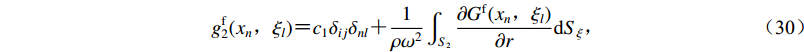
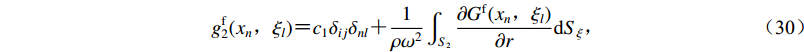
(30) n1＝1，2，···，N1；n2＝1，2，···，N2；N为S1，S2上点的个数。
3. 精度验证
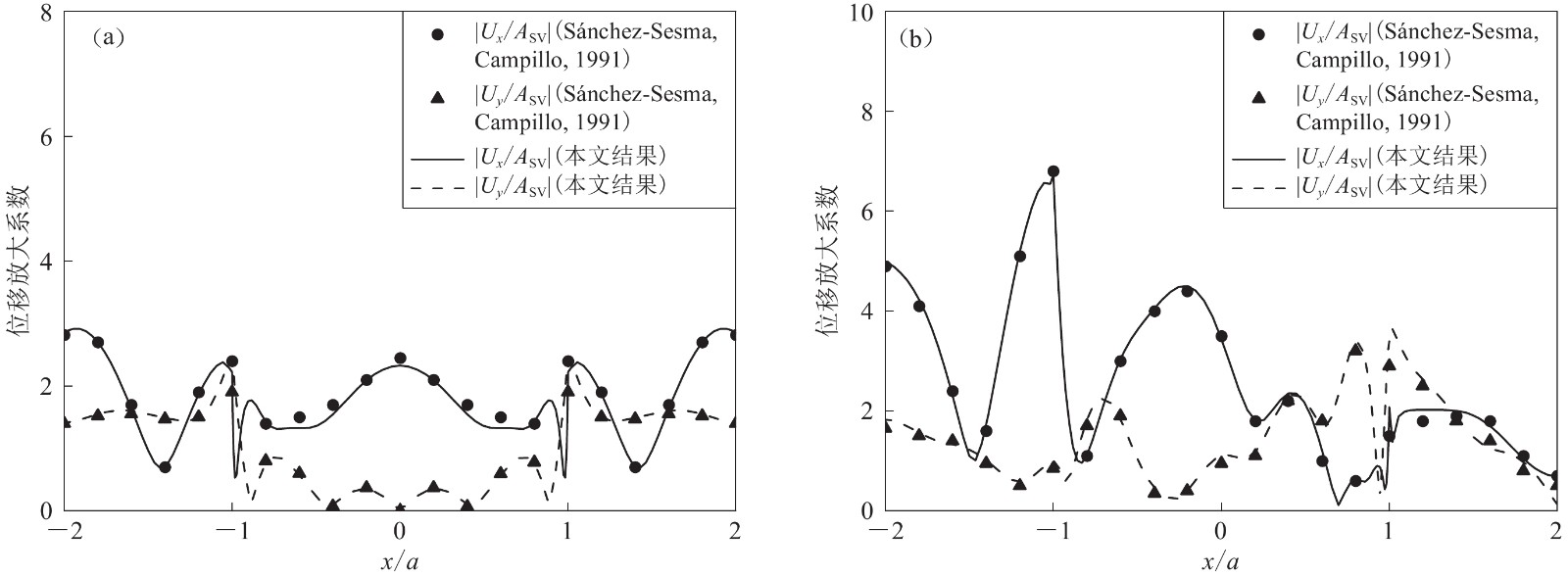
为验证本文IBEM方法的正确性，令流体深度h＝0，计算模型如图2所示，则本文模型退化为不含流体层的河谷地形，并将所得结果与既有文献（Sánchez-Sesma，Campillo，1991）结果进行对比（图3）。计算参数为：入射波为平面SV波，弹性介质中剪切波速cβ1为1 000 m/s，压缩波速cα1为2 000 m/s，密度ρ1为2 400 kg/m3；峡谷中空气的压缩波速cα1为330 m/s，密度ρ2为1.29 kg/m3；无量纲入射波频率η＝ωr/πcβ＝2.0，a为河谷半径，入射波角度分别取θβ＝0°，θβ＝30；阻尼比ζ＝0.001，泊松比v＝1/3。图3表明本文结果与文献结果吻合良好，验证了计算方法的正确性。
![]()
图 3 平面SV波入射下本文含水河谷退化位移与文献结果对比 （引自Sánchez-Sesma，Campillo，1991）Figure 3. Comparison between the degradation displacement of water bearing valley of this paper and the results of literature induced by plane SV waves （after Sánchez-Sesma，Campillo，1991）（a） η＝2.0，θβ＝0°；（b） η＝2.0，θβ＝30°4. 算例分析
采用上述间接边界元方法，研究含流体层的河谷地形对平面P波、SV波的地震响应，计算模型为含流体层的半圆形河谷地形（图2）。阻尼比ζ＝0.001，计算参数列于表1。
表 1 含流体层河谷地形对平面P波、SV波的地震响应计算参数Table 1. Calculation parameters of seismic response of valley terrain with fluid layer for plane P wave and SV waveP波波速cα/（m·s−1） SV波波速cβ/（m·s−1） 密度ρ/（kg·m−3） 空气 330 — 1.29 流体 1 501 — 1 000 弹性土体 2 670 1 090 2 200 4.1 充满流体的河谷对平面P波、SV波的散射作用
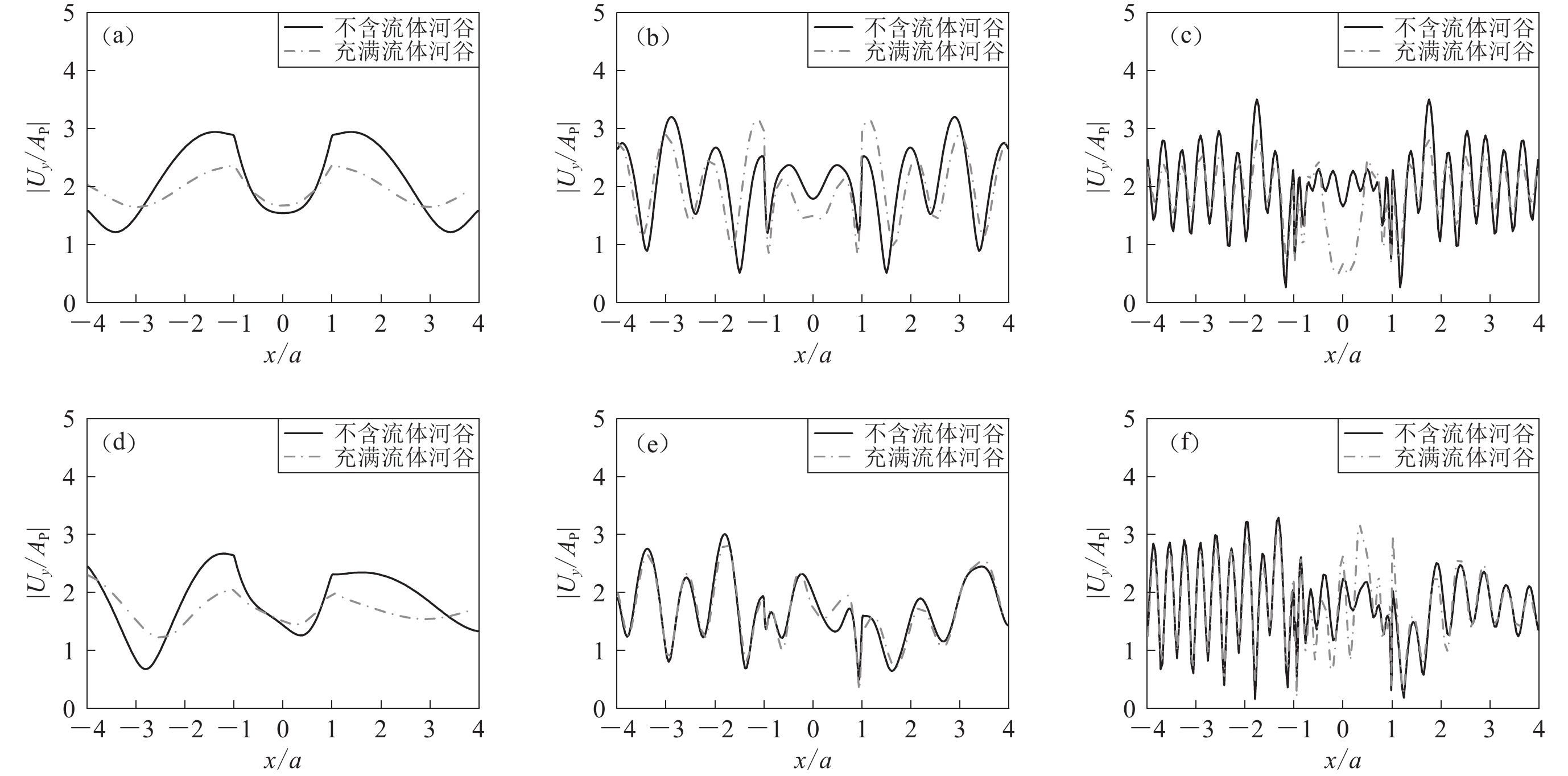
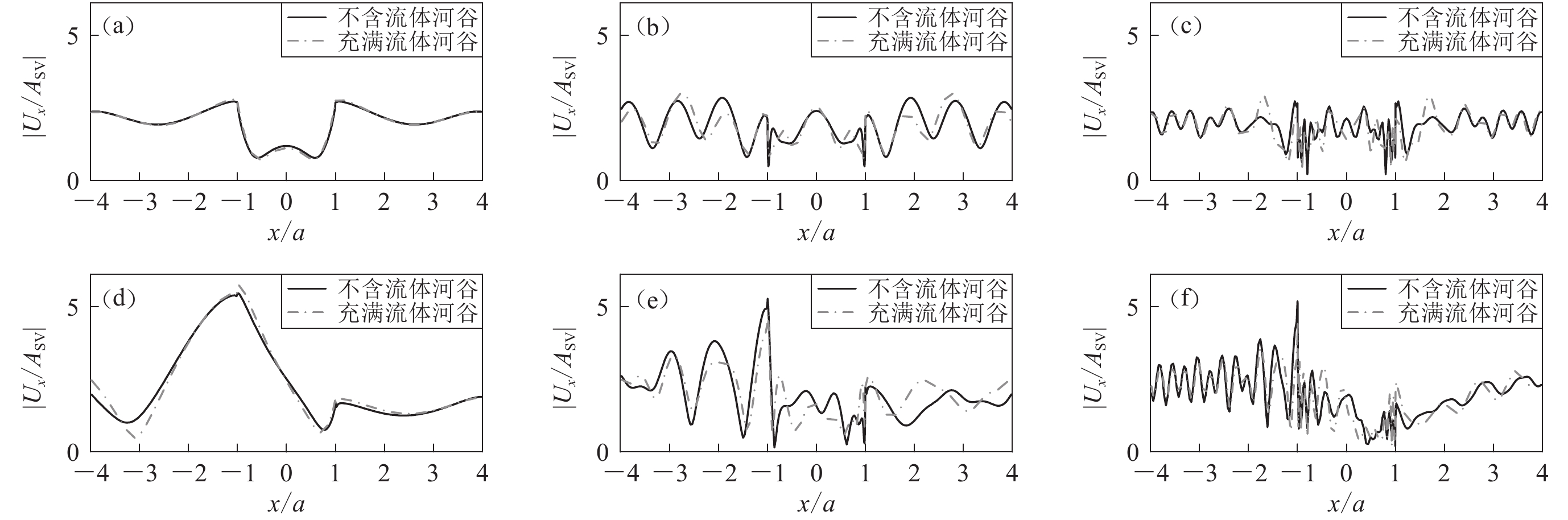
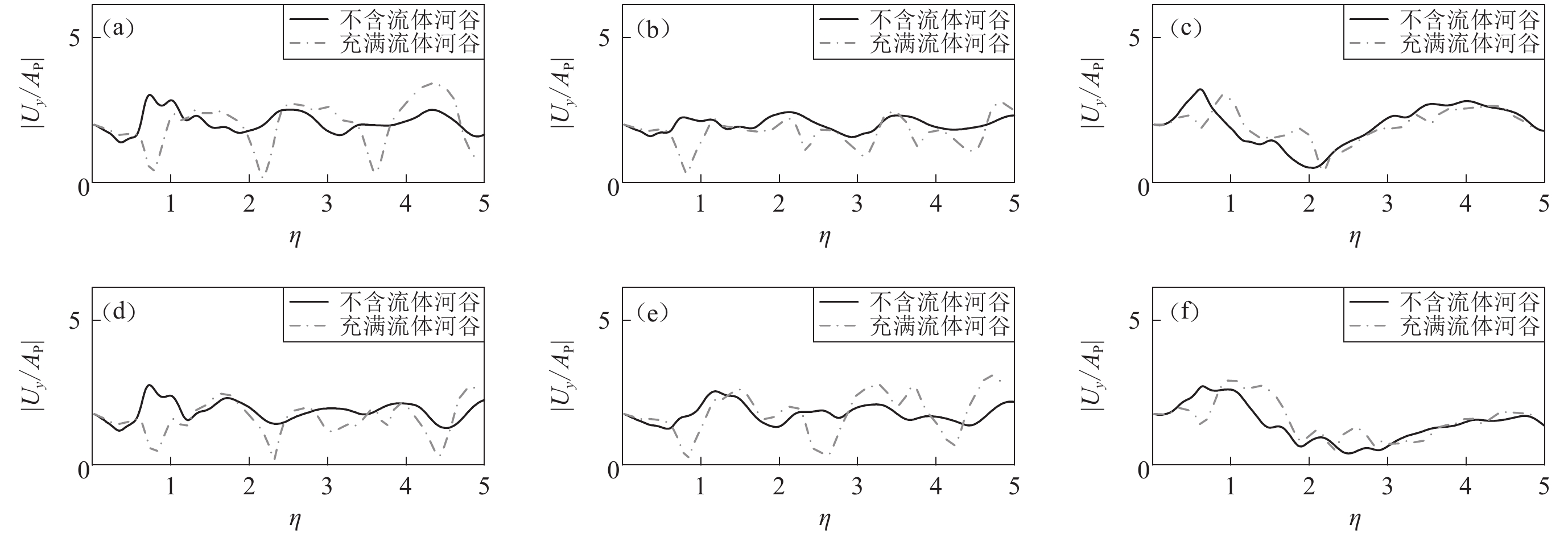
对于含流体层的河谷地形而言，流体层的存在会对峡谷地形的地震波散射产生很大的影响，影响因素主要包括：入射波的类型、角度、频率以及流体深度。图4，5给出了在不同影响因素作用下，平面P波、SV波入射时充满流体层（h/r＝1）的河谷地形和不含流体的河谷地形表面主方向位移放大系数对比。入射波角度取θα＝0°，30°；θβ＝0°，30°。
![]()
图 4 平面P波入射下不含流体的河谷与充满流体的河谷竖向位移放大系数对比Figure 4. Comparison of vertical displacement amplification factors between the valley without fluid and the valley full of fluid induced by plane P waves（a） η＝0.5，θα＝0°；（b） η＝2.0，θα＝0°；（c） η＝5.0，θα＝0°；（d） η＝0.5，θα＝30°；（e） η＝2.0，θα＝30°；（f） η＝5.0，θα＝30°4.1.1 流体对河谷附近地震动响应单频分析
This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
总体来看，与不含流体层的峡谷地形相比，充满流体的河谷场地对地表地震动存在不同程度的缩幅效应。例如P波入射θα＝0°，η＝0.5时，在x/a＝2处，不含流体的峡谷地形的竖向位移放大系数为2.66，含流体层的河谷地形的竖向位移放大系数仅为2.03，降低了约24% （图4）；SV波入射θβ＝0°，η＝0.5时，在x/a＝2处，不含流体的峡谷地形的竖向位移放大系数为2.74，含流体层的河谷地形的竖向位移放大系数仅为2.02，降低了约26% （图5）。
![]()
图 5 平面SV波入射下不含流体的河谷与充满流体的河谷水平位移放大系数对比Figure 5. Comparison of horizontal displacement amplification factors between the valley without fluid and the valley full of fluid induced by plane SV waves（a） η＝0.5，θα＝0°；（b） η＝2.0，θα＝0°；（c） η＝5.0，θα＝0°；（d） η＝0.5，θα＝30°；（e） η＝2.0，θα＝30°；（f） η＝5.0，θα＝30°由图4—5可以看出，由于流体可吸收地震波中的纵波能量，使地震波的散射作用减弱，地表位移震荡较平缓。考虑入射角度的影响，与垂直入射相比，斜入射时，河谷左侧（靠近波入射方向）位移比右侧（远离波入射方向）位移震荡剧烈且幅值较大。
由图4—5还可以看出，与斜入射（θ＝30°）相比，垂直入射（θ＝0°）流体对河谷地形地震动响应的影响较大，位移放大系数的放大与减小效应更加明显。
4.1.2 流体对河谷地形地震动响应频谱分析
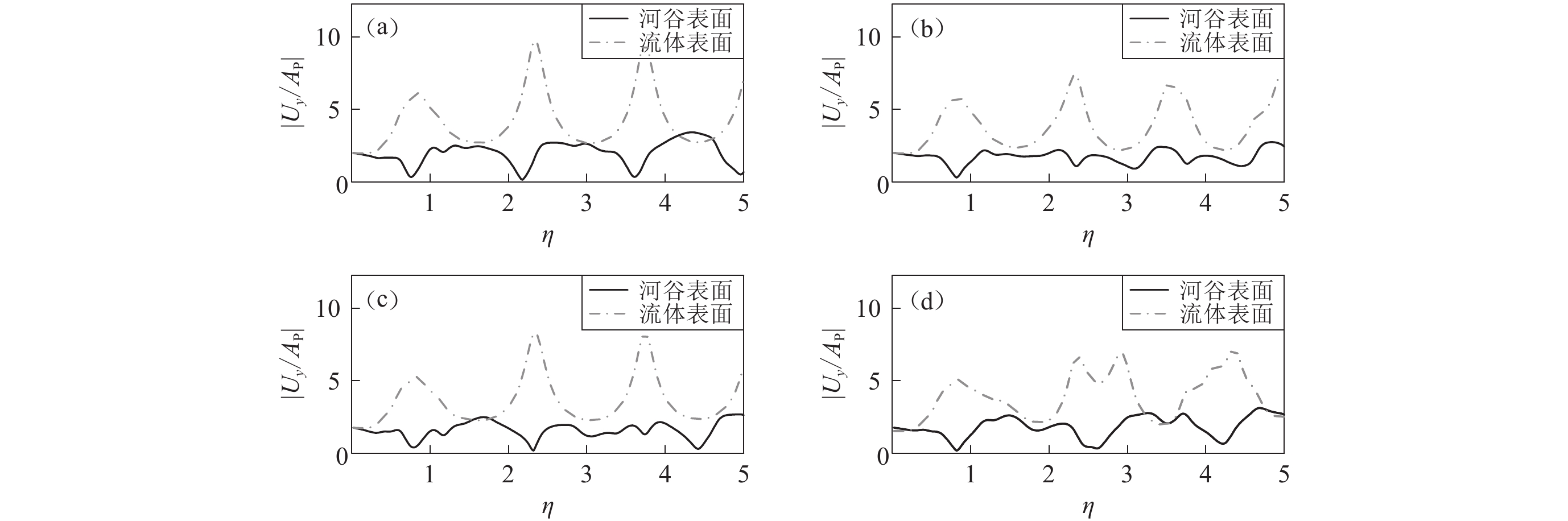
图6为充满流体层的河谷底部与流体表面位移放大系数谱对比，图7，8为不含流体层的河谷场地和含流体层的河谷场地地表典型观察点处的位移放大系数谱，图中选取了x/a＝0，0.5 （河谷底部）和1.5 （右侧水平地表）三个典型点位作为观察点。入射波无量纲频率η＝0—5，入射角度取θα＝0°，30°；θβ＝0°，30°。
![]()
图 6 平面P波入射下充满流体的河谷表面和流体表面位移放大系数谱Figure 6. Displacement amplification factor spectrum of the valley surface with full of fluid and that of the fluid surface induced by plane P waves（a） x/a＝0，θα＝0°；（b） x/a＝0.5，θα＝0°；（c） x/a＝0，θα＝30°；（d） x/a＝0.5，θα＝30°![]()
图 7 平面P波入射下不含流体的河谷与充满流体的河谷竖向位移放大系数谱Figure 7. Amplification factor spectrum of vertical displacement for the valley without fluid and the valley full of fluid induced by plane P waves（a） x/a＝0，θα＝0°；（b） x/a＝0.5，θα＝0°；（c） x/a＝1.5，θα＝0°；（d） x/a＝0，θα＝30°；（e） x/a＝0.5，θα＝30°；（f） x/a＝1.5，θα＝30°![]()
图 8 平面SV波入射下不含流体的河谷与充满流体的河谷水平位移放大系数谱Figure 8. Amplification factor spectrum of horizontal displacement for the valley without fluid and the valley full of fluid induced by plane SV waves（a） x/a＝0，θα＝0°；（b） x/a＝0.5，θα＝0°；（c） x/a＝1.5，θα＝0°；（d） x/a＝0，θα＝30°；（e） x/a＝0.5，θα＝30°；（f） x/a＝1.5，θα＝30°P波入射时，在低频域内（η＜0.5），含流体河谷底部及附近地表的地震动反应与不含流体的河谷反应基本一致。随着入射频率的增大，河谷底部观察点的位移反应特性发生改变，在共振频率处河谷底部位移缩小效应显著，可缩减至接近于0 （图7）。例如，P波垂直入射时，在河谷-流体体系一阶（η＝0.75）、二阶（η＝2.2）和三阶（η＝3.6）共振频率处，河谷底部（x/a＝0）竖向位移分别为0.32，0.13，0.34。P波斜入射和其它点位也表现出这一特性。观察图6可以看出，河谷底部位移最小频率处刚好流体表面位移达到最大，这是因为在该频率下大量地震波动能量进入流体层内，对下部河谷波动反而产生了抑制作用。因此在某些频段，流体层的存在对其下部地层具有明显的减震效应。
SV波入射时，随着入射频率的增大，水层体系共振频率增多，在共振频率处，位移频谱曲线震荡更为剧烈。在河谷外部附近地表观察点处地震动反应主要表现为位移放大系数的减小。
4.2 含流体层河谷地形中流体深度对平面P波、SV波散射的影响
This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
![]()
图 9 平面P波入射下流体深度不同时河谷表面的竖向位移放大系数Figure 9. Vertical displacement amplification factor of the valley surface with different fluid depth induced by P waves（a） η＝0.5，θα＝0°；（b） η＝2.0，θα＝0°；（c） η＝5.0，θα＝0°；（d） η＝0.5，θα＝30°；（e） η＝2.0，θα＝30°；（f） η＝5.0，θα＝30°![]()
图 10 平面SV波入射下流体深度不同时河谷表面的水平位移放大系数Figure 10. Horizontal displacement amplification factor of the valley surface with different fluid depth induced by plane SV waves（a） η＝0.5，θα＝0°；（b） η＝2.0，θα＝0°；（c） η＝5.0，θα＝0°；（d） η＝0.5，θα＝30°；（e） η＝2.0，θα＝30°；（f） η＝5.0，θα＝30°This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
流体深度对P波入射时地震动的影响较大，对SV波入射时地震动的影响较小。P波入射时，流体层的深度对河谷附近地表的位移放大系数影响显著，而对河谷内部位移放大系数影响较小。
5. 结论
本文采用间接边界元方法，求解了含流体层河谷地形对平面P波、SV波的散射，定性和定量地讨论了地震波入射时流体层对河谷地震放大效应的影响规律和地震动空间分布特征。主要结论如下：
1） 流体层具有吸收地震波能量的作用。整体上看，流体深度越大，河谷表面及附近地表的地震动幅值越小，流体深度对P波入射动力反应比对SV波入射情况的影响要更为显著；
2） 当P波入射频率与河谷中流体层的体系共振频率接近时，大量地震能量进入流体层，流体层表面位移放大最为显著，但河谷由于受到流体层的抑制作用，其底部位移放大系数明显降低；
3） 与斜入射（θα＝30°）相比，P波垂直入射（θα＝0°）时，流体对河谷地形地震动响应的影响较大，位移放大系数的放大或减小效应更加明显。
含流体层河谷地形附近地震动空间变化十分显著，因此在河谷中修建水库、桥梁等重要工程时，有必要考虑流体-河谷局部场地效应以更科学地确定地震动参数。
-
![]()
图 1 含流体层沉积河谷对地震波的散射计算模型
（a） 含流体河谷计算模型；（b） 单元离散
Figure 1. Calculation model of seismic wave scattering by sedimentary valley with fluid layer
（a） Calculation model of the valley with fluid layer；（b） Element discretization
![]()
图 3 平面SV波入射下本文含水河谷退化位移与文献结果对比 （引自Sánchez-Sesma，Campillo，1991）
Figure 3. Comparison between the degradation displacement of water bearing valley of this paper and the results of literature induced by plane SV waves （after Sánchez-Sesma，Campillo，1991）
（a） η＝2.0，θβ＝0°；（b） η＝2.0，θβ＝30°
![]()
图 4 平面P波入射下不含流体的河谷与充满流体的河谷竖向位移放大系数对比
Figure 4. Comparison of vertical displacement amplification factors between the valley without fluid and the valley full of fluid induced by plane P waves
（a） η＝0.5，θα＝0°；（b） η＝2.0，θα＝0°；（c） η＝5.0，θα＝0°；（d） η＝0.5，θα＝30°；（e） η＝2.0，θα＝30°；（f） η＝5.0，θα＝30°
![]()
图 5 平面SV波入射下不含流体的河谷与充满流体的河谷水平位移放大系数对比
Figure 5. Comparison of horizontal displacement amplification factors between the valley without fluid and the valley full of fluid induced by plane SV waves
（a） η＝0.5，θα＝0°；（b） η＝2.0，θα＝0°；（c） η＝5.0，θα＝0°；（d） η＝0.5，θα＝30°；（e） η＝2.0，θα＝30°；（f） η＝5.0，θα＝30°
![]()
图 6 平面P波入射下充满流体的河谷表面和流体表面位移放大系数谱
Figure 6. Displacement amplification factor spectrum of the valley surface with full of fluid and that of the fluid surface induced by plane P waves
（a） x/a＝0，θα＝0°；（b） x/a＝0.5，θα＝0°；（c） x/a＝0，θα＝30°；（d） x/a＝0.5，θα＝30°
![]()
图 7 平面P波入射下不含流体的河谷与充满流体的河谷竖向位移放大系数谱
Figure 7. Amplification factor spectrum of vertical displacement for the valley without fluid and the valley full of fluid induced by plane P waves
（a） x/a＝0，θα＝0°；（b） x/a＝0.5，θα＝0°；（c） x/a＝1.5，θα＝0°；（d） x/a＝0，θα＝30°；（e） x/a＝0.5，θα＝30°；（f） x/a＝1.5，θα＝30°
![]()
图 8 平面SV波入射下不含流体的河谷与充满流体的河谷水平位移放大系数谱
Figure 8. Amplification factor spectrum of horizontal displacement for the valley without fluid and the valley full of fluid induced by plane SV waves
（a） x/a＝0，θα＝0°；（b） x/a＝0.5，θα＝0°；（c） x/a＝1.5，θα＝0°；（d） x/a＝0，θα＝30°；（e） x/a＝0.5，θα＝30°；（f） x/a＝1.5，θα＝30°
![]()
图 9 平面P波入射下流体深度不同时河谷表面的竖向位移放大系数
Figure 9. Vertical displacement amplification factor of the valley surface with different fluid depth induced by P waves
（a） η＝0.5，θα＝0°；（b） η＝2.0，θα＝0°；（c） η＝5.0，θα＝0°；（d） η＝0.5，θα＝30°；（e） η＝2.0，θα＝30°；（f） η＝5.0，θα＝30°
![]()
图 10 平面SV波入射下流体深度不同时河谷表面的水平位移放大系数
Figure 10. Horizontal displacement amplification factor of the valley surface with different fluid depth induced by plane SV waves
（a） η＝0.5，θα＝0°；（b） η＝2.0，θα＝0°；（c） η＝5.0，θα＝0°；（d） η＝0.5，θα＝30°；（e） η＝2.0，θα＝30°；（f） η＝5.0，θα＝30°
表 1 含流体层河谷地形对平面P波、SV波的地震响应计算参数
Table 1 Calculation parameters of seismic response of valley terrain with fluid layer for plane P wave and SV wave
P波波速cα/（m·s−1） SV波波速cβ/（m·s−1） 密度ρ/（kg·m−3） 空气 330 — 1.29 流体 1 501 — 1 000 弹性土体 2 670 1 090 2 200 
下载: 导出CSV
-
陈少林,张莉莉,李山有. 2014. 半圆柱型沉积盆地对SH波散射的数值分析[J]. 工程力学,31(4):218–224. doi: 10.6052/j.issn.1000-4750.2012.11.0819 Chen S L,Zhang L L,Li S Y. 2014. Numerical analysis of the plane SH waves scattering by semi-cylindrical alluvial valley[J]. Engineering Mechanics,31(4):218–224 (in Chinese).
杜修力,雷枝,李亮,王进廷. 2015. 地震和波浪联合作用下自由场海水动水压力分析[J]. 世界地震工程,31(3):1–9. Du X L,Lei Z,Li L,Wang J T. 2015. Hydrodynamic pressures analysis of free field seawater under coactions of earthquake and wave[J]. World Earthquake Engineering,31(3):1–9 (in Chinese).
李伟华,赵成刚. 2006. 具有饱和土沉积层的充水河谷对平面波的散射[J]. 地球物理学报,49(1):212–224. doi: 10.3321/j.issn:0001-5733.2006.01.028 Li W H,Zhao C G. 2006. Scattering of plane waves by circular-arc alluvial valleys with saturated soil deposits and water[J]. Chinese Journal of Geophysics,49(1):212–224 (in Chinese).
李伟华. 2010. 考虑水—饱和土场地—结构耦合时的沉管隧道地震反应分析[J]. 防灾减灾工程学报,30(6):607–613. doi: 10.3969/j.issn.1672-2132.2010.06.004 Li W H. 2010. Seismic response analysis of immersed tube tunnel considering the dynamic interactions between water,stratum and structure influence of liquid parameters on the damage of buried pipeline[J]. Journal of Disaster Prevention and Mitigation Engineering,30(6):607–613 (in Chinese).
梁建文,梁佳利,张季,巴振宁. 2017. 深厚软土场地中三维凹陷地形非线性地震响应分析[J]. 岩土工程学报,39(7):1196–1205. doi: 10.11779/CJGE201707005 Liang J W,Liang J L,Zhang J,Ba Z N. 2017. Nonlinear seismic response of 3D canyon in deep soft soils[J]. Chinese Journal of Geotechnical Engineering,39(7):1196–1205 (in Chinese).
刘中宪,于琴,何颖. 2017. 岩土介质随机性对沉积河谷地震动放大效应的影响[J]. 地震学报,39(5):764–777. doi: 10.11939/jass.2017.05.011 Liu Z X,Yu Q,He Y. 2017. Effect of randomness of geotechnical medium on the seismic ground motion amplification effect of a sedimentary valley[J]. Acta Seismologica Sinica,39(5):764–777 (in Chinese).
王进廷,金峰,张楚汉. 2003. 位于弹性半空间上的理想流体层动力反应—平面P波入射[J]. 工程力学,20(6):12–175. doi: 10.3969/j.issn.1000-4750.2003.06.003 Wang J T,Jin F,Zhang C H. 2003. Dynamic response of ideal fluid layer overlying elastic half-space due to P-wave incidence[J]. Engineering Mechanics,20(6):12–175 (in Chinese).
王进廷,张楚汉,金峰. 2004. 位于弹性半空间上的理想流体层动力反应—平面SV波入射[J]. 工程力学,21(1):15–20. doi: 10.3969/j.issn.1000-4750.2004.01.003 Wang J T,Zhang C H,Jin F. 2004. Dynamic response of ideal fluid layer overlying elastic half-space due to SVP wave incidence[J]. Engineering Mechanics,21(1):15–20 (in Chinese).
张奎,李伟华,赵成刚. 2018. 平面波入射下深水地基场地动力响应分析[J]. 岩土工程学报,40(6):1066–1074. doi: 10.11779/CJGE201806012 Zhang K,Li W H,Zhao C G. 2018. Dynamic responses of an underwater site subjected to plane P- or SV- wave incidence[J]. Chinese Journal of Geotechnical Engineering,40(6):1066–1074 (in Chinese).
张宁,高玉峰,何稼,徐婕,陈欣,代登辉. 2017. 平面 SH 波作用下部分充填圆弧形沉积谷的二维土层和地形放大效应[J]. 地震学报,39(5):778–797. doi: 10.11939/jass.2017.05.012 Zhang N,Gao Y F,He J,Xu J,Chen X,Dai D H. 2017. Two-dimensional soil and topographic amplification effects of a partially filled circular-arc alluvial valley under plane SH waves[J]. Acta Seismologica Sinica,39(5):778–797 (in Chinese).
赵成刚,王磊,李伟华. 2008. 具有饱和土沉积层的充水河谷对平面瑞雷波的散射[J]. 地球物理学报,51(5):1567–1573. doi: 10.3321/j.issn:0001-5733.2008.05.032 Zhao C G,Wang L10.3321/j.issn:0001-5733.2008.05.032,Li W H 2008. Scattering of plane Rayleigh waves by circular-arc alluvial valleys with saturated soil deposits and water layer[J]. Chinese Journal of Geophysics,51(5):1567–1573 (in Chinese).
周国良,李小军,侯春林,李铁萍. 2012. SV波入射下河谷地形地震动分布特征分析[J]. 岩土力学,33(4):1161–1166. doi: 10.3969/j.issn.1000-7598.2012.04.029 Zhou G L,Li X J,Hou C L,Li T P. 2012. Characteristic analysis of ground motions of canyon topography under incident SV seismic waves[J]. Rock and Soil Mechanics,33(4):1161–1166 (in Chinese).
Alejandro Rodríguez-Castellanos A,Víctor Martínez-Calzada V,Rodríguez-Sánchez J E,Orozco-del-Castillo M,Carbajal-Romero M. 2014. Induced water pressure profiles due to seismic motions[J]. Appl Ocean Res,47:9–16. doi: 10.1016/j.apor.2014.03.004
Carbajal-Romero M,Flores-Mendez E,Flores-Guzmán N,Núñez-Farfán J,Olivera-Villaseñor J,Sánchez-Sesma F J. 2013. Scholte waves on fluid-solid interfaces by means of an integral formulation[J]. Geofis Int,52(1):21–30.
Liu Z X,Liang J W,Wu C Q. 2016. The diffraction of Rayleigh waves by a fluid-saturated alluvial valley in a poroelastic half-space modeled by MFS[J]. Comput Geosci-UK,91:33–48. doi: 10.1016/j.cageo.2016.03.007
Sánchez-Sesma F J,Campillo M. 1991. Diffraction of P,SV,and Rayleigh waves by topographic features:A boundary integral formulation[J]. Bull Seismol Soc Am,81(6):2234–2253.
Wang J T,Zhang C H,Jin F. 2004. Analytical solutions for dynamic pressures of coupling fluid-solid-porous medium due to P wave incidence[J]. Earthq Eng Eng Vib,3(2):263–271. doi: 10.1007/BF02858240
Wang J T,Zhang C H,Jin F. 2009. Analytical solutions for dynamic pressures of coupling fluid-porous medium-solid due to SV wave incidence[J]. Int J NumerAnal Met,33(12):1467–1484. doi: 10.1002/nag.773
Zhang C,Liu Q J,Deng P. 2017. Surface motion of a half-space with a semicylindrical canyon under P,SV,and Rayleigh waves[J]. B Seismol Soc Am,107(2):809–820. doi: 10.1785/0120160207
-
期刊类型引用(5)
1. 程喜. 饱和复杂场地效应对大跨连续梁桥地震响应影响的研究. 震灾防御技术. 2024(01): 14-23 . 
百度学术
2. 赵仕兴，罗麒锐，唐元旭，吴启红，熊峰，周巧玲，卢丹，张敏. 复杂地形地质条件下地震动放大系数研究进展. 建筑结构. 2024(19): 51-58+73 . 百度学术
3. 方志江，龚文剑，张仰鹏，焦晓东，颜志铭. 基于多种物探方法的公路下伏采空区病害识别与分析. 市政技术. 2024(10): 37-44+51 . 百度学术
4. 刘中宪，周健，李程程，孟思博. 基于修正随机有限断层法的沉积盆地地震动模拟. 防灾减灾工程学报. 2023(05): 999-1008 . 百度学术
5. 孟思博，赵嘉玮，刘中宪. 基于差分进化-人工神经网络的沉积河谷地震动放大效应预测模型. 地震学报. 2022(01): 170-181 . 本站查看
其他类型引用(1)
计量
- 文章访问数: 1091
- HTML全文浏览量: 460
- PDF下载量: 38
- 被引次数: 6
