Characteristics of seismic ambient noise in Sichuan region
-
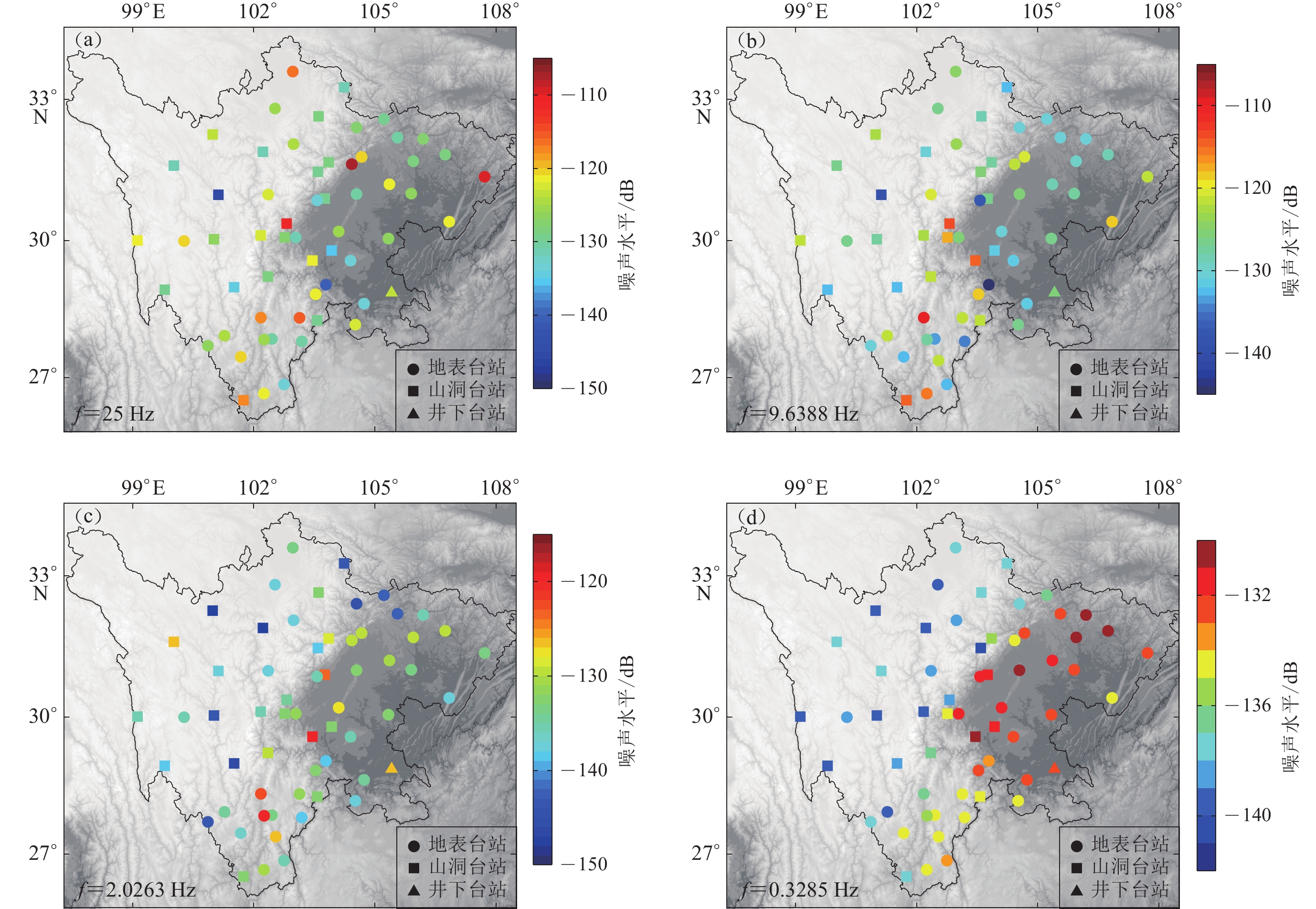
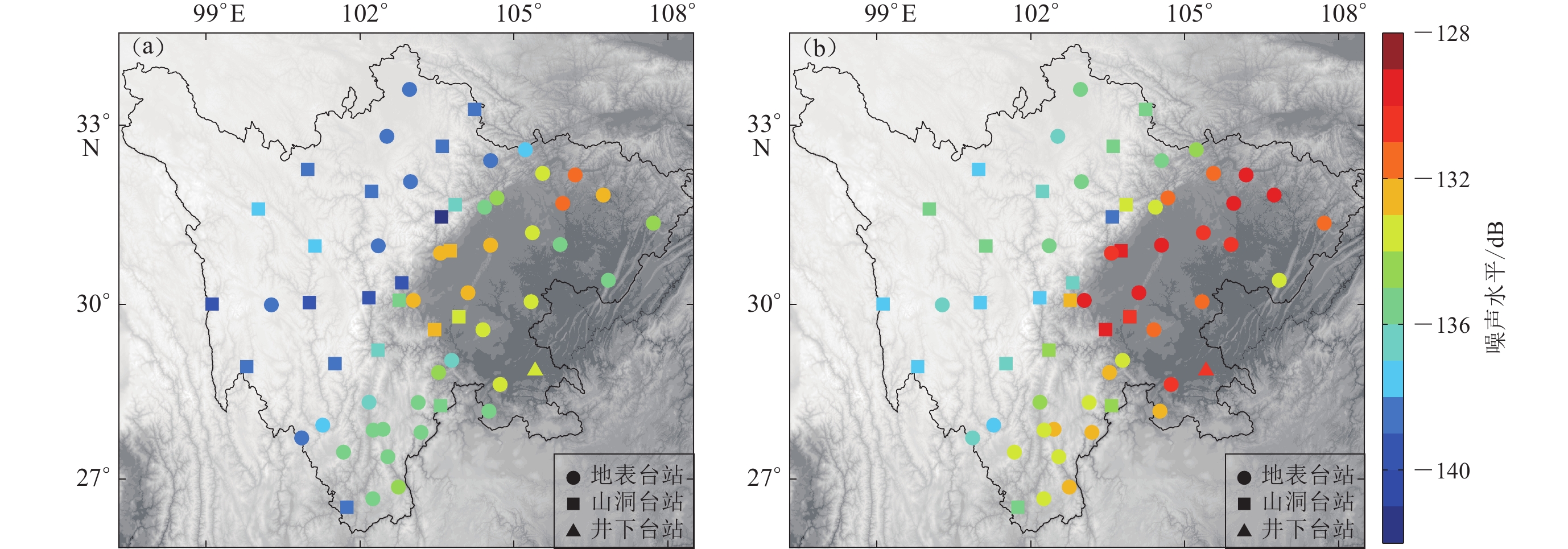
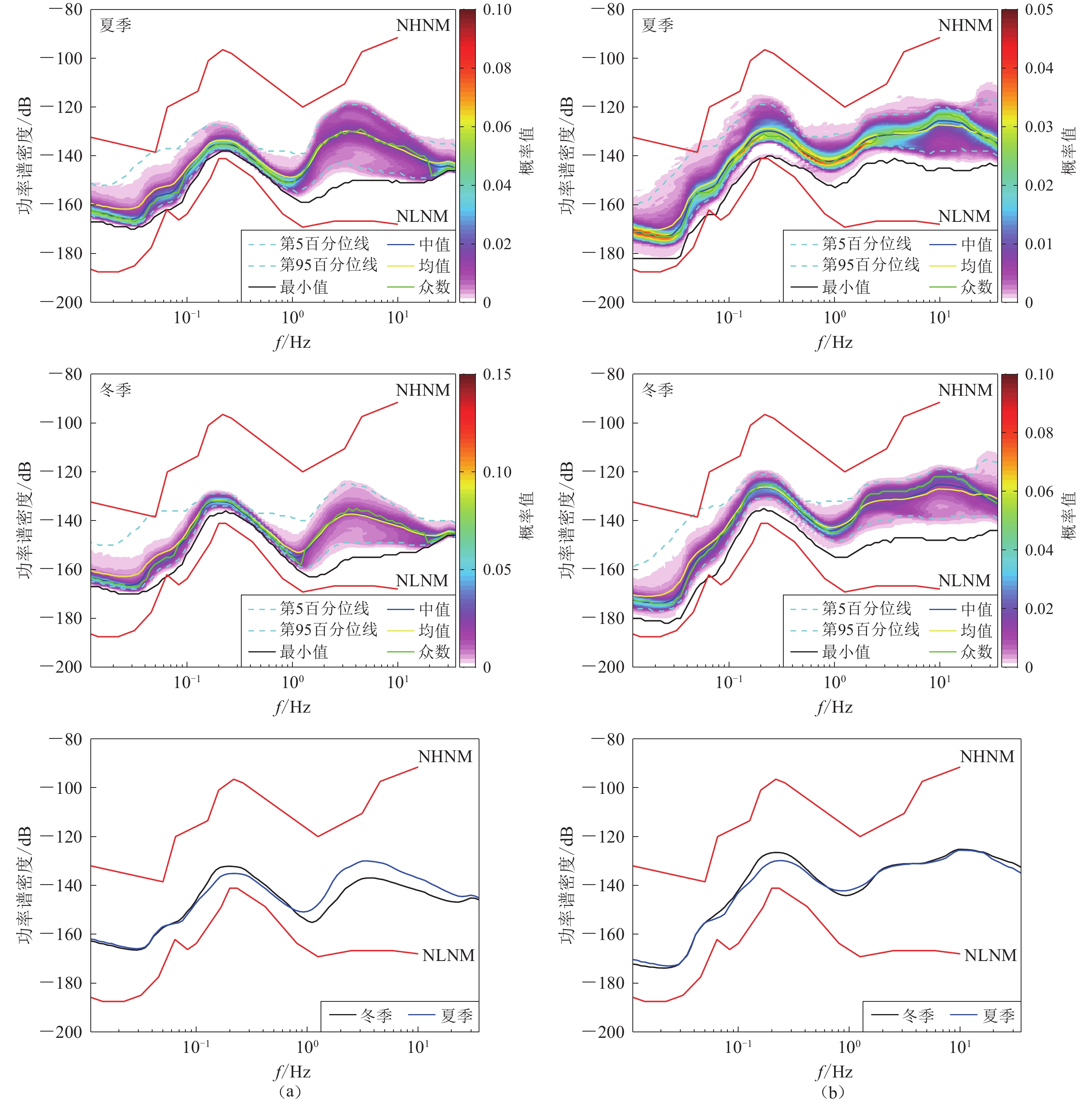
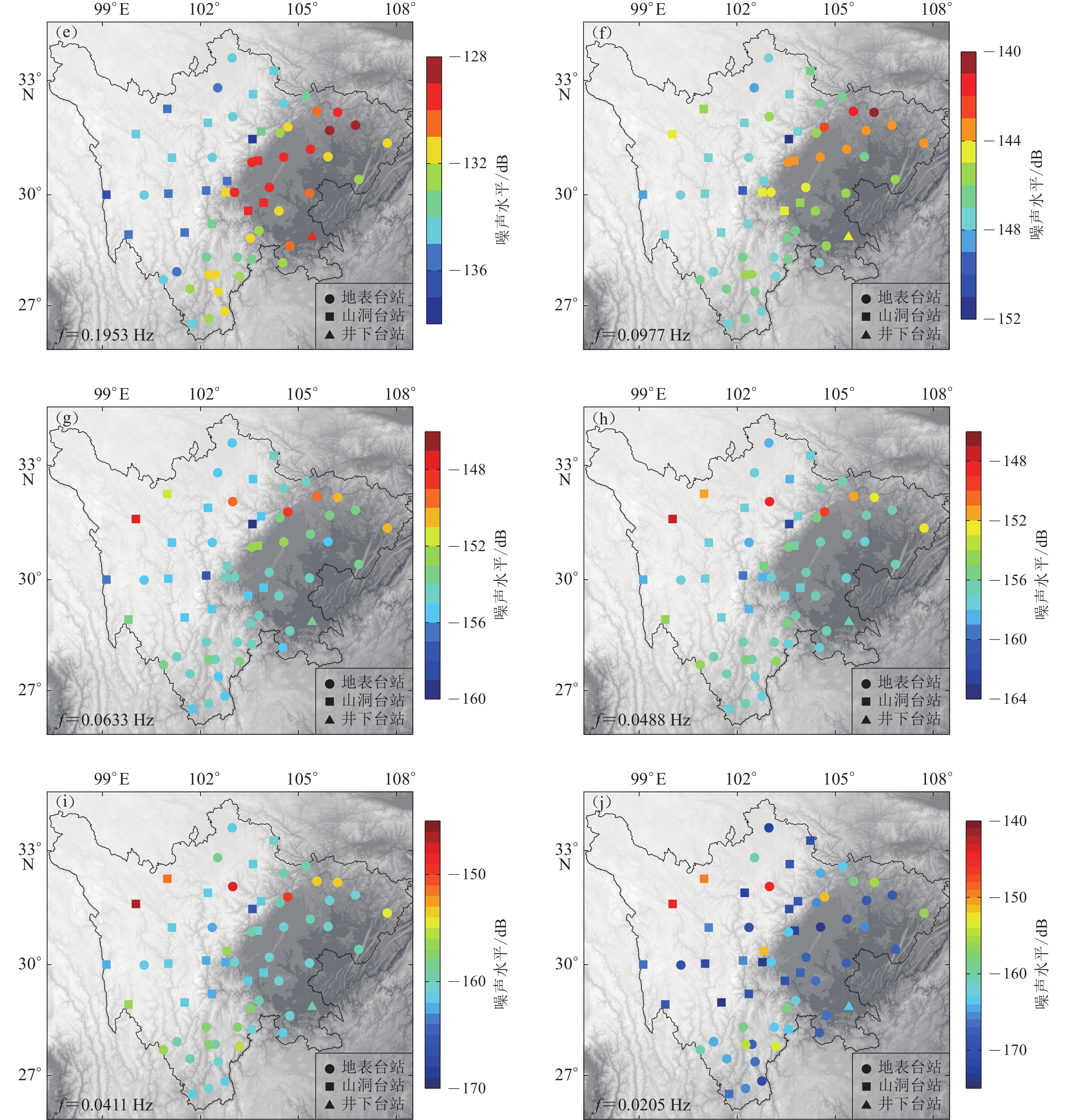
摘要: 选取四川省数字测震台网2015年1月1日至2018年12月31日期间60个固定台站的三分量连续波形记录，计算了台站噪声加速度功率谱密度及相应的概率密度函数分布，统计了不同频率下的噪声功率谱密度值分布，对不同区域、不同频率下背景噪声水平的变化特征予以分析。结果表明：大部分地震台站的高频段噪声由于受到台站附近人为的、规律的作息生活和生产方式的影响，呈现明显的季节性变化和日变化，即夏季噪声水平升高，冬季降低，在农历春节期间达到全年最低值，地理空间分布特征不明显；第二类地脉动冬季噪声水平升高，夏季降低，季节性变化明显，平均变化为1—5 dB，且冬季峰值出现的频率向长周期方向移动1—2 s，呈现明显的地理空间分布特征，川东地区平均噪声水平最高，攀西地区次之，川西高原最低；与第二类地脉动相比，第一类地脉动观测到的噪声能量较弱，季节性变化不明显，地理空间分布的噪声水平差异明显减小；在20 s以上的长周期部分，台站噪声未呈明显的季节性和地理空间分布差异。此外，将地震计安置在山洞和井下，可以有效地降低台站周围干扰源、温度和压强对高频段和长周期观测的影响，噪声水平低于地表安装方式。Abstract: Based on the three-component continuous waveform data recorded by sixty permanent seismic stations in Sichuan seismic network from January 1, 2015 to December 31, 2018, this paper calculated the noise power spectral densities and corresponding probability density functions, then gave the statistical characteristics of noise power spectral density at different frequencies, and finally analyzed the characteristics of noise level at different regions and frequencies. The results show that the high-frequency seismic noises of most stations are affected by the nearby human activities, production mode and lifestyle, which has obvious seasonal and diurnal variations. The noise level increases during summer and decreases during winter with the lowest level during the Spring Festival in the whole year; and the geographical distribution is not obvious. For double-frequency microseisms, the noise level increases during winter and decreases during summer, and has obvious seasonal variation with an average of 1−5 dB, which has obvious geographical distribution characteristics. The average noise level in eastern Sichuan is the highest, followed by Panxi region, and the lowest in western Sichuan Plateau. The microseism peaks have different amplitudes and occur at different frequencies in summer and winter, with the peaks shifted by 1−2 s toward longer periods in the winter. Compared with the double-frequency microseism band, the noise energy at primary microseism band is weaker, the seasonal variation is not obvious, and the difference of noise level in geographical distribution is significantly reduced. While the long-period （＞20 s） noise level has no obvious seasonal variation and no difference in geographical distribution. In addition, installing seismographs in caves and borehole can effectively reduce the influence of noise sources, temperature and pressure on high-frequency band and long-period observations, therefore the noise level is lower than that of shallow installations.
-
Keywords:
- Sichuan region /
- ambient noise /
- noise level /
- probability density function
-
引言
确定输入地震波是结构抗震工程领域进行时程动力分析过程中的一个重要课题，目前国内外使用比较广泛的方法是根据设计反应谱来拟合匹配的人工地震波，将其作为抗震设计的输入地震波。为了满足某些特定的地震动工程特性，如时域、频域信息等，按照一定的数值算法合成地震动时程的过程即为地震动的反应谱拟合。
反应谱拟合分为时域法和频域法两种（刘帅等，2018）。时域法是通过时域内某个特定点的脉冲来调整反应谱上某点发生的最大位移，例如使用窄带时程叠加法（赵凤新，张郁山，2007；何佳，王海涛，2010）或小波变换来拟合地震动（白泉等，2015；Cecini，Palmeri，2015；谢皓宇等，2019），以及使用Broyden算法的地震动拟合方法（Adekristi，Eatherton，2015）。时域法更多是在已有某地震动的条件下，通过修正地震波的时域分量使其反应谱向设计谱逼近，而频域法则不需要自然波或者其它地震波作为必要条件，仅通过一个随机相位谱即可生成人工地震波（陈永祁等，1981；Gupta，2002），因此频域法拟合人工地震波的随机性高于时域法。
然而，传统的频域法拟合人工地震动在计算过程中并未区别傅里叶谱各频率分量对最大反应的贡献为正或负，也未涉及随机相位谱对于拟合结果的影响，这造成了算法的迭代效率偏低、拟合的频域顽固点较多等问题。因此，亟需研发一种改进的人工地震波拟合方法。
鉴于此，本文在传统的频域法拟合人工地震动的基础上，提出考虑每次迭代的相关性，区别傅里叶谱各频率分量对最大反应的贡献的正负，并对随机相位谱进行修正的一种新的综合方法，以期提高拟合精度，加快计算速度。
1. 频域法拟合人工地震波
频域法的基本原理是通过具有随机相位谱的一组三角函数的叠加来构造一个近似的平稳高斯过程，再乘以一个等时程的包络函数，最终得到一个非平稳的加速度时程（杨庆山，姜海鹏，2002）。频域内拟合人工地震波并使用迭代调整幅值谱的方法主要包括以下几部分：
1） 使用单阻尼反应谱与频谱（功率谱密度函数）之间的近似转换关系（Kaul，1978），将目标反应谱转换为相应的功率谱密度函数。通常使用的近似转换关系为
$ {S_{{{\!\!\ddot x}_0}}}\!\!\!\!\!{\text{（}}{{\omega _k}} {\text{）}}\!\!\!\! {\text{＝}} \frac{\textit{ξ} }{{{\rm{\pi }}{\omega _k}}}[S_{\rm{a}}^{\rm{T}}\!\!\!\!{\text{（}}{{\omega _k}} {\text{）}}\!\!\!\!]^2 \cdot {\left\{ { {\text{－}} \ln \left[ {\frac{{ {\text{－}} {\rm{\pi }}}}{{{\omega _k}T}}\ln \!\!\!\!{\text{（}}{1 {\text{－}} \gamma } {\text{）}}\!\!\!\!} \right]} \right\}^{ {\text{－}} 1}} {\text{，}} $
(1) This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
2） 将得到的功率谱密度转化为傅里叶幅值谱：
$ {A}^{2}\!\!\!\!{\text{（}}{\omega }_{k}{\text{）}}\!\!\!\!{\text{＝}} 4{S}_{{ \!\!\ddot{x}}_{0}}\!\!\!\!\!\!{\text{（}}{\omega }_{k}{\text{）}}\!\!\!\!\cdot \Delta \omega {\text{，}} $
(2) 式中，A（ωk）为傅里叶幅值谱，Δω为频域采样间隔。
3） 基于通过步骤2）计算所得的傅里叶幅值谱，再引入随机相位谱，使用三角函数的叠加或者快速傅里叶逆变换将得到的傅里叶幅值谱转换为零均值的平稳高斯过程：
$ {\tilde x}_{0}\!\!\!\!{\text{（}}t{\text{）}}\!\!\!\!{\text{＝}} \sum\limits_{k {\text{＝}} 1}^N A\!\!\!\!{\text{（}}{\omega }_{k}{\text{）}}\!\!\!\!\sin\!\!\!\!{\text{（}}{\omega }_{k}t {\text{＋}}{\varphi }_{k}{\text{）}}\!\!\!\!{\text{，}} $
(3) $ {{\tilde x}_0}\!\!\!\!{\text{（}}t {\text{）}}\!\!\!\! {\text{＝}} {\rm{FF}}{{\rm{T}}^{ {\text{－}} 1}}\left[ {A\!\!\!\!{\text{（}}{{\omega _k}} {\text{）}}\!\!\!\!{{\rm{e}}^{{\rm{i}}{\varphi _k}}}} \right]{\text{，}} $
(4) This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
式（3）与式（4）所对应的两种方法最后的合成结果几乎一致，不同之处在于：快速傅里叶变换的计算速度更快，但对反应谱采样和插值有一定要求；利用三角函数叠加的计算速度较慢，但计算过程中的限制更少。
This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
$ {\ddot{x}}_{{\rm{a}}}\!\!\!\!{\text{（}}t{\text{）}}\!\!\!\! {\text{＝}}I\!\!\!\!{\text{（}}t{\text{）}}\!\!\!\!\cdot {\tilde x}_{0}\!\!\!\!{\text{（}}t{\text{）}}\!\!\!\!{\text{，}} $
(5) $ I\!\!\!\!{\text{（}}t {\text{）}}\!\!\!\! {\text{＝}} \left\{ {\begin{array}{*{20}{l}} {{{\left( {{\dfrac{t}{{{t_1}}}} } \right)}^2}}&\qquad{0 {\text{＜}} t {\text{≤}} {t_1}}{\text{，}}\\ 1&\qquad{{t_1} {\text{＜}} t {\text{≤}} {t_2}}{\text{，}}\\ {\exp [{ {\text{－}} c({t {\text{－}} {t_2}} })]\!\!\!\!\!}&\qquad{{t_2} {\text{＜}} t {\text{≤}} T}{\text{，}} \end{array}} \right. $
(6) This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
$ {A}_{n {\text{＋}}1}\!\!\!\!{\text{（}}\omega_{k}{\text{）}}\!\!\!\!{\text{＝}}{A}_{n}\!\!\!\!{\text{（}}\omega_{k}{\text{）}}\!\!\!\!\cdot \frac{{S}_{{\rm{a}}}^{{\rm{T}}}\!\!\!\!{\text{（}}{\omega }_{k}{\text{）}}\!\!\!\!}{{S}_{{\rm{a}}n}\!\!\!\!{\text{（}}{\omega }_{k}{\text{）}}\!\!\!\!}{\text{，}} $
(7) This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
传统的频域法存在两个明显的问题：其一，在迭代过程中，存在迭代次数增加却无法提高收敛精度的频率控制点，这些点被称为顽固点，这些顽固点对应的傅里叶频率分量可能对于该频率反应谱值的收敛并非正贡献，若此时继续按照式（7）进行迭代，则无法使得顽固点收敛，甚至会产生发散的效果；其二，该方法对于相位谱未作任何约束，然而随机反应谱的选取对最终的拟合程度影响很大（胡聿贤，何训，1986；瞿希梅，吴知丰，1995）。基于以上问题，本文提出改进的综合方法，基于传统频域法进行修正，考虑迭代过程中频率分量是否正相关，同时考虑修改随机相位谱，以期达到更好的收敛效果。
2. 考虑迭代相关的反应谱拟合
This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
$ a\!\!\!\!{\text{（}}{\omega }_{k}{\text{，}}\!\!\!\!\!t{\text{）}}\!\!\!\!\cdot {a}_{{\omega }_{k}}\!\!\!\!{\text{（}}{\omega }_{k}{\text{，}}\!\!\!\!t{\text{）}}\!\!\!\! {\text{＜}} 0{\text{，}} $
(8) This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
$ {S}_{{\rm{a}}n}\!\!\!\!{\text{（}}{\omega }_{k}{\text{）}}\!\!\!\!{\text{＞}}{S}_{{\rm{a}}}^{{\rm{T}}}\!\!\!\!{\text{（}}{\omega }_{k}{\text{）}}\!\!\!\!{\text{，}} $
(9) $ \begin{aligned} {\ddot x_{{\rm{a}}\!\!\!\!{\text{（}}{n {\text{＋}} 1} {\text{）}}\!\!\!\!}}\!\!\!\!{\text{（}}t {\text{）}}\!\!\!\! {\text{＝}}& \sum\limits_{k {\text{＝}} 1}^N {A}_{n {\text{＋}}1} \!\!\!\!{\text{（}}\omega_k {\text{）}}\!\!\!\!{a_{{\omega _k}}}\!\!\!\!{\text{（}}{{\omega _k}{\text{，}}\!\!\!\!t} {\text{）}}\!\!\!\! \cdot I\!\!\!\!{\text{（}}t {\text{）}}\!\!\!\! {\text{＝}} \sum\limits_{k {\text{＝}} 1}^N A_n\!\!\!\!\!\!\!\! &(\omega )\frac{{S_{\rm{a}}^{\rm{T}}\!\!\!\!{\text{（}}{{\omega _k}} {\text{）}}\!\!\!\!}}{{{S_{{\rm{a}}n}}\!\!\!\!{\text{（}}{{\omega _k}} {\text{）}}\!\!\!\!}}{a_{{\omega _k}}}\!\!\!\!{\text{（}}{{\omega _k}{\text{，}}\!\!\!\!t} {\text{）}}\!\!\!\! \cdot I\!\!\!\!{\text{（}}t {\text{）}}\!\!\!\!{\text{，}} \end{aligned}$
(10) This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
${S_{{\rm{a}}\!\!\!\!{\text{（}}{n {\text{＋}} 1} {\text{）}}\!\!\!\!}}\!\!\!\!{\text{（}}{{\omega _k}} {\text{）}}\!\!\!\! {\text{＝}} {\omega _k}{\left| {\int _0^T {{\ddot x}_{{\rm{a}}\!\!\!\!{\text{（}}{n {\text{＋}} 1} {\text{）}}\!\!\!\!}}\!\!\!\!{\text{（}}\tau {\text{）}}\!\!\!\! \cdot {\rm{exp}}\left[{ {\text{－}} \xi {\omega _k}\!\!\!\!{\text{（}}{T {\text{－}} \tau } {\text{）}}\!\!\!\!} \right] \sin {\omega _k}\!\!\!\!{\text{（}}{T {\text{－}} \tau } {\text{）}}\!\!\!\!{\rm d}\tau } \right|_{\rm max}}$
(11) $ {S}_{{\rm{a}}(n {\text{＋}}1)}\!\!\!\!{\text{（}}{\omega }_{k}{\text{）}}\!\!\!\!{ {\text{＞}}S}_{{\rm{a}}n}\!\!\!\!{\text{（}}{\omega }_{k}{\text{）}}\!\!\!\! {\text{＞}}{S}_{{\rm{a}}}^{{\rm{T}}}\!\!\!\!{\text{（}}{\omega }_{k}{\text{）}}\!\!\!\! $
(12) This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
$ {A}_{n{\text{＋}}1}\!\!\!\!{\text{（}}\omega {\text{）}}\!\!\!\!{\text{＝}}{A}_{n}\!\!\!\!{\text{（}}\omega_k {\text{）}}\!\!\!\! \cdot {\left({\frac{{S}_{{\rm{a}}}^{{\rm{T}}}\!\!\!\!{\text{（}}{\omega }_{k}{\text{）}}\!\!\!\!}{{S}_{{\rm{a}}n}\!\!\!\!{\text{（}}{\omega }_{k}{\text{）}}\!\!\!\!}}\right)}^{c}{\text{，}} $
(13) $ c {\text{＝}} \left\{ {\begin{array}{*{20}{l}} 1&\quad{a\!\!\!\!{\text{（}}{{\omega _k}{\text{，}}\!\!\!t} {\text{）}}\!\!\!\! \cdot {a_{{\omega _k}}}\!\!\!\!{\text{（}}{{\omega _k}{\text{，}}\!\!\!t} {\text{）}}\!\!\!\! {\text{＞}} 0}{\text{，}}\\ { {\text{－}} 1}&\quad{a\!\!\!\!{\text{（}}{{\omega _k}{\text{，}}\!\!\!t} {\text{）}}\!\!\!\! \cdot {a_{{\omega _k}}}\!\!\!\!{\text{（}}{{\omega _k}{\text{，}}\!\!\!t} {\text{）}}\!\!\!\! {\text{＜}} 0}{\text{，}} \end{array}} \right. $
(14) 式中，c代表迭代过程中的修正系数。
3. 考虑相位谱的反应谱拟合
某频率ωk在反应谱拟合过程中为顽固点，则存在两种情况：① 合成波对应的谱加速度大于设计反应谱加速度，谱最大加速度发生的时间为t，且存在该频率对应的傅里叶分量所产生的加速度分量在该时间与谱最大加速度同向；② 合成波对应的谱值小于设计反应谱值，谱最大加速度发生的时间为t，且存在该频率对应的傅里叶分量所产生的加速度分量在该时间与谱最大加速度反向。这两种情况由式（15）描述，在此种情况下，传统的迭代方法已经无法有效地使顽固点收敛，因此考虑对相位谱进行修正，过程如下：
$\left\{ { \begin{array}{*{20}{l}} \!\!\!{{S_{{\rm{a}}n}}\!\!\!\!{\text{（}}{{\omega _k}} {\text{）}}\!\!\!\! {\text{＞}} S_{\rm{a}}^{\rm{T}}\!\!\!\!{\text{（}}{{\omega _k}} {\text{）}}\!\!\!\!}\\ \!\!\!{a\!\!\!\!{\text{（}}{{\omega _k}{\text{，}}\!\!\!t} {\text{）}}\!\!\!\! \cdot {a_{{\omega _k}}}\!\!\!\!{\text{（}}{{\omega _k}{\text{，}}\!\!\!t} {\text{）}}\!\!\!\! {\text{＞}} 0} \end{array}\;\;{\text{或}}\;\;} \right.\left\{ {\begin{array}{*{20}{l}} \!\!\!{{S_{{\rm{a}}n}}\!\!\!\!{\text{（}}{{\omega _k}} {\text{）}}\!\!\!\! {\text{＜}} S_{\rm{a}}^{\rm{T}}\!\!\!\!{\text{（}}{{\omega _k}} {\text{）}}\!\!\!\!}\\ \!\!\!{a\!\!\!\!{\text{（}}{{\omega _k}{\text{，}}\!\!\!t} {\text{）}}\!\!\!\! \cdot {a_{{\omega _k}}}\!\!\!\!{\text{（}}{{\omega _k}{\text{，}}\!\!\!t} {\text{）}}\!\!\!\! {\text{＜}} 0} \end{array}} \right. $
(15) $ {\varphi '_k} {\text{＝}} {\varphi _k} {\text{＋}} {\rm{\pi }} $
(16) This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
$ x'\!\!\!\!{\text{（}}t {\text{）}}\!\!\!\! {\text{＝}} \sin \!\!\!\!{\text{（}}{{\omega _k}t {\text{＋}} {\varphi _k} {\text{＋}} {\rm{\pi }}} {\text{）}}\!\!\!\! {\text{＝}} {\text{－}} \sin \!\!\!\!{\text{（}}{{\omega _k}t {\text{＋}} {\varphi _k}} {\text{）}}\!\!\!\! {\text{＝}} {\text{－}} x\!\!\!\!{\text{（}}t {\text{）}}\!\!\!\!{\text{，}} $
(17) $\begin{aligned} a'\!\!\!\!{\text{（}}{{\omega _k}{\text{，}}t} {\text{）}}\!\!\!\! {\text{＝}}& {\rm{F^{-1}}}\left\{ {{H\!\!\!\!{\text{（}}\omega {\text{）}}\!\!\!\! \cdot {\rm{F}}\left[ {{x'\!\!\!\!{\text{（}}t {\text{）}}} } \!\!\!\right]} } \right\} {\text{＝}} {\text{－}} {\rm{F^{-1}}}\left\{ {H {\!\!\!\!\text{（}}\omega {\text{）}}\!\!\!\!} \right.\left. \!\!\!\! \cdot{{\rm{F}}\left[ {x({t})} \right]} \right\} {\text{＝}} {\text{－}} a\!\!\!\!{\text{（}}{{\omega _k}{\text{，}}\!\!\!\!t} {\text{）}}\!\!\!\!{\text{，}} \end{aligned}$
(18) $ H\!\!\!\!{\text{（}}\omega {\text{）}}\!\!\!\! {\text{＝}}\frac{{ {\omega _k^2 {\text{＋}} 2{\rm{i}}\xi {\omega _k}\omega } }}{{{\omega _k^2 {\text{－}} {\omega ^2} {\text{＋}} 2{\rm{i}}\xi {\omega _k}\omega } }}{\text{，}} $
(19) This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
4. 反应谱拟合案例
两种改进反应谱拟合过程的方法是考虑迭代相关的反应谱拟合以及考虑相位谱的反应谱拟合。本文以 《公路桥梁抗震设计规范》 （中华人民共和国交通运输部，2020）中的设计谱及美国核管会标准审查大纲（U.S. NRC，2014）中的核电厂设备的抗震需求谱分别作为目标谱，对比经改进之后的拟合方法与传统方法所生成的模拟结果。
考虑到相位谱的反应谱拟合方法对相位谱的修正较大，修正之后会显著增加迭代计算的计算量、运算时间，所以需严格约束此方法的使用范围和修正的迭代次数。具体改进方案如下：首先使用考虑迭代相关的反应谱拟合方法迭代10次，再使用考虑相位谱的反应谱拟合迭代1次，最后使用考虑迭代相关的反应谱拟合方法迭代9次，总共迭代20次，以此与使用传统拟合方法迭代20次的结果进行对比。
图1为使用3组随机相位谱，针对某公路桥梁抗震设计谱（中华人民共和国交通运输部，2020），通过传统方法和改进方法分别模拟出的6条人工地震波反应谱对比图，图2为传统方法与改进方法在高频以及低频部分的局部对比图，图（a），（b）和（c）分别对应于特定的一组随机相位谱。图3为图1a和1b中下行拟合结果所对应的人工地震波波形。表1列出了6条人工波反应谱与目标谱之间81个控制点的平均误差对比。从图1和表1可以看到，改进方法所生成的人工地震波反应谱与传统方法相比拟合精度有显著提高，尤其在高频段和低频段的顽固点数量大幅减少，误差最少降低50%。
![]()
图 1 使用传统方法（上）和改进方法（下）对三组随机相位谱的拟合结果对比（a） 第一组；（b） 第二组；（c） 第三组Figure 1. Comparison of simulated results for three sets of random phase spectra by conventional method （upper panels） with those by improved method （lower panels）（a） The first set；（b） The second set；（c） The third set表 1 改进方法和传统方法模拟人工地震反应谱的误差Table 1. Errors of response spectra of artificial ground motions generated by conventional and improved methods相位谱编号 反应谱平均误差 传统方法 改进方法 第一组 4.81% 1.40% 第二组 4.44% 1.37% 第三组 3.25% 1.60% ![]()
图 2 传统方法与改进方法在高频部分（上）和低频部分（下）的拟合程度局部对比（a） 第一组；（b） 第二组；（c） 第三组Figure 2. Comparison of simulated results in high frequency range （upper panels） and low frequency range （lower panels） by conventional method and improved method（a） The first set；（b） The second set；（c） The third set![]()
图 3 使用改进方法在两组随机相位谱（图1a和1b下行）下生成的加速度波形Figure 3. Two acceleration time-series generated by improved method by the two random phase spectra as shown in the lower panels of Figs. 1a and 1b图4为使用三组随机相位谱分别通过传统方法和改进方法拟合而得的人工地震波反应谱对比图，其中各列子图对应一组随机相位谱，后两列为低频及高频部分的细部图。可以看到，无论是传统方法还是改进方法对于核电厂设计需求谱的拟合在频率中间段0.4—20 Hz的精度较高，但在高频及低频段均难以有效地拟合目标谱，部分原因是自然频率较高时单自由度体会随着地震波作刚体运动，地震波的加速度峰值即为高频段的谱加速度值，因此难以有效拟合。根据图4b和4c中的细部图可以发现在传统方法与改进方法都难以有效拟合的情况下，改进方法的拟合结果仍然较传统方法更接近目标谱的取值。
![]()
图 4 使用三组随机相位谱通过传统方法与改进方法所生成的人工波反应谱拟合对比图（a） 总体频段；（b） 低频段；（c） 高频段Figure 4. Comparison of response spectra generated by conventional and improved methods with three sets of random phase spectra（a） General frequencies；（b） Low frequencies；（c） High frequencies5. 结论
根据反应谱拟合人工地震波是结构抗震领域一个很重要的课题。然而，传统的频域法拟合人工地震波存在诸多问题，包括迭代效率低、顽固点多等。针对这些问题，本文通过优化频域法迭代过程中相关性的处理以及考虑相位谱的影响，提出综合改进的方法，通过提升拟合过程中迭代过程的工作效率进一步提高人工地震波对设计反应谱的拟合精度。算例结果表明，该方法的拟合精度较高，较传统方法有明显改进。
调整综合方法中两种改进方法的迭代次数、比例，形成更优化的综合方法，以进一步地提升迭代效率将是之后的研究重点。
-
![]()
图 10 四川台网地震台站垂直分量不同周期的噪声水平空间分布
Figure 10. Geographic distribution of vertical-component noise level for different periods at the seismic stations in Sichuan seismic network
（a） f ＝25.000 0 Hz；（b） f ＝9.638 8 Hz；（c） f ＝2.026 3 Hz；（d） f ＝0.328 5 Hz
![]()
图 1 四川省数字测震台网固定台站分布图
Figure 1. Distribution of permanent stations in Sichuan digital seismic network
![]()
图 2 四川台网金鸡寺（JJS）和天全（TQU）地震台2015年1月1日至2018年12月31日三分量连续观测数据的噪声功率谱密度（PSD）的概率密度函数（PDF）分布图
Figure 2. PSD-PDF distribution of three-component ambient noise at the stations JJS and TQU in Sichuan seismic network recorded from January 1，2015 to December 31，2018
![]()
图 3 四川台网金鸡寺（JJS）台（a）和天全（TQU）台（b）三分量统计中值和最小值功率谱密度（PSD）曲线
Figure 3. The three-component median and minimum PSD curves for the stations JJS （a） and TQU （b） in Sichuan seismic network
![]()
图 4 华蓥山台（HYS）台（a）和道孚（DFU）台（b）垂直分量噪声功率谱密度（PSD）值的日变化
Figure 4. Diurnal variations of vertical-component ambient noise PSD for the station HYS （a） and DFU （b）
![]()
图 5 地震台站垂直分量夜晚时段的噪声水平及其与白天的噪声水平变化值
Figure 5. The vertical-component noise levels of the seismic stations at nighttime （color） and their difference from daytime （circles） in the selected period bands
（a） 3—10 Hz；（b） 10—20 Hz；（c） 20—40 Hz
![]()
图 6 2015年1月1日至2018年12月31日道孚（DFU）地震台三分量噪声加速度功率谱密度（PSD）值随时间的变化分布
Figure 6. PSD spectrogram of ambient noise obtained from continuous three-component records at station DFU from January 1，2015 to December 31，2018
![]()
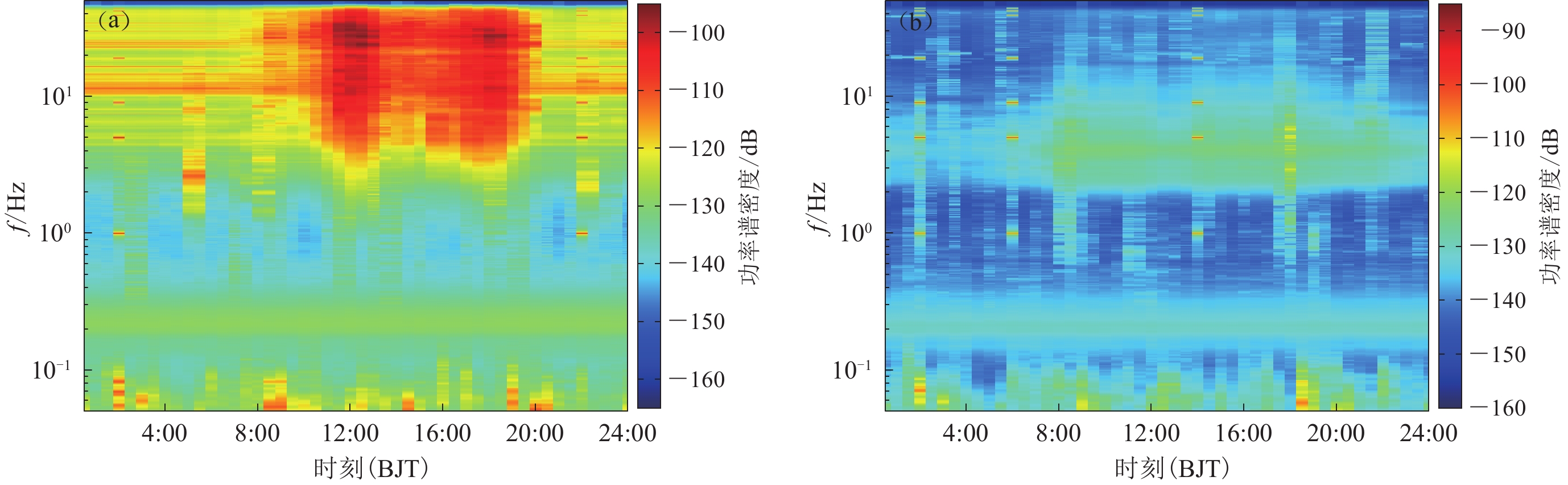
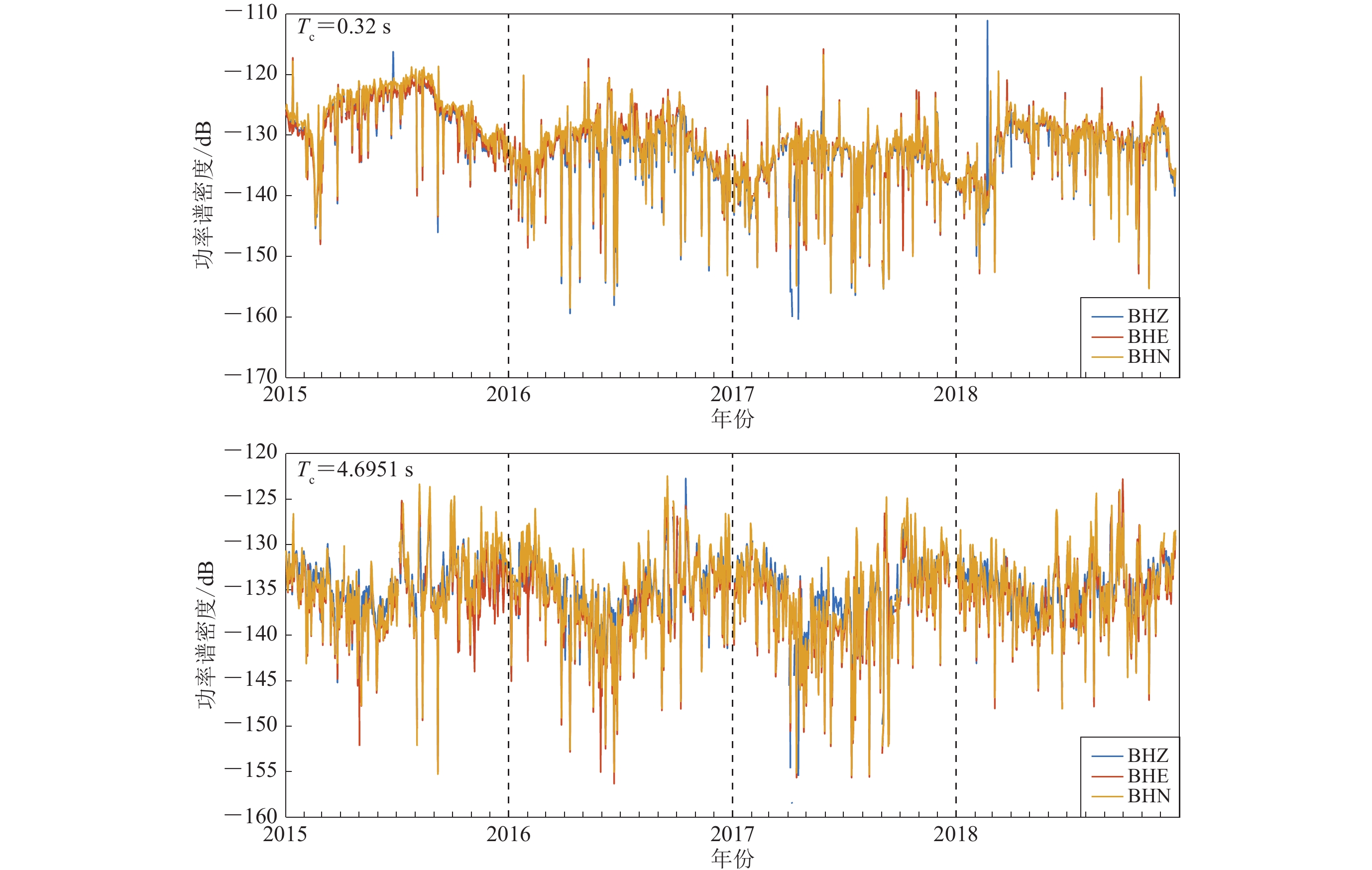
图 7 2015年1月1日至2018年12月31日道孚（DFU）地震台中心周期Tc为0.32 s和4.6951 s时三分量背景噪声功率谱密度（PSD）值随时间的变化
Figure 7. PSD values of ambient noise at the central periods Tc 0.32 s and 4.695 1 s obtained from continuous three-component records for the station DFU in Sichuan seismic network from January 1，2015 to December 31，2018
![]()
图 8 四川台网各台站夏季（a）和冬季（b） 0.1—0.5 Hz频段的垂直分量噪声水平分布
Figure 8. Distribution of vertical-component noise level in Sichuan seismic network in the frequency band 0.1—0.5 Hz during summer （a） and winter （b）
![]()
图 9 道孚（DFU）台（a）和金鸡寺（JJS）台（b）垂直分量夏季和冬季噪声功率谱密度（PSD）的概率密度函数（PDF）分布及中值、最小值PSD曲线
Figure 9. The PDF distribution of PSD for vertical-component seismic noise in summer and winter for the stations DFU （a） and JJS （b） and the median and minimum PSD curves
![]()
图 10 四川台网地震台站垂直分量不同周期的噪声水平空间分布
Figure 10. Geographic distribution of vertical-component noise level for different periods at the seismic stations in Sichuan Seismic Network
（e） f ＝0.195 3 Hz；（f） f ＝0.097 7 Hz；（g） f ＝0.063 3 Hz；（h） f ＝0.048 8 Hz；（i） f ＝0.041 1 Hz；（j） f ＝0.020 5 Hz
表 1 四川数字测震台网各台站垂直分量夜晚时段的噪声水平及昼夜变化值
Table 1 The vertical-component noise level at nighttime for the seismic stations of Sichuan digital seismic networks and their difference from daytime
序号 台站
名称台站
代码3—10 Hz 频带 10—20 Hz 频带 20—40 Hz 频带 夜晚噪声水平
/dB昼夜变化
/dB夜晚噪声水平
/dB昼夜变化
/dB夜晚噪声水平
/dB昼夜变化
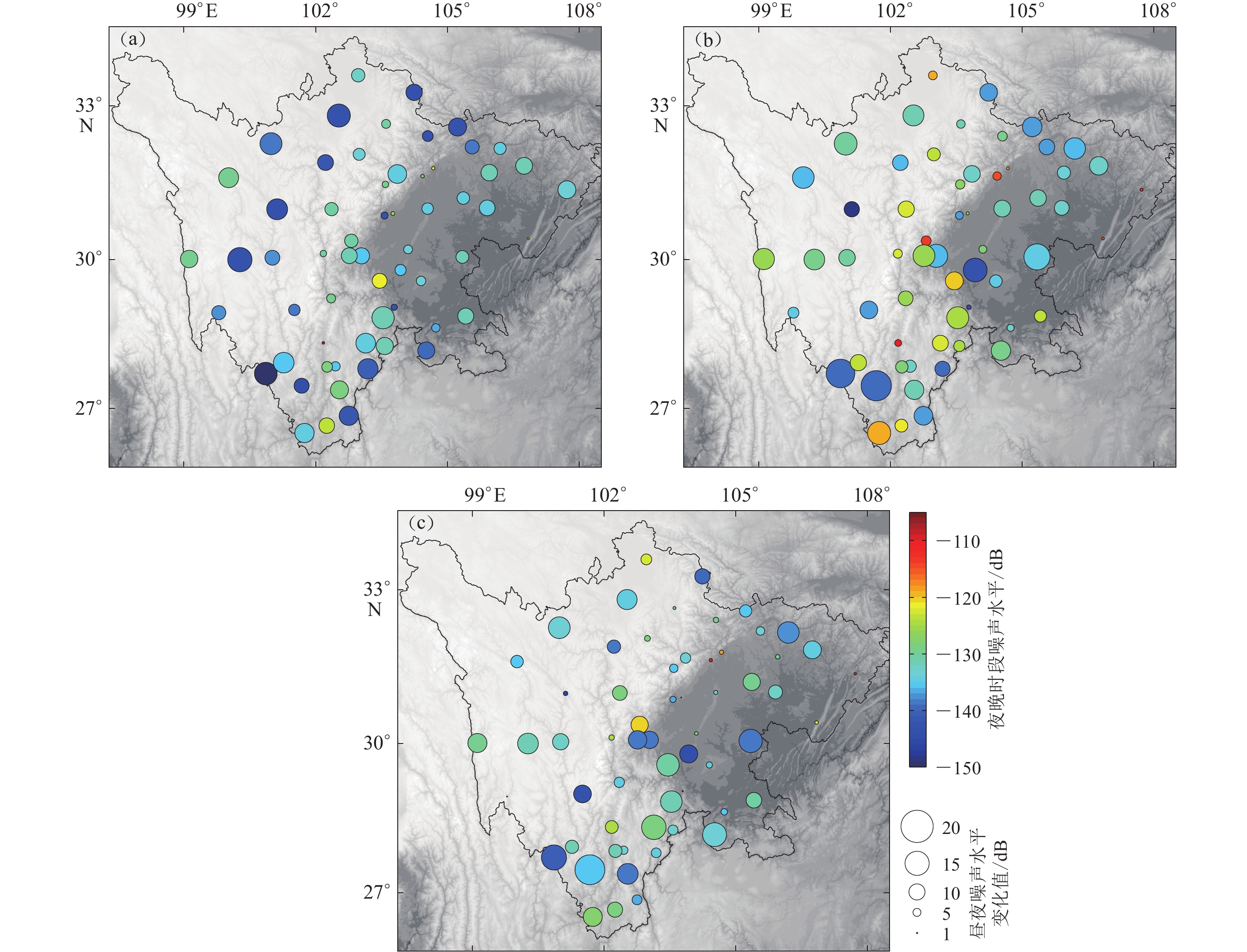
/dB1 安县 AXI −127.27 2.25 −118.32 5.21 −109.54 2.15 2 安岳 AYU −131.74 8.00 −136.56 16.16 −138.21 14.52 3 宝兴 BAX −130.44 8.31 −117.78 6.22 −120.61 10.66 4 巴塘 BTA −129.44 10.80 −128.70 13.22 −129.35 11.84 5 丙乙底 BYD −140.19 12.60 −140.18 9.43 −134.32 6.09 6 巴中 BZH −131.37 10.40 −134.36 11.41 −134.25 11.08 7 成都 CD2 −125.10 2.56 −128.30 2.02 −129.47 0.62 8 苍溪 CXI −131.53 10.28 −135.32 8.14 −129.87 2.86 9 道孚 DFU −143.91 12.94 −148.38 9.48 −146.51 2.91 10 峨眉山 EMS −121.74 9.20 −123.48 11.10 −130.33 13.96 11 姑咱 GZA −130.59 4.06 −125.17 5.59 −124.94 3.35 12 甘孜 GZI −129.86 12.40 −137.94 13.45 −135.92 7.78 13 会理 HLI −123.38 9.63 −124.19 7.98 −128.13 9.39 14 花马石 HMS −133.13 5.94 −136.34 7.78 −134.90 3.90 15 黑水 HSH −133.09 7.44 −126.52 8.01 −128.26 3.66 16 汉王山 HWS −136.54 5.29 −134.05 4.37 −135.98 4.09 17 华蓥山 HYS −125.08 1.27 −118.83 1.77 −122.46 2.25 18 红原 HYU −142.59 14.29 −133.77 13.00 −134.80 12.41 19 金鸡寺 JJS −134.91 7.22 −133.69 10.42 −133.33 2.69 20 筠连 JLI −139.43 10.59 −131.01 12.04 −133.60 14.78 21 九龙 JLO −138.43 7.17 −138.66 10.98 −142.43 11.01 22 剑门关 JMG −138.83 8.68 −138.19 9.74 −133.98 5.33 23 井研 JYA −135.63 6.94 −143.38 14.73 −144.64 11.14 24 九寨沟 JZG −143.93 10.13 −138.60 10.88 −139.05 9.52 25 雷波 LBO −131.24 10.66 −127.53 7.02 −132.99 6.24 26 泸沽湖 LGH −149.44 14.08 −140.25 17.69 −140.59 15.58 27 理塘 LTA −142.38 15.07 −131.62 12.86 −131.36 12.89 28 泸州 LZH −131.22 9.94 −126.66 7.42 −130.66 9.66 29 马边 MBI −131.59 13.84 −127.66 13.60 −131.69 13.40 30 蒙顶山 MDS −135.24 10.02 −137.06 14.35 −138.71 10.78 31 马尔康 MEK −141.97 9.81 −137.25 9.69 −138.41 8.31 32 美姑 MGU −134.78 12.20 −125.74 9.81 −128.07 15.16 33 木里 MLI −135.31 12.81 −126.96 10.05 −131.70 8.18 34 冕宁 MNI −108.62 1.71 −114.16 4.25 −124.79 7.96 35 茂县 MXI −134.25 11.63 −134.37 10.36 −133.92 6.51 36 普格 PGE −128.34 11.18 −133.24 11.94 −138.73 12.89 37 平武 PWU −142.46 6.76 −131.86 5.99 −129.30 3.36 38 攀枝花 PZH −134.60 11.94 −123.00 14.34 −127.04 11.74 39 青川 QCH −142.28 11.17 −138.21 12.09 −135.66 7.61 40 若尔盖 REG −132.80 8.29 −122.80 5.49 −122.06 6.80 41 壤塘 RTA −138.34 13.67 −132.06 14.16 −133.48 13.50 42 石棉 SMI −129.27 5.63 −128.19 9.15 −134.12 6.46 43 石门坎 SMK −141.35 11.93 −138.73 11.61 −136.89 6.15 44 松潘 SPA −130.44 5.59 −133.89 5.33 −128.07 1.97 45 天全 TQU −131.84 9.86 −128.01 13.52 −138.34 11.40 46 旺苍 WAC −134.56 7.56 −137.98 13.32 −137.63 13.41 47 汶川 WCH −129.50 4.09 −129.94 5.84 −135.15 5.22 48 五马坪 WMP −142.63 3.89 −145.97 2.85 −140.49 0.93 49 乡城 XCE −137.05 8.75 −136.27 6.85 −128.68 0.65 50 西充 XCO −134.28 9.76 −134.11 9.14 −132.33 8.64 51 宣汉 XHA −133.50 11.09 −116.23 1.91 −110.09 1.65 52 小金 XJI −130.91 8.22 −125.06 10.11 −128.29 9.12 53 玄生坝 XSB −136.00 5.82 −134.56 7.61 −133.86 5.26 54 油罐顶 YGD −133.25 5.53 −130.74 4.95 −127.52 2.33 55 雅江 YJI −137.74 9.25 −132.05 10.21 −132.70 10.10 56 盐亭 YTI −134.13 7.75 −133.68 10.24 −129.88 10.63 57 园艺场 YYC −128.69 6.71 −130.78 7.70 −131.39 8.14 58 盐源 YYU −144.94 9.33 −140.59 18.68 −135.41 18.48 59 油榨坪 YZP −142.15 4.39 −138.50 4.90 −136.64 3.87 60 仲家沟 ZJG −121.31 2.11 −122.69 1.81 −118.80 −2.69 最小值/dB −149.44 1.27 −148.38 1.77 −146.51 −2.69 最大值/dB −108.62 15.07 −114.16 18.68 −109.54 18.48 平均值/dB −134.11 8.67 −131.67 9.33 −132.01 7.84 中 值/dB −134.19 9.23 −132.65 9.72 −133.16 8.05 
下载: 导出CSV
-
葛洪魁,陈海潮,欧阳飚,杨微,张梅,袁松湧,王宝善. 2013. 流动地震观测背景噪声的台基响应[J]. 地球物理学报,56(3):857–868. doi: 10.6038/cjg20130315 Ge H K,Chen H C,Ouyang B,Yang W,Zhang M,Yuan S Y,Wang B S. 2013. Transportable seismometer response to seismic noise in vault[J]. Chinese Journal of Geophysics,56(3):857–868 (in Chinese).
吴建平,欧阳飚,王未来,姚志祥,袁松涌. 2012. 华北地区地震环境噪声特征研究[J]. 地震学报,34(6):818–829. doi: 10.3969/j.issn.0253-3782.2012.06.008 Wu J P,Ouyang B,Wang W L,Yao Z X,Yuan S Y. 2012. Ambient noise level of North China from temporary seismic array[J]. Acta Seismologica Sinica,34(6):818–829 (in Chinese).
谢江涛,林丽萍,谌亮,赵敏. 2018. 地震台站台基噪声功率谱概率密度函数Matlab实现[J]. 地震地磁观测与研究,39(2):84–89. doi: 10.3969/j.issn.1003-3246.2018.02.012 Xie J T,Lin L P,Chen L,Zhao M. 2018. The program of probability density function of power spectral density curves from seismic noise of a station based on Matlab[J]. Seismological and Geomagnetic Observation and Research,39(2):84–89 (in Chinese).
谢江涛,林丽萍,赵敏,黄春梅,李荪海. 2019. 应急流动观测组网技术在康定6.3级地震中的应用[J]. 华南地震,39(3):23–31. doi: 10.13512/j.hndz.2019.03.004 Xie J T,Lin L P,Zhao M,Huang C M,Li S H. 2019. The application of emergency portable seismological observation network technology in Kangding MS6.3 earthquake[J]. South China Journal of Seismology,39(3):23–31 (in Chinese).
谢江涛,林丽萍,谌亮. 2020. 九寨沟MS7.0地震应急流动台站噪声水平分析[J]. 大地测量与地球动力学,40(9):962–969. doi: 10.14075/j.jgg.2020.09.017 Xie J T,Lin L P,Chen L. 2020. Ambient noise level of MS7.0 Jiuzhaigou earthquake emergency mobile stations[J]. Journal of Geodesy and Geodynamics,40(9):962–969 (in Chinese).
Aster R C,McNamara D E,Bromirski P D. 2008. Multidecadal climate-induced variability in microseisms[J]. Seismol Res Lett,79(2):194–202. doi: 10.1785/gssrl.79.2.194
Beauduin R,Lognonné P,Montagner J P,Cacho S,Karczewski J F,Morand M. 1996. The effects of the atmospheric pressure changes on seismic signals or how to improve the quality of a station[J]. Bull Seismol Soc Am,86(6):1760–1769. doi: 10.1785/BSSA0860061760
Bormann P, Wielandt E. 2013. Seismic signals and noise[G]//New Manual of Seismological Observatory Practice 2 （NMSOP2）. Potsdam: Deutsches GeoForschungsZentrum: 1–62.
Brenguier F,Campillo M,Takeda T,Aoki Y,Shapiro N M,Briand X,Emoto K,Miyake H. 2014. Mapping pressurized volcanic fluids from induced crustal seismic velocity drops[J]. Science,345(6192):80–82. doi: 10.1126/science.1254073
Bromirski P D,Duennebier F K,Stephen R A. 2005. Mid-ocean microseisms[J]. Geochem Geophys Geosyst,6(4):Q04009. doi: 10.1029/2004GC000768
Bromirski P D,Stephen R A,Gerstoft P. 2013. Are deep-ocean-generated surface-wave microseisms observed on land?[J]. J Geophys Res:Solid Earth,118(7):3610–3629. doi: 10.1002/jgrb.50268
Burtin A,Bollinger L,Vergne J,Cattin R,Nábělek J L. 2008. Spectral analysis of seismic noise induced by rivers:A new tool to monitor spatiotemporal changes in stream hydrodynamics[J]. J Geophys Res:Solid Earth,113(B5):B05301. doi: 10.1029/2007JB005034
de la Torre T L,Sheehan A F. 2005. Broadband seismic noise analysis of the Himalayan Nepal Tibet Seismic Experiment[J]. Bull Seismol Soc Am,95(3):1202–1208. doi: 10.1785/0120040098
Demuth A,Ottemöller L,Keers H. 2016. Ambient noise levels and detection threshold in Norway[J]. J Seismol,20(3):889–904. doi: 10.1007/s10950-016-9566-8
Denolle M A,Dunham E M,Prieto G A,Beroza G C. 2013. Ground motion prediction of realistic earthquake sources using the ambient seismic field[J]. J Geophys Res:Solid Earth,118(5):2102–2118. doi: 10.1029/2012JB009603
Denolle M A,Dunham E M,Prieto G A,Beroza G C. 2014. Strong ground motion prediction using virtual earthquakes[J]. Science,343(6169):399–403. doi: 10.1126/science.1245678
Gerstoft P,Tanimoto T. 2007. A year of microseisms in southern California[J]. Geophys Res Lett,34(20):L20304. doi: 10.1029/2007GL031091
Hasselmann K. 1963. A statistical analysis of the generation of microseisms[J]. Rev Geophys,1(2):177–210. doi: 10.1029/RG001i002p00177
Kedar S,Longuet-Higgins M,Webb F,Graham N,Clayton R,Jones C. 2008. The origin of deep ocean microseisms in the North Atlantic ocean[J]. Proc Roy Soc A:Math Phys Eng Sci,464(2091):777–793. doi: 10.1098/rspa.2007.0277
Lecocq T,Hicks S P,Noten K V,Wijk K V,Koelemeijer P,Plaen R S M D,Massin F,Hillers G,Anthony R E,Apoloner M T,Arroyo-Solórzano M,Assink J D,Büyükakpınar P,Cannata A,Cannavo F,Carrasco S,Caudron C,Chaves E J,Cornwell D G,Craig D,Ouden O F C D,Diaz J,Donner S,Evangelidis C P,Evers L,Fauville B,Fernandez G A,Giannopoulos D,Gibbons S J,Girona T,Grecu B,Grunberg M,Hetényi G,Horleston A,Inza A,Irving J C E,Jamalreyhani M,Kafka A,Koymans M R,Labedz C R,Larose E,Lindsey N J,Mckinnon M,Megies T,Miller M S,Minarik W,Moresi L,Márquez-Ramírez V H,Möllhoff M,Nesbitt I M,Niyogi S,Ojeda J,Oth A,Proud S,Pulli J,Retailleau L,Rintamäki A E,Satriano C,Savage M K,Shani-Kadmiel S,Sleeman R,Sokos E,Stammler K,Stott A E,Subedi S,Sørensen M B,Taira T,Tapia M,Turhan F,Pluijm B V D,Vanstone M,Vergne J,Vuorinen T A T,Warren T,Wassermann J,Xiao H. 2020. Global quieting of high-frequency seismic noise due to COVID-19 pandemic lockdown measures[J]. Science,369(6509):1338–1343. doi: 10.1126/science.abd2438
Longuet-Higgins M S. 1950. A theory of the origin of microseisms[J]. Philos Trans R Soc Lond A:Math Phys Eng Sci,243(857):1–35. doi: 10.1098/rsta.1950.0012
Marzorati S,Bindi D. 2006. Ambient noise levels in north central Italy[J]. Geochem Geophys Geosyst,7(9):Q09010. doi: 10.1029/2006GC001256
McNamara D E,Buland R P. 2004. Ambient noise levels in the continental United States[J]. Bull Seismol Soc Am,94(4):1517–1527. doi: 10.1785/012003001
Peterson J R. 1993. Observations and Modeling of Seismic Background Noise[R]. Albuquerque: U.S. Geological Survey, 93-322: 1−95.
Rastin S J,Unsworth C P,Gledhill K R,McNamara D E. 2012. A detailed noise characterization and sensor evaluation of the North Island of New Zealand using the PQLX data quality control system[J]. Bull Seismol Soc Am,102(1):98–113. doi: 10.1785/0120110064
Ringler A T,Hutt C R. 2010. Self-noise models of seismic instruments[J]. Seismol Res Lett,81(6):972–983. doi: 10.1785/gssrl.81.6.972
Sabra K G,Gerstoft P,Roux P,Kuperman W A,Fehler M C. 2005. Extracting time-domain Green’s function estimates from ambient seismic noise[J]. Geophys Res Lett,32(3):L03310. doi: 10.1029/2004GL021862
Shapiro N M,Campillo M. 2004. Emergence of broadband Rayleigh waves from correlations of the ambient seismic noise[J]. Geophys Res Lett,31(7):L07614. doi: 10.1029/2004GL019491
Sleeman R,Melichar P. 2012. A PDF representation of the STS-2 self-noise obtained from one year of data recorded in the Conrad observatory,Austria[J]. Bull Seismol Soc Am,102(2):587–597. doi: 10.1785/0120110150
Stutzmann E,Roult G,Astiz L. 2000. Geoscope station noise levels[J]. Bull Seismol Soc Am,90(3):690–701. doi: 10.1785/0119990025
Stutzmann E,Schimmel M,Patau G,Maggi A. 2009. Global climate imprint on seismic noise[J]. Geochem Geophys Geosyst,10(11):Q11004. doi: 10.1029/2009GC002619
Tasič I,Runovc F. 2012. Seismometer self-noise estimation using a single reference instrument[J]. J Seismol,16(2):183–194. doi: 10.1007/s10950-011-9257-4
Traer J,Gerstoft P,Bromirski P D,Shearer P M. 2012. Microseisms and hum from ocean surface gravity waves[J]. J Geophys Res:Solid Earth,117:B11307. doi: 10.1029/2012JB009550
Traer J,Gerstoft P. 2014. A unified theory of microseisms and hum[J]. J Geophys Res:Solid Earth,119(4):3317–3339. doi: 10.1002/2013JB010504
Waldhauser F,Ellsworth W L. 2000. A double-difference earthquake location algorithm:Method and application to the northern Hayward fault,California[J]. Bull Seismol Soc Am,90(6):1353–1368. doi: 10.1785/0120000006
Webb S C. 1998. Broadband seismology and noise under the ocean[J]. Rev Geophys,36(1):105–142. doi: 10.1029/97RG02287
Webb S C. 2002. Seismic noise on land and on the sea floor[G]//International Geophysics. International Handbook of Earthquake and Engineering Seismology, Part A. Amsterdam: Academic Press: 81: 305-318.
Welch P. 1967. The use of fast Fourier transform for the estimation of power spectra:A method based on time averaging over short,modified periodograms[J]. IEEE Trans Audio Electroacoust,15(2):70–73. doi: 10.1109/TAU.1967.1161901
Wolin E,van der Lee S,Bollmann T A,Wiens D A,Revenaugh J,Darbyshire F A,Frederiksen A W,Stein S,Wysession M E. 2015. Seasonal and diurnal variations in long-period noise at SPREE stations:The influence of soil characteristics on shallow stations’ performance[J]. Bull Seismol Soc Am,105(5):2433–2452. doi: 10.1785/0120150046
Yang Y J,Ritzwoller M H. 2008. Characteristics of ambient seismic noise as a source for surface wave tomography[J]. Geochem Geophys Geosyst,9(2):Q02008. doi: 10.1029/2007GC001814
Young C J,Chael E P,Withers M M,Aster R C. 1996. A comparison of the high-frequency (>1 Hz) surface and subsurface noise environment at three sites in the United States[J]. Bull Seismol Soc Am,86(5):1516–1528. doi: 10.1785/BSSA0860051516
Zhang M,Wen L X. 2015. An effective method for small event detection:Match and locate (M&L)[J]. Geophys J Int,200(3):1523–1537. doi: 10.1093/gji/ggu466
Zhu L P,Zhou X F. 2016. Seismic moment tensor inversion using 3D velocity model and its application to the 2013 Lushan earthquake sequence[J]. Phys Chem Earth:Parts A/B/C,95:10–18. doi: 10.1016/j.pce.2016.01.002
-
期刊类型引用(11)
1. 戴纳新，胡群，刘彦辉，周福霖，彭林欣. 基于核电厂规范反应谱的时-频人工波合成及楼层反应谱研究. 广西大学学报(自然科学版). 2025(01): 70-82 . 
百度学术
2. 王伟，许晨夜. 上承式大跨度钢箱无铰拱桥地震响应分析. 黑龙江交通科技. 2024(09): 127-130+137 . 百度学术
3. 唐光武，于雯，谢皓宇，刘海明. 近场地震作用下桥梁结构动力响应研究进展. 公路交通技术. 2023(01): 60-68 . 百度学术
4. 杨兰兰，傅梓岳，王登峰，XIE Weichau. 基于数字滤波技术拟合规范反应谱的地震动研究. 振动与冲击. 2023(09): 57-67+105 . 百度学术
5. 张树翠，夏宏升，张欣刚，姚文莉，齐朝晖，刘大强. 一种内蕴基线漂移校正的人工地震波反应谱拟合方法. 地震工程学报. 2023(05): 1171-1178 . 百度学术
6. 杨连，林雄伟，王军. 超高层建筑中考虑相位随机性的基岩地震动时程分析. 粉煤灰综合利用. 2022(01): 39-43+49 . 百度学术
7. 高树飞，冯云芬. 基于Newmark滑块位移法的岸坡地震稳定性简易分析方法. 水运工程. 2022(06): 40-48 . 百度学术
8. 谢皓宇，潘飞，朱翊洲，郑万山，仉文岗. 核设备设计地震动包络标准PSD的拟合及试验研究. 土木与环境工程学报(中英文). 2021(03): 128-134 . 百度学术
9. 谢皓宇，朱翊洲，仉文岗，唐光武，谢永诚. 核电厂设备抗震设计标准功率谱密度的生成方法. 核动力工程. 2021(05): 128-133 . 百度学术
10. 别江波，刘星星，刘宏. 某机场高填方边坡的动力特性模拟分析. 工程建设. 2021(09): 13-18 . 百度学术
11. 杜迎乾，王之军，周承浩，许汉兵，贾书海. 人工地震波遗传优化生成方法. 工程抗震与加固改造. 2021(06): 149-156+95 . 百度学术
其他类型引用(0)
计量
- 文章访问数: 1060
- HTML全文浏览量: 411
- PDF下载量: 186
- 被引次数: 11
