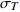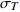高级搜索

俯冲带板内地震竖向加速度谱阻尼修正系数模型研究

陈心锋, 姜妍旭, 刘名吉

陈心锋，姜妍旭，刘名吉. 2022. 俯冲带板内地震竖向加速度谱阻尼修正系数模型研究. 地震学报，44（2）：339−355. DOI: 10.11939/jass.20200198
引用本文: 陈心锋，姜妍旭，刘名吉. 2022. 俯冲带板内地震竖向加速度谱阻尼修正系数模型研究. 地震学报，44（2）：339−355. DOI: 10.11939/jass.20200198
shu
Chen X F，Jiang Y X，Liu M J. 2022. A damping modification factor model for vertical acceleration spectrum from slab earthquakes in subduction zone. Acta Seismologica Sinica，44（2）：339−355. DOI: 10.11939/jass.20200198
Citation: Chen X F，Jiang Y X，Liu M J. 2022. A damping modification factor model for vertical acceleration spectrum from slab earthquakes in subduction zone. Acta Seismologica Sinica，44（2）：339−355. DOI: 10.11939/jass.20200198
shu

俯冲带板内地震竖向加速度谱阻尼修正系数模型研究

  • 中国济南 250000 山东建筑大学土木工程学院

基金项目: 国家自然科学基金（51878396）资助
详细信息
    通讯作者:

    陈心锋，硕士研究生，主要从事岩土地震工程方面的研究，e-mail：chenxf1996@126.com

  • 中图分类号: P315.9

A damping modification factor model for vertical acceleration spectrum from slab earthquakes in subduction zone

  • School of Civil Engineering，Shandong Jianzhu University，Ji’nan 250000，China

HTML全文

    摘要
  • 摘要: 基于日本K-NET和KiK-net台网的4 695条俯冲带板内地震记录，采用最小二乘法对阻尼修正系数（DMF）的几何均值进行关于阻尼比和谱周期的回归拟合，分场地类别建立了考虑阻尼比和谱周期的竖向加速度谱DMF模型。为探究震源、路径和场地效应对该模型残差分布的影响，采用随机效应模型将残差分离得到各类残差及相应的残差标准差，在此基础上进行DMF模型残差分析。研究结果表明，DMF可以采用阻尼比对数值的三次多项式、周期对数值的四次多项式来模拟。由于规范设计反应谱并非针对某一特定地震，规范提出的DMF模型并不包含震源和路径参数，但事件间和事件内残差关于矩震级、断层距离和断层深度的分布表明，在给定地震事件下，包含地震动参数的DMF模型将会改善模型的预测能力。
    Abstract: In this study, 4695 strong-motion records from subduction slab earthquakes in Japan obtained by the K-NET and KiK-net networks were used to develop a damping modification factor （DMF） model for the vertical acceleration spectrum. The DMF model considering the damping ratio and spectral period is established for four site classes, and the geometric mean values for DMF are used to perform regression fitting in respect to the damping ratio and spectral period by least squares method. To evaluate the influence of hypocenter, path, and site effects on the residual distribution of the model, the total model residuals and standard deviations were separated into between-event and within-event parts, and the within-event residuals were further divided into the between-site and within-site parts by using a random effects model. The results show that the effect of damping ratios on DMF can be modelled by the third-order polynomial of the logarithm of the damping ratios, a fourth-order polynomial of the logarithm of spectral periods can be used to model the effect of spectral periods for the DMF. The effects of source and path parameters were not modelled in this study because of DMF model is used to scale a design spectrum not associated with a given scenario earthquake. The distribution of the between- and within-event residual distributions with respect to moment magnitude, fault depth and source distance suggests that including these terms would improve prediction capability of the model when a DMF is designed for scaling a 5% acceleration spectrum associated with a scenario event for a give magnitude and source distance.
    • HTML全文

  • 引言

    巴布亚新几内亚东南部处于澳大利亚板块和西南太平洋板块的斜向汇聚带内（图1），其南部有欧文—斯坦利（Owen-Stanley）断裂带，北连所罗门（Solomon）微板块，东接活跃的伍德拉克（Woodlark）海底扩张海盆，中部发育了快速扩张的伍德拉克裂谷（Wallace et al，2004），并在当特尔卡斯托群岛（D’Entrecasteaux islands）出露了世界上最年轻的超高压岩石（Baldwin et al，2004；Webb et al，2008；Little et al，2011；Gordon et al，2012；Eilon et al，2015）。澳大利亚板块在晚古新世至早始新世期间北向俯冲，形成了一个低角度的拆离断层（欧文—斯坦利断裂带），其上覆洋壳中产生了大量的岩浆活动并生成了巴布亚超镁铁质带，而其下覆大陆岩石圈经受着持续的变质作用（Davies，Smith，1971；Davies，Jaques，1984；Lus et al，2004）。中新世时期，巴布亚（Papua）半岛持续隆升，并伴随着快速的沉积，覆盖于上盘的洋壳之上（Davies，Smith，1971）。与此同时，北部的所罗门板块在早中新世向南俯冲并诱发特罗布里恩（Trobriand）断层的形成，其俯冲相关构造可能在巴布亚半岛的隆升和岩浆活动中起到作用（van Ufford，Cloos，2005；Fitz，Mann，2013）。晚中新世至早上新世，伍德拉克裂谷持续向西张裂发育，并伴有当特尔卡斯托群岛的隆升和花岗岩的侵入（Davies，Jaques，1984；Hill et al，1992）。伍德拉克裂谷的张裂伸展速率为10—15 mm/a，其动力可能来自于所罗门微板块北向俯冲的板片拖拽（Tregoning et al，1998；Wallace et al，2004；Biemiller et al，2020）。超高压岩石的出露可能与伍德拉克裂谷的张裂作用息息相关（Baldwin et al，1993；Monteleone et al，2007；Webb et al，2008；Little et al，2011；Abers et al，2016）。另外，下地壳浮力诱发的底侵（Martinez et al，2001）和古大陆俯冲残片的底辟作用（Ellis et al，2011）也可能是超高压岩石出露的机制之一。为了揭示伍德拉克裂谷张裂和超高压岩石出露的可能构造动力学机制，此地区前期已开展了多个地震学观测计划并积累了充足的地震波形数据来探究深部壳幔结构。

    图  1  伍德拉克裂谷地区海底地震仪的分布
    紫色和黑色的圆圈分别表示含有效和无效数据的海底地震仪；红色三角为新生代火山；黑色虚线代表裂谷扩张轴，红色实线为欧文—斯坦利断裂带，绿色实线为地震测线（Fitz，Mann，2013）。右上插图代表了纵波（蓝色圆圈）和瑞雷波偏振分析（红色圆圈）所用的地震事件分布图，其中绿色三角形表示研究区域的中心位置。底部右侧插图中的黄色方框显示了研究区域的具体位置，其中红线为板块边界（Bird，2003）
    Figure  1.  Topographic map of the Woodlark rift showing the locations of the ocean bottom seismographs （OBSs）
    The purple and black dots represent OBSs with and without valid data，respectively. Red triangles denote Cenozoic volcanos. The black dashed line indicates the rift axis. The red line represents the Owen-Stanley fault zone. The green lines are seismic profiles from previous study （Fitz，Mann，2013）. The top-right inset presents the events used for the polarization analysis of Rayleigh wave （red circles） and P-wave （blue circles）. The green triangle marks the center of the study area. The bottom right inset displays the location of thestudy area highlighted by the yellow rectangle. The red lines denote the plate boundaries （Bird，2003）
    下载: 全尺寸图片 幻灯片

    2010年3月到2011年10月CDPAPUA实验计划（Eilon et al，2014）在伍德拉克裂谷及其周边地区均匀布设了宽频带地震台阵，难能可贵的是其中包含了分布于裂谷内的八台海底地震仪（OBS）（图1），为揭示裂谷内的地壳动力学机制提供了数据支撑。目前对于该裂谷地区的地壳结构认识仍然不足，部分原因可能是对已有OBS数据的挖掘程度不够。基于早期WOODSEIS实验计划采集到的数据，接收函数和远震层析反演结果表明当特尔卡斯托群岛处的地壳厚度与周围地区相比减薄约10—15 km，并被低密度的地幔补偿（Abers et al，2002）。近震层析成像研究显示在伍德拉克海盆海底扩张的前端存在镁铁质和长英质地壳组成的明显过渡区，并进而排除了岩浆活动在海底扩张开始前对地壳改造的可能性（Ferris et al，2006）。利用CDPAPUA地震观测数据开展的接收函数和面波联合反演结果显示：地壳最薄的区域不是在裂谷扩张轴地区，而是在当特尔卡斯托群岛（减薄30%—40%），并且超高压岩石的出露可能主要受控于裂谷的张裂作用，但遗憾的是并未对OBS处的地壳结构展开深入的讨论分析（Abers et al，2002）。因此，伍德拉克裂谷内的地壳结构及其动力学机制还是未被很好地约束，而可能的一种解决途径便是开展接收函数研究。远震接收函数方法已被成功运用于不同地区的海底地壳结构研究中，如邻近南海地区（丘学林等，2006；黄海波等，2011；胡昊等，2016；Hung et al，2021）。然而在开展接收函数相关研究前需要对OBS水平分量的方位进行校正，若不校正或校正不当会产生误差较大甚至错误的结果。

    通常以自由落体的方式来投放OBS，其北向水平分量的方位往往与实际地理北向方位不一致（图2），这会导致一些基于三分量波形数据的地震学方法如接收函数方法应用困难。对中国地震科学台阵探测项目Ⅰ期（CHINArray- Ⅰ ）观测数据的分析表明，当地震计水平分量方位的偏转角度超过20°时，接收函数的h-κ叠加结果会变得不稳定（Zeng et al，2021）。OBS水平分量方位通常是基于已知地震台站和地震事件的位置信息借助面波（Laske，1995；Selby，2001）或体波（Niu，Li，2011）偏振分析来确定。另外，通过环境噪声互相关提取出来的瑞雷波也可以用于确定OBS水平分量的方位（Zha et al，2013）。近期发展起来的波形模拟匹配技术也是确定OBS水平分量方位的可行方法之一（Zhu et al，2020）。

    图  2  （a） OBS水平分量的方位偏转示意图，χ和θ分别为OBS方位偏转角度和相对于北向水平分量的地震后方位角；（b） 地震台站B的瑞雷波偏振分析结果，图中蓝色圆圈为对应单事件最优方位偏转角度，红色虚线为该地震台站最终方位偏转角度115.1°；（c） 地震台站B的纵波偏振分析结果，蓝色虚线表示最佳方位偏转角度108°
    Figure  2.  （a） A schematic map of coordinate system exhibiting the relationship between the geographical North direction，the North component and the back-azimuth （BAZ） of an event. $ \chi $ and θ indicate the sensor orientation and the BAZ of the event relative to the North component，respectively；（b） Example of the Rayleigh-wave polarization analysis from the station B. The blue circles represent the optimal sensor orientations determined from each event based on the maximum correlation coefficient between the vertical and the Hilbert transformed radial components. The red dash line depicts the resulting station orientation 115.1°；（c） The P-wave polarization analysis for determining orientation of station B. The blue dashed line depicts the optimal sensor orientation，which is 108°
    下载: 全尺寸图片 幻灯片

    为了更加准确地测定OBS水平分量方位，揭示OBS水平方位对接收函数研究的影响，并进而获得台站下方的地壳构造，本研究将充分利用CDPAPUA实验计划布设在古迪纳夫盆地和基里比斯盆地的八台OBS宽频带地震数据（图1），分别引入纵波和瑞雷波偏振分析方法，确定OBS水平分量的实际方位，并基于获得的水平分量方位参数校正OBS三分量波形数据，利用接收函数方法反演伍德拉克裂谷地区的地壳结构，以期为深入认识汇聚构造背景下大陆裂谷的起始张裂机制提供更多深部构造证据。

    1.   数据和方法

    1.1   瑞雷波偏振分析

    瑞雷波偏振分析是基于从美国地震学研究联合会数据管理中心（Incorporated Research Institutions for Seismology Data Management Center，缩写为IRIS DMC）下载的CDPAPUA实验计划的八台OBS宽频带地震波形数据，其数据隶属于ZN台网（http://ds.iris.edu/mda/ZN/）。数据的筛选标准与Stachnik等（2012）所采用的类似：选取的地震事件为MS6.0以上；震中距控制在30°—100°范围内。所有的原始数据按照统一时间窗（瑞雷波理论到时前20 s和后600 s）进行截取，瑞雷波的理论到时根据平均速度4 km/s进行计算；之后将对所截取的面波数据段进行25—50 s的带通滤波，并人工检查筛选出高信噪比的数据。这样，最终获得66个地震事件（图1）的面波数据。

    瑞雷波的特点是其质点按照逆时针方向作椭圆运动，理论上只有在垂直分量和径向分量上有观测信号。为了尽可能地避免计算瑞雷波复杂的椭圆特征，通常将希尔伯特变换应用于径向分量，进而建立起径向分量与垂直分量间的线性关系（Baker，Stevens，2004；Stachnik et al，2012）。OBS北向水平分量的方位可表示为

    $$ \chi {{ ＝ |{\rm{BAZ}} － }}\theta {{|}} ，$$ (1)

    式中，BAZ为地震事件相对于地理北向的后方位角，θ为瑞雷波偏振分析获得的相对于地震计北向水平分量的后方位角（图2a）。本研究中，定义OBS的BH2水平分量为北向水平分量。对于某一地震事件，首先给定一个θ值，θ值的扫描区间为1°—360°，扫描间隔为1°；计算希尔伯特变换后的径向分量与垂向分量之间的互相关系数，其最大值所对应的θ值则为利用该地震事件数据确定的OBS北向水平分量的最佳方位（图2）。为了去除传播效应对结果的影响，我们剔除震源深度大于100 km且互相关系数小于0.4的地震事件。此外，每个地震事件确定的OBS方位角均需落在均值的95%置信区间内，其对应中值则为该OBS北向水平分量的最终方位偏转角度（Stachnik et al，2012）。

    1.2   纵波偏振分析

    与瑞雷波偏振分析类似，开展纵波偏振分析所用数据也是通过IRIS DMC下载获取，只是选取数据的标准不同：震中距范围为30°—180°；地震事件的最小阈值震级Mc是通过经验公式确定，其具体展开式为：

    $$ {M}_{{\rm{c}}}＝5.2＋\frac{\varDelta －30}{180－30}－\frac{D}{700} {，} $$ (2)

    式中：D为震源深度，单位为km；Δ为震中距，单位为度（Liu，Gao，2010）。地震事件波形时间窗为远震纵波理论到时前20 s至后260 s，其中纵波的理论到时通过地球一维速度模型IASP91 （Kennett，Engdahl，1991）计算获得。此外，利用垂直分量人工筛选出高信噪比的纵波信号，最终获得45个有效地震事件用于纵波偏振分析。

    远震纵波信号理论上只有在径向分量上可以被观测到，而在切向分量上没有或很少有能量的分布。因此，对含有纵波信号窗口内的所有切向分量数据叠加，当叠加能量最小时（附图1）所对应的扫描方位偏转角度则为该地震台站北向水平分量的最佳方位（Niu，Li，2011）。纵波到时由人工拾取，并定义其前2 s至后2 s为波形叠加窗口。同一地震台站的所有切向分量数据以信噪比为权重进行叠加，具体表达式为（Niu，Li，2011）：

    $$ {E}_{T} （ \chi ） ＝\frac{{\displaystyle\sum _{i＝1}^{N}}{\omega }_{i}{E}_{T}^{i} （ \chi ） }{{\displaystyle\sum_{i＝1} ^{N}}{\omega }_{i}} \text{，} $$ (3)

    式中：N为地震事件的数量；$E_T^i （ \chi ） $为第i个地震事件切向分量在纵波时窗内的能量。式（3）中的加权因子ωi进一步展开为（Niu，Li，2011）：

    $$ {\omega _i} ＝ 0.5 （ {\rm{SN}}{{\rm{R}}_{i， {\rm{BHN}}}} ＋ {\rm{SN}}{{\rm{R}}_{i， {\rm{BHE}}}} ） \text{，} $$ (4)

    式中SNR为信噪比值。ET最小值所对应的χ即为所求值。χ的扫描范围为1°—360°，扫描间隔为1°。纵波偏振分析通常会得到两个最佳的结果（χ和χ＋180°），通过参照瑞雷波偏振分析所得结果确定最终的方位角（图2c），其误差值利用迭代10次的重采样方法（Bootstrap）计算求得（Efron，Tibshirani，1986）。

    1.3   接收函数方法

    开展接收函数研究所用数据的初步筛选条件与纵波偏振分析相同。初筛之后，对所获原始地震波形数据进行0.08—0.8 Hz带通滤波，以提高信噪比。接收函数的产生是利用信噪比大于4的原始记录波形垂向分量对水平径向分量进行反褶积运算（Ammon，1991）。利用h-κ叠加方法处理人工筛选出的高信噪比接收函数，获得每台OBS下方的地壳厚度h和纵横波速比值κ （Zhu，Kanamori，2000）。h和κ的扫描区间分别设为10—40 km （间隔为0.1 km）和1.65—2.15 （间隔为0.001）。h-κ图中能量最强点处所对应的h和κ则为此地震台站下方的最终地壳厚度和纵横波速比值（图3）。根据前人的相关研究，地壳的平均纵波速度设为6.1 km/s （Abers et al，2002，2016）。同样，地壳厚度和纵横波速比的误差值也是通过10次迭代的重采样方法（Efron，Tibshirani，1986）计算获得。

    图  3  地震台站B纵波偏振分析方位偏转校正前（a）和后（b）的h-κ叠加结果
    上图中黑色和红色线分别为每条接收函数和时间域所有接收函数简单叠加后的波形，蓝色线为校正前接收函数的简单叠加波形；下图左侧表示归一化后的h-κ叠加能量图，图中红点为叠加能量最强点，表示最终确定的地壳厚度h和纵横波速比值κ （vP/vS），NRFs为接收函数数量；右下图表示h-κ叠加能量图中不同纵横波速比值所对应的最大能量点连线
    Figure  3.  h-κ stacking results from representative station B before （a） and after （b） misorientation correction from the P-wave polarization analysis
    The upper panel shows individual （black trace） receiver functions （RFs） and a simple time-domain stack （red trace） of all RFs，and the blue trace represents the simple stacked RF trace in time domain before misorientation correction. The lower left panel illustrates the h-κ plot in which the maximum stacking amplitude （red dot） determines the optimal pair of crustal thickness h and vP/vS ratio κ. NRFs is number of receiver functions. The lower right panel displays the maximum stacking amplitude for each candidate vP/vS ratio in the h-κ plot
    下载: 全尺寸图片 幻灯片

    2.   结果与讨论

    2.1   OBS水平分量方位及其对接收函数研究的影响

    通过对伍德拉克裂谷地区八台OBS数据开展纵波和瑞雷波偏振分析，最终确定了其中七台OBS水平分量的方位（图2和附图2—3），台站I由于数据质量较差，无法获得其有效的水平分量方位角。基于纵波和瑞雷波偏振分析分别获得的七台OBS水平分量方位偏转角度相差4°—16.9°，互相关系数高达0.994 6 （图4a），其对应数值变化范围为29°—356°和3°—323° （表1）。Eilon等（2014）利用瑞雷波偏振分析获得了类似的结果（表1）。由图4和表1可见，纵波偏振分析的标准差普遍大于瑞雷波偏振分析的标准差，这可能与计算方法和所用数据集不同有关。理论测试表明地震事件方位的不均匀分布可能使纵波偏振分析产生较大的标准差（Lim et al，2018），为此将每个台站的所有纵波地震事件根据其后方位角分成四组，分别为0°—90°，90°—180°，180°—270°和270°—360°；之后基于每组后方位角内的地震事件数据进行纵波偏振分析，以求取所对应的OBS水平分量的方位偏转角度。虽然大多数地震事件的后方位角区间处于270°—360°，但每组数据计算获得的OBS水平分量方位角相差并不是很大（附表1）。基于纵波和瑞雷波偏振分析获得的结果表明CDPAPUA实验计划的OBS北向水平分量与地理北向有明显偏差，在利用水平分量波形数据开展反演研究时，需要首先对这些OBS水平分量的方位进行校正。

    图  4  瑞雷波和纵波偏振分析得到的OBS方位结果对比图（a）以及本文与Abers等（2016）的地壳厚度结果对比（b）
    Figure  4.  Comparisons of OBS orientations from the Rayleigh-wave and P-wave polarization analyses （a） and crustal thickness from this study and Abers et al （2016） （b），respectively
    下载: 全尺寸图片 幻灯片
    表  1  纵波和瑞雷波偏振分析得到的每台OBS方位偏转角χ及其标准差STD
    Table  1.  The resulting OBS orientations χ from analysis of the Rayleigh-wave and P-wave polarization analyses and their standard error STD for each station
    台站瑞雷波偏振分析纵波偏振分析χ*/°
    χ/°STD/°χ/°STD/°
    B 115.1 0.55 108 3.03 116.8
    D 3.0 1.48 356 2.68 0.3
    E 176.0 1.65 168 3.17 183.1
    F 293.1 1.78 310 5.26 301.0
    G 44.2 1.92 29 7.68 47.2
    H 323.0 1.88 319 1.12 324.5
    J 131.8 2.53 144 12.97 143.9
    注：χ*为Eilon等（2014）基于瑞雷波偏振分析所得。
    下载: 导出CSV 
    | 显示表格

    为了直观地展示OBS水平分量的方位偏转可能对接收函数研究的影响，我们分别利用原始的和经过纵波与瑞雷波偏振分析校正后的地震波形数据产生接收函数，并通过h-κ叠加方法确定地壳结构信息（附图4—6）。方位校正后的有效接收函数数量明显增多，尤其是对于一些方位偏转角大的地震台站，例如地震台站B，经纵波和瑞雷波偏振分析校正后其接收函数数量分别从原来的11条增加至17条和18条；而基于体波和瑞雷波分析校正后最终分别获得103条和126条接收函数。另外，地震台站方位校正后的有效地震事件的后方位角比校正前分布更均匀（附图4—5）。对于方位偏转角较大的地震台站，校正前、后的地壳厚度和纵横波速比值相差较大（图3），与CHINArray-I数据分析所得结论（Zeng et al，2021）一致。相比之下，方位偏转角较小的地震台站，其方位校正前后的h-κ叠加结果基本一致，如水平分量方位偏转角小于5°的地震台站D （附图4a和5b）。纵波和瑞雷波偏振分析校正后获得的地壳结构基本一致（表2和附表2），但地震台站G和H由瑞雷波偏振分析校正后得到的结果不佳（附图5e和5f）。由于远震纵波通常比面波含有更多的高频成分，并且受岩石圈的强横向非均质性影响较小（Zeng et al，2021），在下面讨论中主要基于纵波偏振分析校正后获得的地壳结构。

    表  2  本文和前人获得的地壳厚度h和纵横波速比值κ
    Table  2.  Crustal thickness h and vP/vS ratios κ from this and previous studies
    台站南纬/°东经/°h /kmh*/kmκ
    B9.749150.35030.4±0.2127.8±0.531.94±0.028
    D9.943150.70736.7±0.1644.9±0.731.89±0.005
    E10.080 150.62134.2±2.69− 1.82±0.059
    F9.950150.20031.7±0.9636.2±0.611.90±0.072
    G9.333149.66725.8±0.7331.5±0.842.04±0.039
    H9.000149.66816.8±0.7136.8±0.741.94±0.085
    J8.870149.93429.8±0.6039.2±0.381.99±0.043
    注：h*为Abers等（2016）的结果。
    下载: 导出CSV 
    | 显示表格

    2.2   古迪纳夫盆地和基里比斯盆地的地壳结构

    伍德拉克裂谷地区获得的七台OBS下方地壳结构呈现显著的横向变化（图5和表2），其地壳厚度为16.8—36.7 km，平均值为（29.3±6.03） km。本研究获得的地壳厚度与瑞雷波-接收函数联合反演的大多数结果（Abers et al，2016）不太一致，其相关系数仅为0.323 7 （图4b），最大不同点在于基里比斯盆地地壳更薄（表2）。整个研究区域的纵横波速比κ值较大，处于1.82—2.04之间，平均值为1.93±0.66。Holbrook等（1992）的岩石实验研究表明κ值可指示地壳的大致组成，其中长英质岩石的κ值一般小于1.76，中间质岩石的κ值为1.76—1.81，镁铁质岩石的κ值大于1.81。Davies和Smith （1971）发现在东巴布亚半岛存在一厚度为12—18 km，由橄榄岩、辉长岩和玄武岩组成的超镁铁质岩体。因此，研究区域如此一致的高κ值则表明其地壳可能以镁铁质岩石为主。此外，流体和部分熔融体的存在也可使κ值增大（Watanabe，1993），特别在伍德拉克裂谷中心区域存在大量岩浆活动（图1）。古迪纳夫盆地和基里比斯盆地存在异常鲜明的地壳结构（厚度与纵横波波速比值），下面将对这两个区域展开详细讨论。

    图  5  方位校正后地壳的平面（a，b）与剖面（c）结果图
    （a） 地壳厚度平面分布图；（b） 纵横波速比值κ平面分布图；（c） 地壳厚度（蓝色三角形）和地壳纵横波速比值（红色圆圈）沿图（a）中AA′测线的剖面结果图，其中上图为地形起伏，中图红色波形为时深转换后的叠加接收函数
    Figure  5.  Planar （a，b） and vertical （c） display of the resulting crustal measurements after misorientation corrections
    （a，b） Crustal thickness and vP/vS ratio in planar view，respectively；（c） Vertical display of crustal thickness （blue triangles） and vertical display of crustal vP/vS ratio （red circles） along the AA′ profile in fig.（a）. The upper panel shows the topography，red traces in the middle panel are the stacked receiver function traces after time-depth conversion
    下载: 全尺寸图片 幻灯片

    古迪纳夫盆地布置的四台OBS分布于伍德拉克裂谷扩张轴附近（图1），四台OBS下的地壳厚度由裂谷两侧36.7 km减薄至裂谷扩张轴处30.4 km （图5），扩张轴附近的地壳平均厚度为（33.3±2.42） km。Abers等（2016）也观测到了类似的地壳减薄趋势。因此，裂谷的张裂活动在地壳减薄中可能起到主要作用。前人研究大多集中在当特尔卡斯托群岛及其周边区域（Zelt et al，2001；Abers et al，2002，2016；Ferris et al，2006），其中接收函数相关研究显示：此群岛地区的地壳有明显减薄（大约20 km）；当特尔卡斯托群岛和裂谷地区的上地幔呈现出大范围的低速异常（Abers et al，2002；Ferris et al，2006；Eilon et al，2015；Jin et al，2015），然而此群岛的地壳大致由长英质或中间质岩石组成（Ferris et al，2006）。靠近当特尔卡斯托群岛处κ值呈增大的趋势（图5），可能是与古俯冲残片的脱水有关。远震体波层析成像观测到上地幔中存在高速异常的古俯冲残片（Eilon et al，2015；Yu et al，2022），在其沉降过程中会把脱水作用产生的轻物质带到浅层地壳，从而改变地壳组成进而导致其高κ值，并可能激发超高压岩石的出露（Zheng，2009；Little et al，2011；Yu et al，2022）。

    由三台OBS计算得到的基里比斯盆地地区的地壳平均厚度为（24.1±5.44） km，相比于古迪纳夫盆地要薄得多，平均κ值为1.99±0.041。虽然地壳厚度观测结果与面波-接收函数联合反演的结果（地壳厚度范围为31.5—39.2km，平均值为35.8km）相差较大（Abers et al，2016），但与此地区早期主动源地震折射研究获得的地壳结构（地壳厚度为22—24 km）一致（Finlayson et al，1976，1977）。同时，瑞雷波层析成像结果显示基里比斯盆地在20 km以下更深处不存在明显的速度不连续界面，在30—60 km的浅层地幔处呈强横波慢速异常（Jin et al，2015），与本文观测到的较薄地壳厚度一致。另外，基于伍德拉克裂谷海域的四条地震测线（图1）开展的构造重建和沉降研究分析显示，测线1181所处的地壳水平伸展了21.72 km，比其余三条测线1168，1190和1193的伸展量都大（对应地壳水平伸展量依次为18.2，18.3和17.0 km）（Fitz，Mann，2013）。前期（Eilon et al，2015）和近期（Yu et al，2022）远震体波层析成像研究表明在基里比斯盆地100 km地幔深度处存在高速异常和地震活动，这可能是由于古俯冲残片脱水熔融导致上覆地壳的减薄并改变地壳的局部组成，从而引起κ值的增大。

    3.   结论

    本文通过纵波和瑞雷波偏振分析确定了伍德拉克裂谷地区七台OBS水平分量的方位，利用方位校正后的地震波形数据开展接收函数的地壳构造研究，主要得到以下结论：

    1） 伍德拉克裂谷大部分OBS存在水平分量的方位偏差，在开展基于三分量波形数据地震学研究时需对其进行校正；

    2） 古迪纳夫盆地的地壳厚度由裂谷两侧向裂谷扩张轴处逐渐变薄，而位于裂谷西北部的基里比斯盆地的地壳更薄，可能是古俯冲残片脱水熔融改造的结果；

    3） 研究区域整体呈现较大的纵横波速比值，可能是巴布亚超镁铁质体的存在和古俯冲残片脱水熔融共同作用的结果。

    本文研究揭示出古俯冲残片在汇聚构造背景下的大陆裂谷起始张裂过程中起到推动作用，后续将进一步开展高精度深部构造研究，揭示其作用机制与过程。

    本文附图和附表请参见本刊网站https://www.dzxb.org/cn/article/doi/10.11939/jass.20220091上的资源附件。

  • 图  1   地震数据分布图

    （a） 地震记录关于矩震级和断层距离的分布；（b） 地震事件关于矩震级和断层深度的分布

    Figure  1.   The distribution of earthquake dataset （a） The distribution of earthquake records with respect to moment magnitude and source distance；（b） The distribution of earthquakes with respect to moment magnitude and fault depth

    下载: 全尺寸图片 幻灯片

    图  2   四类场地阻尼修正系数DMF的几何均值分布情况

    Figure  2.   The geometric mean values of DMF for four site classes

    （a） ζ＝1%；（b） ζ＝3%；（c） ζ＝15% ；（d） ζ＝30%

    下载: 全尺寸图片 幻灯片

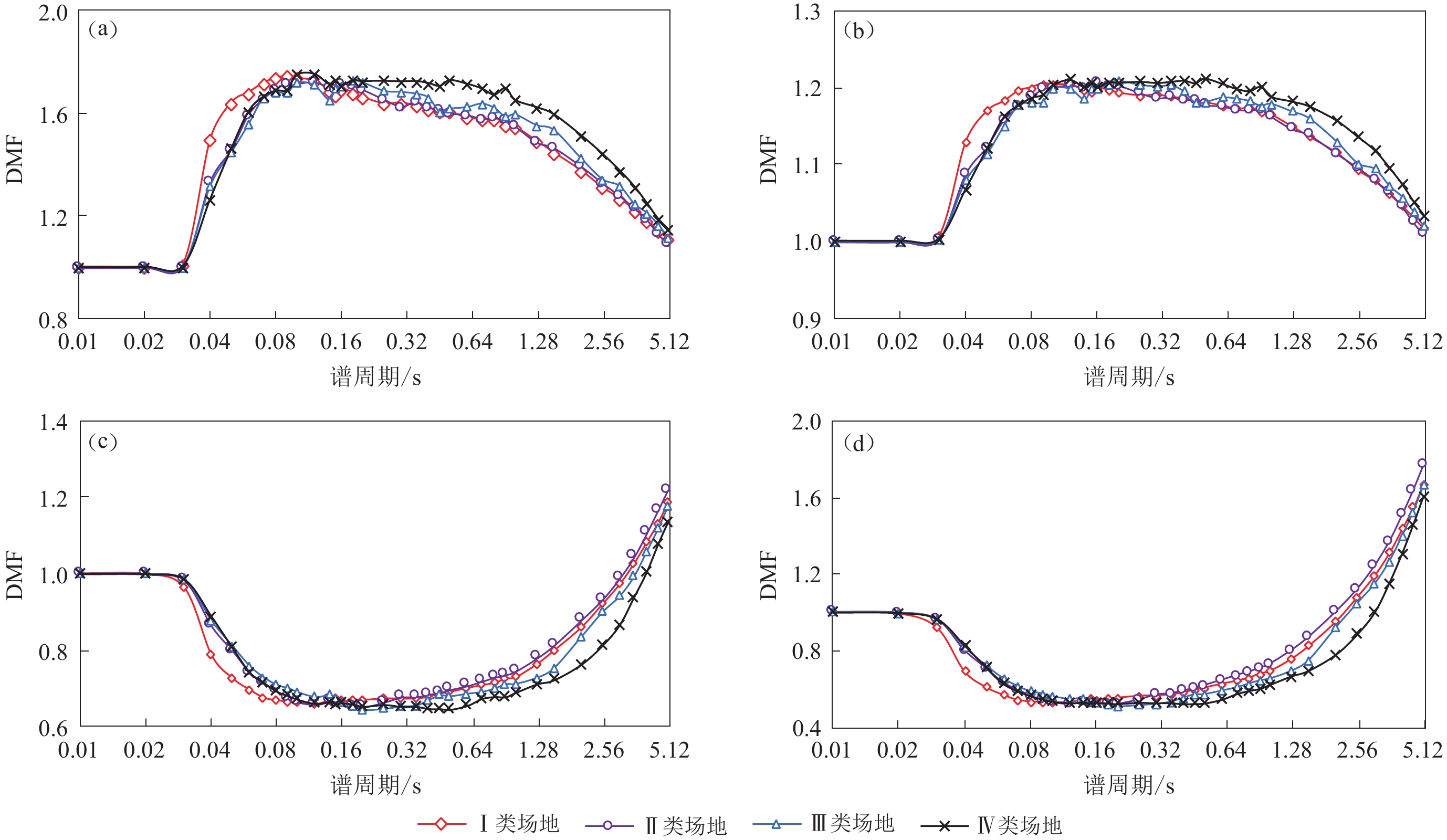
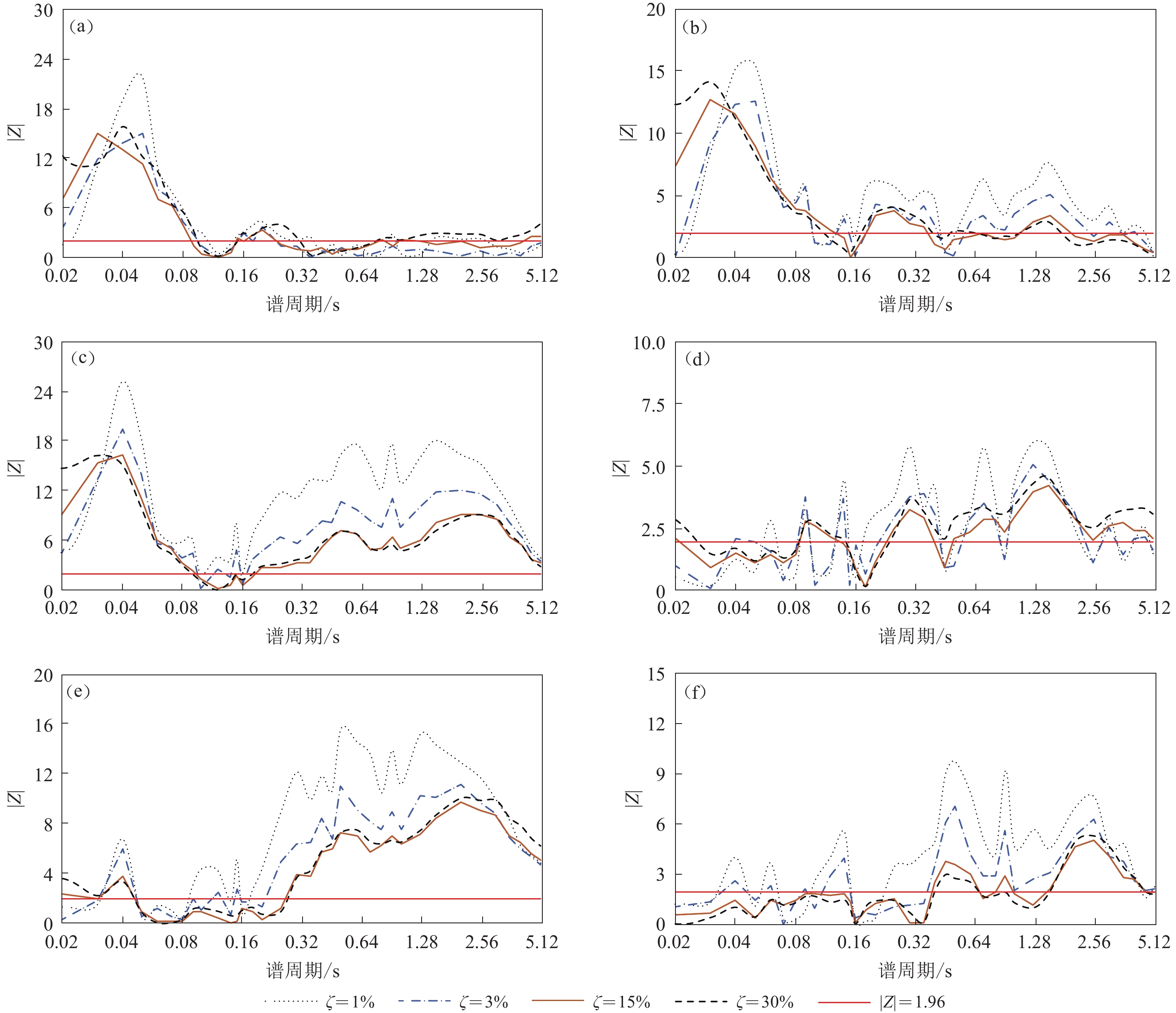
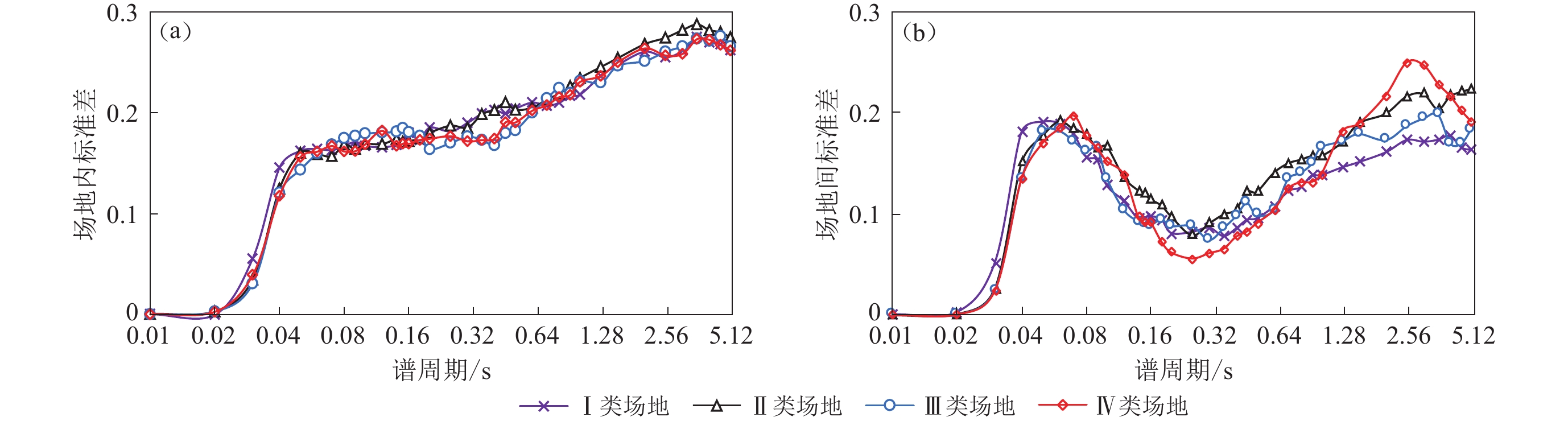
    图  3   四类场地间显著性检验统计值|Z|

    （a） Ⅰ类场地与Ⅱ类场地；（b） Ⅰ类场地与Ⅲ类场地；（c） Ⅰ类场地与Ⅳ类场地；（d） Ⅱ类场地与Ⅲ类场地；（e） Ⅱ类场地与Ⅳ类场地；（f） Ⅲ类场地与Ⅳ类场地

    Figure  3.   |Z| values for the statistical tests between each pair of four site classes

    （a） Site class Ⅰ vs site class Ⅱ；（b） Site class Ⅰ vs site class Ⅲ；（c） Site class Ⅰ vs site class Ⅳ； （d） Site class Ⅱ vs site class Ⅲ；（e） Site class Ⅱ vs site class Ⅳ；（f） Site class Ⅲ vs site class Ⅳ

    下载: 全尺寸图片 幻灯片

    图  4   四类场地不同阻尼比的阻尼修正系数DMF模型拟合曲线与数据几何均值的对比

    （a） Ⅰ类场地；（b） Ⅱ类场地；（c） Ⅲ类场地；（d） Ⅳ类场地

    Figure  4.   DMF model comparisons with the geometrical mean of the vertical components for eight damping ratios of four site classes

    （a） Site class Ⅰ；（b） Site class Ⅱ；（c） Site class Ⅲ；（d） Site class Ⅳ

    下载: 全尺寸图片 幻灯片

    图  5   阻尼修正系数DMF模型的残差标准差关于谱周期的分布图

    Figure  5.   The distribution of standard deviations of DMF models

    （a） ζ＝1%；（b） ζ＝3%；（c） ζ＝10%；（d） ζ＝30%

    下载: 全尺寸图片 幻灯片

    图  6   阻尼比为25%时四类场地的场地内标准差（a）和场地间标准差（b）分布图

    Figure  6.   Distribution of within-site （a） and between-site （b） standard deviation for four site classes for a damping ratio of 25%

    下载: 全尺寸图片 幻灯片

    图  7   T＝2.5 s时阻尼修正系数DMF模型标准差关于对数坐标系下阻尼比的变化图

    （a） Ⅰ类场地；（b） Ⅱ类场地；（c） Ⅲ类场地；（d） Ⅳ类场地

    Figure  7.   Variations of standard deviation of DMF model with damping ratio at logarithmic scale by T＝2.5 s

    （a） Site class Ⅰ；（b） Site class Ⅱ；（c） Site class Ⅲ；（d） Site class Ⅳ

    下载: 全尺寸图片 幻灯片

    图  8   阻尼比为25%时阻尼修正系数DMF模型事件间残差分布图

    （a） T＝0.1 s时残差关于断层深度的分布图；（b） T＝0.1 s时残差关于矩震级的分布图；（c） T＝3.0 s时残差关于断层深度的分布图；（d） T＝3.0 s时残差关于矩震级的分布图

    Figure  8.   The distributions of between-event residuals of DMF model for a damping ratio of 25%

    （a） The distribution of residuals with respect to fault depth at T＝0.1 s；（b） The distribution of residuals with respect to moment magnitude at T＝0.1 s；（c） The distribution of residuals with respect to fault depth at T＝3.0 s；（d） The distribution of residuals with respect to moment magnitude at T＝3.0 s

    下载: 全尺寸图片 幻灯片

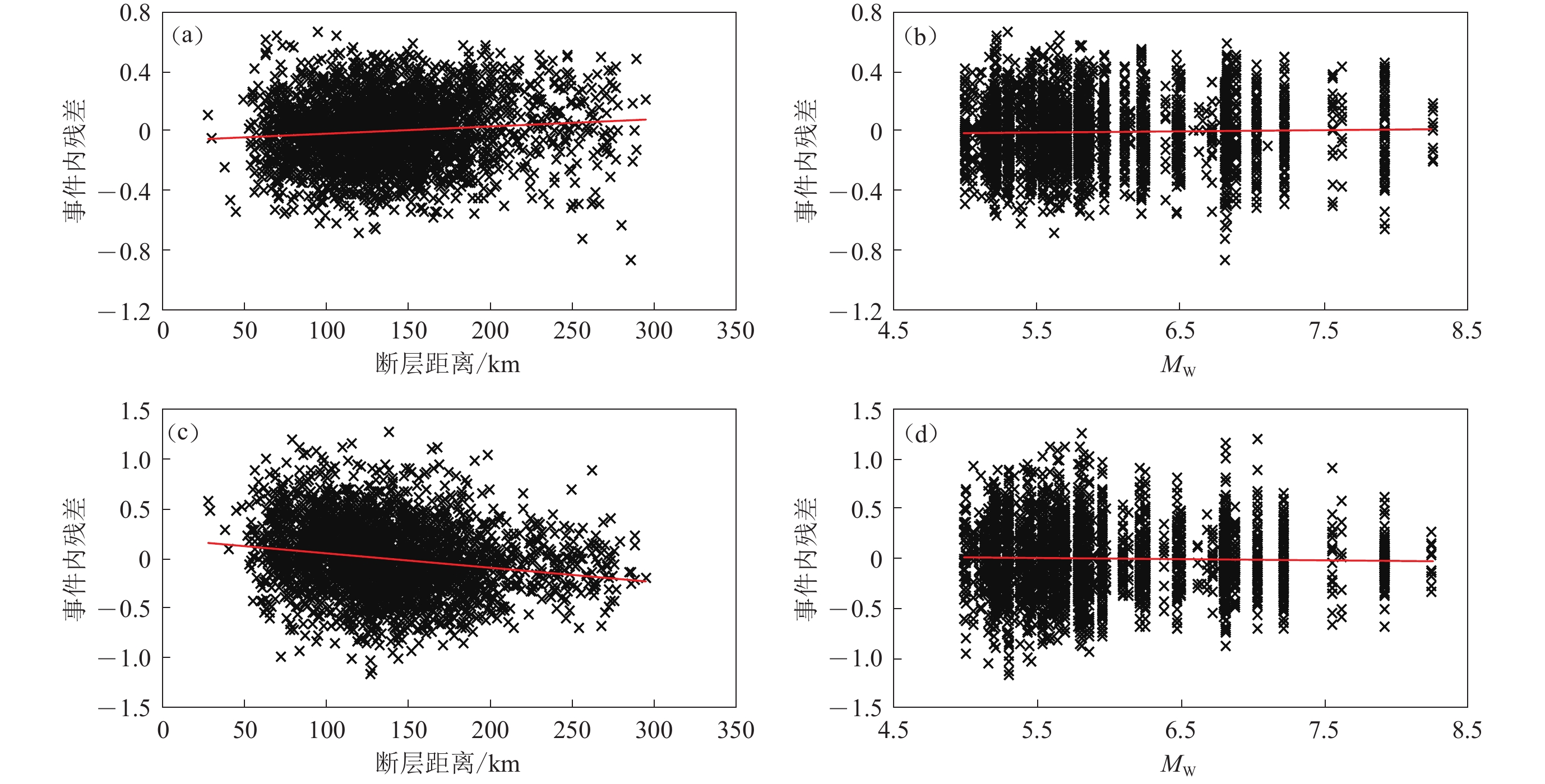
    图  9   阻尼比为25%时DMF模型事件内残差分布图

    （a） T＝0.1 s时残差关于断层距离的分布图；（b） T＝0.1 s时残差关于矩震级的分布图；（c） T＝3.0 s时残差关于断层距离的分布图；（d） T＝3.0 s时残差关于矩震级的分布图

    Figure  9.   The distributions of within-event residuals of DMF model for a damping ratio of 25%

    （a） The distribution of residuals with respect to fault distance at T＝0.1 s；（b） The distribution of residuals with respect to moment magnitude at T＝0.1 s；（c） The distribution of residuals with respect to fault distance at T＝3.0 s；（d） The distribution of residuals with respect to moment magnitude at T＝3.0 s

    下载: 全尺寸图片 幻灯片

    表  1   场地类别定义和各类场地记录数量

    Table  1   Site class definition and number of the records in each site class

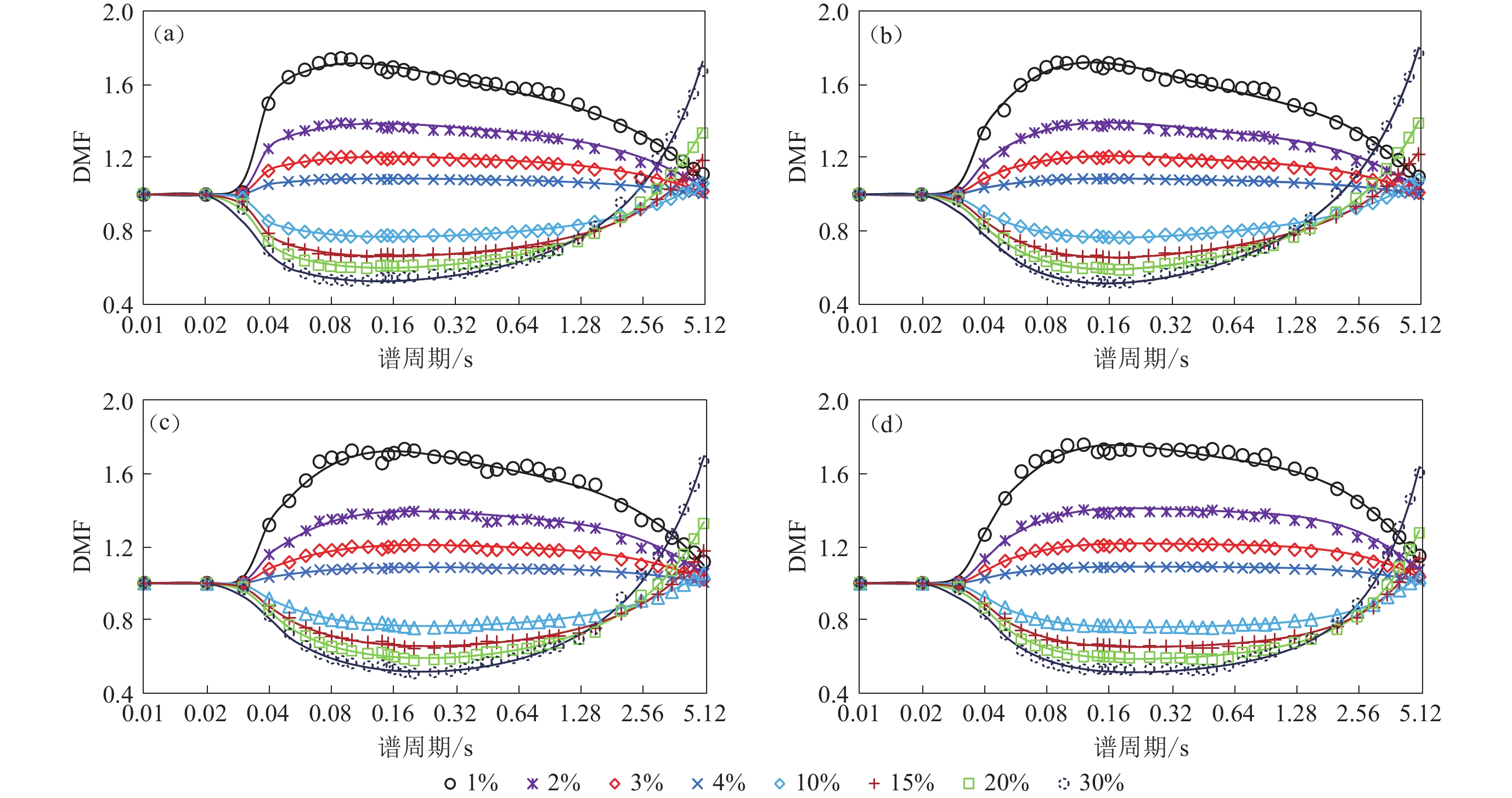
    场地类别土质类型场地周期/s记录条数
    Ⅰ岩石Ts＜0.22 022
    Ⅱ硬土0.2≤Ts＜0.41 353
    Ⅲ中硬土0.4≤Ts＜0.6442
    Ⅳ软土Ts≥0.6878
    下载: 导出CSV

    表  2   四类场地下阻尼修正系数模型的系数值

    Table  2   Coefficients of DMF model for four site classes

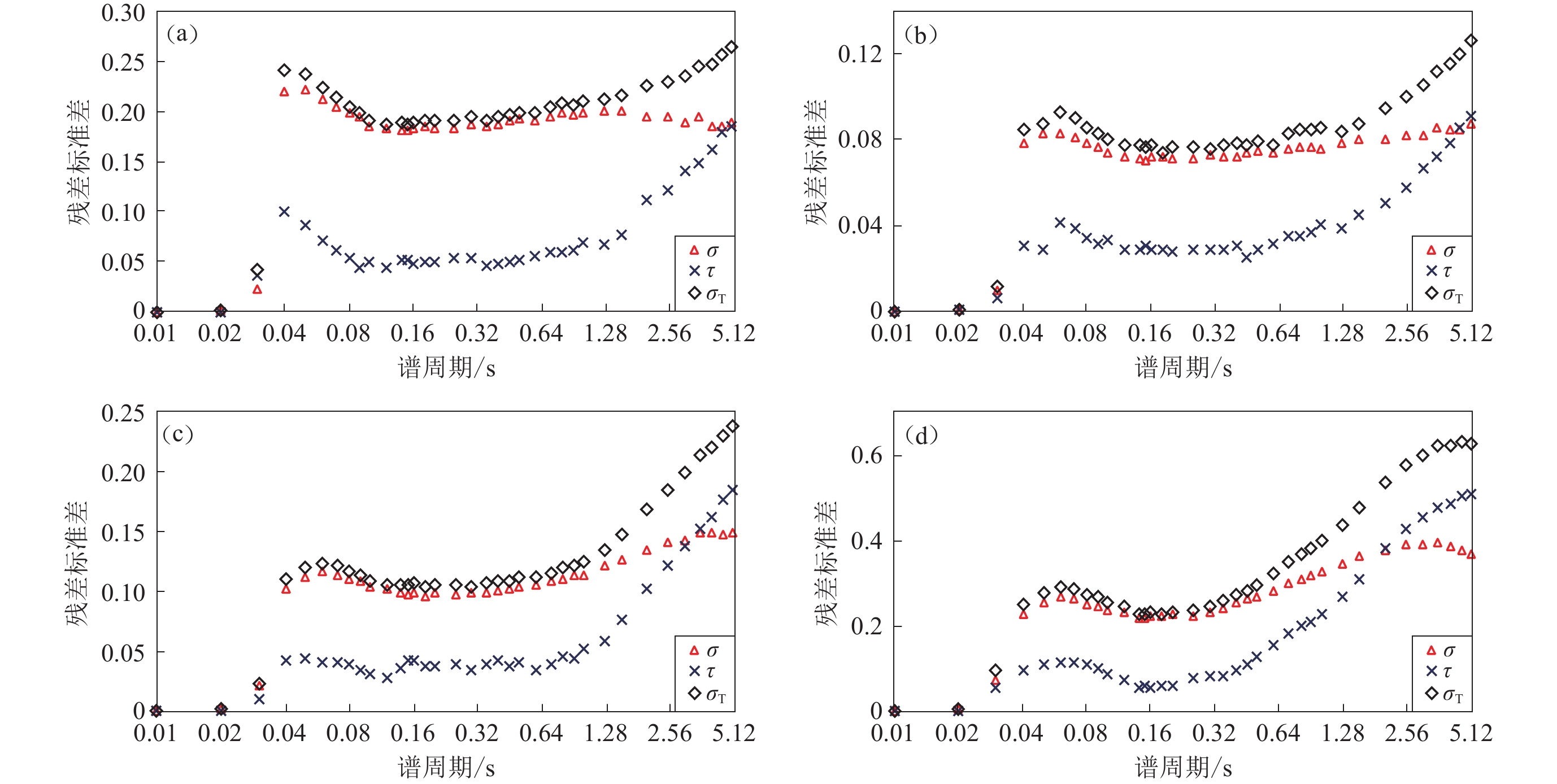
    T/sⅠ类场地Ⅱ类场地Ⅲ类场地Ⅳ类场地
    $ {c}_{1} $$ {c}_{2} $$ {c}_{3} $$ {c}_{1} $$ {c}_{2} $$ {c}_{3} $$ {c}_{1} $$ {c}_{2} $$ {c}_{3} $$ {c}_{1} $$ {c}_{2} $$ {c}_{3} $
    0.03 −0.020 0 −0.011 3 −0.015 0 −0.020 0 −0.005 4 −0.008 0 −0.008 3 −0.004 8 −0.008 0 −0.007 1 −0.004 7 −0.010 0
    0.04 −0.234 3 0.012 9 0.001 0 −0.152 1 0.016 7 −0.005 0 −0.138 9 0.018 3 −0.004 0 −0.120 8 0.013 3 −0.004 0
    0.05 −0.294 9 0.005 7 0.000 1 −0.235 9 0.012 9 0.001 4 −0.213 8 0.012 3 −0.001 1 −0.220 4 0.013 0 0.002 4
    0.06 −0.322 8 0.001 8 0.002 6 −0.283 5 0.006 7 0.003 1 −0.262 1 0.009 1 0.001 9 −0.273 8 0.008 2 0.005 0
    0.07 −0.340 8 −0.001 1 0.004 4 −0.314 7 0.002 1 0.004 4 −0.294 4 0.006 1 0.003 8 −0.308 7 0.004 7 0.006 6
    0.08 −0.352 8 −0.003 2 0.005 9 −0.335 7 −0.001 4 0.005 4 −0.317 1 0.003 4 0.005 1 −0.332 4 0.002 0 0.007 7
    0.09 −0.361 1 −0.004 9 0.007 1 −0.350 3 −0.004 0 0.006 2 −0.333 4 0.001 0 0.006 1 −0.349 1 −0.000 1 0.008 5
    0.10 −0.366 8 −0.006 3 0.008 1 −0.360 5 −0.006 1 0.007 0 −0.345 4 −0.001 0 0.006 8 −0.361 1 −0.001 8 0.009 1
    0.12 −0.373 5 −0.008 2 0.009 7 −0.372 8 −0.008 9 0.008 2 −0.361 2 −0.004 4 0.007 9 −0.376 3 −0.004 3 0.009 9
    0.14 −0.376 5 −0.009 4 0.011 0 −0.378 8 −0.010 6 0.009 3 −0.370 3 −0.007 0 0.008 7 −0.384 6 −0.006 1 0.010 5
    0.15 −0.377 1 −0.009 8 0.011 5 −0.380 3 −0.011 2 0.009 9 −0.373 4 −0.008 1 0.009 0 −0.387 3 −0.006 7 0.010 8
    0.16 −0.377 4 −0.010 1 0.012 1 −0.381 2 −0.011 6 0.010 4 −0.375 7 −0.009 0 0.009 3 −0.389 3 −0.007 3 0.011 0
    0.18 −0.377 1 −0.010 5 0.013 0 −0.381 6 −0.012 0 0.011 3 −0.378 8 −0.010 5 0.009 9 −0.391 8 −0.008 2 0.011 4
    0.20 −0.376 2 −0.010 7 0.013 9 −0.380 8 −0.012 1 0.012 2 −0.380 4 −0.011 6 0.010 5 −0.393 1 −0.008 8 0.011 8
    0.25 −0.372 3 −0.010 4 0.015 7 −0.376 3 −0.011 3 0.014 2 −0.381 1 −0.013 1 0.011 8 −0.393 4 −0.009 6 0.012 9
    0.30 −0.367 6 −0.009 5 0.017 2 −0.370 3 −0.009 7 0.016 0 −0.379 3 −0.013 5 0.013 1 −0.392 2 −0.009 7 0.013 9
    0.35 −0.362 7 −0.008 3 0.018 6 −0.364 2 −0.007 7 0.017 6 −0.376 6 −0.013 1 0.014 4 −0.390 6 −0.009 5 0.014 9
    0.40 −0.357 9 −0.006 9 0.019 8 −0.358 2 −0.005 5 0.019 2 −0.373 5 −0.012 2 0.015 6 −0.388 9 −0.008 9 0.016 0
    0.45 −0.353 2 −0.005 4 0.020 8 −0.352 5 −0.003 1 0.020 5 −0.370 1 −0.011 0 0.016 8 −0.387 3 −0.008 2 0.017 0
    0.50 −0.348 6 −0.003 8 0.021 8 −0.347 1 −0.000 8 0.021 8 −0.366 7 −0.009 5 0.018 0 −0.385 7 −0.007 3 0.018 0
    0.60 −0.339 8 −0.000 5 0.023 6 −0.337 0 0.003 9 0.024 1 −0.359 9 −0.006 2 0.020 2 −0.382 5 −0.005 4 0.020 0
    0.70 −0.331 3 0.002 9 0.025 0 −0.327 7 0.008 4 0.026 0 −0.353 1 −0.002 6 0.022 2 −0.379 3 −0.003 1 0.021 8
    0.80 −0.323 0 0.006 2 0.026 3 −0.319 0 0.012 8 0.027 7 −0.346 2 0.001 1 0.024 1 −0.375 9 −0.000 8 0.023 5
    0.90 −0.314 9 0.009 4 0.027 5 −0.310 6 0.016 9 0.029 2 −0.339 2 0.004 8 0.025 8 −0.372 2 0.001 6 0.025 1
    1.00 −0.306 8 0.012 6 0.028 5 −0.302 4 0.020 9 0.030 5 −0.332 2 0.008 5 0.027 3 −0.368 1 0.004 1 0.026 6
    1.25 −0.286 8 0.020 2 0.030 6 −0.282 5 0.030 0 0.033 1 −0.314 2 0.017 4 0.030 5 −0.356 2 0.010 4 0.029 8
    1.50 −0.266 7 0.027 4 0.032 1 −0.262 7 0.038 1 0.034 9 −0.295 4 0.025 6 0.033 1 −0.342 1 0.016 7 0.032 4
    2.00 −0.226 4 0.040 4 0.034 2 −0.222 5 0.052 1 0.037 2 −0.256 0 0.040 4 0.036 5 −0.307 9 0.029 0 0.036 2
    2.50 −0.185 6 0.052 2 0.035 3 −0.181 3 0.063 7 0.038 1 −0.214 6 0.053 0 0.038 4 −0.267 9 0.040 9 0.038 7
    3.00 −0.144 7 0.062 9 0.035 8 −0.139 1 0.073 8 0.038 1 −0.171 9 0.064 0 0.039 1 −0.223 6 0.052 3 0.040 3
    3.50 −0.103 8 0.072 8 0.035 9 −0.096 2 0.082 6 0.037 6 −0.128 3 0.073 7 0.039 1 −0.176 3 0.063 2 0.041 1
    4.00 −0.063 1 0.082 0 0.035 6 −0.052 9 0.090 4 0.036 6 −0.084 1 0.082 2 0.038 4 −0.126 9 0.073 8 0.041 3
    4.50 −0.022 6 0.090 6 0.035 1 −0.009 2 0.097 4 0.035 2 −0.039 6 0.089 8 0.037 3 −0.075 9 0.084 0 0.041 1
    5.00 0.017 7 0.098 7 0.034 4 0.034 6 0.103 8 0.033 6 0.005 0 0.096 7 0.035 8 −0.023 8 0.093 9 0.040 6
    下载: 导出CSV

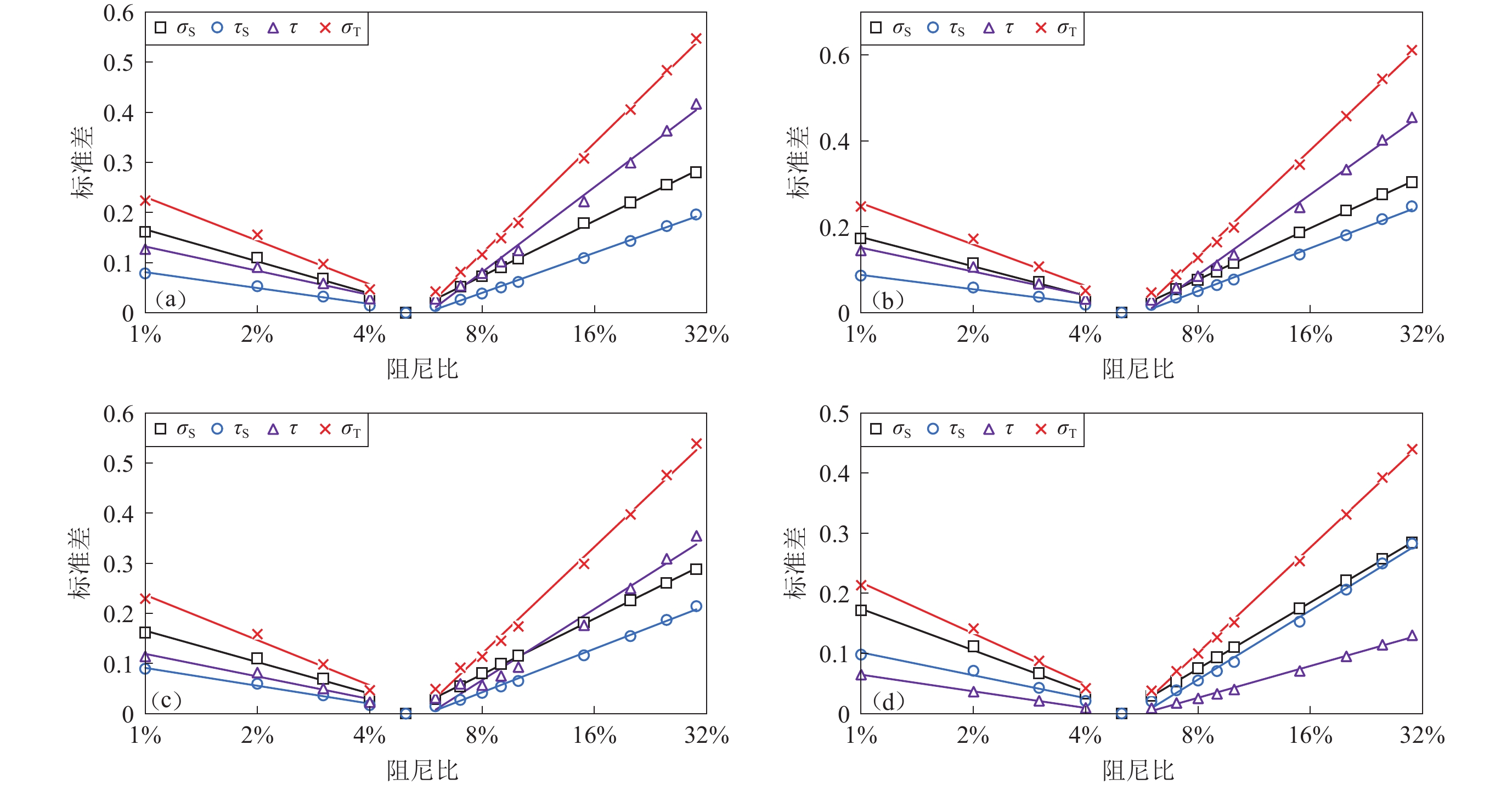
    表  3   阻尼修正系数模型的总残差标准差${\sigma _T} $

    Table  3   Total standard deviations ${\sigma _T} $ of DMF model

    T/sσT
    ζ＝1%ζ＝2%ζ＝3%ζ＝4%ζ＝6%ζ＝7%ζ＝8%ζ＝9%ζ＝10%ζ＝15%ζ＝20%ζ＝25%ζ＝30%
    0.030.056 00.014 70.011 60.005 70.000 00.005 40.010 40.015 20.019 80.024 30.046 00.066 50.086 4
    0.040.242 60.146 30.084 40.039 90.030 80.055 70.079 90.094 80.110 70.167 60.204 10.229 50.248 6
    0.050.238 60.148 70.087 90.042 50.033 00.060 10.082 90.102 70.120 20.184 10.225 50.255 40.277 7
    0.060.225 00.144 40.092 90.042 70.035 80.061 40.090 20.105 60.123 70.190 90.235 10.267 00.290 8
    0.070.215 50.139 60.090 00.041 50.034 60.059 80.088 00.103 00.120 70.187 60.232 00.263 60.287 1
    0.080.206 30.141 40.085 50.039 80.033 20.057 50.084 00.099 00.115 90.178 70.220 10.250 30.273 1
    0.090.200 40.136 40.082 70.038 50.030 80.056 20.082 30.096 20.112 70.174 60.215 70.245 10.266 9
    0.100.192 70.132 40.080 70.037 30.029 50.053 70.078 70.097 70.107 80.165 90.205 10.233 30.254 2
    0.120.188 50.122 90.077 80.034 50.030 50.055 60.076 60.094 80.105 70.160 90.197 30.223 80.243 6
    0.140.189 90.123 00.077 20.035 70.029 70.053 70.073 40.090 30.104 60.157 00.184 40.208 30.227 0
    0.150.188 80.122 10.076 80.033 10.029 40.053 40.069 30.090 80.105 90.159 40.194 30.208 60.227 8
    0.160.190 80.122 60.077 60.033 90.030 10.054 40.074 80.092 20.107 10.161 30.196 20.210 30.230 1
    0.180.191 80.123 90.074 00.035 20.029 00.052 60.072 00.088 50.102 90.155 70.190 80.207 50.228 1
    0.200.191 20.123 50.076 80.035 70.029 70.053 90.070 90.090 90.105 60.159 20.194 60.212 30.233 3
    0.250.191 70.124 30.077 00.035 50.029 50.053 30.069 80.085 90.104 50.150 00.184 40.211 70.235 5
    0.300.195 60.125 80.075 40.035 90.029 80.053 50.073 10.089 70.104 20.152 30.189 10.219 40.246 6
    0.350.191 20.129 80.077 50.034 00.029 50.053 40.070 00.090 90.106 40.163 80.195 20.227 40.256 7
    0.400.195 00.131 80.078 40.033 90.030 10.051 60.070 90.093 20.108 90.159 60.201 90.238 10.270 9
    0.450.198 70.128 20.077 90.035 80.029 80.054 40.072 80.089 90.108 60.163 80.208 50.247 50.283 4
    0.500.200 20.127 60.079 80.036 70.030 70.056 00.077 10.095 40.111 50.168 60.216 10.257 70.296 3
    0.600.199 60.128 40.077 50.037 70.030 10.054 90.076 10.097 80.111 40.177 90.231 70.278 70.321 8
    0.700.204 60.131 80.083 30.036 70.032 30.056 40.081 90.097 90.115 50.187 30.246 80.299 60.347 5
    0.800.208 80.133 90.084 60.037 00.033 20.058 00.084 90.100 90.119 30.196 40.260 30.316 30.367 4
    0.900.207 70.132 60.085 00.036 90.033 40.057 80.086 00.102 10.121 30.202 30.270 00.329 00.382 3
    1.000.211 50.134 70.086 10.039 80.031 80.058 90.083 20.104 90.125 00.210 30.281 60.343 50.398 6
    1.250.212 40.139 30.084 30.041 00.033 80.063 00.088 80.112 60.134 90.230 70.309 60.377 60.436 5
    1.500.216 50.143 50.087 80.041 00.036 10.067 90.096 30.122 60.147 10.252 20.340 20.413 00.475 9
    2.000.226 10.153 80.095 20.045 10.040 40.076 70.109 00.139 10.167 80.290 50.389 20.470 30.536 1
    2.500.231 10.160 70.100 60.048 00.043 90.083 50.119 70.153 30.185 10.321 30.426 20.510 20.576 5
    3.000.237 00.166 70.105 60.050 80.046 70.089 10.128 20.164 50.198 30.341 70.450 80.533 90.598 3
    3.500.245 70.175 60.112 10.054 30.050 20.096 00.137 50.176 20.212 80.363 00.473 30.556 30.619 1
    4.000.248 40.179 30.115 30.055 50.051 50.098 90.142 60.183 20.220 50.372 70.481 90.561 40.621 2
    4.500.258 30.187 30.120 40.058 40.054 00.103 50.149 10.191 10.229 90.385 10.495 00.573 20.630 5
    5.000.264 90.194 20.126 40.061 30.056 70.108 00.154 90.197 50.237 10.391 70.498 40.573 20.627 1
    下载: 导出CSV

    表  4   阻尼修正系数模型的事件内残差标准差σ

    Table  4   Within-event standard deviation σ of DMF model

    T/sσ
    ζ＝1%ζ＝2%ζ＝3%ζ＝4%ζ＝6%ζ＝7%ζ＝8%ζ＝9%ζ＝10%ζ＝15%ζ＝20%ζ＝25%ζ＝30%
    0.030.017 80.013 80.009 70.005 10.000 00.004 90.009 40.013 70.017 60.021 30.037 90.051 10.061 9
    0.040.221 00.134 70.078 60.035 30.028 80.051 80.071 20.088 00.102 60.154 90.188 30.211 50.228 8
    0.050.222 30.140 00.083 10.037 90.031 00.056 30.077 60.096 10.112 20.171 20.209 10.236 10.256 0
    0.060.213 50.137 80.083 20.038 40.032 30.058 50.080 80.100 10.117 00.178 80.218 80.247 10.268 1
    0.070.206 40.134 00.081 10.037 40.031 20.057 00.078 60.097 30.113 70.174 80.214 50.242 40.263 1
    0.080.199 10.129 50.078 40.036 40.030 40.055 00.075 80.093 80.109 30.165 90.202 60.229 20.249 4
    0.090.195 30.126 80.076 60.035 50.029 90.054 40.074 80.092 40.107 60.163 70.200 80.227 10.246 8
    0.100.186 10.121 20.073 60.034 10.028 50.051 80.071 40.088 40.103 10.157 00.193 10.218 90.238 2
    0.120.183 30.119 80.072 40.033 50.028 20.051 30.070 70.087 40.101 80.154 20.188 50.213 60.232 3
    0.140.182 70.119 00.071 60.033 20.027 60.050 00.068 70.084 60.098 20.147 60.179 50.202 20.219 6
    0.150.181 40.117 50.070 30.032 20.027 00.049 10.067 50.083 20.097 00.146 60.178 90.202 10.220 1
    0.160.184 50.118 70.071 90.033 20.027 60.050 00.068 80.084 80.098 50.148 40.180 60.204 10.222 8
    0.180.185 30.120 00.072 30.033 10.027 30.049 40.067 50.082 70.096 10.144 70.177 10.201 10.220 6
    0.200.184 50.119 50.071 50.033 20.027 8−0.050 50.069 20.085 10.098 80.148 50.181 40.206 00.225 5
    0.250.184 00.118 80.071 40.032 90.027 30.049 30.067 80.083 40.096 90.144 50.176 50.201 10.221 7
    0.300.188 10.120 80.072 90.033 50.027 9−0.050 20.068 80.084 70.098 30.147 60.181 70.208 70.231 9
    0.350.185 60.119 70.072 10.033 30.027 70.050 10.068 70.085 00.099 20.151 90.188 50.217 70.242 9
    0.400.188 70.120 70.072 30.033 20.027 9−0.050 60.069 50.085 80.100 10.154 80.194 00.226 40.254 2
    0.450.192 20.123 60.073 60.033 70.028 30.051 40.070 80.087 30.101 80.156 90.197 70.231 80.261 7
    0.500.193 40.123 40.074 50.034 30.028 60.052 20.071 90.088 80.103 60.160 30.202 00.237 10.268 4
    0.600.191 40.122 70.074 20.034 30.029 00.052 80.072 90.090 40.105 90.165 70.211 60.250 00.283 3
    0.700.195 40.125 90.075 50.035 10.029 50.053 80.074 50.092 40.108 50.171 50.220 60.262 20.297 4
    0.800.199 80.128 10.076 80.035 50.030 10.054 70.075 60.093 80.110 20.176 30.228 30.271 80.308 7
    0.900.198 20.127 10.076 40.035 40.029 90.054 90.076 50.095 70.113 00.182 00.236 10.280 80.318 7
    1.000.199 60.127 00.075 80.035 00.029 70.054 80.076 80.096 20.113 90.185 80.242 10.287 90.326 1
    1.250.201 30.130 10.078 40.036 50.031 30.058 00.081 20.102 10.121 50.199 90.259 80.307 20.346 0
    1.500.201 90.131 70.080 00.037 20.032 40.060 10.084 60.106 50.126 40.208 10.271 40.321 30.361 7
    2.000.196 50.130 10.080 40.038 10.033 70.063 10.088 90.112 20.133 50.219 60.284 90.336 00.376 7
    2.500.195 70.132 50.082 40.039 20.035 00.065 40.092 50.117 20.139 90.232 20.299 50.351 30.390 6
    3.000.190 20.130 30.082 10.039 50.035 40.066 60.094 40.119 60.142 40.234 60.302 10.352 00.390 3
    3.500.194 90.135 90.086 00.041 20.036 90.069 50.098 40.124 70.148 30.243 00.309 70.358 20.395 1
    4.000.187 00.132 20.084 80.040 80.037 00.069 80.099 20.125 70.149 50.242 60.307 10.353 00.387 1
    4.500.185 70.132 60.085 00.041 10.037 00.069 50.098 50.124 50.147 90.239 30.301 50.345 30.378 0
    5.000.189 10.135 90.087 40.042 10.037 90.070 50.099 40.125 20.148 30.237 20.296 40.337 80.368 3
    下载: 导出CSV

    表  5   阻尼修正系数模型的事件间残差标准差τ

    Table  5   Between-event standard deviation τ of DMF model

    T/sτ
    ζ＝1%ζ＝2%ζ＝3%ζ＝4%ζ＝6%ζ＝7%ζ＝8%ζ＝9%ζ＝10%ζ＝15%ζ＝20%ζ＝25%ζ＝30%
    0.030.053 10.004 90.006 30.002 50.000 00.002 20.004 40.006 70.009 10.011 70.026 10.042 60.060 3
    0.040.100 10.056 90.030 90.018 60.011 00.020 50.036 20.035 30.041 50.064 00.078 60.089 10.097 1
    0.050.086 70.050 10.028 40.019 40.011 30.020 90.029 10.036 40.043 00.067 70.084 40.097 40.107 6
    0.060.071 00.043 30.041 40.018 60.015 40.018 60.040 00.033 50.040 10.066 90.086 20.101 20.112 7
    0.070.062 10.039 30.039 00.017 80.014 80.018 00.039 70.033 90.040 60.068 00.088 30.103 50.114 9
    0.080.053 80.056 80.034 00.016 00.013 30.016 70.036 10.031 70.038 30.066 30.086 00.100 50.111 4
    0.090.044 70.050 50.031 20.014 90.007 40.014 30.034 30.027 00.033 70.060 60.078 90.092 20.101 7
    0.100.050 10.053 40.033 10.015 10.007 50.014 10.033 20.041 70.031 60.053 60.069 10.080 80.088 8
    0.120.044 00.027 60.028 50.008 10.011 60.021 30.029 40.036 70.028 20.046 10.058 30.066 90.073 3
    0.140.051 80.031 10.028 70.013 00.010 90.019 50.025 90.031 50.035 80.053 50.042 10.050 20.057 5
    0.150.052 30.033 30.031 00.007 90.011 70.021 10.015 70.036 40.042 60.062 50.075 90.051 70.058 7
    0.160.048 60.030 50.029 10.006 80.012 00.021 40.029 40.036 30.042 10.063 20.076 70.050 60.057 5
    0.180.049 50.030 70.028 50.011 90.010 00.018 20.025 30.031 50.036 80.057 40.071 00.051 00.057 7
    0.200.050 00.031 00.027 90.013 00.010 40.019 00.015 30.032 00.037 20.057 20.070 40.051 60.059 5
    0.250.053 60.036 40.028 70.013 50.011 20.020 10.016 70.020 70.039 00.040 40.053 60.066 10.079 4
    0.300.053 80.035 30.028 60.013 00.010 40.018 40.024 50.029 50.034 40.037 60.052 40.067 60.084 0
    0.350.045 90.050 10.028 50.006 80.010 10.018 30.013 40.032 40.038 40.061 40.050 70.065 60.083 0
    0.400.049 30.053 10.030 30.007 20.011 30.010 00.014 10.036 30.042 80.038 60.055 70.073 70.093 7
    0.450.050 50.034 20.025 40.012 20.009 40.017 80.017 00.021 60.037 80.047 10.066 30.086 90.108 7
    0.500.051 60.032 50.028 50.013 20.011 00.020 10.027 70.034 70.041 10.052 30.076 90.101 00.125 5
    0.600.056 40.037 70.031 90.015 40.008 00.015 20.021 70.037 20.034 70.064 80.094 30.123 30.152 8
    0.700.060 50.039 10.035 20.010 80.013 20.016 80.034 00.032 20.039 60.075 30.110 60.144 90.179 7
    0.800.060 80.038 90.035 50.010 70.014 10.019 20.038 60.037 00.045 70.086 30.125 10.161 90.199 3
    0.900.062 20.037 90.037 30.010 30.014 80.018 20.039 30.035 40.044 10.088 40.131 00.171 50.211 1
    1.000.069 70.044 80.040 70.019 00.011 30.021 60.031 90.041 80.051 70.098 60.143 80.187 30.229 1
    1.250.067 60.049 90.038 50.018 60.012 80.024 50.036 00.047 50.058 70.115 00.168 30.219 50.266 2
    1.500.078 10.057 10.044 70.017 10.016 00.031 50.046 00.060 70.075 30.142 60.205 20.259 50.309 3
    2.000.111 80.082 00.050 90.024 20.022 30.043 50.063 10.082 20.101 60.190 20.265 20.329 10.381 4
    2.500.123 00.091 00.057 70.027 80.026 50.051 90.076 00.098 90.121 20.222 10.303 30.370 00.423 9
    3.000.141 40.103 90.066 40.032 00.030 40.059 10.086 80.112 90.138 00.248 50.334 60.401 40.453 5
    3.500.149 60.111 20.071 80.035 30.034 10.066 20.096 00.124 50.152 60.269 60.357 90.425 60.476 6
    4.000.163 60.121 10.078 20.037 70.035 90.070 10.102 40.133 30.162 00.282 90.371 40.436 50.485 8
    4.500.179 50.132 30.085 30.041 40.039 30.076 70.111 90.145 00.176 00.301 70.392 60.457 50.504 5
    5.000.185 60.138 70.091 30.044 60.042 20.081 90.118 80.152 80.185 00.311 70.400 70.463 10.507 5
    下载: 导出CSV
    • 参考文献(25)
  • 郝安民,周德源,李亚明,张晖. 2012. 考虑震级影响的规范阻尼修正系数评估[J]. 同济大学学报(自然科学版),40(5):657–661.

    Hao A M,Zhou D Y,Li Y M,Zhang H. 2012. Evaluation of damping modification factors in codes with a consideration of effect of earthquake magnitude[J]. Journal of Tongji University (Natural Science),40(5):657–661 (in Chinese).
    蒋建,吕西林,周颖,蒋欢军. 2009. 考虑场地类别的阻尼比修正系数研究[J]. 地震工程与工程振动,29(1):153–161.

    Jiang J,Lü X L,Zhou Y,Jiang H J. 2009. Research on modification factors of damping ratios considering site conditions[J]. Journal of Earthquake Engineering and Engineering Vibration,29(1):153–161 (in Chinese).

    Abrahamson N A,Youngs R R. 1992. A stable algorithm for regression analyses using the random effects model[J]. Bull Seismol Soc Am,82(1):505–510. doi: 10.1785/BSSA0820010505

    Ambraseys N N,Douglas J. 2003. Near-field horizontal and vertical earthquake ground motions[J]. Soil Dyn Earthq Eng,23(1):1–18. doi: 10.1016/S0267-7261(02)00153-7

    Bozorgnia Y,Campbell K W. 2004. The vertical-to-horizontal response spectral ratio and tentative procedures for developing simplified V/H and vertical design spectra[J]. J Earthq Eng,8(2):175–207.

    Brillinger D R,Preisler H K. 1984. An exploratory analysis of the Joyner-Boore attenuation data[J]. Bull Seismol Soc Am,74(4):1441–1450.

    Japan Road Association. 1980. Specifications for Highway Bridges. Part V, Seismic Design[S]. Tokyo: Maruzen Company Limited: 24–25.

    Jiang F,Zhao J X. 2017. A ground‐motion prediction equation for vertical spectra of the strong‐motion records from the subduction slab events in Japan using site class as the site term[J]. Bull Seismol Soc Am,107(5):2328–2341. doi: 10.1785/0120160387

    Li H,Chen F. 2017. Damping modification factors for acceleration response spectra[J]. Geodesy Geodyn,8(5):361–370. doi: 10.1016/j.geog.2017.04.009

    Lin Y Y,Chang K C. 2003. Study on damping reduction factor for buildings under earthquake ground motions[J]. J Struct Eng,129(2):206–214. doi: 10.1061/(ASCE)0733-9445(2003)129:2(206)

    Lin Y Y,Chang K C. 2004. Effects of site classes on damping reduction factors[J]. J Struct Eng,130(11):1667–1675. doi: 10.1061/(ASCE)0733-9445(2004)130:11(1667)

    Newmark N M, Hall W J. 1982. Earthquake Spectra and Design[M]. Berkeley: Earthquake Engineering Research Institute: 1–103.

    Rezaeian S,Bozorgnia Y,Idriss I M,Abrahamson N,Campbell K,Silva W. 2014. Damping scaling factors for vertical elastic response spectra for shallow crustal earthquakes in active tectonic regions[J]. Earthq Spectra,30(3):1335–1358. doi: 10.1193/100512EQS298M

    Xiang Y,Huang Q L. 2019. Damping modification factor for the vertical seismic response spectrum:A study based on Japanese earthquake records[J]. Eng Struct,179:493–511. doi: 10.1016/j.engstruct.2018.11.021

    Zhao J X,Irikura K,Zhang J,Fukushima Y,Somerville P G,Asano A,Ohno Y,Oouchi T,Takahashi T,Ogawa H. 2006a. An empirical site-classification method for strong-motion stations in Japan using H/V response spectral ratio[J]. Bull Seismol Soc Am,96(3):914–925. doi: 10.1785/0120050124

    Zhao J X,Zhang J,Asano A,Ohno Y,Oouchi T,Takahashi T,Ogawa H,Irikura K,Thio H K,Somerville P G,Fukushima Y,Fukushima Y. 2006b. Attenuation relations of strong ground motion in Japan using site classification based on predominant period[J]. Bull Seismol Soc Am,96(3):898–913. doi: 10.1785/0120050122

    Zhao J X,Zhou S L,Gao P J,Long T,Zhang Y B,Thio H K,Lu M,Rhoades D A. 2015. An earthquake classification scheme adapted for Japan determined by the goodness of fit for ground-motion prediction equations[J]. Bull Seismol Soc Am,105(5):2750–2763. doi: 10.1785/0120150013

    Zhao J X,Zhou S L,Zhou J,Zhao C,Zhang H,Zhang Y B,Gao P J,Lan X W,Rhoades D,Fukushima Y,Somerville P G,Irikura K. 2016a. Ground-motion prediction equations for shallow crustal and upper-mantle earthquakes in Japan using site class and simple geometric attenuation functions[J]. Bull Seismol Soc Am,106(4):1552–1569. doi: 10.1785/0120150063

    Zhao J X,Liang X,Jiang F,Xing H,Zhu M,Hou R B,Zhang Y B,Lan X W,Rhoades D A,Irikura K,Fukushima Y,Somerville P G. 2016b. Ground-motion prediction equations for subduction interface earthquakes in Japan using site class and simple geometric attenuation functions[J]. Bull Seismol Soc Am,106(4):1518–1534. doi: 10.1785/0120150034

    Zhao J X,Jiang F,Shi P,Xing H,Huang H F,Hou R B,Zhang Y B,Yu P C,Lan X W,Rhoades D A,Somerville P G,Irikura K,Fukushima Y. 2016c. Ground-motion prediction equations for subduction slab earthquakes in Japan using site class and simple geometric attenuation functions[J]. Bull Seismol Soc Am,106(4):1535–1551. doi: 10.1785/0120150056

    Zhao J X,Jiang F,Ma Y,Zhou J,Cheng Y,Chang Z W. 2017. Ground‐motion prediction equations for the vertical component from shallow crustal and upper‐mantle earthquakes in Japan using site classes as the site term[J]. Bull Seismol Soc Am,107(5):2310–2327. doi: 10.1785/0120160376

    Zhao J X,Yang Q S,Su K W,Liang J G,Zhou J,Zhang H,Yang X G. 2019. Effects of earthquake source,path,and site conditions on damping modification factor for the response spectrum of the horizontal component from subduction earthquakes[J]. Bull Seismol Soc Am,109(6):2594–2613. doi: 10.1785/0120190105

    Zhou J,Zhao J X. 2020. A damping modification factor prediction model for horizontal displacement spectrum from subduction interface earthquakes in Japan accounting for site conditions[J]. Bull Seismol Soc Am,110(3):1231–1246. doi: 10.1785/0120190275
    • 相关文章
    • 施引文献(6)

  • 期刊类型引用(5)

    1. 程喜. 饱和复杂场地效应对大跨连续梁桥地震响应影响的研究. 震灾防御技术. 2024(01): 14-23 . 百度学术
    2. 赵仕兴，罗麒锐，唐元旭，吴启红，熊峰，周巧玲，卢丹，张敏. 复杂地形地质条件下地震动放大系数研究进展. 建筑结构. 2024(19): 51-58+73 . 百度学术
    3. 方志江，龚文剑，张仰鹏，焦晓东，颜志铭. 基于多种物探方法的公路下伏采空区病害识别与分析. 市政技术. 2024(10): 37-44+51 . 百度学术
    4. 刘中宪，周健，李程程，孟思博. 基于修正随机有限断层法的沉积盆地地震动模拟. 防灾减灾工程学报. 2023(05): 999-1008 . 百度学术
    5. 孟思博，赵嘉玮，刘中宪. 基于差分进化-人工神经网络的沉积河谷地震动放大效应预测模型. 地震学报. 2022(01): 170-181 . 本站查看

    其他类型引用(1)

    • 资源附件(0)
图(9)  /  表(5)
计量
  • 文章访问数:  414
  • HTML全文浏览量:  151
  • PDF下载量:  21
  • 被引次数: 6
出版历程
  • 收稿日期:  2020-12-02
  • 修回日期:  2021-05-25
  • 网络出版日期:  2022-04-23
  • 发布日期:  2022-04-23

目录

摘要
HTML全文

  • 引言

  • 1.   数据和方法

  • 1.1   瑞雷波偏振分析

  • 1.2   纵波偏振分析

  • 1.3   接收函数方法

  • 2.   结果与讨论

  • 2.1   OBS水平分量方位及其对接收函数研究的影响

  • 2.2   古迪纳夫盆地和基里比斯盆地的地壳结构

  • 3.   结论

参考文献(25)
相关文章
施引文献(6)
资源附件(0)

/

返回文章
返回

本系统由 北京仁和汇智信息技术有限公司 开发  技术支持：info@rhhz.net 百度统计