Quantitative evaluation of modeling error in Lg-wave attenuation model
-
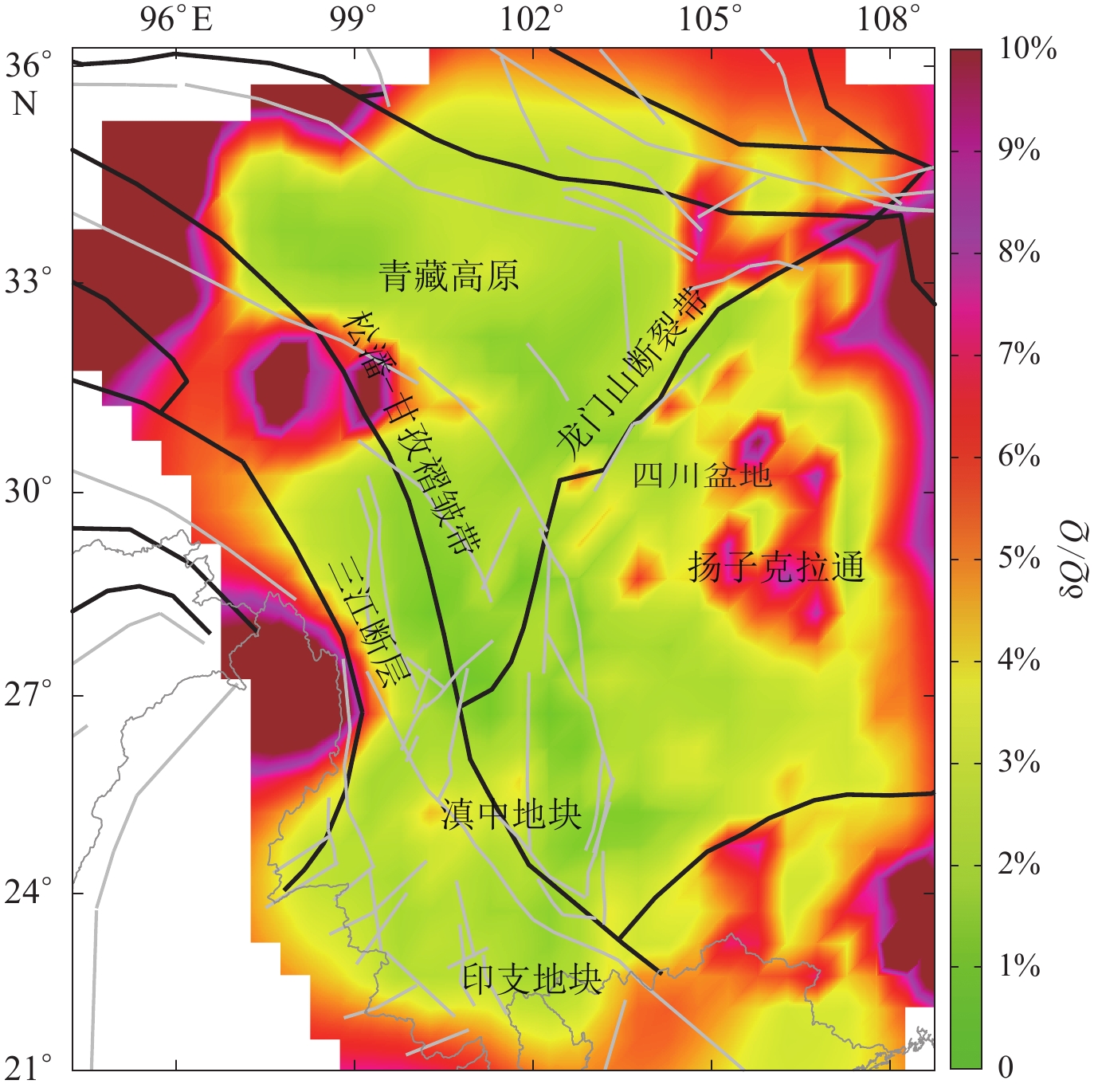
摘要: 对Lg波衰减模型中建模误差的统计特征进行了系统研究，并建立了地壳二维Lg波衰减模型。由于Lg波振幅可能受到几何扩散函数的强烈影响，合理评估反演过程的误差对于能否使用最小二乘意义下的反演非常重要。通过在川滇及其邻近地区收集的建模误差样本，使用K-S数值检验方法、Q-Q图和正态分布图形检验方法对Lg波衰减层析成像反演的输入数据中建模误差的分布特征进行了统计分析。采用奇异值分解（SVD）和反投影方法，分别获得了川滇地区的QLg模型，定量计算模型的协方差矩阵和分辨率矩阵，定量评估了QLg模型中每个格点的分辨率和误差。结果表明：在一阶近似条件下建模误差服从正态分布；通过开发的数据筛选程序，可以产生一个接近完美正态分布的数据集；与反投影方法相比，利用SVD方法获得的地壳Q值的分辨率更高；在射线覆盖较好的区域，QLg模型的分辨率达到100 km，相对误差小于3%。Abstract: In this study, we performed comprehensive statistical studies for the characteristics of the modeling error in Lg-wave attenuation model and obtained a two-dimensional crustal Lg-wave attenuation model. The amplitudes of Lg-wave can be strongly influenced by the geometrical spreading function and it is essential to reasonably evaluate the error of the inversion process if we use the inverted method under the meaning of least squares. Based on the modeling error samples collected in the Sichuan-Yunnan region and its adjacent areas, we use three statistical test methods, namely the K-S test, the Q-Q plot, and the normal distribution plot, to analyze the distribution characteristics of modeling errors in the input data of Lg- wave attenuation tomography inversion. We used both the SVD-based tomographic method and the back-projection method to invert for the Lg Q model of the Sichuan-Yunnan region and its adjacent area respectively. Then, we calculated the covariance matrix and the resolution matrix of the model and evaluated the resolution and error of each grid point in the Lg Q model quantitatively. Our results indicate that the modeling errors in the input data obeyed a normal distribution under first-order approximation. By using the data screening technique, we generated a new dataset with nearly perfect normally distributed for Q tomography. Compared with the result of back-projection, the SVD-based method could allow for obtaining a finer resolution of crustal Q value in the areas with dense ray path coverages, where the model resolution can be resolved within a range of 100 km with a relative error of less than 3%.
-
引言
地电阻率前兆观测从物探电法移植而来，在我国已开展50余年.多年的监测结果表明，大震前在震源区及其附近一般均会出现视电阻率变化 (钱复业等, 1982, 1990；桂燮泰等，1989；Lu et al，1999；叶青等，2005；张学民等，2009；钱家栋等，2013)，地电阻率是一种比较可靠的地震前兆观测方法 (张国民等，2001；杜学彬，2010).为了减小乃至消除来自表层的干扰以获得可能的深部地震或构造运动信息，研究人员已经逐渐认识到开展井下地电阻率前兆监测的必要性，并陆续开展了相关试验与理论研究 (苏鸾声等，1982；刘允秀等，1985；孟庆武，阎洪朋，1991；聂永安，姚兰予，2009；聂永安等，2010；解滔等，2012).近年来我国加快了井下地电阻率监测台站的建设并加强了观测数据的分析工作 (王兰炜等，2015)，杨兴悦等 (2015)和张磊等 (2015)的研究表明，无论是获取强震前的视电阻率变化信息，还是压制表层干扰，井下地电阻率观测均优于传统的地表观测，是一种十分有发展前景的地震前兆观测方式.
目前在我国地电阻率井下观测台站建设中，关于装置电极布设深度对观测结果的影响仍缺乏深入研究，且观测井深和极距的设计缺乏合理性，因此应加快推动相关理论和技术研究，以保障此类观测系统建设的科学性和合理性 (王兰炜等，2015).以观测目的为出发点，装置电极的合理埋深取决于地电阻率的影响系数 (毛先进等，2014；解滔等，2016) 沿深度的分布和理论探测深度，二者均与地电结构密切相关，同时需要避开地表干扰源.物探中研究探测深度的目的是为了将观测值与某个深度相关联，通过选取最佳的电极距使观测数据与地质目标最相关 (霍军廷等，2011).在地震前兆监测方面，赵和云和钱家栋 (1982)研究了对称四极装置布置于地表时的勘探深度和探测范围，认为对于给定的电极距，勘探深度和探测范围与监测区电性结构密切相关；杜学彬等 (2008)分析了强震附近对称四极装置电阻率观测的探测深度，其结果显示对于各向异性介质，在强震孕震晚期和震中附近可检测到较深部地壳介质的电阻率变化.这些工作对台址选择及布极参数的确定均有很好的指导意义，但其仅针对观测装置位于地表的情况.
在井下电阻率观测方面, 毛先进等 (2014)和解滔等 (2016)从影响系数的角度, 研究了其在水平层状介质中的变化特征, 结果显示，不同地层地电阻率影响系数的大小与电阻率结构、装置电极埋深和供电极距等密切相关.本文则在此基础上，进一步研究井下地电阻率观测的探测深度，为确定井下地电阻率观测中最佳装置电极埋深等参数提供理论依据.
1. 探测深度计算方法
常规物探电法对探测深度的定义是，对于均匀半空间，在不同供电极距AB的情况下，AB中垂线上给定深度处的水平方向电流密度是随AB变化的，当水平方向电流密度达到最大时，该深度称为探测深度 (傅良魁，1983)，这一定义给出了探测深度与供电极距AB的关系.由于地震前兆监测中实际台站的台址多为多层介质，电流密度的最大值可能存在多值性.为了克服该缺陷，赵和云和钱家栋 (1982)给出了新的探测深度的定义：设地表至深度z处测量电极M与N之间水平方向的面电流为IMN(z)，全部面电流为IMN(∞)，令二者的比值
(1) 则满足上式的z即为探测深度.比值取
在地电阻率前兆监测分析中，采用式 (1) 作为探测深度的定义更为合理，本文采用该式计算均匀半空间、水平层状介质地表及井下地电阻率观测的探测深度.
1.1 均匀半空间
若均匀半空间的电阻率为ρ，对称四极装置中供电极A，B与测量极M，N的埋深均为h(简称为装置电极埋深)，AB=2L(L为半供电极距)，MN=2a (a为半测量极距)，供电电流强度为I，则深度z处分别与M，N水平坐标相同的两点间的电位差为
(2) 式中，Q=ρI/(2π)，深度z处分别与M，N水平坐标相同的两点间的面电流密度为
(3) 地表至深度z处的面电流为
(4) 由式 (2)，(3)，(4) 计算得到
(5) 
(6) 式 (5) 与式 (6) 相除得
(7) 根据式 (1)，求出使式 (7) 等于
1.2 水平层状结构
对于水平层状结构，当供电电极及测量电极位于地表或地下时，可用边界积分方程法或有限元法求得点电流源激励下的地下任意两点间的电位差.本文选用边界积分方程法，该方法能对任意层数的一维及二维介质进行模拟，且其在理论和数值模拟两方面的准确性已被证明 (毛先进，鲍光淑，1998).求得地下各层分界面上分别与M，N水平坐标相同的两个网格节点间的电位差后，根据式 (1)，(3)，(4) 计算得到探测深度.
2. 计算结果
2.1 均匀半空间
我国地电阻率观测中半供电极距L为500 m左右，表 1给出了目前常用装置 (装置参数分别用C1，C2，C3，C4，C5表示，下同) 在不同埋深时探测深度z的计算结果.探测深度随埋深的变化见图 1.
表 1 几种常用观测装置在井下的探测深度 (均匀半空间)Table 1. The borehole probing depths of several common observation configurations (homogeneous half-space)装置电极埋深
h/mC1
(L=150 m，
a=25 m)C2
(L=300 m，
a=75 m)C3
(L=400 m，
a=75 m)C4
(L=500 m，
a=125 m)C5
(L=600 m，
a=125 m)0 148.2 292.1 394.1 486.8 589.0 10 148.8 292.3 394.3 486.9 589.1 20 150.2 293.1 394.8 487.4 589.5 50 160.3 298.4 398.8 490.6 592.2 100 190.9 316.3 412.5 501.8 601.5 200 276.5 378.1 462.5 543.9 637.3 300 371.9 459.4 533.4 606.1 691.9 500 569.1 644.2 705.6 765.7 838.2 注：L=AB/2，a=MN/2，下同. ![]()
图 1 几种常用观测装置的探测深度z随电极埋深h的变化 (均匀半空间)Figure 1. The variation curves of probing depths z along with electrode buried depths h of several common observation configurations (homogeneous half-space)由表 1和图 1可知，与观测装置位于地表时 (h=0) 的探测深度z相比，h分别为10，20，50 m时探测深度的增加值依次为0.1—0.6 m，0.5—2.0 m，3.2—12.1 m；h < 100 m时探测深度随埋深的增加速度缓慢，且L越大这一现象越明显；h>100 m时探测深度随埋深的增加速度明显增大.
2.2 水平层状结构
水平层状结构的探测深度与地电阻率的结构有关 (赵和云，钱家栋，1982；霍军廷等，2011)，实际地电阻率的结构因地而异.水平层状电阻率均匀分层结构可定性地分为下伏高阻与下伏低阻两种情形，本文则针对这两种情形分别进行研究.
2.2.1 下伏低阻结构计算结果
给定一个4层电阻率水平层状均匀分层结构，从上至下电阻率依次为80，40，90, 20 Ω·m，第1—3层厚度均为50 m，第4层厚度趋于∞.计算中设定对称四极装置中供电极A，B与测量极M，N在地表或地下同一深度，不同装置 (C1，C2，C3，C4，C5) 在不同埋深时探测深度z的计算结果见表 2，探测深度随埋深的变化情况如图 2a所示.
表 2 几种常见观测装置在井下的探测深度 (下伏低阻)Table 2. The borehole probing depths of several common observation configurations (underlying low resistivity structure)装置电极埋深
h/mC1
(L=150 m，
a=25 m)C2
(L=300 m，
a=75 m)C3
(L=400 m，
a=75 m)C4
(L=500 m，
a=125 m)C5
(L=600 m，
a=125 m)0 184.4 344.3 458.2 551.3 645.0 10 184.9 344.6 458.5 551.5 645.2 20 186.2 345.3 459.0 551.8 645.6 50 194.9 350.0 462.3 554.7 647.8 100 221.5 365.3 473.6 563.9 655.7 200 297.7 419.4 516.3 600.0 686.4 300 377.9 477.6 563.2 639.6 720.6 500 570.2 646.9 712.9 773.8 841.1 ![]()
图 2 几种常用观测装置的探测深度z随电极埋深h的变化(a) 下伏低阻; (b) 下伏高阻Figure 2. The variation curves of probing depths z along with electrode buried depths h of several common observation configurations(a) Underlying low resistivity structure; (b) Underlying high resistivity structure由表 2和图 2a可知，与观测装置位于地表时 (h=0) 的探测深度z相比，h分别为10，20，50 m时探测深度的增加值依次为0.2—0.5 m，0.6—1.8 m，2.8—10.5 m；h < 100 m时探测深度随埋深的增加速度缓慢，且L越大这一现象越明显；h>100 m时探测深度随埋深的增加速度明显增大.
2.2.2 下伏高阻结构计算结果
将上述下伏低阻结构中底层 (即第四层) 的电阻率改为200 Ω·m，其它各层电阻率及层厚保持不变，依然设定对称四极装置中供电极A，B与测量极M，N在地表或地下同一深度处，不同装置 (C1，C2，C3，C4，C5) 在不同埋深时探测深度z的计算结果见表 3，探测深度随埋深的变化见图 2b.
表 3 几种常见观测装置在井下的探测深度 (下伏高阻)Table 3. The borehole probing depths of several common observation configurations (underlying high resistivity structure)装置电极埋深
h/mC1
(L=150 m，
a=25 m)C2
(L=300 m，
a=75 m)C3
(L=400 m，
a=75 m)C4
(L=500 m，
a=125 m)C5
(L=600 m，
a=125 m)0 104.5 162.6 238.8 305.6 388.7 10 104.9 162.7 239.0 305.8 388.8 20 105.8 163.3 239.5 306.2 389.2 50 111.0 167.3 242.4 308.6 391.2 100 124.3 180.3 252.4 317.1 398.1 200 251.1 300.0 351.0 402.4 469.7 300 365.5 424.3 472.5 516.3 572.1 500 568.0 632.2 681.2 721.9 769.7 由表 3和图 2b可知，与观测装置位于地表时 (h=0) 的探测深度z相比，h分别为10，20，50 m时探测深度的增加值依次为0.1—0.4 m，0.5—1.3 m，2.5—6.5 m；h < 100 m时探测深度随埋深的增加速度缓慢，且L越大这一现象越明显；h>100 m时探测深度随埋深的增加速度明显增大.
3. 讨论与结论
本文以电流的主要分布范围为指标，讨论了均匀半空间和电阻率均匀分层模型中下伏低阻及下伏高阻两种典型结构，并通过模拟计算的方法对不同结构下地电阻率在井下前兆观测中的探测深度进行了初步研究.结果显示，在供电极距为数百米至上千米时，与地表观测相比，井下观测的探测深度的增加值具有如下特点：
1) 对于目前我国常用的观测装置和典型的地电阻率结构来说，当装置电极埋深h≤100 m时，与观测装置位于地表时相比，探测深度随埋深增加的速率比埋深h>100 m时小得多.作者此前对其影响系数的研究 (毛先进等，2014) 也得到相似的结论：对于均匀分层结构，在装置电极埋深h≤100 m时基底层 (最下层) 的影响系数随埋深的增加速率比埋深h>100 m时小得多，同时表层介质的影响系数还呈现“减小—增加—减小”起伏变化的现象.
2) 当供电极距在数百米至上千米、装置电极埋深在50 m以内时，与地表观测相比，其探测深度的增加很小，最大只有十余米，且当装置电极埋深相同时，供电极距越大，探测深度的增加值越小.这表明，虽然这样的装置电极埋深对减小来自地表的干扰是有利的，但从获得深部电阻率变化信息的角度来看，并不能达到井下观测的目的.
3) 当装置和电极埋深均相同时，下伏高阻结构的探测深度最小，下伏低阻结构的探测深度最大，均匀半空间的探测深度介于二者之间.比较表 2与表 3可知，按照本文选取的典型地电结构参数，在装置相同的情况下，下伏低阻结构中装置电极埋深为100 m时的探测深度与下伏高阻结构中装置电极埋深为200—300 m时的探测深度相当.实际上，下伏高阻结构是一种比较常见的情形，在这种情况下要增加探测深度就需要加大装置电极埋深.
根据上述特点，本文认为，为获得深部电阻率的变化信息，在井下观测中观测装置应达到一定的埋深，才能获得与地表观测相比更有意义的探测深度.为此，首先需要查明测区电性结构，然后通过计算分析，确定井下地电阻率观测的装置电极埋深.对于我国目前常用的观测装置 (供电极距为数百米至上千米)，在均匀分层电性结构下：当观测区为下伏低阻结构时，装置电极埋深应不小于100 m；当观测区为下伏高阻结构时，装置电极埋深应不小于200 m.
需要指出的是，本文仅计算了给定分层参数时两种典型电性结构下 (高阻、低阻) 的装置电极埋深结果，并不能代表所有的高阻或低阻分层结构的情况.对于不同台址，由于电性分层结构存在差异，因此合理的装置电极埋深亦有所差异，应通过具体的计算分析而确定.
-
![]()
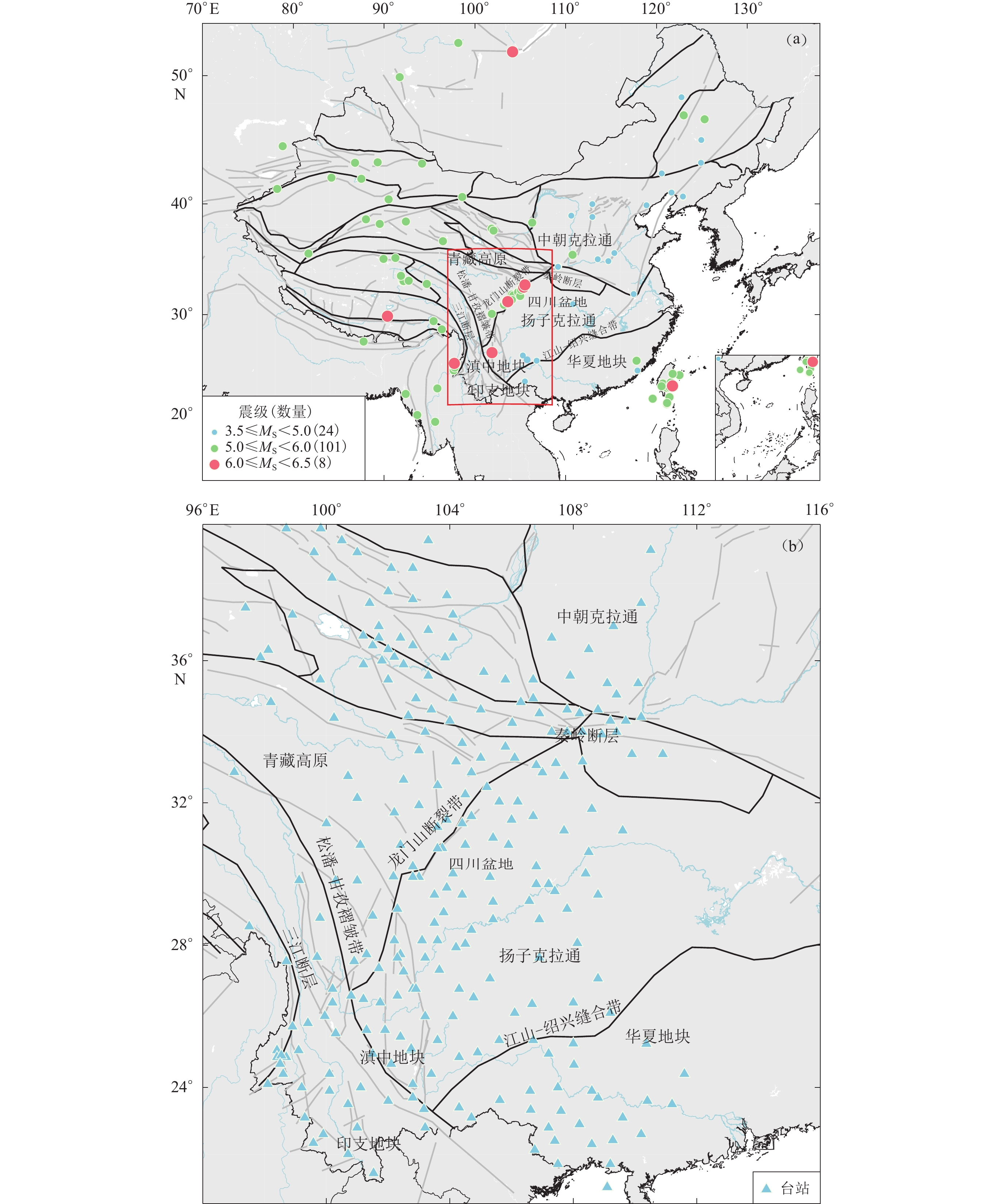
图 1 研究所用地震事件震中（a）和研究区台站（b）分布图
图中红色矩形框代表研究区域和台站所在区域；黑线为主要地块分界线，灰线为主要断裂带，下同
Figure 1. Distribution of epicenters of events （a） and seismic stations （b） in this study
Red rectangular represents the extent of the study area；the black solid lines indicate the major tectonic block boundaries and the grey dashed lines indicate faults，the same below
![]()
图 2 基于双台法的各个路径上Q值重复测量次数分布
（a） TS方法；（b） RTS方法；（C） RTE方法
Figure 2. Distribution of the number of repeated two-stations based method over individual paths
（a） TS method；（b） RTS method；（c） RTE method
![]()
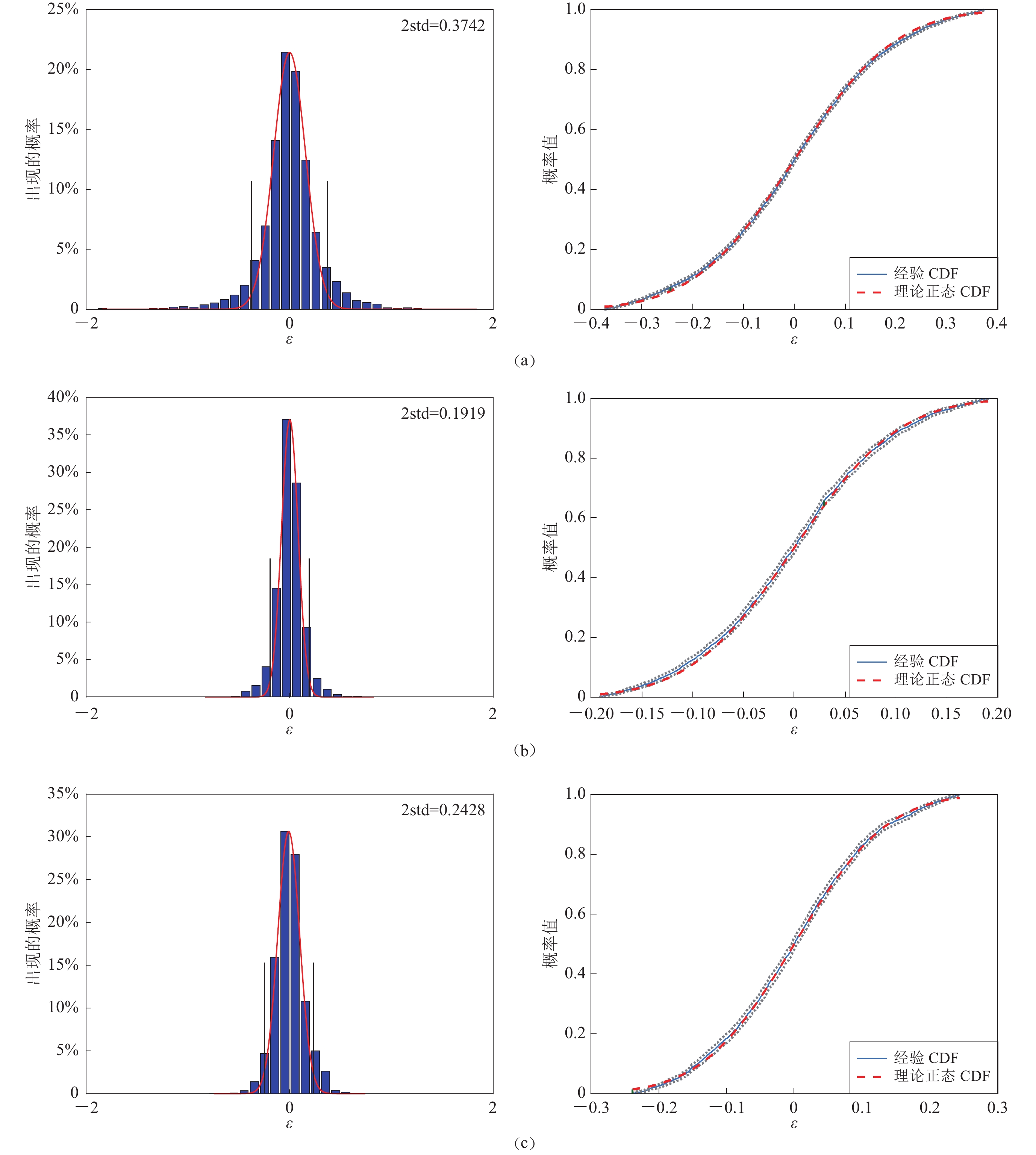
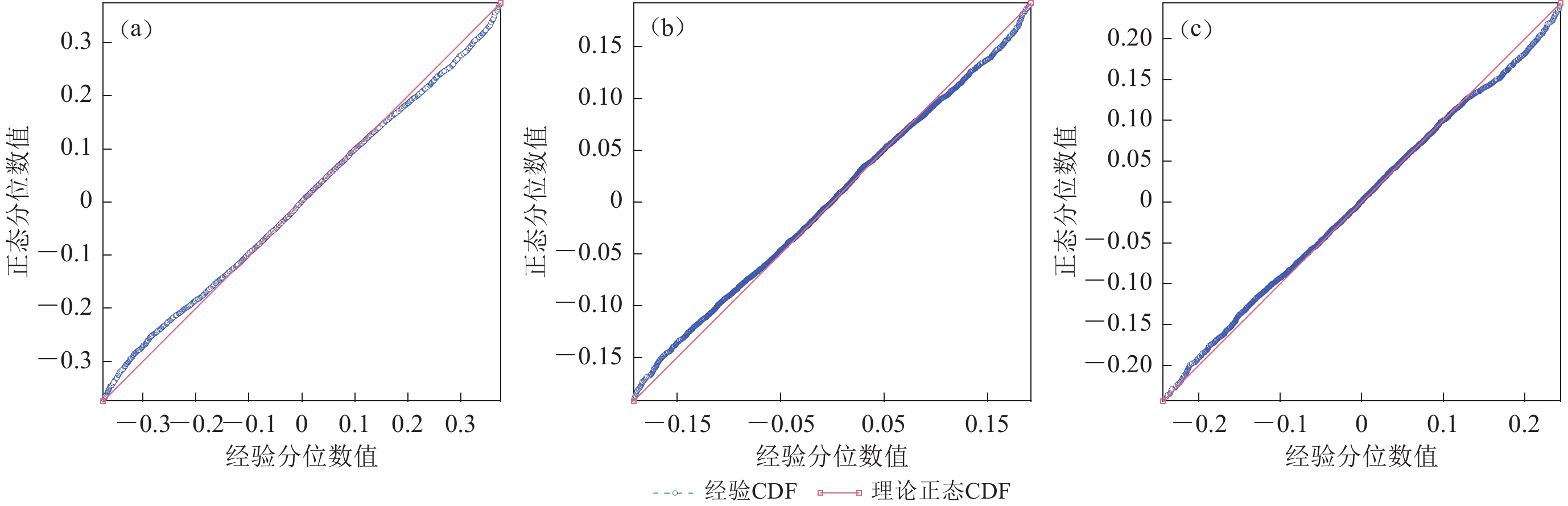
图 3 建模误差
$\varepsilon$ 样本分布（左）和K-S检测图（右）（a） TS方法；（b） RTS方法；（c）RTE方法。左图中两条黑色直线标记了数据筛选准则|ε-median（ε）|＞2std（ε）的区间，红色线表示最佳的正态概率密度函数值；右图中灰色线代表着95%置信区间的CDF的上下界限，绿色小线段代表着K-S检验的经验CDF和理论CDF之间的最大垂向差值
Figure 3. Distribution of samples of modeling error
$\varepsilon$ （left）and results of K-S test （right）（a） TS method；（b） RTS method ；（c） RTS method. The black lines in the left figure mark the interval of |ε-median（ε）|＞2std（ε），which we use as criteria for data screening and the best-fit theoretical normal PDF of the screened samples is plotted over the distribution map with the red line. The grey lines in the right figure indicate lower and upper 95 percent confidence bounds for the CDF and the green segment indicates the maximum distance from the empirical CDF to the theoretical normal CDF which is used in the K-S test
![]()
图 4 样本ε的Q-Q图
（a） TS方法；（b） RTS方法；（c） RTE方法
Figure 4. Q-Q plots of samples of
$\varepsilon$ （a） TS method；（b） RTS method；（c） RTE method
![]()
图 5 （a） 1°×1°棋盘格结果；（b）研究区棋盘格恢复
Figure 5. （a） The checkboard with 1°×1° grid；（b） The recovery of the original checkboard
![]()
图 6
$ {Q}_{{\rm{Lg}}} $ 模型的Backus-Gilbert扩散函数（a） 基于双台方法的射线路径覆盖图；（b） 用Backus-Gilbert扩散函数来表示研究区域的模型分辨率
Figure 6. Backus-Gilbert spread functions of the tomographic Lg Q model
（a） The path coverage of two-station based method over the studied region；（b） Spread function of the tomographic Q model
![]()
图 7 川滇地区及其邻近地区的Q值模型
（a） 新的层析成像方法结果；（b） 反投影方法结果
Figure 7. The Lg Q model of Sichuan-Yunnan area and its neighborhood
（a） Result from the new tomographic method；（b） Result from the back projection method
表 1 使用TS，RTS和RTE方法估计ε的详细信息
Table 1 Estimated ε information using TS， RTS and RTE methods
方法 $ {\varepsilon } $样本的
数量误差在两个标准差
内的$ \varepsilon $占比ECDF和NCDF的
相关系数K-S测试结果 标准差
std$ （ \varepsilon ） $方差Var$ （ \varepsilon ） $ P值 显著性水平$ \alpha $ TS 8 220 87.09% 99.99% 在置信度为95%下接受零假设 0.158 5 0.025 1 0.693 1 0.05 RTS 3 652 88.12% 99.98% 在置信度为95%下接受零假设 0.081 6 0.006 7 0.324 0 0.05 RTE 2 839 93.59% 99.64% 在置信度为95%下接受零假设 0.104 9 0.011 0 0.280 1 0.05 
下载: 导出CSV
-
邓起东,张培震,冉勇康,杨晓平,闵伟,楚全芝. 2002. 中国活动构造基本特征[J]. 中国科学:D辑,32(12):1020–1030. Deng Q D,Zhang P Z,Ran Y K,Yang X P,Min W,Chu Q Z. 2003. Basic characteristics of active tectonics of China[J]. Science in China:Series D,46(4):356–372.
盛骤, 谢式千, 潘承毅. 2008. 概率论与数理统计[M]. 北京: 高等教育出版社: 119–126. Sheng Z, Xie S Q, Pan C Y. 2008. Probability Theory and Mathematical Statistics[M]. Beijing: Higher Education Press: 119–126 （in Chinese）.
郑秀芬,欧阳飚,张东宁,姚志祥,梁建宏,郑洁. 2009. “国家数字测震台网数据备份中心”技术系统建设及其对汶川大地震研究的数据支撑[J]. 地球物理学报,52(5):1412–1417. Zheng X F,Ouyang B,Zhang D N,Yao Z X,Liang J H,Zheng J. 2009. Technical system construction of Data Backup Centre for China Seismograph Network and the data support to researches on the Wenchuan earthquake[J]. Chinese Journal of Geophysics,52(5):1412–1417 (in Chinese).
周连庆,赵翠萍,修济刚,陈章立. 2008. 川滇地区Lg波Q值层析成像[J]. 地球物理学报,51(6):1745–1752. doi: 10.3321/j.issn:0001-5733.2008.06.015 Zhou L Q,Zhao C P,Xiu J G,Chen Z L. 2008. Tomography of QLg in Sichuan-Yunnan zone[J]. Chinese Journal of Geophysics,51(6):1745–1752 (in Chinese).
Akinci A,Del Pezzo E,Ibáñez J M. 1995. Separation of scattering and intrinsic attenuation in southern Spain and western Anatolia (Turkey)[J]. Geophys J Int,121(2):337–353. doi: 10.1111/j.1365-246X.1995.tb05715.x
Backus G,Gilbert F. 1970. Uniqueness in the inversion of inaccurate gross Earth data[J]. Philosophical Transactions of the Royal Society of London. Series A:Mathematical and Physical Sciences,266(1173):123–192.
Bostock M G,Kennett B L N. 1990. The effect of 3-D structure on Lg propagation patterns[J]. Geophys J Int,101(2):355–364. doi: 10.1111/j.1365-246X.1990.tb06574.x
Chen Y L,Xie J K. 2017. Resolution,uncertainty and data predictability of tomographic Lg attenuation models:Application to southeastern China[J]. Geophys J Int,210(1):166–183. doi: 10.1093/gji/ggx147
Chun K Y,West G F,Kokoski R J,Samson C. 1987. A novel technique for measuring Lg attenuation-results from eastern Canada between 1 to 10 Hz[J]. Bull Seismol Soc Am,77(2):398–419. doi: 10.1785/BSSA0770020398
Dai A,Tang C C,Liu L,Xu R. 2020. Seismic attenuation tomography in southwestern China:Insight into the evolution of crustal flow in the Tibetan Plateau[J]. Tectonophysics,792:228589. doi: 10.1016/j.tecto.2020.228589
Gallegos A,Ranasinghe N,Ni J,Sandvol E. 2014. Lg attenuation in the central and eastern United States as revealed by the Earth Scope Transportable Array[J]. Earth Planet Sci Lett,402:187–196. doi: 10.1016/j.jpgl.2014.01.049
Gibbons S J,Kværna T,Ringdal F. 2010. Considerations in phase estimation and event location using small-aperture regional seismic arrays[J]. Pure Appl Geophys,167(4/5):381–399.
Gök R,Sandvol E,Türkelli N,Seber D,Barazangi M. 2003. Sn attenuation in the Anatolian and Iranian plateau and surrounding regions[J]. Geophys Res Lett,30(24):8042.
Gung Y,Romanowicz B. 2004. Q tomography of the upper mantle using three-component long-period waveforms[J]. Geophys J Int,157(2):813–830. doi: 10.1111/j.1365-246X.2004.02265.x
Hearn T M,Wang S Y,Pei S P,Xu Z H,Ni J F,Yu Y X. 2008. Seismic amplitude tomography for crustal attenuation beneath China[J]. Geophys J Int,174(1):223–234. doi: 10.1111/j.1365-246X.2008.03776.x
Ji C,Tsuboi S,Komatitsch D,Tromp J. 2005. Rayleigh-wave multipathing along the West coast of North America[J]. Bull Seismol Soc Am,95(6):2115–2124. doi: 10.1785/0120040180
Kennett B L N. 1986. Lg waves and structural boundaries[J]. Bull Seismol Soc Am,76(4):1133–1141.
Larsen R M, 1998. Lanczos Bidiagonalization With Partial Reorthogonalization[D]. Jutland: Aarhus University: 8–87.
Liang X F,Sandvol E,Kay S,Heit B,Yuan X H,Mulcahy P,Chen C,Brown L,Comte D,Alvarado P. 2014. Delamination of southern Puna lithosphere revealed by body wave attenuation tomography[J]. J Geophys Res:Solid Earth,119(1):549–566. doi: 10.1002/2013JB010309
Marden J I. 2004. Positions and QQ plots[J]. Statist Sci,19(4):606–614.
Massey F J. 1951. The Kolmogorov-Smirnov test for goodness of fit[J]. J Am Statist Associat,46(253):68–78. doi: 10.1080/01621459.1951.10500769
McNamara D E,Owens T J,Walter W R. 1996. Propagation characteristics of L
g across the Tibetan Plateau[J]. Bull Seismol Soc Am,86(2):457–469. doi: 10.1785/BSSA0860020457 Menke W,Holmes R C,Xie J K. 2006. On the nonuniqueness of the coupled origin time-velocity tomography problem[J]. Bull Seismol Soc Am,96(3):1131–1139. doi: 10.1785/0120050192
Mitchell B J. 1995. Anelastic structure and evolution of the continental crust and upper mantle from seismic surface wave attenuation[J]. Rev Geophys,33(4):441–462. doi: 10.1029/95RG02074
Mitchell B J,Hwang H J. 1987. Effect of low Q sediments and crustal Q on L
g attenuation in the United States[J]. Bull Seismol Soc Am,77(4):1197–1210. doi: 10.1785/BSSA0770041197 Mitchell B J,Pan Y,Xie J K,Cong L L. 1997. L
g coda Q variation across Eurasia and its relation to crustal evolution[J]. J Geophys Res:Solid Earth,102(B10):22767–22779. doi: 10.1029/97JB01894 Nolet G. 1987. Seismic Tomography: With Applications in Global Seismology and Exploration Geophysics[M]. Dordrecht: Springer: 1–24.
Nuttli O W. 1980. The excitation and attenuation of seismic crustal phases in Iran[J]. Bull Seismol Soc Am,70(2):469–485. doi: 10.1785/BSSA0700020469
Pasyanos M E,Matzel E M,Walter W R,Rodgers A J. 2009. Broad-band L
g attenuation modelling in the Middle East[J]. Geophys J Int,177(3):1166–1176. doi: 10.1111/j.1365-246X.2009.04128.x Pei S P,Zhao J M,Rowe C A,Wang S Y,Hearn T M,Xu Z H,Liu H B,Sun Y S. 2006. ML amplitude tomography in North China[J]. Bull Seismol Soc Am,96(4A):1560–1566. doi: 10.1785/0120060021
Pukelsheim F. 1994. The three sigma rule[J]. Am Statist,48(2):88–91.
Rietbrock A. 2001. P wave attenuation structure in the fault area of the 1995 Kobe earthquake[J]. J Geophys Res:Solid Earth,106(B3):4141–4154. doi: 10.1029/2000JB900234
Rodgers A J,Ni J F,Hearn T M. 1997. Propagation characteristics of short-period Sn and Lg in the Middle East[J]. Bull Seismol Soc Am,87(2):396–413.
Romanowicz B. 1998. Attenuation tomography of the earth’s mantle:A review of current status[J]. Pure Appl Geophys,153(2):257–272.
Schurr B,Asch G,Rietbrock A,Trumbull R,Haberland C. 2003. Complex patterns of fluid and melt transport in the central Andean subduction zone revealed by attenuation tomography[J]. Earth Planet Sci Lett,215(1/2):105–119.
Sereno T J Jr,Bratt S R,Bache T C. 1988. Simultaneous inversion of regional wave spectra for attenuation and seismic moment in Scandinavia[J]. J Geophys Res:Solid Earth,93(B3):2019–2035. doi: 10.1029/JB093iB03p02019
Street R L,Herrmann R B,Nuttli O W. 1975. Spectral characteristics of the L
g wave generated by central United States earthquakes[J]. Geophys J Int,41(1):51–63. doi: 10.1111/j.1365-246X.1975.tb05484.x Tian H Y,He C S,Santosh M. 2021. S-wave velocity structure of the Sichuan-Yunnan region,China:Implications for extrusion of Tibet Plateau and seismic activities[J]. Earth Space Sci,8(7):e2021EA001640.
Vrbik J. 2020. Deriving CDF of Kolmogorov-Smirnov test statistic[J]. Appl Math,11(3):227–246. doi: 10.4236/am.2020.113018
Wei Z,Zhao L. 2019. Lg Q model and its implication on high-frequency ground motion for earthquakes in the Sichuan and Yunnan region[J]. Earth Planet Phys,3(6):526–536.
Wheelan C. 2013. Naked Statistics[M]. London: W W Norton & Company: 19–292.
Xie J K. 1993. Simultaneous inversion for source spectrum and path Q using L
g with application to three semipalatinsk explosions[J]. Bull Seismol Soc Am,83(5):1547–1562. doi: 10.1785/BSSA0830051547 Xie J K. 1998. Spectral inversion of Lg from earthquakes:A modified method with applications to the 1995,western Texas earthquake sequence[J]. Bull Seismol Soc Am,88(6):1525–1537.
Xie J. 2002. Lg Q in the eastern Tibetan Plateau[J]. Bull Seismol Soc Am,92(2):871–876. doi: 10.1785/0120010154
Xie J,Mitchell B J. 1990. A back-projection method for imaging large-scale lateral variations of L
g coda Q with application to continental Africa[J]. Geophys J Int,100(2):161–181. doi: 10.1111/j.1365-246X.1990.tb02477.x Xie J,Gok R,Ni J,Aoki Y. 2004. Lateral variations of crustal seismic attenuation along the INDEPTH profiles in Tibet from LgQ inversion[J]. J Geophys Res:Solid Earth,109(B10):B10308.
Xie J,Wu Z,Liu R,Schaff D,Liu Y,Liang J. 2006. Tomographic regionalization of crustal Lg Q in eastern Eurasia[J]. Geophys Res Lett,33(3):L03315.
Yang X. 2002. A numerical investigation of Lg geometrical spreading[J]. Bull Seismol Soc Am,92(8):3067–3079. doi: 10.1785/0120020046
Zhao L F,Xie X B,Wang W M,Zhang J H,Yao Z X. 2010. Seismic Lg-wave Q tomography in and around northeast China[J]. J Geophys Res:Solid Earth,115(B8):B08307.
Zhao L F,Xie X B,He J K,Tian X B,Yao Z X. 2013. Crustal flow pattern beneath the Tibetan Plateau constrained by regional Lg-wave Q tomography[J]. Earth Planet Sci Lett,383:113–122. doi: 10.1016/j.jpgl.2013.09.038
Zhao L F,Xie X B. 2016. Strong Lg-wave attenuation in the Middle East continental collision orogenic belt[J]. Tectonophysics,674:135–146. doi: 10.1016/j.tecto.2016.02.025
Zor E,Sandvol E,Xie J,Türkelli N,Mitchell B,Gasanov A H,Yetirmishli G. 2007. Crustal attenuation within the Turkish Plateau and surrounding regions[J]. Bull Seismol Soc Am,97(1B):151–161. doi: 10.1785/0120050227
计量
- 文章访问数: 476
- HTML全文浏览量: 239
- PDF下载量: 62
