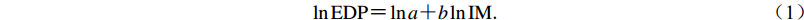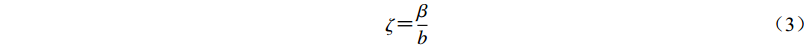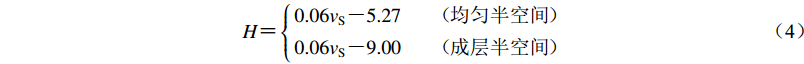Variation law of optimal seismic peak intensity measures for underground structures with burial depth
-
摘要: 选取了50条实际地震动，采用一维场地等效线性化方法分别对均匀半空间场地和成层半空间场地进行地震响应分析，同时选择效益性作为判别标准来探究最优地震动峰值指标（峰值加速度PGA，峰值速度PGV，峰值位移PGD）随埋深变化的规律。研究结果表明：对于选取的两类场地，最优地震动峰值指标均随埋深的改变而变化，埋深浅时PGA效益性最优，随着埋深的增加，效益性最优逐渐由PGA转变至PGV；PGA与PGV转折的交点位置随场地剪切波速的不同而不同，且该转折交点位置与场地剪切波速呈线性相关。Abstract: Determination of a reasonable seismic intensity measure is very important for structural seismic performance evaluation. The seismic response of the underground structure is closely related to the deformation of the engineering site under earthquake excitation due to the constraint of the surrounding rock and soil. Besides, the burial depth of the underground structure also has critical effects on its seismic response. Therefore it is of great significance to investigate the variation of the optimal seismic intensity measure with burial depths of underground structures in engineering sites. In this paper, one-dimensional equivalent-linear earthquake site response analyses was performed by using 50 actual seismic records as the input motions to estimate the seismic response of homogeneous half-space sites and layered half-space sites. For the convenience of comparison among different numerical results, the engineering bedrock is assumed to be a linear elastic medium herein and the earthquake ground motions are input in the engineering bedrock at the same burial depth of 200 m from the ground surface. Based on the proficiency of the results, the optimal peak seismic intensity measures （peak ground acceleration PGA, peak ground velocity PGV, peak ground displacement PGD） varying with the burial depth of the site were investigated herein. The numerical results show that for the selected two types of sites, the optimal peak seismic intensity measure changes with the burial depth of the site. When the burial depth is small, the proficiency of PGA is the best. With the increase of the burial depth, the optimal proficiency changes from PGA to PGV. Moreover, although the critical burial depth corresponding to the transition from PGA to PGV are different for different sites, it exhibits a linear correlation with shear wave velocity of the engineering sites.
-
引言
目前关于地下结构的研究发展迅速，众多研究人员认为地下结构的抗震性能优于地上结构，因而大量建成的地下结构均未考虑抗震设计（Hashash et al，2001；于翔，2002）。但近年来大量震后调查（Wang et al，2001；Scawthorn et al，2006；崔光耀等，2017）表明以地铁、隧道为代表的地下结构也遭遇了严重的震害。通常对于地下结构所遭受的损害，其修复费用和时间远超地上结构。因此对于地下结构抗震性能的研究尤为重要。确定一个合理的地震动强度指标（intensity measure，缩写为IM）是基于性能的抗震设计方法的重要环节之一，同时合理的IM可以有效地降低结构响应预测的离散性，因此确定合理的IM具有重要的意义。
目前已有不少针对IM与地上结构响应之间关系的研究，并取得了诸多成果，相同IM对预测不同结构形式响应时的效用不同，不同IM对相似结构的效用也不同。Riddell （2007）和Yang等（2009）选择单自由度体系展开研究，其结果表明加速度型指标适用于刚性系统，速度型指标适用于中频系统，位移型指标适用于柔性系统。于晓辉（2012）选取了60个地震动强度参数和6个结构反应参数，经过综合性评价分析得出与结构性质有关的地震动强度参数有更好的评价效果。陈健云等（2017）利用相关系数对不同周期框架结构进行三维分析，给出了13种常用的地震动强度指标与不同周期结构响应之间的相关性，其结果表明加速度型、速度型及位移型强度指标与不同周期结构响应参数的相关性不同。左占宣等（2019）采用变异系数对比了新强度指标等效周期谱加速度Sa（Teq）与已有的强度指标结构弹性基本周期对应的谱加速度Sa（T1），结果表明运用Sa（Teq）可以有效地降低倒塌分析结果的离散性。Yang等（2019）对两种不同形式的隔震结构进行了有效性、充分性以及灵敏度的分析，进而得出修正速度谱强度是预测大部分工程需求参数（engineering demand parameter，缩写为EDP）的有效指标。另有众多研究人员也针对诸如隔震结构（耿方方等，2013）、桥梁（李雪红等，2014）、超高建筑（卢啸等，2014）、网壳结构（于天昊，2016）等不同结构与多种IM之间的关系展开了研究。
由于受到围岩土体的约束，地下结构的地震响应不同于地上结构。适用于地下结构的地震动强度指标IM的研究还相对有限。Chen和Wei （2013）分析了埋深44 m的山岭隧道衬砌整体损伤指数与地震动强度指标之间的关系，结果表明山岭隧道衬砌整体损伤指数与速度相关型地震动强度指标的相关性较高。钟紫蓝等（2020）以日本神户埋深4.8 m的大开地铁车站为研究对象，分析了22个地震动强度指标的有效性、效益性和实用性，其结果表明对于文中采用的结构形式，以峰值加速度（peak ground acceleration，缩写为PGA）和复合加速度Ia为代表的加速度型指标和以加速度谱强度为代表的谱相关型地震动强度指标有更强的适用性。
目前针对地下结构的研究都是固定埋深的，但地下结构埋深的变化对地下结构的内力、变形等地震响应具有显著的影响（李长青等，2011；Pitilakis et al，2014），而且埋深是地下结构抗震设计不可忽视的重要因素之一。随着城市用地紧张，对地下空间的开发日趋加深，日本就设想将城市地下规划到50—80 m （董正方等，2017），因此研究最优地震动峰值指标随地下结构埋深变化的规律具有重要的意义。由于地下结构受周围土体的约束，其地震响应与周围场地变形密切相关，因此本文从简单一维场地地震响应着手，拟采用从太平洋地震工程研究中心（Pacific Earthquake Engineering Research Center，缩写为PEER）获取的实际地震动作为输入，以不同波速的均匀半空间场地以及成层半空间场地为对象，基于效益性准则探究最优地震动峰值指标随埋深变化的规律，以期确定不同埋深下的最优地震动强度指标，为结构抗震性能评价提供合理的地震动指标参考。
1. 模型与计算方法
本文涉及的均匀半空间场地以实际场地为例，剪切波速从100 m/s到500 m/s，每间隔50 m/s设计一个场地，加上波速为85 m/s的场地共计10个均匀半空间场地，囊括了 《GB 50011—2010建筑地震设计规范》（中华人民共和国住房和城乡建设部，中华人民共和国国家质量监督检验检疫总局，2010）中Ⅰ —Ⅳ类场地条件，均匀半空间场地均为线弹性无阻尼介质，相关信息详见表1。
表 1 均匀半空间场地信息Table 1. Information of homogeneous half-space sites场地序号 密度/（kg·m−3） 剪切波速/（m·s−1） 场地类别 1 1 800 85 Ⅳ 2 1 820 100 Ⅳ 3 1 850 150 Ⅲ 4 1 920 200 Ⅲ 5 1 920 250 Ⅲ 6 1 970 300 Ⅱ 7 1 970 350 Ⅱ 8 2 100 400 Ⅱ 9 2 100 450 Ⅱ 10 2 300 500 Ⅰ 成层半空间场地由一层土层和半空间基岩层组成，土层参数参考某地铁工程场地的地震安全性报告①选取。为方便对比，设计土层厚度为40 m，所有场地基岩取相同深度，其详细信息见表2，土体剪切模量比和阻尼比随剪应变的变化曲线如图1所 示。基岩为线弹性无阻尼介质。
表 2 成层半空间场地信息Table 2. Information of layered half-space sites场地序号 分层 土类号 厚度/m 泊松比 密度/（kg·m−3） 剪切波速/（m·s−1） 场地类别 11 土层 1 40 0.42 1 820 113 Ⅲ 基岩 6 ∞ 0.20 2 300 500 12 土层 2 40 0.38 1 850 166 Ⅱ 基岩 6 ∞ 0.20 2 300 500 13 土层 3 40 0.35 1 920 210 Ⅱ 基岩 6 ∞ 0.20 2 300 500 14 土层 4 40 0.26 1 920 254 Ⅱ 基岩 6 ∞ 0.20 2 300 500 15 土层 5 40 0.30 1 970 312 Ⅱ 基岩 6 ∞ 0.20 2 300 500 16 土层 5 40 0.30 1 970 360 Ⅱ 基岩 6 ∞ 0.20 2 300 500 17 土层 5 40 0.27 2 100 425 Ⅱ 基岩 6 ∞ 0.20 2 300 500 18 土层 5 40 0.27 2 100 493 Ⅱ 基岩 6 ∞ 0.20 2 300 500 ![]()
图 1 剪切模量比和阻尼比随剪应变的变化Figure 1. Shear modulus ratio and damping ratio varying with the shear strain采用等效线性化方法考虑土的非线性特性，从目前较常用的等效线性化分析软件中选择EERA软件进行分析，分析时场地底部采用开放边界，统一在200 m基岩处输入地震动。
2. 地震动及指标的选取
2.1 地震动记录的选取
Dávalos和Miranda （2019）指出仅采用简单的地震动振幅缩放进行结构非线性分析，可能会使得IM与结构地震响应之间的相关性出现偏差；同时，地震记录的选取还要综合考虑工程场地条件并避免对某个地震事件的依赖性。本文从PEER强震记录数据库中选取25个不同地震事件的50条远场地震动记录。到目前为止，对于近远场地震的划分并无统一的规定，通常以断层距作为近远场的划分依据。已有文献给出了不同的划分标准，如20 km （Bray，Rodriguez-Marek，2004），23 km （Akkar，Özen，2005），10 km （FEMA，2009）等，综合考虑后本文选取15 km作为近远场的划分依据。因此，本文所选取地震动记录的断层距均大于15 km，其PGA范围为0.019g—0.229g，PGV范围为0.52—19.07 cm/s，PGD范围为0.07—11.58 cm。所选取地震动的详细信息见表3，相应的伪加速度反应谱如图2所示。
![]()
表 3 本研究中使用的地震动记录Table 3. Ground motions records used in this study编号 地震名称 年份 vS30/（m·s−1） 地震动分量 断层距/km PGA/g PGV/（cm·s−1） PGD/cm 1 Kern County 1952 514.99 SBA042 82.19 0.090 11.41 3.43 SBA132 0.132 19.07 5.49 2 Lytle Creek 1970 667.13 DCF090 20.24 0.172 3.57 0.40 DCF180 0.162 6.50 0.99 3 San Fernando 1971 529.09 PPP000 38.97 0.104 4.95 1.26 PPP270 0.138 5.46 1.09 4 Northern Calif-07 1975 518.98 SCP070 63.64 0.074 2.13 0.09 SCP160 0.108 2.28 0.09 5 Livermore-01 1980 517.06 A3E146 30.59 0.065 3.91 0.79 A3E236 0.057 2.68 0.50 6 Anza （Horse Canyon）-01 1980 724.89 PFT045 17.26 0.099 2.04 0.18 PFT135 0.122 5.19 0.59 7 Coalinga-01 1983 522.74 TM2000 42.92 0.026 3.61 1.13 TM2090 0.037 5.72 1.43 8 Taiwan SMART1（25） 1983 671.52 25EO2EW 92.04 0.020 1.45 0.36 25EO2NS 0.020 2.50 0.44 9 Borah Peak_ID-02 1983 612.78 HAU000 49.02 0.029 0.63 0.07 HAU090 0.033 0.52 0.08 10 Morgan Hill 1984 543.63 SJL270 31.88 0.081 7.31 3.74 SJL360 0.070 5.22 2.20 11 Veroia_Greece 1984 551.30 NS 16.89 0.032 3.13 0.26 WE 0.044 3.94 0.35 12 N. Palm Springs 1986 532.85 H01000 54.82 0.054 1.70 0.13 H01090 0.049 1.28 0.16 13 Chalfant Valley-02 1986 529.39 MAM020 36.47 0.042 2.15 0.60 MAM290 0.048 3.17 0.70 14 Taiwan SMART1（45） 1986 671.52 45EO2EW 51.35 0.136 14.42 6.72 45EO2NS 0.142 12.54 6.61 15 Whittier Narrows-01 1987 508.08 PKC000 36.12 0.158 7.73 1.08 PKC090 0.163 7.71 1.08 16 Loma Prieta 1989 517.06 A3E000 52.53 0.079 6.14 4.64 A3E090 0.084 7.07 4.27 17 Griva_Greece 1990 551.30 NS 33.29 0.103 11.03 1.22 WE 0.098 8.69 0.89 18 Cape Mendocino 1992 518.98 SHL000 28.78 0.229 6.92 0.39 SHL090 0.189 6.30 0.52 19 Landers 1992 659.09 SIL000 50.85 0.050 3.76 1.93 SIL090 0.040 5.08 4.04 20 Big Bear-01 1992 509.10 CUC090 59.87 0.051 3.42 0.59 CUC180 0.032 1.95 0.43 21 Northridge-01 1994 572.57 ATB000 46.91 0.046 3.20 1.82 ATB090 0.068 4.16 1.97 22 Kobe_Japan 1995 609.00 CHY000 49.91 0.092 5.32 2.86 CHY090 0.110 4.12 0.97 23 Kozani_Greece-01 1995 579.40 L 49.66 0.019 1.40 0.27 T 0.019 1.49 0.26 24 Hector Mine 1999 724.89 PFT090 89.98 0.036 5.12 1.77 PFT360 0.027 2.30 1.90 25 Duzce_Turkey 1999 782.00 N 25.88 0.053 5.75 5.28 E 0.025 9.98 11.58 2.2 地震动强度指标
研究人员基于不同的标准提出了多种IM，包括单一参数型和复合型。Nau和Hall （1984）指出复合型IM针对地面运动也未能全面反映输入地震动记录对结构损伤程度的影响规律，且复合型指标的计算较为复杂，不便于工程应用，因此形式简单、使用方便的地震动峰值指标仍旧使用较多，故本文选择PGA，PGV和PGD作为研究指标。Riddell （2007）将指标分为加速度相关型、速度相关型以及位移相关型三种，本文选取的三个指标分别作为这三种指标类型的代表。
2.3 工程需求参数
工程需求参数EDP是用来描述结构地震响应及损伤的参数。在基于性能的地震工程计算中，EDP的选取对计算结果的准确性至关重要。对于地上结构，诸如最大层间位移比、最大层间加速度等EDP被广泛应用（Luco，Cornell，2007；Padgett et al，2008；Yang et al，2009）。而针对地下结构的EDP目前尚无统一标准，多项研究选择了各种各样的结构地震响应进行地下结构的评价分析（An et al，1997；Liu et al，2017；钟紫蓝等，2020），但地下结构的最大层间位移被广泛应用。因此，本文选择矩形地下结构（结构高度为7 m）顶底板处对应场地的最大水平位移差作为场地的EDP，埋深设定为结构顶板到地表的距离，如图3所示。因地下结构的响应受到周围场地变形的控制，该EDP的选择有一定的代表性。
3. 最优地震动强度指标的评价
3.1 有效性
有效性可以描述在确定的地震动强度指标IM下响应的离散程度，即在确定的IM下，EDP的离散性较小，有效性较好，此时，可以在不降低精度的情况下减少计算时输入地震动记录的数量和动力时程分析的次数（Luco，Cornell，2007）。Cornell等（2002）指出EDP与IM之间大致满足幂函数关系，可以写为对数线性关系，即
$$ \ln {\rm{EDP}} {\text{＝}} \ln a {\text{＋}} b\ln {\rm{IM}}{\text{.}} $$ (1) 对计算结果进行线性回归，可得常数a和b的值，进而求得代表有效性的标准差β
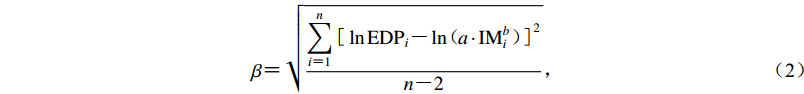
$$ \beta {\text{＝}} \sqrt {\frac{{\displaystyle\sum\limits_{i {\text{＝}} 1}^n {{{{\text{[}} \;{\ln {{\rm{ED}}{{\rm{P}}_{i}}} {\text{－}} \ln （ {a \cdot {\rm{I}}{{\rm{M}}^{b}_{i}}} {\text{）}}} {\text{]}}}^2}} }}{{n {\text{－}} 2}}} {\text{，}} $$ (2) 式中，EDPi为每条地震动下的场地响应值，IMi为每条地震动的指标值，n为地震动数量。有效性越好，β越小。如图4所示，PGV的有效性优于PGA。
3.2 实用性
实用性是指EDP与IM之间是否存在直接关系，如果某IM实用性不强，则表明EDP几乎不受该IM变化的影响。实用性采用式（1）中的线性回归常数b来判断，b值越大，地面运动强度指标变化对EDP的影响就越大，即实用性较高。如果b值趋于0，则IM的变化对EDP无影响。由图4可见PGA比PGV的实用性更强。
3.3 效益性
只使用有效性或实用性来评价IM可能会出现相互矛盾的情况，如图4所示，基于有效性评价，PGV的有效性优于PGA，而基于实用性评价，PGA的实用性优于PGV。效益性综合考虑有效性和实用性（Padgett et al，2008），采用
$$ \zeta {\text{＝}} \frac{\beta }{b} $$ (3) 表示，ζ值越小表示IM的效益性越强。本文以效益性作为IM的评价准则。
4. 计算结果与讨论
通常地下结构响应受控于周围岩土体的变形，所以从简单场地开始探索规律。本文将设计均匀半空间和成层半空间两类场地展开规律的探究，对均匀半空间场地先粗略地取0，2，5，7，10，13，15，18，20，25，30，35，40，60 m等14个埋深进行研究，由此获得效益性结果随埋深的变化曲线。
4.1 最优IM转折位置
4.1.1 均匀半空间场地
图5为10个场地的效益性随埋深的变化曲线，可以看到：对于剪切波速较小的场地1，所有埋深下PGV均为最优IM；对于剪切波速相对较大的场地2—10，在埋深浅时PGA为最优IM，埋深较深时PGV为最优IM，因此存在一个随埋深增加最优IM由PGA转变为PGV的转折深度。为了更准确地确定转折深度，在转折深度附近每隔1 m取一个埋深值加密计算。
![]()
图 5 均匀半空间场地1—10中效益性ζ随埋深变化图（a） 场地1；（b） 场地 2；（c） 场地 3；（d） 场地 4；（e） 场地 5；（f） 场地 6；（g）场地 7；（h） 场地 8 ；（i） 场地 9；（j） 场地 10Figure 5. The proficiency ζ varying with burial depth in homogeneous half-space sites 1−10（a） Site 1；（b） Site 2；（c） Site 3；（d） Site 4；（e） Site 5；（f） Site 6；（g） Site 7；（h） Site 8；（i） Site 9；（j） Site 10存在上述转折现象的原因可能是由于埋深较浅时，场地响应受惯性力的影响较大，因此PGA为最优IM；随着埋深增加，场地响应受土体剪切变形控制，而场地土体剪应变与PGV具有相关性，因此埋深较深时PGV为最优IM。
4.1.2 成层半空间场地
成层半空间场地的埋深取值与均匀半空间场地一致。图6为场地11—18的效益性随埋深的变化曲线。从图中可以看到：对于剪切波速较小的场地11，所有埋深下PGV均为最优IM；对于剪切波速相对较大的场地12—18，在埋深浅时PGA为最优IM，埋深较深时PGV为最优IM，存在一个随埋深增加最优IM由PGA转变为PGV的转折深度，规律与均匀半空间场地相同。
![]()
图 6 成层半空间场地11—18中效益性ζ随埋深变化图（a） 场地 11；（b） 场地 12；（c） 场地 13；（d） 场地 14；（e） 场地 15；（f） 场地 16；（g） 场地 17；（h） 场地 18Figure 6. The proficiency ζ varying with burial depth in layered half-space sites 11−18（a） Site 11；（b） Site 12；（c） Site 13；（d） Site 14；（e） Site 15；（f） Site 16；（g） Site 17；（h） Site 184.2 IM转折深度与场地剪切波速的关系
从图5和图6可以看到，在均匀半空间和成层半空间场地中，不同场地条件下最优IM的转折深度不同。图7给出了两种场地类型下最优IM的转折深度随场地剪切波速变化的关系，两者的线性回归曲线也绘于图中。
![]()
图 7 最优IM的转折深度与剪切波速的关系Figure 7. The depth of the transition for optimal IM varying with shear wave velocity$$ H{\text{＝}}\left\{\begin{array}{c}0.06{v}_{{\rm{S}}}{\text{－}}5.27\qquad （{\text{均匀半空间}}） \\ 0.06{v}_{{\rm{S}}}{\text{－}}9.00\qquad （{\text{成层半空间}}）\end{array}\right. $$ (4) 为两种场地的线性回归方程，式中H为转折深度，vS为场地剪切波速。
从图7可以看到，最优IM的转折深度与场地剪切波速较好地符合线性关系，因此可用回归方程计算其它剪切波速大于100 m/s的均匀半空间场地以及本文涉及的成层半空间场地的最优IM转折深度。从图中还可看出，均匀半空间场地中的线性拟合优于成层半空间场地，这可能是由于成层半空间引入了阻尼和覆盖层厚度等参数，对其产生了一定的影响。
5. 结论
本文基于从PEER中获取的实际地震动，采用EERA软件计算得到均匀半空间场地、成层半空间场地不同埋深处的水平位移差，利用效益性评价了地震动峰值指标（PGA，PGV，PGD）随埋深的变化规律，得到以下结论：
1） 最优IM随埋深变化，个别波速较小场地的最优IM始终为PGV；大多数场地下，随着埋深增加出现最优IM由PGA转向PGV的转折深度。本文研究场地条件下的转折深度范围为0—25 m。
2） 最优IM的转折深度与场地剪切波速存在线性关系。均匀半空间场地的转折深度与回归直线相差0—1.1%，成层半空间场地的相差2.64%—18.75%。
本文将场地水平位移差作为EDP，后续研究中应考虑将结构响应作为EDP进行研究；同时实际场地的覆盖层厚度各不相同，后续将考虑不同的覆盖层厚度展开研究。
广州南粤地震工程勘察有限公司. 2020. 深圳市城市轨道交通3号线四期工程：工程场地地震安全性评价专题报告：145–148. -
![]()
图 1 剪切模量比和阻尼比随剪应变的变化
Figure 1. Shear modulus ratio and damping ratio varying with the shear strain
![]()
![]()
图 5 均匀半空间场地1—10中效益性ζ随埋深变化图
（a） 场地1；（b） 场地 2；（c） 场地 3；（d） 场地 4；（e） 场地 5；（f） 场地 6；（g）场地 7；（h） 场地 8 ；（i） 场地 9；（j） 场地 10
Figure 5. The proficiency ζ varying with burial depth in homogeneous half-space sites 1−10
（a） Site 1；（b） Site 2；（c） Site 3；（d） Site 4；（e） Site 5；（f） Site 6；（g） Site 7；（h） Site 8；（i） Site 9；（j） Site 10
![]()
图 6 成层半空间场地11—18中效益性ζ随埋深变化图
（a） 场地 11；（b） 场地 12；（c） 场地 13；（d） 场地 14；（e） 场地 15；（f） 场地 16；（g） 场地 17；（h） 场地 18
Figure 6. The proficiency ζ varying with burial depth in layered half-space sites 11−18
（a） Site 11；（b） Site 12；（c） Site 13；（d） Site 14；（e） Site 15；（f） Site 16；（g） Site 17；（h） Site 18
![]()
图 7 最优IM的转折深度与剪切波速的关系
Figure 7. The depth of the transition for optimal IM varying with shear wave velocity
表 1 均匀半空间场地信息
Table 1 Information of homogeneous half-space sites
场地序号 密度/（kg·m−3） 剪切波速/（m·s−1） 场地类别 1 1 800 85 Ⅳ 2 1 820 100 Ⅳ 3 1 850 150 Ⅲ 4 1 920 200 Ⅲ 5 1 920 250 Ⅲ 6 1 970 300 Ⅱ 7 1 970 350 Ⅱ 8 2 100 400 Ⅱ 9 2 100 450 Ⅱ 10 2 300 500 Ⅰ 
下载: 导出CSV
表 2 成层半空间场地信息
Table 2 Information of layered half-space sites
场地序号 分层 土类号 厚度/m 泊松比 密度/（kg·m−3） 剪切波速/（m·s−1） 场地类别 11 土层 1 40 0.42 1 820 113 Ⅲ 基岩 6 ∞ 0.20 2 300 500 12 土层 2 40 0.38 1 850 166 Ⅱ 基岩 6 ∞ 0.20 2 300 500 13 土层 3 40 0.35 1 920 210 Ⅱ 基岩 6 ∞ 0.20 2 300 500 14 土层 4 40 0.26 1 920 254 Ⅱ 基岩 6 ∞ 0.20 2 300 500 15 土层 5 40 0.30 1 970 312 Ⅱ 基岩 6 ∞ 0.20 2 300 500 16 土层 5 40 0.30 1 970 360 Ⅱ 基岩 6 ∞ 0.20 2 300 500 17 土层 5 40 0.27 2 100 425 Ⅱ 基岩 6 ∞ 0.20 2 300 500 18 土层 5 40 0.27 2 100 493 Ⅱ 基岩 6 ∞ 0.20 2 300 500
下载: 导出CSV
表 3 本研究中使用的地震动记录
Table 3 Ground motions records used in this study
编号 地震名称 年份 vS30/（m·s−1） 地震动分量 断层距/km PGA/g PGV/（cm·s−1） PGD/cm 1 Kern County 1952 514.99 SBA042 82.19 0.090 11.41 3.43 SBA132 0.132 19.07 5.49 2 Lytle Creek 1970 667.13 DCF090 20.24 0.172 3.57 0.40 DCF180 0.162 6.50 0.99 3 San Fernando 1971 529.09 PPP000 38.97 0.104 4.95 1.26 PPP270 0.138 5.46 1.09 4 Northern Calif-07 1975 518.98 SCP070 63.64 0.074 2.13 0.09 SCP160 0.108 2.28 0.09 5 Livermore-01 1980 517.06 A3E146 30.59 0.065 3.91 0.79 A3E236 0.057 2.68 0.50 6 Anza （Horse Canyon）-01 1980 724.89 PFT045 17.26 0.099 2.04 0.18 PFT135 0.122 5.19 0.59 7 Coalinga-01 1983 522.74 TM2000 42.92 0.026 3.61 1.13 TM2090 0.037 5.72 1.43 8 Taiwan SMART1（25） 1983 671.52 25EO2EW 92.04 0.020 1.45 0.36 25EO2NS 0.020 2.50 0.44 9 Borah Peak_ID-02 1983 612.78 HAU000 49.02 0.029 0.63 0.07 HAU090 0.033 0.52 0.08 10 Morgan Hill 1984 543.63 SJL270 31.88 0.081 7.31 3.74 SJL360 0.070 5.22 2.20 11 Veroia_Greece 1984 551.30 NS 16.89 0.032 3.13 0.26 WE 0.044 3.94 0.35 12 N. Palm Springs 1986 532.85 H01000 54.82 0.054 1.70 0.13 H01090 0.049 1.28 0.16 13 Chalfant Valley-02 1986 529.39 MAM020 36.47 0.042 2.15 0.60 MAM290 0.048 3.17 0.70 14 Taiwan SMART1（45） 1986 671.52 45EO2EW 51.35 0.136 14.42 6.72 45EO2NS 0.142 12.54 6.61 15 Whittier Narrows-01 1987 508.08 PKC000 36.12 0.158 7.73 1.08 PKC090 0.163 7.71 1.08 16 Loma Prieta 1989 517.06 A3E000 52.53 0.079 6.14 4.64 A3E090 0.084 7.07 4.27 17 Griva_Greece 1990 551.30 NS 33.29 0.103 11.03 1.22 WE 0.098 8.69 0.89 18 Cape Mendocino 1992 518.98 SHL000 28.78 0.229 6.92 0.39 SHL090 0.189 6.30 0.52 19 Landers 1992 659.09 SIL000 50.85 0.050 3.76 1.93 SIL090 0.040 5.08 4.04 20 Big Bear-01 1992 509.10 CUC090 59.87 0.051 3.42 0.59 CUC180 0.032 1.95 0.43 21 Northridge-01 1994 572.57 ATB000 46.91 0.046 3.20 1.82 ATB090 0.068 4.16 1.97 22 Kobe_Japan 1995 609.00 CHY000 49.91 0.092 5.32 2.86 CHY090 0.110 4.12 0.97 23 Kozani_Greece-01 1995 579.40 L 49.66 0.019 1.40 0.27 T 0.019 1.49 0.26 24 Hector Mine 1999 724.89 PFT090 89.98 0.036 5.12 1.77 PFT360 0.027 2.30 1.90 25 Duzce_Turkey 1999 782.00 N 25.88 0.053 5.75 5.28 E 0.025 9.98 11.58
下载: 导出CSV
-
陈健云,李静,韩进财,徐强. 2017. 地震动强度指标与框架结构响应的相关性研究[J]. 振动与冲击,36(3):105–112. Chen J Y,Li J,Han J C,Xu Q. 2017. Correlation between ground motion intensity indexes and seismic responses of frame structures[J]. Journal of Vibration and Shock,36(3):105–112 (in Chinese).
崔光耀,伍修刚,王明年,林国进. 2017. 汶川8.0级大地震公路隧道震害调查与震害特征[J]. 现代隧道技术,54(2):9–16. Cui G Y,Wu X G,Wang M N,Lin G J. 2017. Earthquake damages and characteristics of highway tunnels in the 8.0-magnitude Wenchuan earthquake[J]. Modern Tunnelling Technology,54(2):9–16 (in Chinese).
董正方,朱红云,蔡宝占,闫超. 2017. 不同埋深处土质场地地下结构地震内力变化规律[J]. 工程抗震与加固改造,39(5):143–148. Dong Z F,Zhu H Y,Cai B Z,Yan C. 2017. Law of internal force changes for understructures in soil site with different depth under earthquake[J]. Earthquake Resistant Engineering and Retrofitting,39(5):143–148 (in Chinese).
耿方方,丁幼亮,谢辉,李爱群,宋建永,李万恒,王玉倩. 2013. 近断层地震动作用下长周期结构的地震动强度指标[J]. 东南大学学报(自然科学版),43(1):203–208. Geng F F,Ding Y L,Xie H,Li A Q,Song J Y,Li W H,Wang Y Q. 2013. Ground motion intensity indices for long period structures subjected to near-fault ground motion[J]. Journal of Southeast University (Natural Science Edition)
,43(1):203–208 (in Chinese). 李长青,戚承志,罗健. 2011. 地下结构埋深对结构自身响应的影响分析[J]. 防灾减灾工程学报,31(增刊1):167–172. Li C Q,Qi C Z,Luo J. 2011. Study of influence of buried depth on seismic response of underground structures[J]. Journal of Disaster Prevention and Mitigation Engineering,31(S1):167–172 (in Chinese).
李雪红,李晔暄,吴迪,徐秀丽,李枝军. 2014. 地震动强度指标与结构地震响应的相关性研究[J]. 振动与冲击,33(23):184–189. Li X H,Li Y X,Wu D,Xu X L,Li Z J. 2014. Correlation between ground motion intensity and structural seismic response[J]. Journal of Vibration and Shock,33(23):184–189 (in Chinese).
卢啸,陆新征,叶列平,李梦珂. 2014. 适用于超高层建筑的改进地震动强度指标[J]. 建筑结构学报,35(2):15–21. Lu X,Lu X Z,Ye L P,Li M K. 2014. Development of an improved ground motion intensity measure for super high-rise buildings[J]. Journal of Building Structures,35(2):15–21 (in Chinese).
于天昊. 2016. 近场地震动下适于单层网壳结构响应评估的地震动强度参数[D]. 天津: 天津大学: 1–92. Yu T H. 2016. Ground Motion Intensity Measures for the Evaluation of Effects of Pulse-Type Ground Motions on the Response of Single-Layer Reticulated Shells[D]. Tianjin: Tianjin University: 1–92 （in Chinese）.
于翔. 2002. 地下建筑结构应充分考虑抗震问题:1995年阪神地震破坏的启示[J]. 工程抗震,(4):17–20. Yu X. 2002. Anti-seismic action should be sufficiently considered in constructing metro:Illumine of the 1995 Hyogoken-Nanbu earthquake[J]. Earthquake Resistant Engineering,(4):17–20 (in Chinese).
于晓辉. 2012. 钢筋混凝土框架结构的概率地震易损性与风险分析[D]. 哈尔滨: 哈尔滨工业大学: 61–90. Yu X H. 2012. Probabilistic Seismic Fragility and Risk Analysis of Reinforced Concrete Frame Structures[D]. Harbin: Harbin Institute of Technology: 61–90 （in Chinese）.
中华人民共和国住房和城乡建设部, 中华人民共和国国家质量监督检验检疫总局. 2010. GB 50011—2010 建筑抗震设计规范[S]. 北京: 中国建筑工业出版社: 18–21. Ministry of Housing and Urban-Rural Development of the People’s Republic of China, General Administration of Quality Supervision, Inspection and Quarantine of the People’s Republic of China. 2010. GB 50011−2010 Code for Seismic Design of Buildings[S]. Beijing: China Architecture & Building Press: 18–21 （in Chinese）.
钟紫蓝,申轶尧,甄立斌,张成明,赵密,杜修力. 2020. 地震动强度参数与地铁车站结构动力响应指标分析[J]. 岩土工程学报,42(3):486–494. Zhong Z L,Shen Y Y,Zhen L B,Zhang C M,Zhao M,Du X L. 2020. Ground motion intensity measures and dynamic response indexes of metro station structures[J]. Chinese Journal of Geotechnical Engineering,42(3):486–494 (in Chinese).
左占宣,李爽,翟长海,谢礼立. 2019. 结构周期延长对倒塌分析中地震动强度指标选择的影响[J]. 建筑结构学报,40(5):141–148. Zuo Z X,Li S,Zhai C H,Xie L L. 2019. Influence of structural period elongation on ground motion intensity index in collapse analysis[J]. Journal of Building Structures,40(5):141–148 (in Chinese).
Akkar S,Özen Ö. 2005. Effect of peak ground velocity on deformation demands for SDOF systems[J]. Earthq Eng Struct Dyn,34(13):1551–1571. doi: 10.1002/eqe.492
An X H,Shawky A A,Maekawa K. 1997. The collapse mechanism of a subway station during the great Hanshin earthquake[J]. Cem Concr Compos,19(3):241–257. doi: 10.1016/S0958-9465(97)00014-0
Bray J D,Rodriguez-Marek A. 2004. Characterization of forward-directivity ground motions in the near-fault region[J]. Soil Dyn Earthq Eng,24(11):815–828. doi: 10.1016/j.soildyn.2004.05.001
Chen Z Y,Wei J S. 2013. Correlation between ground motion parameters and lining damage indices for mountain tunnels[J]. Nat Hazards,65(3):1683–1702. doi: 10.1007/s11069-012-0437-5
Cornell C A,Jalayer F,Hamburger R O,Foutch D A. 2002. Probabilistic basis for 2000 SAC Federal Emergency Management Agency steel moment frame guidelines[J]. J Struct Eng,128(4):526–533. doi: 10.1061/(ASCE)0733-9445(2002)128:4(526)
Dávalos H,Miranda E. 2019. Evaluation of bias on the probability of collapse from amplitude scaling using spectral-shape-matched records[J]. Earthq Eng Struct Dyn,48(8):970–986. doi: 10.1002/eqe.3172
FEMA. 2009. Quantification of Building Seismic Performance Factors: FEMA P695[R]. Washington: Federal Emergency Management Agency: 280–281.
Hashash Y M A,Hook J J,Schmidt B,Yao J I C. 2001. Seismic design and analysis of underground structures[J]. Tunn Undergr Space Technol,16(4):247–293. doi: 10.1016/S0886-7798(01)00051-7
Liu T,Chen Z Y,Yuan Y,Shao X Y. 2017. Fragility analysis of a subway station structure by incremental dynamic analysis[J]. Adv Struct Eng,20(7):1111–1124. doi: 10.1177/1369433216671319
Luco N,Cornell C A. 2007. Structure-specific scalar intensity measures for near-source and ordinary earthquake ground motions[J]. Earthq Spectra,23(2):357–392. doi: 10.1193/1.2723158
Nau J M,Hall W J. 1984. Scaling methods for earthquake response spectra[J]. J Struct Eng,110(7):1533–1548. doi: 10.1061/(ASCE)0733-9445(1984)110:7(1533)
Padgett J E,Nielson B G,DesRoches R. 2008. Selection of optimal intensity measures in probabilistic seismic demand models of highway bridge portfolios[J]. Earthq Eng Struct Dyn,37(5):711–725. doi: 10.1002/eqe.782
Pitilakis K,Tsinidis G,Leanza A,Maugeri M. 2014. Seismic behaviour of circular tunnels accounting for above ground structures interaction effects[J]. Soil Dyn Earthq Eng,67:1–15. doi: 10.1016/j.soildyn.2014.08.009
Riddell R. 2007. On ground motion intensity indices[J]. Earthq Spectra,23(1):147–173. doi: 10.1193/1.2424748
Scawthorn C,O’Rourke T D,Blackburn F T. 2006. The 1906 San Francisco earthquake and fire:Enduring lessons for fire protection and water supply[J]. Earthq Spectra,22(S2):135–158.
Wang W L,Wang T T,Su J J,Lin C H,Seng C R,Huang T H. 2001. Assessment of damage in mountain tunnels due to the Taiwan Chi-Chi earthquake[J]. Tunn Undergr Space Technol,16(3):133–150. doi: 10.1016/S0886-7798(01)00047-5
Yang C T,Xie L L,Li A Q,Jia J B,Zeng D M. 2019. Ground motion intensity measures for seismically isolated RC tall buildings[J]. Soil Dyn Earthq Eng,125:105727. doi: 10.1016/j.soildyn.2019.105727
Yang D X,Pan J W,Li G. 2009. Non-structure-specific intensity measure parameters and characteristic period of near-fault ground motions[J]. Earthq Eng Struct Dyn,38(11):1257–1280. doi: 10.1002/eqe.889
-
期刊类型引用(7)
1. 张斌，孙尧，马秀敏，彭华，姜景捷，毛佳睿，张文汇，翟玉栋. 东构造结墨脱关键区域地应力场特征及其构造稳定性分析. 地质力学学报. 2023(03): 388-401 . 
百度学术
2. 刘莎，杨建思，郑钰. 南迦巴瓦地区近震剪切波分裂研究. 地球物理学报. 2023(09): 3692-3703 . 百度学术
3. 曹学来，常利军，鲁来玉，吴萍萍，郭慧丽，吕苗苗，丁志峰. 2021年青海玛多M_S7.4地震震源区横波分裂变化特征. 地球物理学报. 2022(05): 1644-1659 . 百度学术
4. 张晨，季灵运，朱良玉，徐晶. 基于震源应力与GPS应变分析喜马拉雅东构造结及其邻区地壳变形特征. 地震研究. 2022(04): 526-534 . 百度学术
5. 李鸿儒，白玲，詹慧丽. 嘉黎断裂带活动性研究进展. 地球与行星物理论评. 2021(02): 182-193 . 百度学术
6. 黄臣宇，常利军. 基于横波分裂的青藏高原多圈层各向异性研究进展. 地球与行星物理论评. 2021(02): 164-181 . 百度学术
7. 黄臣宇，常利军，丁志峰. 喜马拉雅东构造结及周边地区地壳各向异性特征. 地球物理学报. 2021(11): 3970-3982 . 百度学术
其他类型引用(6)
计量
- 文章访问数: 324
- HTML全文浏览量: 149
- PDF下载量: 52
- 被引次数: 13
