Influence of the finite fault location on the three-dimensional basin seismic effect
-
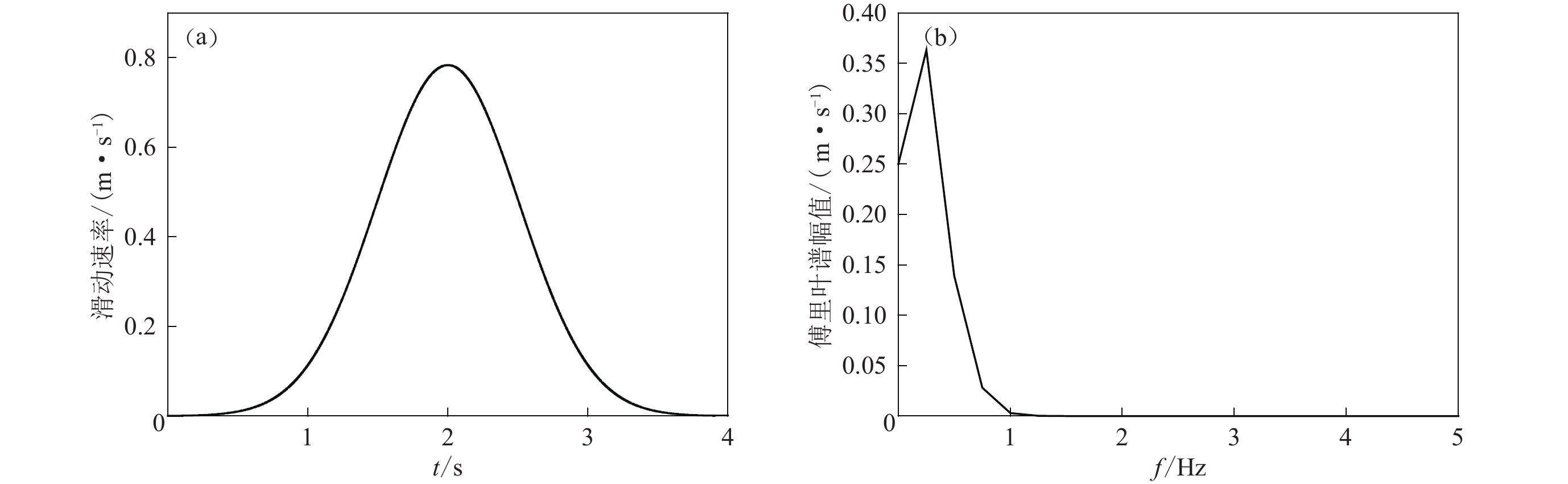
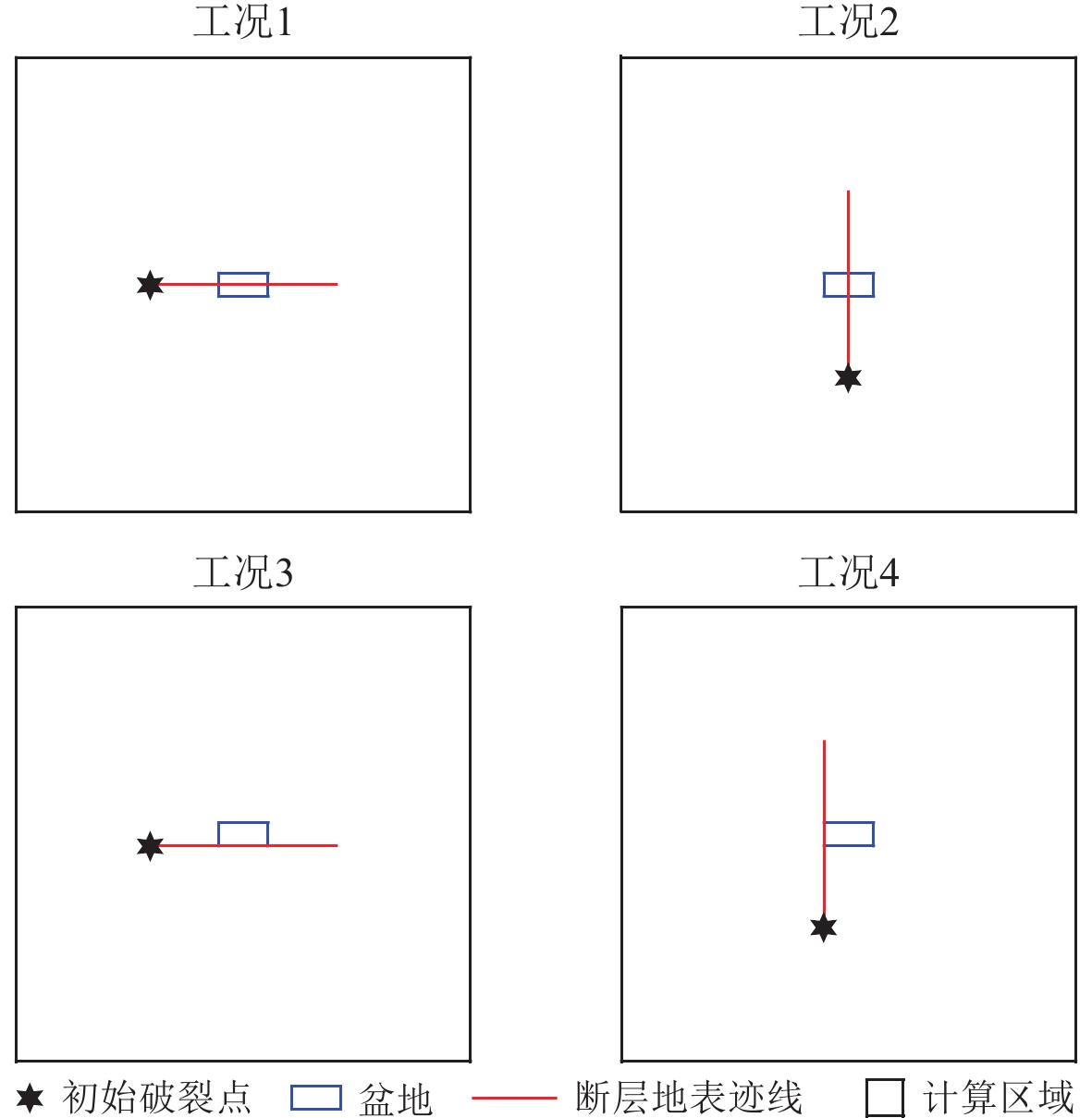
摘要: 采用谱元法模拟了盆地模型和一维水平成层模型的地震动强度和放大系数的分布特征，探究了直下型断层与盆地的相对位置对盆地地震效应的影响。结果显示：盆地地震动受断层位置的影响显著，特别是当断层邻近且平行于盆地边缘时，盆地地表地震动的边缘效应更加明显，强烈地震动区域的位置随断层位置的移动而改变明显；不同分量放大系数的分布特征差别显著，平行断层分量和垂直分量的显著放大区域主要分布于断层地表迹线两侧或紧邻迹线的盆地边缘，而垂直断层分量主要位于盆地内部，其放大系数最小；断层沿盆地短边边缘破裂时的地震动强度和放大系数最大，为最危险情况。Abstract: The earthquake caused by underneath faults often causes serious damage to the cities above the basin. In this paper, by comparing the distribution characteristics of ground motion intensity and amplification factor between the basin model and the one-dimensional horizontal layered model, the influence of the fault location （FL） on the basin seismic effect is investigated in detail. The results show that the FL has significant influence on the strong motion inside the basin. The basin edge effect is more significant when the fault is close to and parallel to the basin boundary, and the locations of the strongest ground motion regions change with the FL. The amplification factor （AF） exhitbits different distribution characteristics for three components. The main amplification regions of the fault-parallel and vertical components generally locate on both sides of the fault trace or near the basin edge close to it, while the main amplification regions for the fault-normal component locate inside the basin, which also displays the smallest AF. Both the strong motion and AF show greatest values when the fault just beneath the basin short-side edge, which is the most hazardous case.
-
-
![]()
图 2 震源滑动速率时间函数（a）及其对应的傅里叶振幅谱（b）
Figure 2. Slip rate time function （a） and its Fourier spectrum （b） of the source
![]()
图 3 本文考虑的四种不同断层位置工况（俯视图）
Figure 3. The four fault location cases considered in this study （vertical view）
![]()
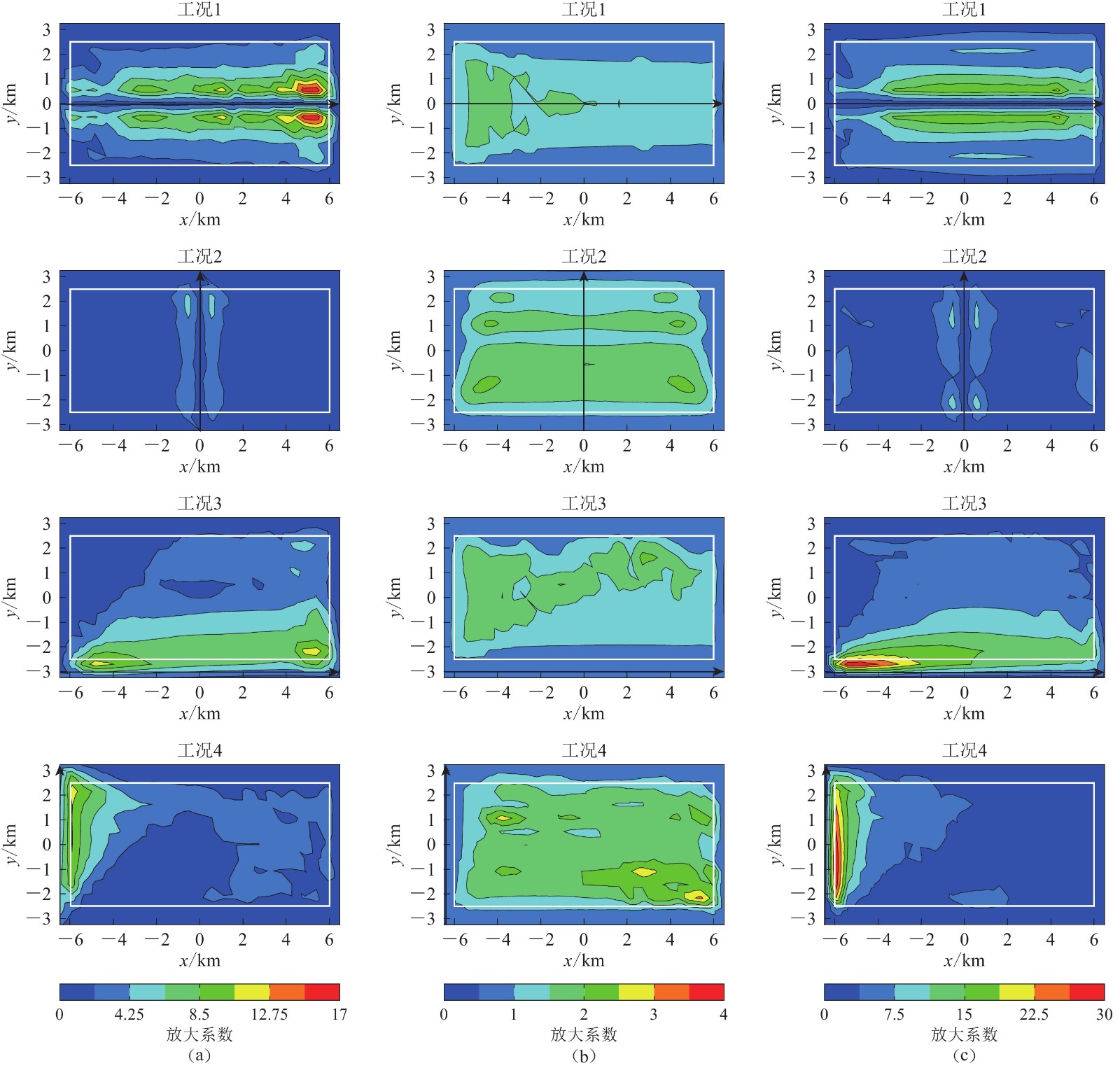
图 4 不同断层位置下盆地内三分量的PGV分布
箭头所示为断层的破裂方向，白色框为盆地基底边界。（a） 平行断层分量；（b） 垂直断层分量；（c） 垂直分量
Figure 4. PGV distributions inside the basin for different fault location models
The arrows denote the rupture direction of the fault，and the white frame gives the boundary of the basin base（a） Fault-parallel component；（b） Fault-normal component；（c） UD component
![]()
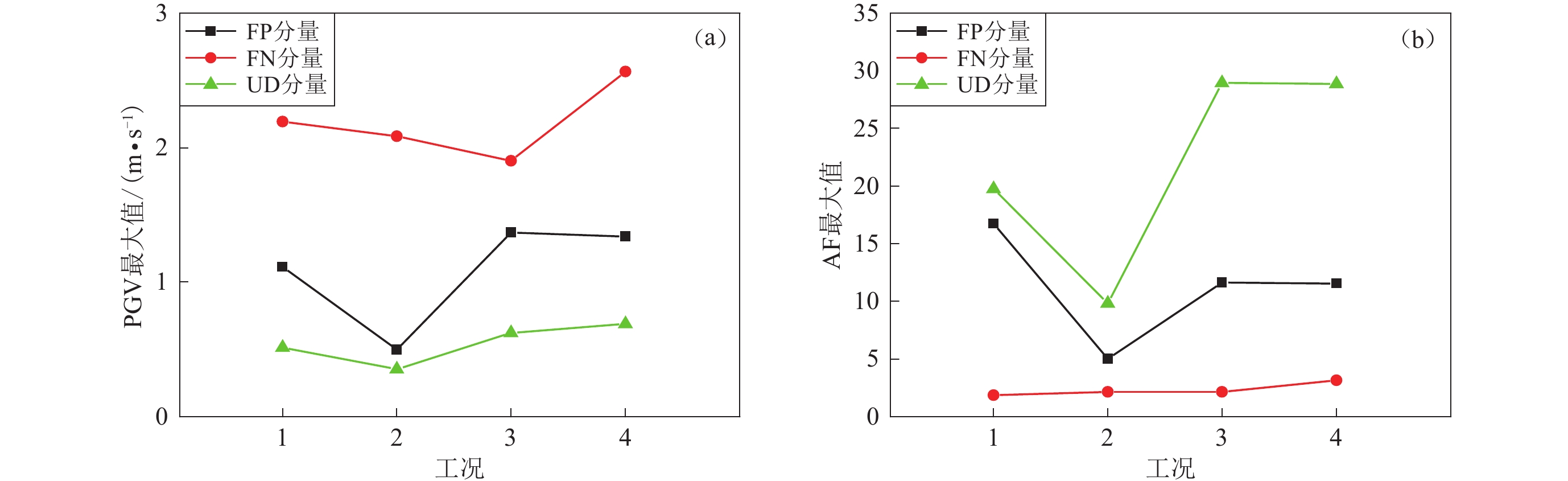
图 5 不同断层位置下盆地内三分量放大系数分布
箭头所示为断层的破裂方向，白色框为盆地基底边界。（a） 平行断层分量；（b） 垂直断层分量；（c） 垂直分量
Figure 5. Amplification factor distributions inside the basin for different fault location models
The arrows denote the rupture direction of the fault，and the white frame gives the boundary of the basin base（a） Fault-parallel component；（b） Fault-normal component；（c） UD component
![]()
图 6 不同断层位置时盆地内PGV （a）及放大因子AF （b）的最大值变化
Figure 6. The maximum values of PGV （a） and AF （b） inside the basin for different fault location models
表 1 盆地模型的介质参数
Table 1 Medium parameters of basin model
vS/（km·s−1） vP/（km·s−1） ρ/（g·cm−3） 盆地外 2.4 5.2 2.4 盆地内 0.7 2.0 1.9 
下载: 导出CSV
表 2 盆地模型地震动数值模拟参数
Table 2 Numerical simulation parameters of ground motion in the basin model
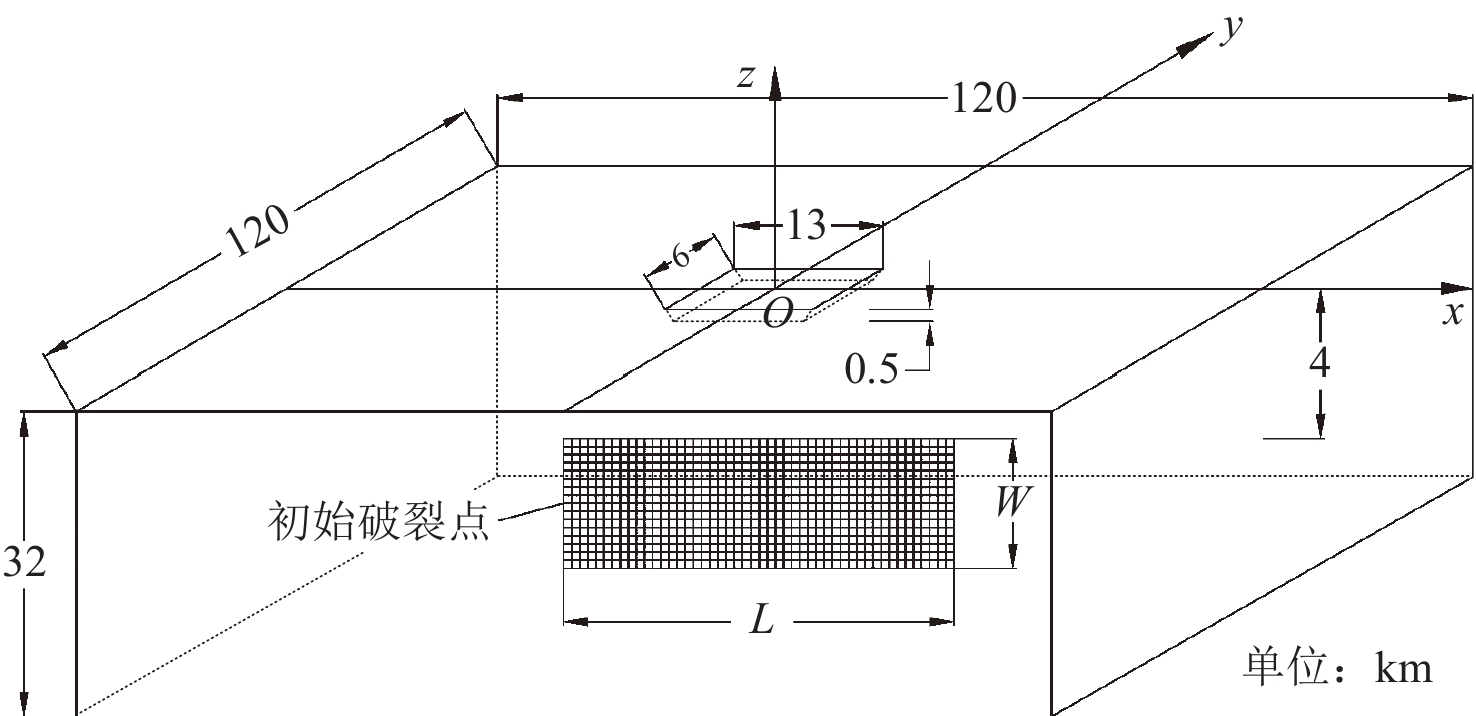
断层参数 初始破裂点
深度/km破裂速度
/（km·s−1）平均上升
时间/s平均位错
/m长度
/km宽度
/km倾角
/°走向
/°滑动角
/°顶面埋深
/km49 17 90 90 0 4.0 12.0 2.16 1.1 1.1
下载: 导出CSV
-
韩天成,于彦彦,丁海平. 2020. 直下型断层的破裂速度对盆地地震效应的影响[J]. 地震学报,42(4):457–470. doi: 10.11939/jass.20190177 Han T C,Yu Y Y,Ding H P. 2020. Influence of rupture velocity of the directly-beneath fault on the basin seismic effect[J]. Acta Seismologica Sinica,42(4):457–470 (in Chinese).
刘启方,袁一凡,金星. 2004. 断层附近地面地震动空间分布[J]. 地震学报,26(2):183–192. doi: 10.3321/j.issn:0253-3782.2004.02.008 Liu Q F,Yuan Y F,Jin X. 2004. Spatial distribution of near-fault ground motion[J]. Acta Seismologica Sinica,26(2):183–192 (in Chinese).
刘中宪,尚策,王小燕,王冬. 2017. 三维沉积盆地对地震动的放大效应:间接边界元法模拟[J]. 地震学报,39(1):111–131. doi: 10.11939/jass.2017.01.010 Liu Z X,Shang C,Wang X Y,Wang D. 2017. Simulation on the amplification effect of a three-dimensional alluvial basin on the earthquake ground motion using the indirect boundary element method[J]. Acta Seismologica Sinica,39(1):111–131 (in Chinese).
杨永强,谢礼立,李明,胡进军. 2011. 汶川地震垂直和平行断层方向地震动特征差异[J]. 西南交通大学学报,46(2):235–240. doi: 10.3969/j.issn.0258-2724.2011.02.010 Yang Y Q,Xie L L,Li M,Hu J J. 2011. Differences between fault-normal and fault-parallel components of horizontal ground motion in Wenchuan earthquake[J]. Journal of Southwest Jiaotong University,46(2):235–240 (in Chinese).
Bradley B A,Wotherspoon L M,Kaiser A E. 2017. Ground motion and site effect observations in the Wellington region from the 2016 MW7.8 Kaikōura,New Zealand earthquake[J]. Bull N Z Soc Earthq Eng,50(2):94–105.
Furumura T,Koketsu K. 1998. Specific distribution of ground motion during the 1995 Kobe earthquake and its generation mechanism[J]. Geophys Res Lett,25(6):785–788. doi: 10.1029/98GL50418
Galvez P,Ampuero J P,Dalguer L A,Somala S N,Nissen-Meyer T. 2014. Dynamic earthquake rupture modelled with an unstructured 3-D spectral element method applied to the 2011 M9 Tohoku earthquake[J]. Geophys J Int,198(2):1222–1240. doi: 10.1093/gji/ggu203
Hanks T C,Kanamori H. 1979. A moment magnitude scale[J]. J Geophys Res:Solid Earth,84(B5):2348–2350. doi: 10.1029/JB084iB05p02348
He C H,Wang J T,Zhang C H. 2016. Nonlinear spectral-element method for 3D seismic-wave propagation[J]. Bull Seismol Soc Am,106(3):1074–1087. doi: 10.1785/0120150341
Komatitsch D,Tromp J. 1999. Introduction to the spectral element method for three-dimensional seismic wave propagation[J]. Geophys J Int,139(3):806–822. doi: 10.1046/j.1365-246x.1999.00967.x
Liu Y S,Teng J W,Lan H Q,Si X,Ma X Y. 2014. A comparative study of finite element and spectral element methods in seismic wavefield modeling[J]. Geophysics,79(2):T91–T104. doi: 10.1190/geo2013-0018.1
Miksat J,Wen K L,Wenzel F,Sokolov V,Chen C T. 2010. Numerical modelling of ground motion in the Taipei Basin:Basin and source effects[J]. Geophys J Int,183(3):1633–1647. doi: 10.1111/j.1365-246X.2010.04818.x
Olsen K B. 2000. Site amplification in the Los Angeles basin from three-dimensional modeling of ground motion[J]. Bull Seismol Soc Am,90(6B):S77–S94. doi: 10.1785/0120000506
Pitarka A,Irikura K,Iwata T,Sekiguchi H. 1998. Three-dimensional simulation of the near-fault ground motion for the 1995 Hyogo-Ken Nanbu (Kobe),Japan,earthquake[J]. Bull Seismol Soc Am,88(2):428–440. doi: 10.1785/BSSA0880020428
Somerville P,Irikura K,Graves R,Sawada S,Wald D,Abrahamson N,Iwasaki Y,Kagawa T,Smith N,Kowada A. 1999. Characterizing crustal earthquake slip models for the prediction of strong ground motion[J]. Seismol Res Lett,70(1):59–80. doi: 10.1785/gssrl.70.1.59
Computational Infrastructure for Geodynamics. 2012. SPECFEM3D cartesian[CP/OL]. [2021-03-12]. https://geodynamics.org/ cig/software/specfem3d/.
Wells D L,Coppersmith K J. 1994. New empirical relationships among magnitude,rupture length,rupture width,rupture area,and surface displacement[J]. Bull Seismol Soc Am,84(4):974–1002.
Wirth E A,Vidale J E,Frankel A D,Pratt T L,Marafi N A,Thompson M,Stephenson W J. 2019. Source-dependent amplification of earthquake ground motions in deep sedimentary basins[J]. Geophys Res Lett,46(12):6443–6450. doi: 10.1029/2019GL082474
Yu Y Y,Ding H P,Liu Q F. 2017. Three-dimensional simulations of strong ground motion in the Sichuan basin during the Wenchuan earthquake[J]. Bull Earthq Eng,15(11):4661–4679. doi: 10.1007/s10518-017-0154-2
Zhang W,Shen Y,Chen X F. 2008. Numerical simulation of strong ground motion for the MS8.0 Wenchuan earthquake of 12 May 2008[J]. Science in China: Series D,51(12):1673–1682. doi: 10.1007/s11430-008-0130-4
-
期刊类型引用(10)
1. 任雪梅，李文君，罗国富. 1970年以来“固原窗”M_L≥3.0地震活动增强现象及震兆意义. 华南地震. 2022(03): 58-64 . 
百度学术
2. 张琳琳，聂晓红. 2021年3月24日拜城M_S5.4地震前拜城地震窗异常分析. 内陆地震. 2022(04): 297-302 . 百度学术
3. 罗恒之，曾宪伟，卫定军，罗国富，司学芸，李新艳. 2017年内蒙古阿拉善左旗5.0级地震震前异常总结. 地震地磁观测与研究. 2020(05): 183-191 . 百度学术
4. 张琳琳，敖雪明. 基于Molchan模型的乌恰地震窗预测效能评价. 内陆地震. 2019(01): 8-13 . 百度学术
5. 钱蕊，王亮，张志宏，杨士超，夏彩韵. 辽宁海城地震窗开窗指标及效能分析. 防灾减灾学报. 2019(03): 84-91 . 百度学术
6. 张琳琳，敖雪明. 新疆天山地震窗口网的进一步研究. 内陆地震. 2018(01): 33-42 . 百度学术
7. 王霞，宋美琴. 大同窗地震活动频次和应变能特征. 中国地震. 2017(02): 328-337 . 百度学术
8. 张琳琳，敖雪明，聂晓红. 2017年精河6.6级、库车5.7级地震前“库米什地震窗”异常特征分析. 中国地震. 2017(04): 721-727 . 百度学术
9. Li Yutong，Zhang Bo，Wang Liang，Li Tongxia. The Precursory Significance of Cumulative Slip of Repeating Earthquake Sequences Prior to Moderately Strong Earthquakes— A Case Study of Four Remarkable Earthquake Sequences of HaichengXiuyan. Earthquake Research in China. 2016(01): 22-32 . 必应学术
10. 李宇彤，张博，王亮，李彤霞. 地震序列中重复地震的累积滑动量对后续中强地震的前兆意义——以辽宁海城-岫岩地区4个显著地震序列为例. 中国地震. 2015(02): 235-244 . 百度学术
其他类型引用(0)
计量
- 文章访问数: 483
- HTML全文浏览量: 149
- PDF下载量: 86
- 被引次数: 10
