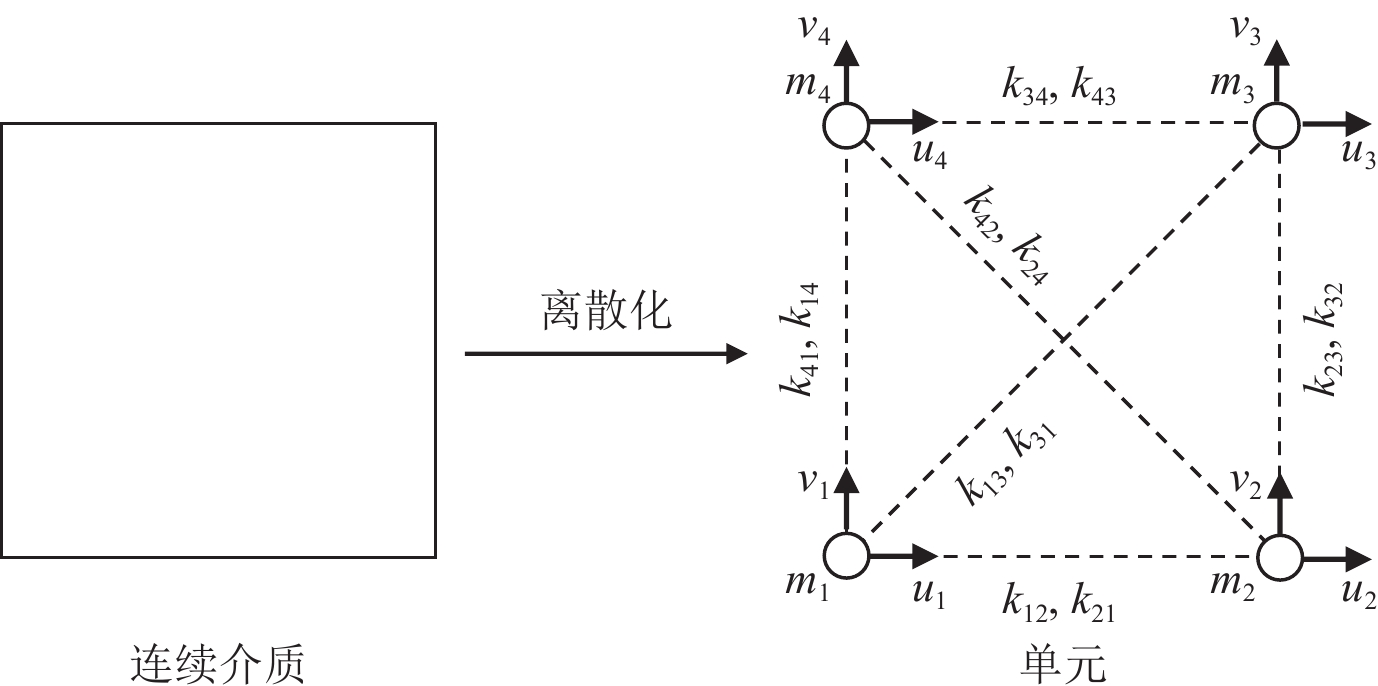
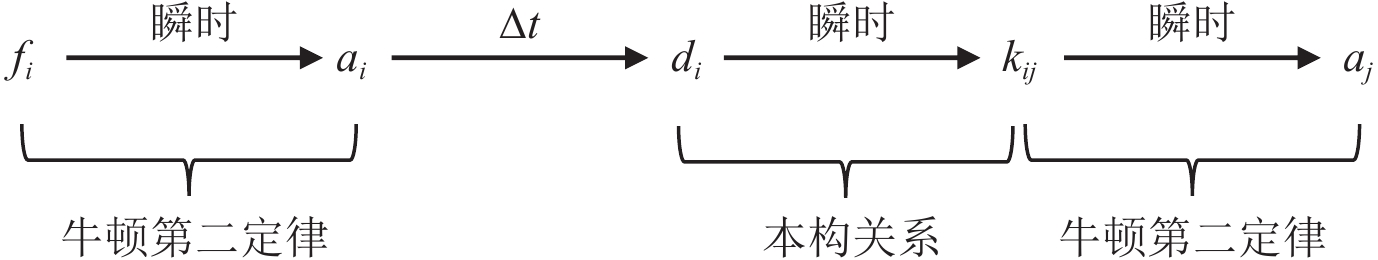
The mass property model and its implementation in the time-domain spectral element method
-
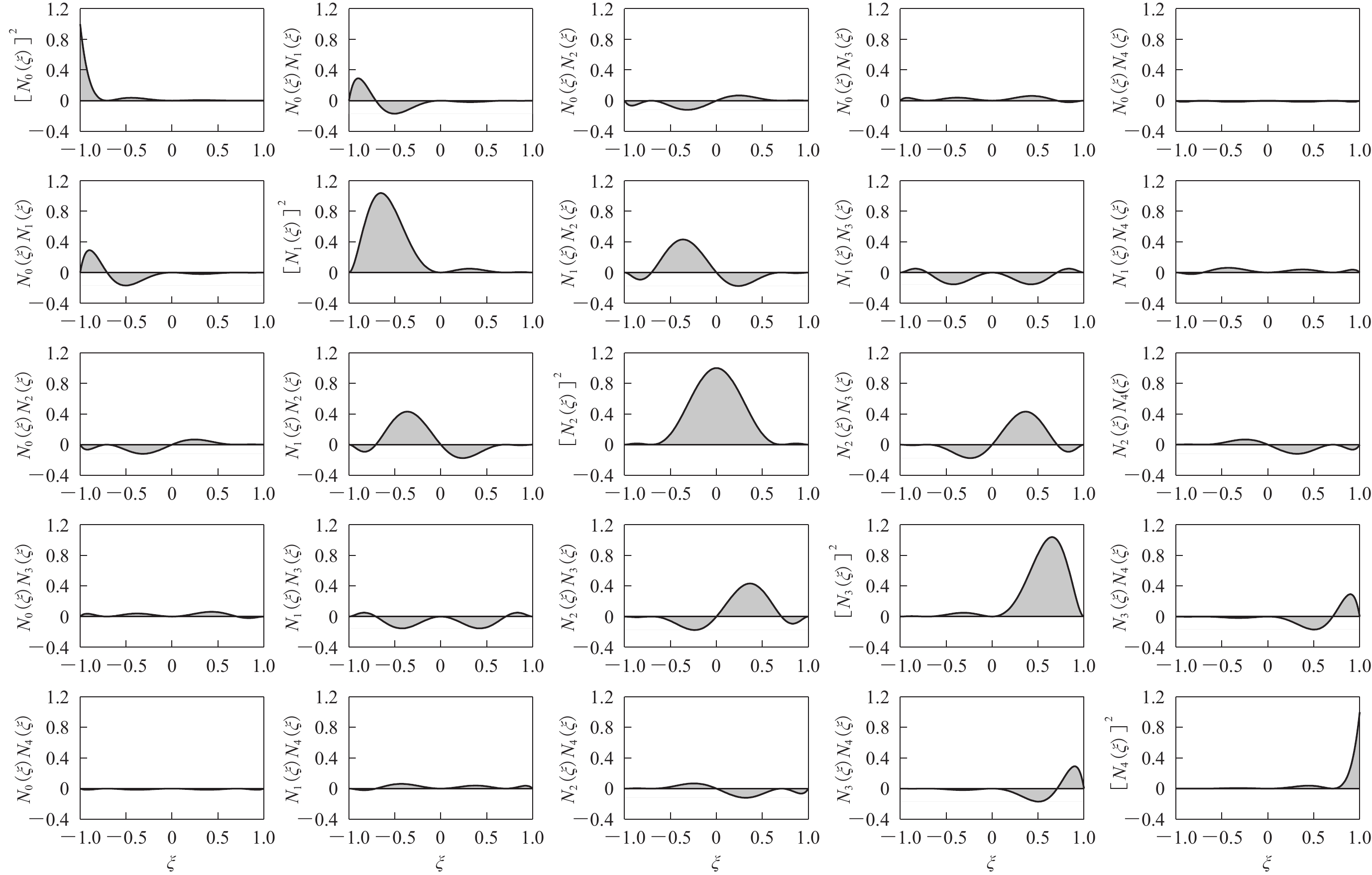
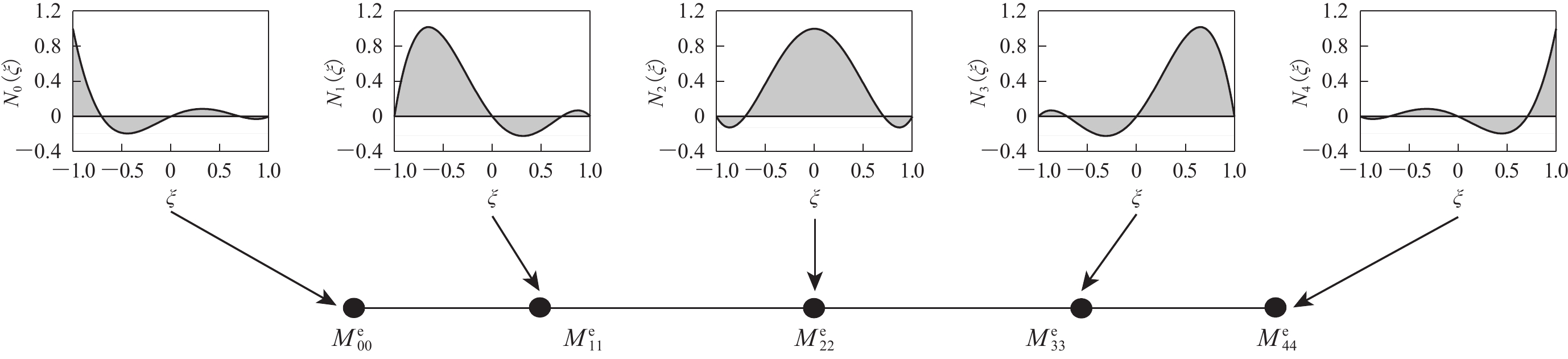
摘要: 研究了构建时域谱单元质量特性模型的数学机制，针对时域切比雪夫谱单元和勒让德谱单元建立了一种直接导出谱单元一致质量矩阵和集中质量矩阵的统一数学方法，对比分析两种谱单元质量特性模型的特征，并从物理角度探讨了谱单元质量特性模型的合理性。研究表明，数值积分点与谱单元节点选取是否一致是决定时域谱单元形成一致质量模型或集中质量模型的根本原因，当采用高斯-勒让德积分计算谱单元质量模型时将导出一致质量矩阵，而采用高斯-洛巴托积分则导出集中质量矩阵。而集中质量模型更具有物理合理性，两种谱单元质量特性模型优劣相当，均可取得很好的动力问题分析结果。Abstract: The mathematical mechanism of constructing mass property model for the time-domain spectral elements is studied in this paper. A unified mathematical method for directly deriving consistent and lumped mass matrix is established for the time-domain Chebyshev and Legendre spectral elements. The characteristics of two mass property models of the spectral elements are analyzed through comparison. Meanwhile, the rationality of mass property model of spectral element is discussed from physical perspective. This study reveals that the formation of consistent or lumped mass matrix in time-domain spectral elements depends on whether the quadrature points are coincident with the element nodes or not. To be specific, the Gauss-Legendre quadrature results in consistent mass matrix for spectral elements, and the Gauss-Lobatto quadrature leads to lumped mass matrix. The lumped mass matrix is more reasonable in physics. The two mass property models of spectral elements have comparable performance and they can both achieve good results for dynamic problems.
-
-
![]()
图 2 一维集中质量切比雪夫谱单元（左）和勒让德谱单元（右）质量分布特征
（a） 3节点单元；（b） 4节点单元；（c） 5节点单元
Figure 2. Mass distribution characteristics of 1-D lumped mass Chebyshev （left） and Legendre （right） spectral elements
（a） 3-node element；（b） 4-node element；（c） 5-node element
![]()
图 3 二维集中质量切比雪夫（左）和勒让德（右）谱单元质量分布特征
（a） 9节点单元；（b） 16节点单元；（c） 25节点单元
Figure 3. Mass distribution characteristics of 2-D lumped mass Chebyshev （left） and Legendre （right） spectral elements
（a） 9-node element；（b） 16-node element；（c） 25-node element
![]()
图 4 一维5节点切比雪夫谱单元一致质量模型数学图示
Figure 4. Mathematical representation for consistent mass model of 1-D 5-node Chebyshev element
![]()
图 5 一维5节点切比雪夫谱单元集中质量模型数学图示
Figure 5. Mathematical representation for lumped mass model of 1-D 5-node Chebyshev element
![]()
图 6 不同谱单元数量下简支梁的前6阶固有频率计算误差
Figure 6. Error of first six natural frequencies with different number of elements
![]()
图 9 离散化和积分过程引起的质量特性误差
Figure 9. Error in mass property caused by discretization and quadrature
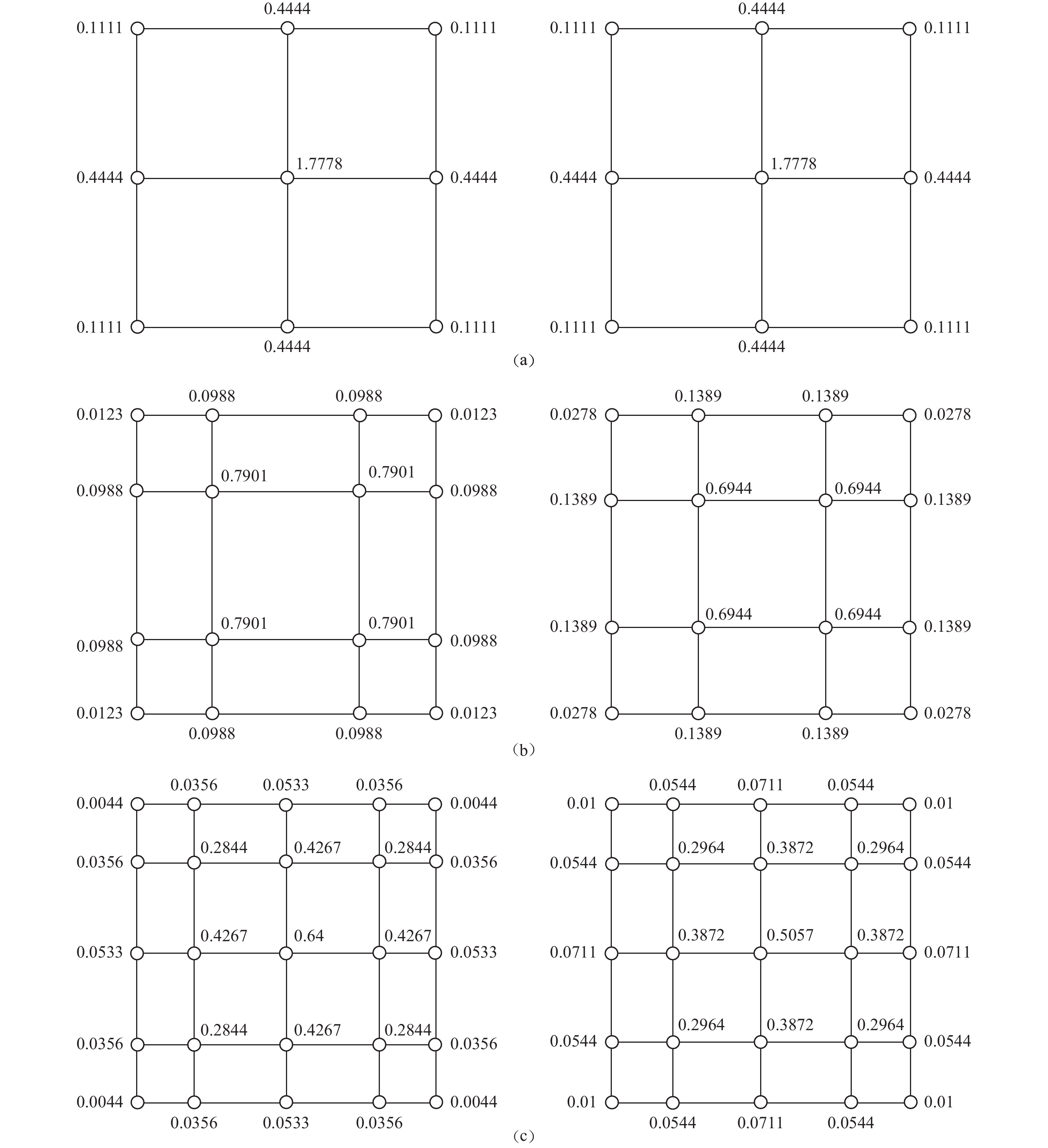
表 1 GLL节点坐标和积分权系数
Table 1 Abscissas of GLL points and quadrature weights
谱单元阶次 GLL节点坐标 积分权系数 1 （−1，1） 1，1 2 （−1，0，1） 0.333 333，1.333 333，0.333 333 3 （−1，−0.447 214，0.447 214，1） 0.166 667，0.833 333，0.833 333，0.166 667 4 （−1，−0.654 654，0，0.654 654，1） 0.1，0.544 444，0.711 111，0.544 444，0.1 5 （−1，−0.765 055，−0.285 232，
0.285 232，0.765 055，1）0.066 667，0.378 475，0.554 858，
0.554 858，0.378 475，0.066 667
下载: 导出CSV
表 2 GLC节点坐标和高斯-洛巴托积分权系数
Table 2 Abscissas of GLC points and Gauss-Lobatto quadrature weights
谱单元阶次 GLC节点坐标 积分权系数 1 （−1，1） 1，1 2 （−1，0，1） 0.333 333，1.333 333，0.333 333 3 （−1，−0.5，0.5，1） 0.111 111，0.888 889，0.888 889，0.111 111 4 （−1，−0.707 107，0，0.707 107，1） 0.066 667，0.533 333，0.8，0.533 333，0.066 667 5 （−1，−0.809 017，−0.309 017，
0.309 017，0.809 017，1）0.04，0.360 743，0.599 257，
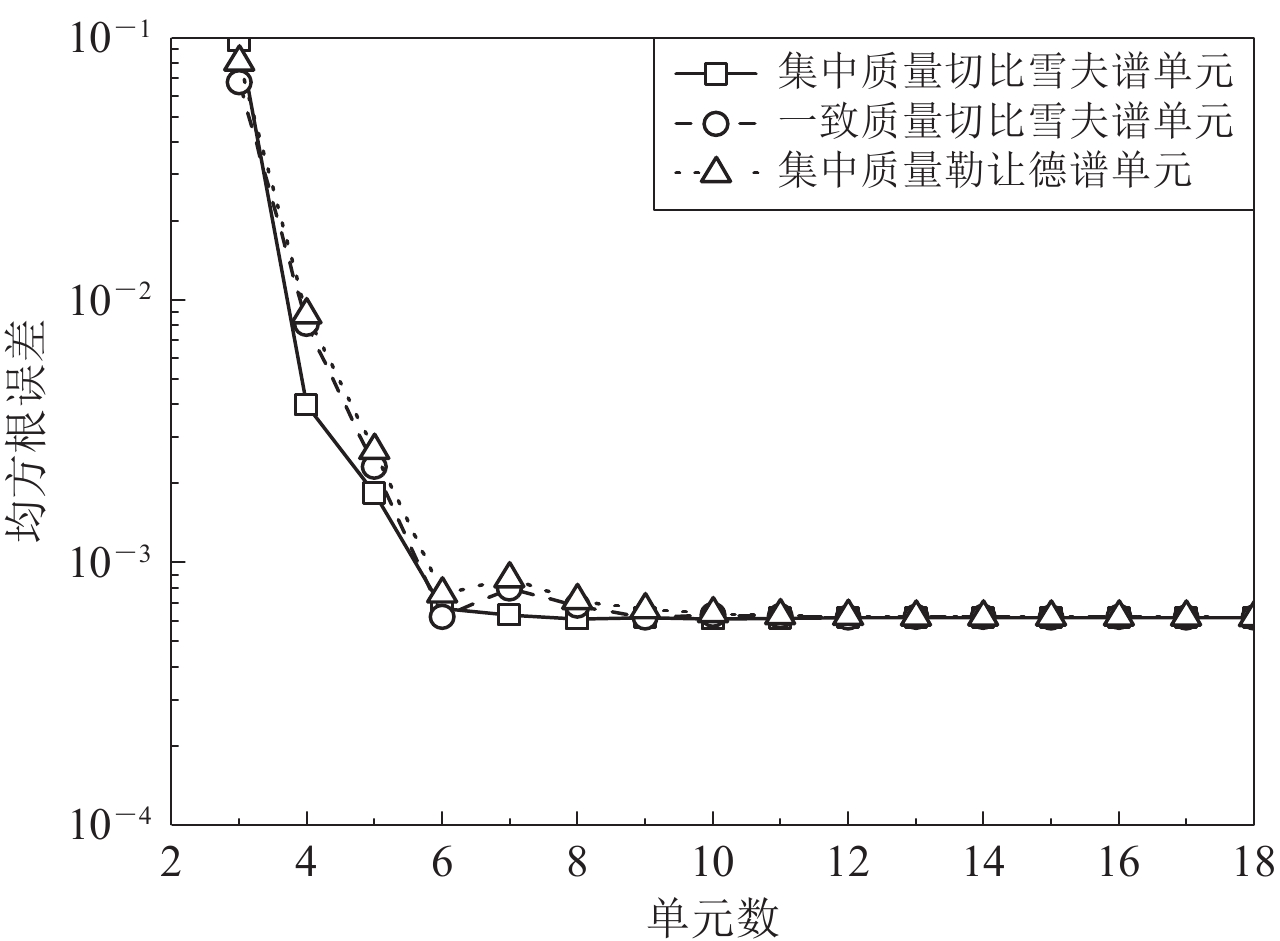
0.599 257，0.360 743，0.04
下载: 导出CSV
表 3 简支梁前6阶固有频率计算结果
Table 3 First six natural frequencies of simply supported beam
谱单元 单元阶次 固有频率 /Hz 1阶 2阶 3阶 4阶 5阶 6阶 切比雪夫
（集中质量）4 26.093 6 103.808 9 230.472 6 408.857 7 633.689 4 1 092.290 1 5 26.093 6 103.794 3 231.362 4 406.506 8 626.318 0 876.153 0 6 26.093 6 103.793 9 231.425 2 406.355 0 625.330 3 884.533 9 切比雪夫
（一致质量）4 26.093 7 103.818 0 231.485 4 410.983 5 647.442 7 1 025.303 2 5 26.093 6 103.794 3 231.453 3 406.625 7 627.393 8 887.768 5 6 26.093 6 103.793 9 231.418 6 406.356 5 625.379 6 886.020 8 勒让德
（集中质量）4 26.093 7 103.823 4 231.410 2 411.380 2 646.890 0 1 054.997 5 5 26.093 6 103.794 4 231.465 2 406.682 7 627.654 9 884.530 9 6 26.093 6 103.793 9 231.418 6 406.359 7 625.409 9 886.581 6 解析解 26.093 5 103.791 8 231.395 7 406.225 1 624.820 5 883.181 3
下载: 导出CSV
-
丁志华, 周红, 蒋涵. 2014. 三维台阶地形地震动效应研究[J]. 地震学报, 36（2）: 184-199 doi: 10.3969/j.issn.0253-3782.2014.02.004 Ding Z H, Zhou H, Jiang H. 2014. Effect of 3-D step topography on ground motion[J]. Acta Seismologica Sinica, 36（2）: 184-199 （in Chinese）. doi: 10.3969/j.issn.0253-3782.2014.02.004
韩天成, 于彦彦, 丁海平. 2020. 直下型断层的破裂速度对盆地地震效应的影响[J]. 地震学报, 42（4）: 457-470 doi: 10.11939/jass.20190177 Han T C, Yu Y Y, Ding H P. 2020. Influence of rupture velocity of the directly-beneath fault on the basin seismic effect[J]. Acta Seismologica Sinica, 42（4）: 457-470 （in Chinese）. doi: 10.11939/jass.20190177
廖振鹏. 2002. 工程波动理论导论[M]. 第2版. 北京: 科学出版社: 59–63 Liao Z P. 2002. Introduction to Wave Motion Theories in Engineering[M]. 2nd ed. Beijing: Science Press: 59–63 （in Chinese）.
王勖成. 2003. 有限单元法[M]. 北京: 清华大学出版社: 472–475 Wang X C. 2003. Finite Element Method[M]. Beijing: Tsinghua University Press: 472–475 （in Chinese）.
邢浩洁. 2017. 透射边界机理及其在地震波动谱元模拟中的应用[D]. 南京: 南京工业大学: 39–82 Xing H J. 2017. Mechanism of Transmitting Boundary and Its Application to Seismic Wave Simulation With Spectral Element Method[D]. Nanjing: Nanjing Tech University: 39–82 （in Chinese）.
Bathe K J. 2014. Finite Element Procedures[M]. 2nd ed. USA: Prentice-Hall: 476–480.
Bottero A, Cristini P, Komatitsch D, Asch M. 2016. An axisymmetric time-domain spectral-element method for full-wave simulations: Application to ocean acoustics[J]. J Acoust Soc Am, 140（5）: 3520-3530. doi: 10.1121/1.4965964
Chan H C, Cai C W, Cheung Y K. 1993. Convergence studies of dynamic analysis by using the finite element method with lumped mass matrix[J]. J Sound Vib, 165（2）: 193-207. doi: 10.1006/jsvi.1993.1253
Craig Jr R R, Kurdila A J. 2006. Fundamentals of Structural Dynamics[M]. 2nd ed. Hoboken, New Jersey: John Wiley & Sons: 400–401.
Cristini P, Komatitsch D. 2012. Some illustrative examples of the use of a spectral-element method in ocean acoustics[J]. J Acoust Soc Am, 131（3）: EL229-EL235. doi: 10.1121/1.3682459
Dauksher W, Emery A F. 1997. Accuracy in modeling the acoustic wave equation with Chebyshev spectral finite elements[J]. Finite Elem Anal Des, 26（2）: 115-128. doi: 10.1016/S0168-874X(96)00075-3
Dauksher W, Emery A F. 1999. An evaluation of the cost effectiveness of Chebyshev spectral and p-finite element solutions to the scalar wave equation[J]. Int J Numer Methods Eng, 45（8）: 1099-1113. doi: 10.1002/(SICI)1097-0207(19990720)45:8<1099::AID-NME622>3.0.CO;2-5
Dauksher W, Emery A F. 2000. The solution of elastostatic and elastodynamic problems with Chebyshev spectral finite elements[J]. Comput Methods Appl Mech Eng, 188（1/2/3）: 217-233.
Duczek S, Gravenkamp H. 2019a. Mass lumping techniques in the spectral element method: On the equivalence of the row-sum, nodal quadrature, and diagonal scaling methods[J]. Comput Methods Appl Mech Eng, 353: 516-569. doi: 10.1016/j.cma.2019.05.016
Duczek S, Gravenkamp H. 2019b. Critical assessment of different mass lumping schemes for higher order serendipity finite elements[J]. Comput Methods Appl Mech Eng, 350: 836-897. doi: 10.1016/j.cma.2019.03.028
Fried I, Malkus D S. 1975. Finite element mass matrix lumping by numerical integration with no convergence rate loss[J]. Int J Solids Struct, 11（4）: 461-466. doi: 10.1016/0020-7683(75)90081-5
Hinton E, Rock T, Zienkiewicz O C. 1976. A note on mass lumping and related processes in the finite element method[J]. Earthq Eng Struct Dyn, 4（3）: 245-249. doi: 10.1002/eqe.4290040305
Hughes T J R. 1987. The Finite Element Method: Linear Static and Dynamic Finite Element Analysis[M]. Englewood Cliffs, NJ: Prentice-Hall: 436−446.
Jensen M S. 1996. High convergence order finite elements with lumped mass matrix[J]. Int J Numer Methods Eng, 39（11）: 1879-1888. doi: 10.1002/(SICI)1097-0207(19960615)39:11<1879::AID-NME933>3.0.CO;2-2
Komatitsch D, Vilotte J P. 1998. The spectral element method: An efficient tool to simulate the seismic response of 2D and 3D geological structures[J]. Bull Seismol Soc Am, 88（2）: 368-392.
Komatitsch D, Tromp J. 1999. Introduction to the spectral element method for three-dimensional seismic wave propagation[J]. Geophys J Int, 139（3）: 806-822. doi: 10.1046/j.1365-246x.1999.00967.x
Kudela P, Krawczuk M, Ostachowicz W. 2007a. Wave propagation modelling in 1D structures using spectral finite elements[J]. J Sound Vib, 300（1/2）: 88-100.
Kudela P, Żak A, Krawczuk M, Ostachowicz W. 2007b. Modelling of wave propagation in composite plates using the time domain spectral element method[J]. J Sound Vib, 302（4/5）: 728-745.
Patera A T. 1984. A spectral element method for fluid dynamics: Laminar flow in a channel expansion[J]. J Comput Phys, 54（3）: 468-488. doi: 10.1016/0021-9991(84)90128-1
Priolo E, Carcione J M, Seriani G. 1994. Numerical simulation of interface waves by high-order spectral modeling techniques[J]. J Acoust Soc Am, 95（2）: 681-693. doi: 10.1121/1.408428
Tong P, Pian T H H, Bucciarblli L L. 1971. Mode shapes and frequencies by finite element method using consistent and lumped masses[J]. Comput Struct, 1（4）: 623-638. doi: 10.1016/0045-7949(71)90033-2
Wu S R. 2006. Lumped mass matrix in explicit finite element method for transient dynamics of elasticity[J]. Comput Methods Appl Mech Eng, 195（44/47）: 5983-5994.
Yang Y T, Zheng H, Sivaselvan M V. 2017. A rigorous and unified mass lumping scheme for higher-order elements[J]. Comput Methods Appl Mech Eng, 319: 491-514. doi: 10.1016/j.cma.2017.03.011
Żak A. 2009. A novel formulation of a spectral plate element for wave propagation in isotropic structures[J]. Finite Elem Anal Des, 45（10）: 650-658. doi: 10.1016/j.finel.2009.05.002
Żak A, Krawczuk M, Palacz M, Doliński Ł, Waszkowiak W. 2017. High frequency dynamics of an isotropic Timoshenko periodic beam by the use of the Time-domain Spectral Finite Element Method[J]. J Sound Vib, 409: 318-335. doi: 10.1016/j.jsv.2017.07.055
Żak A, Krawczuk M. 2018. A higher order transversely deformable shell-type spectral finite element for dynamic analysis of isotropic structures[J]. Finite Elem Anal Des, 142: 17-29. doi: 10.1016/j.finel.2017.12.007
Zhang G H, Yang Y T, Zheng H. 2019a. A mass lumpig scheme for the second-order numerical manifold method[J]. Comput Struct, 213: 23-39. doi: 10.1016/j.compstruc.2018.12.005
Zhang G H, Yang Y T, Sun G H, Zheng H. 2019b. A mass lumping scheme for the 10-node tetrahedral element[J]. Eng Anal Bound Elem, 106: 190-200. doi: 10.1016/j.enganabound.2019.04.018
Zheng H, Yang Y T. 2017. On generation of lumped mass matrices in partition of unity based methods[J]. Int J Numer Methods Eng, 112（8）: 1040-1069. doi: 10.1002/nme.5544
Zhu C Y, Qin G L, Zhang J Z. 2011. Implicit Chebyshev spectral element method for acoustics wave equations[J]. Finite Elem Anal Des, 47（2）: 184-194. doi: 10.1016/j.finel.2010.09.004
Zienkiewicz O C, Taylor R L, Zhu J Z. 2013. The Finite Element Method: Its Basis and Fundamentals[M]. 7th ed. London: Elsevier: 383–386.
计量
- 文章访问数: 375
- HTML全文浏览量: 200
- PDF下载量: 41
