Prediction model of seismic amplification effect in sedimentary valley based on differential evolution-artificial neural network
-
摘要: 探讨了基于差分进化-人工神经网络构建沉积河谷地震响应代理模型的可行性。首先建立沉积河谷对地震波散射的求解方法，以半圆形、V形沉积河谷为例，以入射波条件、沉积内外介质属性、场地形状为特征参数，以沉积河谷地震动放大系数为预测目标参数，构建数据集；其次，建立沉积河谷地震动放大效应人工神经网络、差分进化-人工神经网络算法预测模型，对比两种算法计算精度和稳定性，并进行了特征参数敏感性分析。结果表明：人工神经网络能较好地预测沉积河谷地震动放大效应，使差分进化-人工神经网络预测模型的精度和稳定性显著提高；入射波频率是影响沉积河谷地震动放大系数的主要原因，沉积内外介质密度比的影响较小。本研究结论可对地震作用下更为复杂的局部场地效应预测和评估提供参考。Abstract: Sedimentary valley has obvious amplification effect on ground motions, which has an increase on the engineering damage. However, the propagation mechanism of seismic wave in sedimentary valley is complex, resulting in high nonlinearity and high coupling of influences of incident wave and site parameters on seismic amplification effect. First, based on the boundary element method, the scattering of seismic waves by sedimentary valley is solved. Prediction models of seismic amplification effect of semicircular and V-shaped sedimentary valley are established, with incident wave conditions, material properties and valley shapes as characteristic parameters and the seismic amplification factor of sedimentary valley as target parameters, and the dataset is constructed; Second, the calculation accuracy and stability of artificial neural network （ANN） and its optimization algorithm, i.e., differential evolution, are compared, and the sensitivity of characteristic parameters is analyzed. The results show that the ANN can predict the amplification effect of sedimentary valley, and the accuracy and stability of differential evolution-ANN prediction model are significantly improved; The incident wave frequency is the main influence factor of the seismic amplification coefficient of sedimentary valley, and the density ratio of internal and external medium has little effect. The conclusions can provide references for more complex local site effect prediction and assessment.
-
引言
非火山型微震颤（non-volcanic tremor，简写为NVT）是位于板块隐没、远离火山地区的一种类似火山地区微震颤的地震活动。该类活动首次在日本西南部被发现，不同于一般的地震活动，NVT信号没有明显的体波到时；振幅小；能量频段集中在1—10 Hz，较普通地震低；活动持续时间较长，约数分钟至数天甚或数周。大地测量学证据表明非火山型微震颤常伴随着缓慢的板块滑移事件（Obara，2002；Rogers，Dragert，2003）。全球许多地方均观测到了NVT信号，例如卡斯凯迪亚断层区（Ide，2012）、圣安得烈斯断层区（Nadeau，Dolenc，2005；Shelly，2010）、中国台湾（Peng，Chao，2008；Tang et al，2010）、阿拉斯加（Peterson，Christensen，2009）、智利南部及新西兰（Fry et al，2011；Kim et al，2011）等地区。常规地震通常发生在上地壳脆性带，而NVT通常发生在下地壳至莫霍面之间的区域，因此观测NVT信号成为监测下地壳破裂及研究变形机理的一种方法（Daub et al，2011；Chao et al，2012）。
Peng和Chao （2008）首次在台湾观察到NVT事件，此次事件由2001年11月14日我国青海省昆仑山口西MS8.1地震所触发，NVT震源位于中央山脉深处的滑脱断层上，深度约20 km；出现信号的时间点与远场地震的勒夫波到时几乎一致，因此此次事件被认为是由昆仑山口西地震勒夫波产生的动态应力触发。Tang等（2010）分析得出中央山脉南部的部分NVT信号由一些P波和S波信号较微弱的低频地震（low-frequency earthquakes，缩写为LFEs）组成，这与观测到的位于其它地区板块边界的NVT非常相似。Chao等（2012）分析了1998—2009年45次MW≥7.5的远场地震事件，其中有9次触发了NVT事件，其定位结果显示，这9次NVT事件的震源位于台湾中央山脉的南段，震源深度介于15—25 km之间，分析认为远场地震面波的振幅大小是触发的关键条件，并得出触发NVT的动态应力阈值为7—8 kPa。由于Chao等（2012）主要针对切向分量的分析，确定的触发阀值不够全面，因此，本文拟针对波形的三分量进行综合考量，以确定更全面的触发阈值。
虽然NVT已在全球很多地区被观测到，但对于不同构造环境中NVT的活动特性尚未明确。因此，本文选择对NVT事件有一定先验条件、区内构造复杂、台站分布密集、地震活动活跃的我国台湾地区为研究区，讨论NVT触发所需的条件和潜在机理，并对其空间分布特征予以分析，以期了解NVT震源附近的孕震构造，为地震学的进一步研究提供更多的参考。
1. 研究区域概况与研究方法
1.1 研究区域概况
中国台湾地处地球上板块运动最活跃的区域—环太平洋构造带上，位于欧亚大陆板块与菲律宾海板块的碰撞边界。古生代以来，台湾地区就位于华夏古陆的边缘，也因如此，长期受板块作用的影响远大于板块内部环境的影响。中新世至今，菲律宾海板块每年以7—8 cm的速度向西北方向移动（Yu et al，1997），在两个板块相互作用之下，菲律宾海板块向北隐没于欧亚大陆板块之下（Teng，1996），而南中国海板块则向东俯冲于菲律宾海板块之下（Chai，1972）（图1b）。台湾岛位在两个俯冲带之间，两个俯冲带与左移兼具逆冲的花东纵谷断层相连接。复杂的构造环境导致了台湾地区的地震活动频繁（Wu et al，2007）。本文在台湾南部地区（21°30'N—24°00'N，120°00'E—121°30'E）展开研究，研究区内台站和由4次远震触发的NVT事件震中和震源深度分布如图1所示。
![]()
图 1 研究区域的构造背景及台站和非火山型微震颤（NVT）事件分布（a） 研究区台站和NVT震中分布；（b） 区域构造背景；（c） 触发NVT的4次地震位置示意图；（d） AA′ 剖面上的NVT事件的震源深度分布Figure 1. Tectonic settings and distribution of stations and non-volcanic tremor （NVT） locations（a） Distribution of seismic stations and locations of NVTs；（b） Regional tectonic settings；（c） Schematic diagram for location of four earthquakes that triggered NVT；（d） Distribution of NVT depths along the cross-section AA′1.2 数据与方法
本研究首先从全球地震矩张量解（global centroid-moment-tensor，缩写为GCMT）目录中选取2011年1月1日至2016年12月31日的38次距离台湾岛大于1 000 km且MW≥7.5的地震事件。采用台湾宽频地震观测网（Broadband Array in Taiwan for Seismology，缩写为BATS）、台湾气象局（Weather Bureau Seismic Network，缩写为WBSN）及美国地震学研究联合会（Incorporated Research Institutions for Seismology，缩写为IRIS）发布的地震发生后3小时内的连续波形资料进行分析，波形数据的采样率为20 Hz或100 Hz。
参考Peng和Chao （2008）一文中的方法，去除仪器响应后，对波形进行频谱分析，如图2所示。在远震信号中，频率最高的能量通常来自P波，若在频谱分析过程中面波经过的时段内存在比P波频率高的信号，则可以初步认定此信号为区域地震信号。由于受波形数据采样率限制，参考Chao等（2012）的信号处理流程，本文统一对波形进行2—8 Hz带通滤波，若5个以上台站均接收到高频、无脉冲且持续时段大于100 s的连续波包信号时，即可认定为NVT事件发生。
![]()
图 2 2011年东日本MW9.1地震波形频谱分析图（a） TPUB台站记录到的地震原始波形；（b） 2—8 Hz滤波后波形；（c） 频谱图Figure 2. The spectrogram of Tohoku earthquake on 11 March 2011（a） Broad-band seismogram recorded at station TPUB；（b） 2−8 Hz bandpass-filtered seismogram； （c） The spectrogram of seismogram at station TPUB检测到NVT信号后，计算每个信号的包络函数，并使用包络函数互相关法进行定位（Obara，2002；Peng，Chao，2008；Chao et al，2013）。此方法的原理是将台站对之间的观测走时差与理论走时差进行比较，以网格搜寻的方式将所有台站对的走时残差均方根（root mean square，缩写为RMS）最小的位置确定为震源位置，本文中4次NVT事件的定位结果如图3所示。假设使用N个台站的包络函数进行定位，则有ni， j＝N（N－1）/2个台站对，网格点（经度x，纬度y，深度z）的均方差如下：
![]()
图 3 4次NVT事件的定位示意图（a） 东日本MW9.1地震触发事件；（b） 苏门答腊MW8.6地震触发事件；（c） 所罗门群岛MW7.6地震触发事件；（d） 尼泊尔MW7.9地震触发事件Figure 3. The schematic diagrams of locating four NVT events（a） NVT triggered by MW9.1 Tohoku earthquake；（b） NVT triggered by MW8.6 Sumatra earthauake； （c） NVT triggered by MW7.6 Solomon earthquake；（d） NVT triggered by MW7.9 Nepal earthquake${\rm{RM}}{{\rm{S}}_{\left( {x{\text{，}}\!\!\!\! y{\text{，}}\!\!\!\!z} \right)}} {\text{＝}} \sqrt {\frac{{\displaystyle\mathop \sum \limits_{i {\text{＝}} 1,j {\text{＝}} 2}^n {{(\Delta {T_{i,j}})}^2}}}{n}} {\text{＝}} \sqrt {\frac{{\displaystyle\mathop \sum \limits_{i {\text{＝}} 1,j {\text{＝}} 2}^n {{\left( {\Delta t_{i{\text{，}}\!\!\!\!j}^{{\rm{theo}}} - \Delta t_{i{\text{，}}\!\!\!\!j}^{{\rm{obs}}}} \right)}^2}}}{n}}{\text{，}} $
(1) This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
表 1 台湾地区一维速度模型（Wu et al，2007）Table 1. 1-D velocity model in Taiwan region （Wu et al，2007）vP/（km·s−1） vP/vS vS/km·s−1 深度范围/km vP/（km·s−1） vP/vS vS/km·s−1 深度范围/km 3.82 1.74 2.20 0—2 6.13 1.75 3.50 21—25 4.93 1.68 2.93 2—4 6.25 1.71 3.65 25—30 5.51 1.70 3.24 4—6 6.54 1.73 3.78 30—35 5.63 1.70 3.31 6—9 7.03 1.75 4.02 35—50 5.83 1.76 3.31 9—13 8.00 1.74 4.60 50—70 5.99 1.75 3.42 13—17 8.34 1.73 4.82 70—80 6.06 1.75 3.46 17—21 This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
2. 远场大规模地震触发的NVT事件
在38次远场大规模地震中，触发研究区内NVT事件的4次地震分别为：2011年3月11日东日本MW9.1地震，2012年4月11日苏门答腊岛MW8.6地震，2014年4月12日所罗门群岛MW7.6地震和2015年4月25日尼泊尔MW7.9地震，这4次地震的相对发震位置如图1c所示，具体信息列于表2。
表 2 触发NVT的四次远震事件信息概要Table 2. General information of the four NVT-triggered teleseismic events发震时间 地点 震中位置 震源深度/km MW 震中距/° TPUB台站
反方位角/°年−月−日 时：分：秒 北纬/° 东经/° 2011−03−11 5：47：32.8 日本本州岛东岸近海 37.52 143.05 20.0 9.1 23.893 48 2012−04−11 8：39：31.4 苏门答腊岛北部西岸远海 2.35 92.82 45.6 8.6 34.049 236 2014−04−12 20：14：49.9 所罗门群岛 11.35 162.24 27.3 7.6 53.327 126 2015−04−25 6：11：58.6 尼泊尔 27.91 85.33 12.0 7.9 32.092 286 注：表中震中距是以TPUB台站为标准计算得到的。 2.1 2011年3月11日东日本MW9.1地震触发的NVT事件
2011年3月11日东日本地震距研究区2 652 km，勒夫波理论到时为647 s，瑞雷波理论到时为758 s。此次地震在4次触发NVT的地震事件中震中距最小、震级最大，而且在全球很多地区均触发了浅层微地震和NVT事件（Miyazawa，2011；Gonzalez-Huizar et al，2012；Hill et al，2013；Peng et al，2013），在日本四国岛、美国阿留申岛、美国阿拉斯加、美国卡斯凯迪亚、美国加州中部的帕克菲尔德、美国加州南部的圣哈辛托、新西兰北岛等地区均观测到NVT信号（Chao et al，2013）。而台湾地区观测到该地震触发的较为明显的NVT信号从震后650 s左右开始，持续了约300 s （图4a）。通过对明显NVT的出现时间及面波理论到时（图4b）的对比，推断本次NVT事件由东日本MW9.1地震的勒夫波触发，触发的NVT震中位置为（23.05°N，120.90°E）。
![]()
图 4 2011年3月11日东日本大地震触发的NVT事件波形图（a） 各台站南北向分量2—8 Hz带通滤波后的波形图，波形左侧为台站名及其距NVT震源距离；（b） 仪器校正后距离NVT震源最近台站的径向、垂向、切向的波形及滤波后波形，红色、黑色箭头分别指勒夫波（4.1 km/s）和瑞雷波（3.5 km/s）理论到时，下同Figure 4. Tremor triggered by the Tohoku earthquake on 11 March 2011（a） The 2−8 Hz bandpass-filtered seismograms of N-S component， The hypocentral distance between each station and tremor source is shown next to the station name on left of each trace；（b） The radial，vertical and transverse component seismograms，in which instrument response has been removed，recorded at the nearest station and bandpass-filtered seismograms The red and black vertical arrows indicate the predicted arrivals of the Love and Rayleigh waves with the apparent velocity of 4.1 and 3.5 km/s，respectively，the same below2.2 2012年4月11日苏门答腊岛MW8.6地震触发的NVT事件
2012年4月11日印度尼西亚苏门答腊岛北部西岸远海发生MW8.6地震，距本文研究区约3 779 km，勒夫波与瑞雷波理论到时分别为922 s和1 080 s。该地震在日本多个位置触发了NVT事件，这些震源位于不同的断层系统中，如：日本九州岛西部的走滑断层、日本关东的逆冲断层、琉球群岛西南部的俯冲带区域等（Chao，Obara，2016）。由图5可见：南北分量原始波形经过2—8 Hz带通滤波后可以看到NVT信号，从1 150 s左右开始，持续了大约500 s，被YULB，FULB，TWGB，ALSB和TPUB等5个台站记录到；而一些微弱的NVT信号在920 s左右出现，被YULB，FULB，TWGB和ALSB等4个台站记录到。使用网格搜寻的方法对此次事件进行定位，结果显示其震中位置为（23.05°N，120.90°E）。
![]()
图 5 2012年4月11日苏门答腊岛地震触发NVT事件波形图（a） 各台站南北向分量2—8 Hz带通滤波后的波形图；（b） 仪器校正后距离NVT震源最近台站的径向、垂向、切向的波形及滤波后波形Figure 5. Tremor triggered by the Sumatra earthquake occurred on 11 April 2012（a） The 2−8 Hz bandpass-filtered seismograms of N-S component；（b） The radial，vertical and transverse component seismograms，in which instrument response has been removed，recorded at the nearest station and bandpass-filtered seismograms2.3 2014年4月12日所罗门群岛MW7.6地震触发的NVT事件
2014年4月12日所罗门群岛MW7.6地震，距本文研究区5 913 km，勒夫波理论到时为1 444 s，瑞雷波理论到时为1 691 s。与其它3次触发NVT的地震事件相比，此次地震的震中距最大，震级最小。由图6波形可以看出，明显NVT信号从1 450 s左右开始，持续了大约450 s。此NVT事件的震中位置为（23.05°N，121.00°E）。
![]()
图 6 2014年4月12日所罗门群岛地震触发的NVT事件波形图（a） 各台站南北向分量2—8 Hz带通滤波后的波形图；（b） 仪器校正后距离NVT震源最近台站的径向、垂向、切向的波形及滤波后波形Figure 6. Tremor triggered by the Solomon earthquake on 12 April 2014（a） The 2−8 Hz bandpass-filtered seismograms of N-S component；（b） The radial，vertical and transverse component seismograms，in which instrument response has been removed，recorded at the nearest station and bandpass-filtered seismograms2.4 2015年4月25日 尼泊尔MW7.9地震触发的NVT事件
2015年4月25日尼泊尔MW7.9大地震，距本文研究区3 562 km，勒夫波理论到时为869 s，瑞雷波理论到时为1 018 s。此次地震与其它3次地震事件相比，其震源深度最浅，为12 km，且其在重庆綦江触发了ML3.8地震（Han et al，2017）。明显的NVT信号从1 150 s左右开始，持续了大约420 s，一些微弱的NVT信号在900 s左右出现，被SYNB，TPUB，TWGB和FULB等4个台站记录到（图7），推断本次事件由尼泊尔地震的勒夫波触发，其震中位置为（23.15°N，120.90°E）。
![]()
图 7 2015年4月25日尼泊尔地震触发的NVT事件波形图（a） 各台站南北向分量2—8 Hz带通滤波后的波形图；（b） 仪器校正后距离NVT震源最近台站的径向、垂向、切向的波形及滤波后波形Figure 7. Tremor triggered by the Nepal earthquake on 25 April 2015（a） The 2−8 Hz bandpass-filtered seismograms of N-S component；（b） The radial，vertical and transverse component seismograms，in which instrument response has been removed，recorded at the nearest station and bandpass-filtered seismograms3. 讨论与结论
3.1 NVT位置
本研究中，4次远场大地震在台湾南部触发了明显的NVT事件，震源位于中央山脉南段，潮州—梨山断层与花东纵谷之间，即欧亚大陆板块与菲律宾海板块交界处，深度分布在21—53 km。Peng和Chao （2008）以及Tang等（2010）定位到NVT震中在TPUB台站与TWGB台站之间，震源深度范围为15—25 km，本研究结果与其相比，震中位置大致相同，震源深度较深。Peng和Gomberg （2010）研究表明，NVT的出现与断层的蠕滑行为有密切关联，而蠕滑产生的重要因素则是液体的存在。中央山脉南段深部区域（15—50 km）的地下介质品质因子Q值较周边地区低（陈光荣，1993），电导率较周边地区高（Chen，Chen，1998），且液体孔隙压力较高（Lee et al，2010），表明该地区流体含量较周边地区大，导致断层面的耦合度降低，在受到来自面波的瞬时动态应力扰动时产生蠕滑（Chao et al，2012；Tang et al，2010），进而产生NVT信号。此外，NVT震源区域几乎无地震发生（图1a），而在此区域之内发生的地震震源机制多以正断层为主（Kao，Jian，2001；Hsu et al，2010），因此推测NVT发生在拉张型的地质环境中。
3.2 触发NVT的地震特征
为了检测动态应力是否为触发NVT的主要因素，本文利用PGV计算了38次远震的切向、垂向与径向动态应力，结果如图8所示，可见：切向、垂向和径向的动态应力范围分别为0.207—33.6 kPa，0.279—50.5 kPa，0.257—38.8 kPa；除了所罗门群岛MW7.6地震，其余3次触发NVT的地震动态应力均高于未触发地震；如图8c所示，在尼泊尔MW7.9地震附近的灰色正方形代表东日本MW9.1地震的一次MW7.9余震，其后十分钟左右又有一次MW7.6余震，余震MW7.9可能触发NVT，但由于其面波受到后面发生地震的干扰，无法辨别出NVT事件。而所罗门群岛MW7.6地震的震中距较其它3次地震大，且震级最小，这使得其地震波传播到研究区时产生的地表运动速度较小，动态应力值未超过14 kPa，但也触发了NVT事件。Chao等（2012）提出切向分量的触发阈值为7—8 kPa，本研究中仅所罗门群岛地震的阈值不满足此条件。除此之外，我们还计算了波形资料的信噪比，结果显示信噪比结果并无规律可循，不能利用其对是否触发NVT事件进行有效区分。
![]()
图 8 TPUB台站记录到的大地震径向 （a）、垂向 （b） 和切向 （c） 分量的PGV、动态应力及信噪比分布图背景噪声分析时窗长度为远震事件发震前600 sFigure 8. The scattergram of PGV，dynamic stress and signal-to-noise ratio for the radial （a），vertical （b） and tangential （c） components of the earthquakes recorded by the station TPUBThe ambient noise level of each event is calculated from a 600 s time window before the occurrence of each main shock通过NVT出现时间和面波理论到时的对比，分析得出本文研究的4次远震触发NVT事件均由勒夫波触发，但在瑞雷波到达后振幅增大，这与Chao等（2012）的研究结果有差异。该研究认为当远震面波垂直于中央山脉走向（N16°E）时，勒夫波触发NVT，但除了垂直中央山脉走向入射的尼泊尔地震的勒夫波触发NVT以外，其余3次地震的勒夫波到达时均有NVT信号出现，故推断勒夫波到达时NVT事件被触发，随后瑞雷波使NVT震源振动幅度增大，起到了调节的作用。
3.3 结论
本研究对2011年至2016年间发生的38次MW≥7.5地震（震中距离台湾大于1 000 km）在台湾南部触发的NVT行为进行了调查，结果显示共有4次远震在台湾南部触发了NVT事件，主要频率范围为2—8 Hz，持续时间为300 s至500 s不等，震中位置位于台湾中央山脉南段，深度范围为21—53 km，震源深度的定位结果仍有较大的误差，希望日后能够改进定位方法，得到更加精确的深部位置。本文还讨论了触发NVT事件的远震特征，包括远震产生的径向、垂向和切向动态应力，其中：东日本MW9.1地震、苏门答腊岛MW8.6地震、尼泊尔MW7.9地震产生的动态应力均大于14 kPa，其它未触发NVT事件的地震产生的应力均小于该值；所罗门群岛MW7.6地震产生的动态应力较小，但也触发了NVT事件，究其原因还需今后对大地震的触发行为进行统计，同时需要对远震进行更多空间域及频率域的讨论。
台湾宽频地震观测网、台湾气象局和美国地震学研究联合会为本文提供了地震目录和地震波形资料，审稿专家对本文提出了宝贵建议，作者在此一并表示感谢。
-
![]()
图 3 本文地表位移放大系数结果与文献结果对比
Figure 3. Comparison of amplification coefficient of surface displacement between the results in this paper and those in literature
![]()
图 4 半圆形河谷（a）和V形河谷（b）的预测模型点位分布
Figure 4. Location distribution of prediction model for semicircular valley （a） and V-shaped valley （b）
![]()
图 6 人工神经网络（左）和差分进化-人工神经网络（右）预测模型测试集决定系数
（a） 半圆形河谷； （b） V形河谷
Figure 6. Artificial neural network （ANN） （left） and differentical evolution-ANN （right） determination coefficient of test set of prediction model
（a） Semicircular valley； （b） V-shaped valley
![]()
图 7 采用边界元法和神经网络预测模型得到的半圆形河谷地表点A−E （a−e）的位移幅值对比
Figure 7. Comparison of displacement amplitudes between boundary element method and neural network prediction model of surface points A−E （a−e） for semicircular valley
![]()
图 8 采用边界元法和神经网络预测模型得到的V形河谷地表点A−E （a−e） 的位移幅值对比
Figure 8. Comparison of displacement amplitudes between boundary element method and neural network prediction model of surface points A−E （a−e） for V-shaped valley
![]()
图 9 基于差分进化-神经网络预测模型的半圆形河谷（a）和V型河谷（b） 地表位移放大系数频谱
Figure 9. Surface displacement amplification factor spectrum based on differential evolution-ANN prediction model for semicircular valley （a） and V-shaped valley （b）
![]()
图 10 基于边界元法的半圆形河谷 （a）和V型河谷（b）地表位移放大系数频谱
Figure 10. Surface displacement amplification factor spectrum based on boundary element method for semicircular valley （a） and V-shaped valley （b）
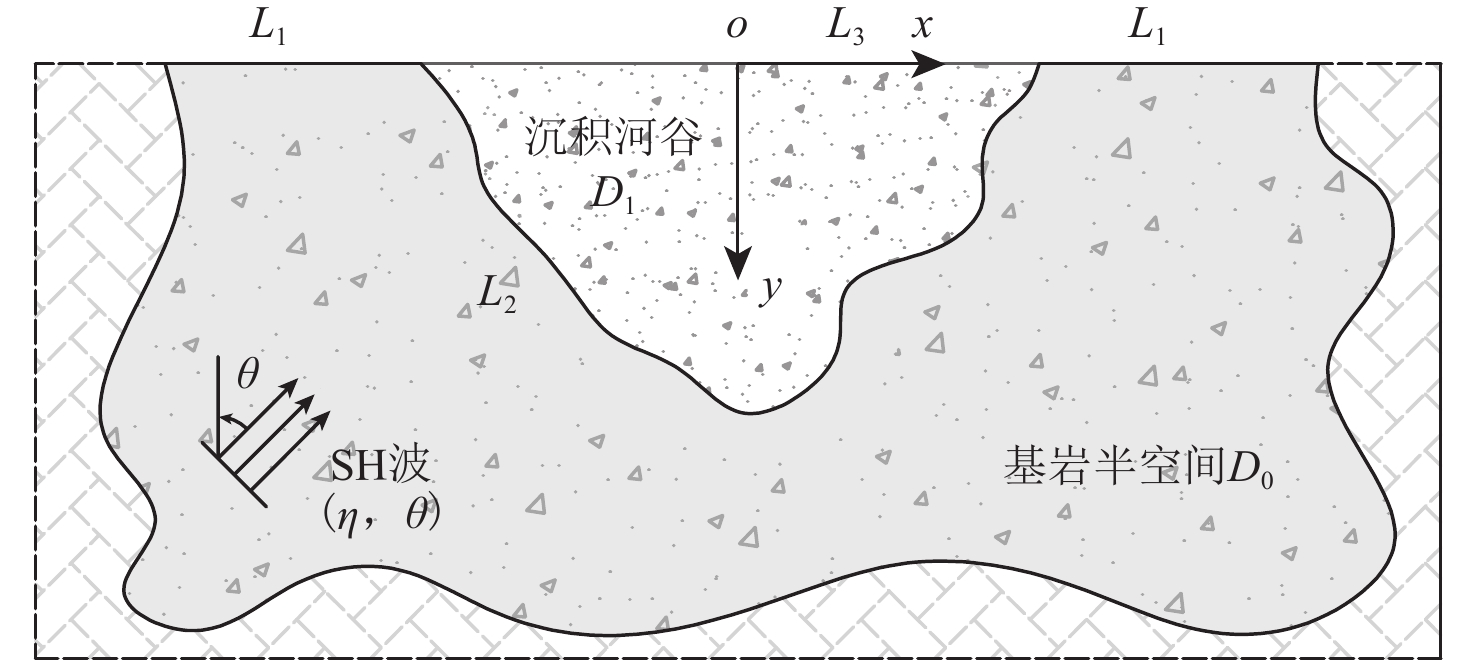
表 1 预测模型的特征参数及取值范围
Table 1 Characteristic parameters and value ranges of the prediction model
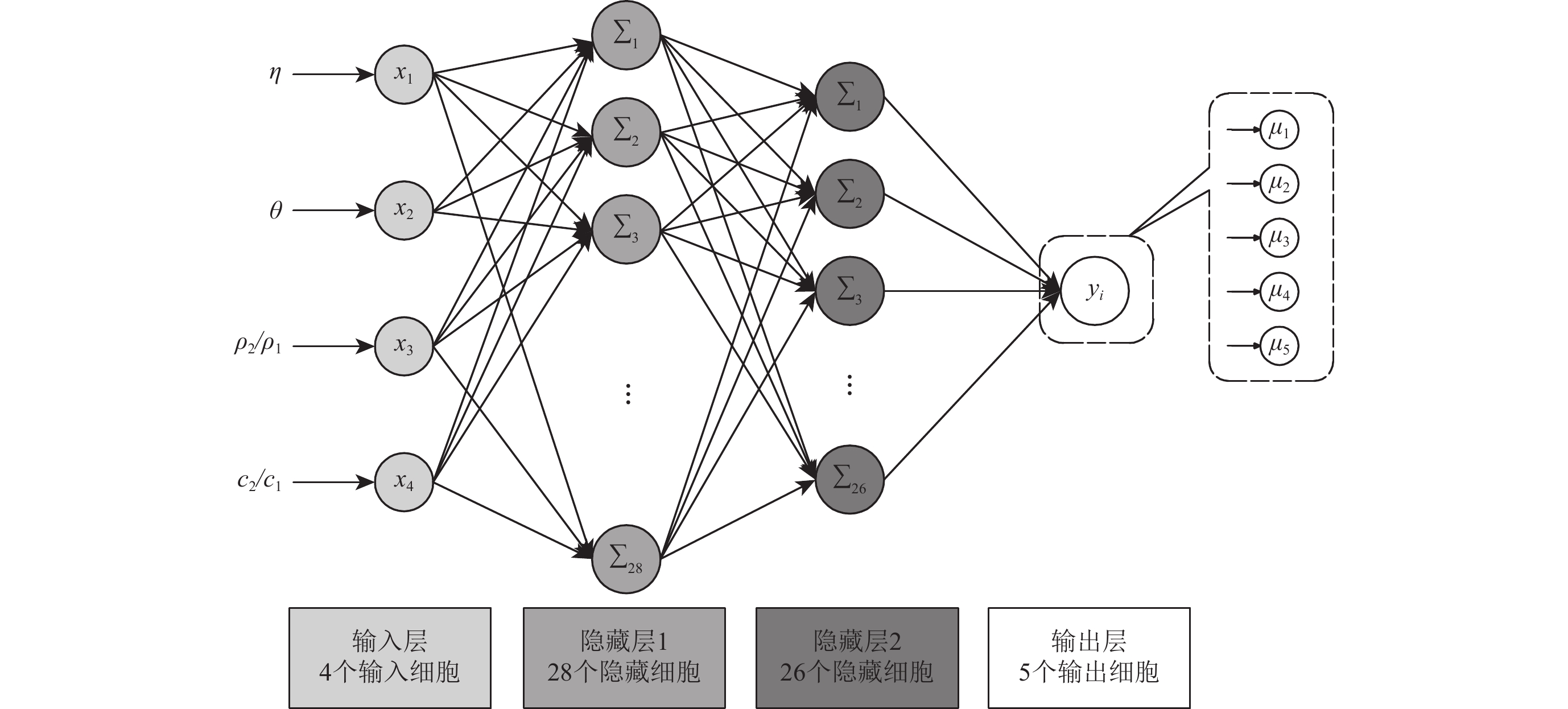
入射角θ/° 无量纲频率η 密度比ρ2/ρ1 剪切波速比c2/c1 半圆形河谷 ［60，90］ ［0.1，5］ ［0.5，1］ ［0.7，0.8］ V形河谷 ［60，90］ ［0.1，5］ ［0，1］ ［0.4，0.6］ 
下载: 导出CSV
-
陈国兴,金丹丹,朱姣,李小军. 2015. 河口盆地非线性地震效应及设计地震动参数[J]. 岩土力学,36(6):1721–1736. Chen G X,Jin D D,Zhu J,Li X J. 2015. Nonlinear seismic response of estuarine basin and design parameters of ground motion[J]. Rock and Soil Mechanics,36(6):1721–1736 (in Chinese).
陈少林,张莉莉,李山有. 2014. 半圆柱型沉积盆地对SH波散射的数值分析[J]. 工程力学,31(4):218–224. Chen S L,Zhang L L,Li S Y. 2014. Numerical analysis of the plane SH waves scattering by semi-cylindrical alluvial valley[J]. Engineering Mechanics,31(4):218–224 (in Chinese).
高玉峰,代登辉,张宁. 2021. 河谷地形地震放大效应研究进展与展望[J]. 防灾减灾工程学报,41(4):734–752. Gao Y F,Dai D H,Zhang N. 2021. Progress and prospect of topographic amplification effects of seismic wave in canyon sites[J]. Journal of Disaster Prevention and Mitigation Engineering,41(4):734–752 (in Chinese).
黄磊,刘中宪,张雪,李程程. 2020. 含流体层的河谷场地对地震波散射的间接边界元法模拟[J]. 地震学报,42(6):657–668. Huang L,Liu Z X,Zhang X,Li C C. 2020. IBEM simulation of seismic wave scattering by valley topography with fluid layer[J]. Acta Seismologica Sinica,42(6):657–668 (in Chinese).
李平,薄景山,李孝波,肖瑞杰. 2016. 安宁河河谷及邛海地区土层场地对地震动的放大作用[J]. 岩土工程学报,38(2):362–369. doi: 10.11779/CJGE201602022 Li P,Bo J S,Li X B,Xiao R J. 2016. Amplification effect of soil sites on ground motion in Anning River valley and Qionghai Lake area[J]. Chinese Journal of Geotechnical Engineering,38(2):362–369 (in Chinese).
李伟华,赵成刚. 2006. 具有饱和土沉积层的充水河谷对平面波的散射[J]. 地球物理学报,49(1):212–224. doi: 10.3321/j.issn:0001-5733.2006.01.028 Li W H,Zhao C G. 2006. Scattering of plane waves by circular-arc alluvial valleys with saturated soil deposits and water[J]. Chinese Journal of Geophysics,49(1):212–224 (in Chinese).
梁建文,巴振宁. 2007. 弹性层状半空间中沉积谷地对入射平面SH波的放大作用[J]. 地震工程与工程振动,27(3):1–9. doi: 10.3969/j.issn.1000-1301.2007.03.001 Liang J W,Ba Z N. 2007. Surface motion of an alluvial valley in layered half-space for incident plane SH waves[J]. Journal of Earthquake Engineering and Engineering Vibration,27(3):1–9 (in Chinese).
刘必灯,周正华,刘培玄,李小军,王伟. 2011. SV波入射情况下V型河谷地形对地震动的影响分析[J]. 地震工程与工程振动,31(2):17–24. Liu B D,Zhou Z H,Liu P X,Li X J,Wang W. 2011. Influence of V-shaped canyon site on ground motions for incident SV waves[J]. Journal of Earthquake Engineering and Engineering Vibration,31(2):17–24 (in Chinese).
潘兆东,谭平,刘良坤,周福霖. 2018. 基于自适应RBF神经网络算法的建筑结构递阶分散控制研究[J]. 土木工程学报,51(1):51–57. Pan Z D,Tan P,Liu L K,Zhou F L. 2018. Hierarchical decentralized control of building structure based on adaptive RBF neural network algorithm[J]. China Civil Engineering Journal,51(1):51–57 (in Chinese).
肖文海. 2009. 大型河谷场地地震动特征研究[D]. 哈尔滨: 中国地震局工程力学研究所: 1–2. Xiao W H. 2009. Research on Ground Motion Characteristic at the Site of Large-Scale Valley[D]. Harbin: Institute of Engineering Mechanics, China Earthquake Administration: 1–2 （in Chinese）.
张宁,高玉峰,何稼,徐婕,陈欣,代登辉. 2017. 平面SH波作用下部分充填圆弧形沉积谷的二维土层和地形放大效应[J]. 地震学报,39(5):778–797. doi: 10.11939/jass.2017.05.012 Zhang N,Gao Y F,He J,Xu J,Chen X,Dai D H. 2017. Two-dimensional soil and topographic amplification effects of a partially filled circular-arc alluvial valley under plane SH waves[J]. Acta Seismologica Sinica,39(5):778–797 (in Chinese).
周国良,李小军,侯春林,李铁萍. 2012. SV波入射下河谷地形地震动分布特征分析[J]. 岩土力学,33(4):1161–1166. doi: 10.3969/j.issn.1000-7598.2012.04.029 Zhou G L,Li X J,Hou C L,Li T P. 2012. Characteristic analysis of ground motions of canyon topography under incident SV seismic waves[J]. Rock and Soil Mechanics,33(4):1161–1166 (in Chinese).
Boore D M. 1973. The effect of simple topography on seismic waves:Implications for the accelerations recorded at Pacoima Dam,San Fernando Valley,California[J]. Bull Seismol Soc Am,63(5):1603–1609. doi: 10.1785/BSSA0630051603
Derras B,Bard P Y,Cotton F,Bekkouche A. 2012. Adapting the neural network approach to PGA prediction:An example based on the KiK-net data[J]. Bull Seismol Soc Am,102(4):1446–1461. doi: 10.1785/0120110088
Derras B,Bard P Y,Cotton F. 2014. Towards fully data driven ground-motion prediction models for Europe[J]. Bull Earthq Eng,12(1):495–516. doi: 10.1007/s10518-013-9481-0
Dhanya J,Raghukanth S T G. 2018. Ground motion prediction model using artificial neural network[J]. Pure Appl Geophys,175(3):1035–1064. doi: 10.1007/s00024-017-1751-3
Ducellier A,Aochi H. 2012. Interactions between topographic irregularities and seismic ground motion investigated using a hybrid FD-FE method[J]. Bull Earthq Eng,10(3):773–792. doi: 10.1007/s10518-011-9335-6
Giacinto G,Paolucci R,Roli F. 1997. Application of neural networks and statistical pattern recognition algorithms to earthquake risk evaluation[J]. Pattern Recogn Lett,18(11/12/13):1353–1362.
Kong Q K,Trugman D T,Ross Z E,Bianco M J,Meade B J,Gerstoft P. 2019. Machine learning in seismology:Turning data into insights[J]. Seismol Res Lett,90(1):3–14. doi: 10.1785/0220180259
Liu Z X,Wang D,Liang J W,Wu F J,Wu C Q. 2018. The fast multi-pole indirect BEM for solving high-frequency seismic wave scattering by three-dimensional superficial irregularities[J]. Eng Anal Bound Elem,90:86–99. doi: 10.1016/j.enganabound.2018.02.009
Luzón F,Sánchez-Sesma F J,Pérez-Ruiz J A,Ramírez-Guzmán L,Pech A. 2009. In-plane seismic response of inhomogeneous alluvial valleys with vertical gradients of velocities and constant Poisson ratio[J]. Soil Dyn Earthq Eng,29(6):994–1004. doi: 10.1016/j.soildyn.2008.11.007
Moayedi H,Raftari M,Sharifi A,Jusoh W A W,Rashid A S A. 2020. Optimization of ANFIS with GA and PSO estimating α ratio in driven piles[J]. Eng Comput,36(1):227–238. doi: 10.1007/s00366-018-00694-w
Paolucci R,Colli P,Giacinto G. 2000. Assessment of seismic site effects in 2-D alluvial valleys using neural networks[J]. Earthq Spectra,16(3):661–680. doi: 10.1193/1.1586133
Raghucharan M C,Somala S N,Rodina S. 2019. Seismic attenuation model using artificial neural networks[J]. Soil Dyn Earthq Eng,126:105828. doi: 10.1016/j.soildyn.2019.105828
Shyu W S,Teng T J,Chou C S. 2018. Effect of geometry on in-plane responses of a symmetric canyon subjected by P waves[J]. Soil Dyn Earthq Eng,113:215–229. doi: 10.1016/j.soildyn.2018.06.003
Sun Y C,Ren H X,Zheng X Z,Li N,Zhang W,Huang Q H,Chen X F. 2019. 2-D poroelastic wave modelling with a topographic free surface by the curvilinear grid finite-difference method[J]. Geophys J Int,218(3):1961–1982. doi: 10.1093/gji/ggz263
Tavakoli H,Kutanaei S S. 2015. Evaluation of effect of soil characteristics on the seismic amplification factor using the neural network and reliability concept[J]. Arab J Geosci,8(6):3881–3891. doi: 10.1007/s12517-014-1458-z
Trifunac M D. 1971. Surface motion of a semi-cylindrical alluvial valley for incident plane SH waves[J]. Bull Seismol Soc Am,61(6):1755–1770. doi: 10.1785/BSSA0610061755
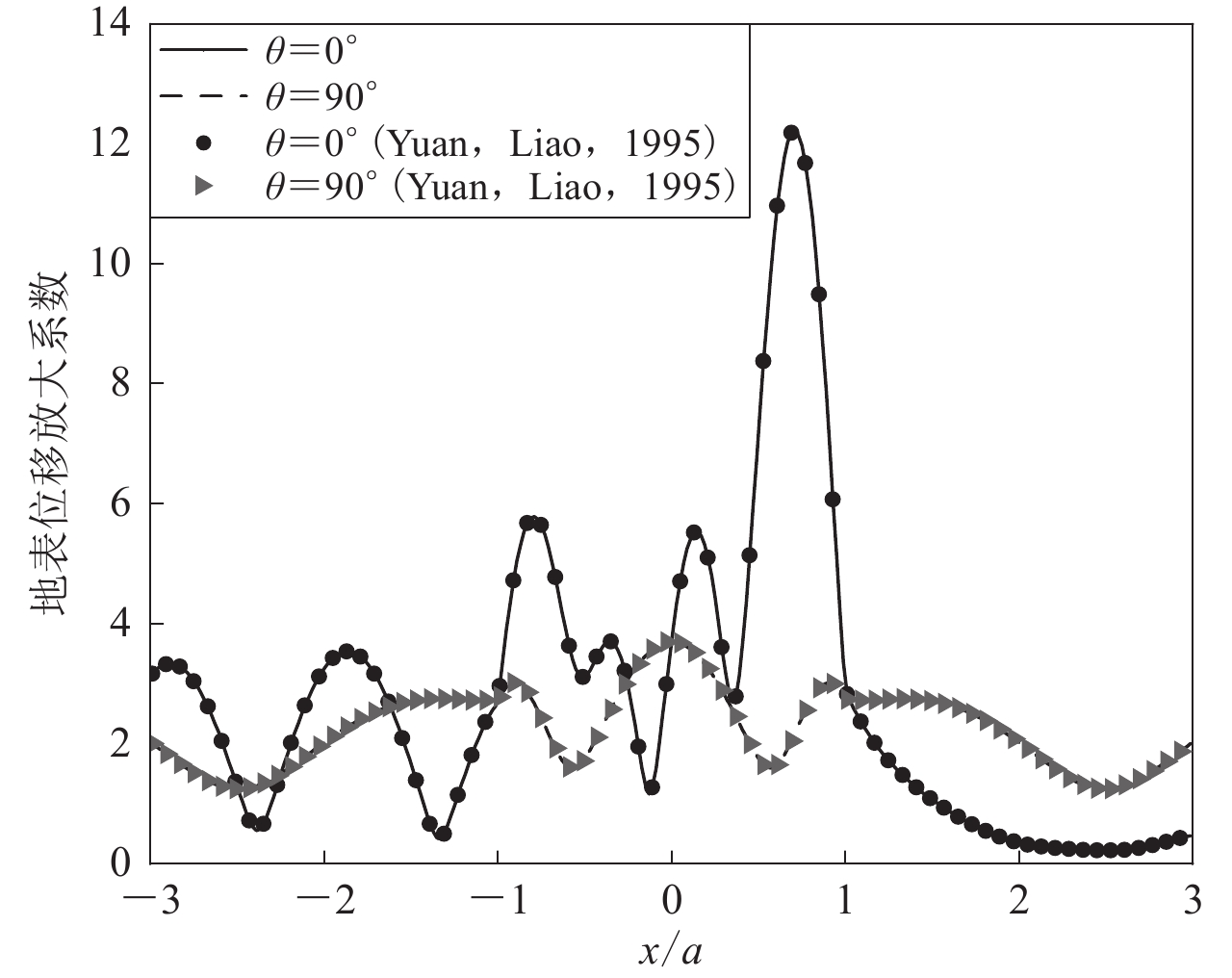
Yuan X M,Liao Z P. 1995. Scattering of plane SH waves by a cylindrical alluvial valley of circular-arc cross-section[J]. Earthq Eng Struct Dyn,24(10):1303–1313. doi: 10.1002/eqe.4290241002
Zhou H,Chen X F. 2008. The localized boundary integral equation-discrete wavenumber method for simulating P-SV wave scattering by an irregular topography[J]. Bull Seismol Soc Am,98(1):265–279. doi: 10.1785/0120060249
计量
- 文章访问数: 362
- HTML全文浏览量: 164
- PDF下载量: 70
