Estimating stress drop of the 2013 Lushan earthquake sequence based on the empirical Green’s function spectral ratio method
-
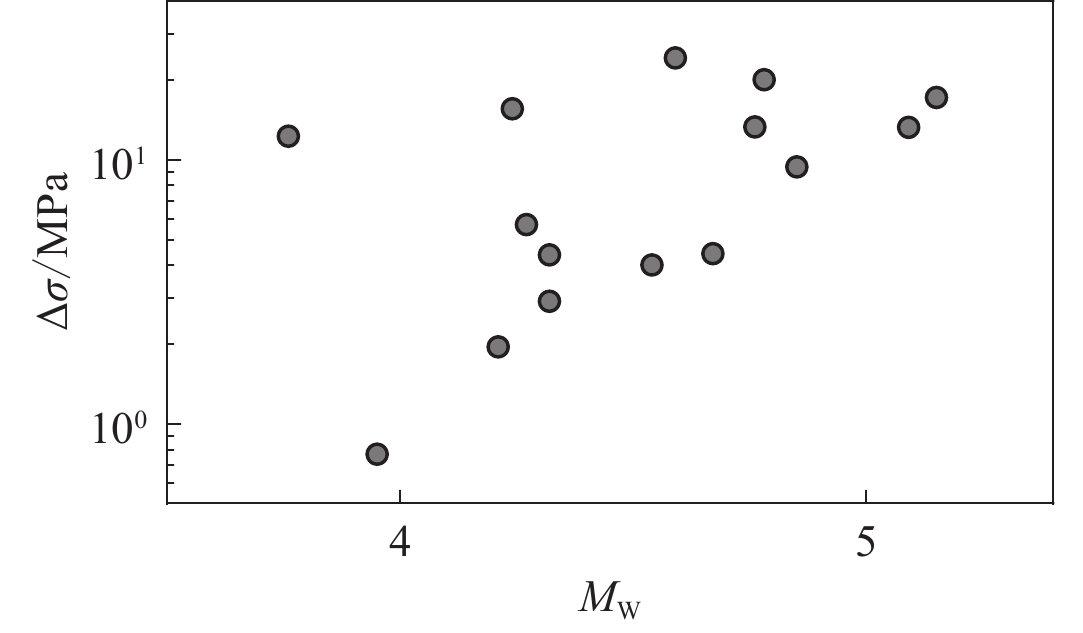
摘要: 本文以芦山地震余震为例，分析了基于强震动观测记录的经验格林函数（EGF）谱比法估计地震的拐角频率和应力降的可行性。首先给出能够可靠估计地震拐角频率和应力降的经验格林函数谱比曲线的质量标准；然后利用其估算出17次ML3.8—5.4芦山强余震的拐角频率；最后，参考其它研究给出的地震矩震级，计算出地震应力降。结果显示，芦山强余震的拐角频率主要介于1.0—2.0 Hz之间，应力降平均值为9.98 MPa，且地震应力降表现出明显的震级相关性。Abstract: We adopted the 2013 Lushan aftershocks as a typical example, to investigate the feasibility of estimating corner frequency and stress drop by the empirical Green’s function （EGF） spectral ratio method based on the strong motion observation data. Firstly, we suggested the quality standard on the EGF spectral ratio curve to guarantee the reliable estimation of seismic corner frequency and stress drop. The corner frequencies for the 17 Lushan aftershocks with magnitude in the range of 3.8−5.4 were then estimated. Referring to the seismic moment magnitude given by other studies, we further computed the seismic stress drops.The results show that the corner frequency of Lushan strong aftershocks is mainly in the range of 1.0−2.0 Hz, the average stress drop is 9.98 MPa, and seismic stress drop presents obvious dependency on seismic magnitude.
-
Keywords:
- Empirical Green’s function /
- spectral ratio /
- Lushan earthquake /
- stress drop
-
-
![]()
图 1 目标地震及其经验格林函数地震分布
图中数字编号为筛选后的目标地震编号（按发震时间排序）
Figure 1. Distribution of target earthquakes and its EGF earthquakes
The numbers in the figure mean the selected target earthquakes’ number （sorted by occuring time）
![]()
图 2 EGF谱比法计算目标地震拐角频率算例
（a） 目标地震及其EGF地震和触发台站位置分布；（b） 水平向傅氏谱；（c） EGF谱比曲线拟合；（d） M01/M0j，ƒcj随ƒc1可能值的变化；（e） Var随ƒc1可能值（颜色与图（c）对应）的变化；（f） 基于多个EGF谱比曲线的目标地震拐角频率估计值
Figure 2. An example of estimating target earthquake 's corner frequency by spectral ratio method
（a） Target earthquakes ，EGF earthquakes and trigger stations location distribution ； （b） Horizontal fourier spectrum ；（c） EGF spectral ratio curve fitting；（d） M01/M0j，fcj vary with the possible value of fc1；（e） Var varies with the possible value of fc1 ，color corresponding to figure （c）；（f） Estimated value of target earthquake’s corner frequency based on multiple EGF spectral ratio curves
表 1 目标地震的震源参数
Table 1 Source parameters of target earthquakes
地震序号 发震时间 ML 震中位置 EGF地震个数 记录数 ƒc1/Hz MW Δσ/MPa 年-月-日 时:分:秒 北纬/° 东经/° 1 2013-04-20 08:06:38 4.8 30.18 102.88 16 22 1.56 2 2013-04-20 08:31:34 4.1 30.39 103.01 2 3 1.91 5 2013-04-20 09:02:57 4.6 30.28 102.93 9 9 1.28 4.67* 4.41 6 2013-04-20 09:11:51 4.3 30.25 102.83 9 14 1.67 4.76* 13.35 7 2013-04-20 09:20:09 4.6 30.17 102.99 3 3 2.48 4.59* 24.31 8 2013-04-20 09:26:00 4.4 30.19 102.95 3 4 1.66 4.21† 1.96 9 2013-04-20 09:37:28 4.9 30.28 102.99 18 20 1.87 4.78* 20.09 10 2013-04-20 09:39:34 4.5 30.13 102.84 5 5 1.44 4.54* 4.00 14 2013-04-20 11:34:17 5.3 30.24 102.94 23 114 1.16 5.15* 17.21 16 2013-04-21 11:59:37 4.9 30.26 103.00 7 8 1.34 4.85* 9.41 17 2013-04-21 12:39:18 3.8 30.22 102.89 2 2 1.64 3.95* 0.77 18 2013-04-21 17:05:22 5.4 30.34 103.00 15 91 1.14 5.09* 13.28 19 2013-04-21 17:30:24 4.2 30.28 102.98 14 15 2.21 4.27* 5.70 21 2013-04-21 22:16:54 4.3 30.33 102.93 20 28 1.91 4.32* 4.37 22 2013-04-23 05:54:49 4.5 30.35 103.00 6 6 1.67 4.32* 2.92 23 2013-04-23 22:07:15 3.8 30.29 102.96 4 4 5.14 3.76* 12.31 24 2013-05-01 02:14:13 4.1 30.25 102.89 9 9 3.2 4.24* 15.59 注：标*数据来自易桂喜等（2016），标†数据来自温瑞智等（2015）。 
下载: 导出CSV
表 2 部分EGF地震的拐角频率
Table 2 Corner frequency of partial EGF earthquakes
发震时间 ML 震中位置 记录数 ƒcj/Hz 年-月-日 时:分:秒 北纬/° 东经/° 2013-04-20 22:10:32 3.1 30.28 102.39 4 6.78 2013-04-29 13:45:40 3.0 30.26 102.88 3 2.79 2013-04-25 01:20:15 3.2 30.29 102.88 5 3.58 2013-04-25 14:11:22 3.0 30.28 102.92 12 6.51 2013-04-22 19:04:52 3.1 30.21 102.96 3 2.42 2013-04-24 21:36:35 3.1 30.26 102.90 12 3.91
下载: 导出CSV
-
林向东,葛洪魁,徐平,Dreger D,苏金蓉,王宝善,武敏捷. 2013. 近场全波形反演:芦山7.0级地震及余震矩张量解[J]. 地球物理学报,56(12):4037–4047. doi: 10.6038/cjg20131209 Lin X D,Ge H K,Xu P,Dreger D,Su J R,Wang B S,Wu M J. 2013. Near field full waveform inversion:Lushan magnitude 7.0 earthquake and its aftershock moment tensor[J]. Chinese Journal of Geophysics,56(12):4037–4047 (in Chinese).
吕坚,王晓山,苏金蓉,潘林山,李正,尹利文,曾新福,邓辉. 2013. 芦山7.0级地震序列的震源位置与震源机制解特征[J]. 地球物理学报,56(5):1753–1763. doi: 10.6038/cjg20130533 Lü J,Wang X S,Su J R,Pan L S,Li Z,Yin L W,Zeng X F,Deng H. 2013. Hypocentral location and source mechanism of the MS7.0 Lushan earthquake sequence[J]. Chinese Journal of Geophysics,56(5):1753–1763 (in Chinese).
温瑞智,王宏伟,任叶飞,冀昆. 2015. 芦山余震震源参数及震源区品质因子反演[J]. 哈尔滨工业大学学报,47(4):58–63. doi: 10.11918/j.issn.0367-6234.2015.04.010 Wen R Z,Wang H W,Ren Y F,Ji K. 2015. Estimation of source parameters and quality factor based on generalized inversion method in Lushan earthquake[J]. Journal of Harbin Institute of Technology,47(4):58–63 (in Chinese).
易桂喜,龙锋,Vallage A,Klinger Y,梁明剑,王思维. 2016. 2013年芦山地震序列震源机制与震源区构造变形特征分析[J]. 地球物理学报,59(10):3711–3731. doi: 10.6038/cjg20161017 Yi G X,Long F,Vallage A,Klinger Y,Liang M J,Wang S W. 2016. Focal mechanism and tectonic deformation in the seismogenic area of the 2013 Lushan earthquake sequence,southwestern China[J]. Chinese Journal of Geophysics,59(10):3711–3731 (in Chinese).
喻畑,李小军. 2012. 汶川地震余震震源参数及地震动衰减与场地影响参数反演分析[J]. 地震学报,34(5):621–632. doi: 10.3969/j.issn.0253-3782.2012.05.004 Yu T,Li X J. 2012. Inversion of strong motion data for source parameters of Wenchuan aftershocks,attenuation function and average site effect[J]. Acta Seismologica Sinica,34(5):621–632 (in Chinese).
郑勇,葛粲,谢祖军,Yang Y J,熊熊,许厚泽. 2013. 芦山与汶川地震震区地壳上地幔结构及深部孕震环境[J]. 中国科学:地球科学,43(6):1027–1037. Zheng Y,Ge C,Xie Z J,Yang Y J,Xiong X,Hsu H T. 2013. Crustal and upper mantle structure and the deep seismogenic environment in the source regions of the Lushan earthquake and the Wenchuan earthquake[J]. Science China Earth Sciences,56(7):1158–1168. doi: 10.1007/s11430-013-4641-2
Abercrombie R E,Bannister S,Ristau J,Doser D. 2017. Variability of earthquake stress drop in a subduction setting,the Hikurangi Margin,New Zealand[J]. Geophys J Int,208(1):306–320. doi: 10.1093/gji/ggw393
Allmann B P,Shearer P M. 2009. Global variations of stress drop for moderate to large earthquakes[J]. J Geophys Res:Solid Earth,114(B1):B01310.
Boatwright J. 1980. A spectral theory for circular seismic sources; simple estimates of source dimension,dynamic stress drop,and radiated seismic energy[J]. Bull Seismol Soc Am,70(1):1–27.
Boatwright J,Fletcher J B,Fumal T E. 1991. A general inversion scheme for source,site,and propagation characteristics using multiply recorded sets of moderate-sized earthquakes[J]. Bull Seismol Soc Am,81(5):1754–1782.
Boyd O S,McNamara D E,Hartzell S,Choy G. 2017. Influence of lithostatic stress on earthquake stress drops in North America[J]. Bull Seismol Soc Am,107(2):856–868. doi: 10.1785/0120160219
Brune J N. 1970. Tectonic stress and the spectra of seismic shear waves from earthquakes[J]. J Geophys Res,75(26):4997–5009. doi: 10.1029/JB075i026p04997
Eshelby J D. 1957. The determination of the elastic field of an ellipsoidal inclusion,and related problems[J]. Proc Roy Soc A:Math Phys Eng Sci,241(1226):376–396.
Hanks T C,Kanamori H. 1979. A moment magnitude scale[J]. J Geophys Res:Solid Earth,84(B5):2348–2350. doi: 10.1029/JB084iB05p02348
Hartzell S H. 1978. Earthquake aftershocks as Green's functions[J]. Geophys Res Lett,5(1):1–4. doi: 10.1029/GL005i001p00001
Holmgren J M,Atkinson G M,Ghofrani H. 2019. Stress drops and directivity of induced earthquakes in the western Canada sedimentary basin[J]. Bull Seismol Soc Am,109(5):1635–1652. doi: 10.1785/0120190035
Ide S,Beroza G C,Prejean S G,Ellsworth W L. 2003. Apparent break in earthquake scaling due to path and site effects on deep borehole recordings[J]. J Geophys Res:Solid Earth,108(B5):2271.
Kaneko Y,Shearer P M. 2014. Seismic source spectra and estimated stress drop derived from cohesive-zone models of circular subshear rupture[J]. Geophys J Int,197(2):1002–1015. doi: 10.1093/gji/ggu030
Konno K,Ohmachi T. 1998. Ground-motion characteristics estimated from spectral ratio between horizontal and vertical components of microtremor[J]. Bull Seismol Soc Am,88(1):228–241. doi: 10.1785/BSSA0880010228
Li J Y,Zhou B G,Rong M S,Chen S,Zhou Y. 2020. Estimation of source spectra,attenuation,and site responses from strong‐motion data recorded in the 2019 Changning earthquake sequence[J]. Bull Seismol Soc Am,110(2):410–426.
Oth A,Bindi D,Parolai S,Di Giacomo D. 2010. Earthquake scaling characteristics and the scale-(in)dependence of seismic energy-to-moment ratio:Insights from KiK-net data in Japan[J]. Geophys Res Lett,37(19):L19304.
Pacor F,Spallarossa D,Oth A,Luzi L,Puglia R,Cantore L,Mercuri A,D’Amico M,Bindi D. 2016. Spectral models for ground motion prediction in the L’Aquila region (central Italy):Evidence for stress-drop dependence on magnitude and depth[J]. Geophys J Int,204(2):697–718. doi: 10.1093/gji/ggv448
Trugman D T. 2020. Stress-drop and source scaling of the 2019 Ridgecrest,California,earthquake sequence[J]. Bull Seismol Soc Am,110(4):1859–1871. doi: 10.1785/0120200009
Trugman D T,Shearer P M. 2018. Strong correlation between stress drop and peak ground acceleration for recent M1−4 earthquakes in the San Francisco bay area[J]. Bull Seismol Soc Am,108(2):929–945. doi: 10.1785/0120170245
Viegas G,Abercrombie R E,Kim W Y. 2010. The 2002 M5 Au Sable Forks,NY,earthquake sequence:Source scaling relationships and energy budget[J]. J Geophys Res:Solid Earth,115(B7):B07310.
Wang H W,Wen R Z. 2020. Earthquake source characteristics and S-wave propagation attenuation in the junction of the Northwest Tarim Basin and Kepingtage fold-and-thrust zone[J]. Front Earth Sci,8:567939. doi: 10.3389/feart.2020.567939
Yoshimitsu N,Ellsworth W L,Beroza G C. 2019. Robust stress drop estimates of potentially induced earthquakes in Oklahoma:Evaluation of empirical green's function[J]. J Geophys Res:Solid Earth,124(6):5854–5866. doi: 10.1029/2019JB017483
计量
- 文章访问数: 371
- HTML全文浏览量: 128
- PDF下载量: 112
