A transfer function based on Laplace transform for correcting narrow-frequency velocity recording
-
摘要: 受窄频地震仪平坦响应范围影响,窄频速度记录存在低频成分失真问题,导致地震记录可用范围受限。针对此问题,本文推导基于拉普拉斯变换和双线性变换的传递函数,实现由窄频地震记录向宽频地震记录的校正,并以日本Hi-net速度记录为例进行验证,将校正后的速度记录与同台KiK-net加速度积分所得的速度记录予以对比。结果显示,原始速度记录在低频处存在失真,而校正后的波形与KiK-net加速度积分速度记录波形一致,这表明改进的传递函数能有效地解决原速度记录中的低频成分失真问题,有效地拓宽了低频可使用范围,而且相较于Nakata校正速度记录方法,以本文给出的传递函数校正的速度记录在振幅和波形等方面的精度更高。Abstract: Affected by the flat response range of the narrow-band seismograph, the narrow-band velocity recording has the problem of low-frequency component distortion, which limits the usable range of the seismic recording. To solve this problem, this paper deduces an improved transfer function based on the Laplace transform and bilinear transform to realize the correction from narrow-band seismic records to broadband ones. And then the Japanese Hi-net velocity records are used as an example for correction, and the corrected velocity records are compared with the KiK-net acceleration integral velocity records from the same station. The results show that the original velocity records are distorted at low frequencies, while the corrected waveforms are consistent with the KiK-net acceleration integral velocity records. The analyse show that the improved transfer function can effectively solve the distortion of the low-frequency components in the original velocity records, which effectively widens the usable range of low-frequency. Moreover, compared with the Nakata-corrected velocity recording method, the velocity recording corrected with the transfer function given in this paper is more accurate in terms of amplitude and waveform.
-
Keywords:
- velocity record /
- transport function /
- Laplacetransform /
- bilinear transformation /
- Hi-net
-
引言
窄频地震仪的平坦响应范围无法覆盖低频段,导致地震记录中的低频信号被抑制。以日本地震台网记录仪器为例,KiK-net强震仪的平坦响应范围是DC—20 Hz,F-net宽频带地震仪的平坦响应范围为360 s—8.5 Hz 和120 s—50 Hz (任枭等,2009),而与它们同台布设的Hi-net窄频地震仪的平坦响应范围为1—30 Hz (Nakata,2013),其中Hi-net窄频地震仪记录的低频(<1 Hz)成分存在失真现象,使得低频段Hi-net的速度波形峰值比F-net宽频带地震仪记录的速度波形峰值小,基于窄频速度记录重构的地面位移也小于基于宽频加速度或宽频速度记录重构的地面位移(文雪康等,2020)。
国内外研究人员针对如何校正窄频地震记录开展了大量研究,现有的校正方法主要分为时域方法和频域方法两大类。关于采用时域方法的研究较多,例如:周云耀等(2002)提出从速度输出通道测定超宽频带与甚宽频带地震计二阶传递函数的时域响应测试方法;马强等(2003)提出了一套能较精确模拟单自由度系统传递函数的时域方法,采用递归格式进行计算,并证明了该方法的有效性;金星等(2004)提出时域内实时计算公式,利用传递函数以数字化速度记录仿真位移和加速度时程,并用实例证明了该方法速度快、精度高;杨笑梅和郭钦华(2007)在二维复杂场地条件下,利用时域递归公式完成了地震动的修正,并给出了相应的简化方法;Maeda等(2011)推导时域递归滤波器,以时域内传递函数修复窄频记录,以恢复失真的地面运动记录长周期分量;李永振等(2012)使用金星等(2004)提出的传递函数,由宽频带加速度记录仿真得到了速度和位移时程。但时域校正方法并不能直接的获得信号频率分量的变化情况。
频域方法是从频率的角度出发,调整信号的组成部分,主要基于传递函数展开。俞言祥等(1999)用传递函数扣除地震仪器响应来恢复地面位移、速度和加速度,使用宽频带记录计算长周期地震动反应谱以验证其可靠性;张宁等(2022)利用传递函数对窄频地震记录中1Hz以下成分进行校正,以提高用速度记录计算地震反应谱的精度;盛谦等(2012)将传递函数引入地下工程地震响应研究中,给出了地下工程地震响应的频谱特性分析、地震响应时频估算以及地震动输入频谱校正方法。潘岳怡等(2019)以广西地区地震记录为例,利用传递函数对仪器采集的加速度和速度进行折算,重构地面运动速度和加速度,验证了一定条件下速度波形与加速度波形可相互转化,同时指出对于强震记录要考虑场地效应。Nakata (2013)利用频域传递函数对Hi-net窄频速度记录进行校正,但得到的加速度波形与KiK-net加速度波形相比,在低频段重合度差。
鉴于此,本文拟利用双线性变换和拉普拉斯变换,推导校正窄频速度记录的频域传递函数,并选取日本Hi-net窄频速度记录为例进行验证,将校正后的速度记录先与同台站KiK-net加速度积分速度记录进行对比,再与Nakata校正速度记录结果对比,验证本文推导的传递函数有效性及其计算精度,从而达到扩宽窄频速度记录使用范围的目的。
1. 传递函数校正方法
地震仪动力平衡方程为
$$ \ddot x ( t ) + 2\xi {\omega _0}\dot x ( t ) + \omega _0^2x ( t ) = \ddot y ( t ) \text{,} $$ (1) 式中,ω0为自振角频率,ξ为阻尼常数,x(t)为地震仪的输出位移,
$\ddot x ( t ) $ 为地震仪输出加速度,$\dot x ( t ) $ 为地震仪输出速度,$\ddot y ( t ) $ 为输入地面运动加速度。对式(1)进行拉普拉斯变换,可获得地震仪在拉普拉斯域内的传递函数为:
$$ H ( s ) = \frac{{{s^2}}}{{{s^2} - 2{\rm{i}}\xi {\omega _0}s - {\omega _0^2}}} {\text{,}} $$ (2) 式中s为微分算子。利用传递函数对窄频记录进行校正时,需对窄频记录进行解卷积,并对原始宽频记录进行卷积处理。将窄频记录和原始宽频记录代入式(2)再相除,有
$$ {H^{\rm{c}}} ( s ) = \frac{{{s^2} - 2{\rm{i}}{\xi _{\rm{N}}}{\omega _{0{\rm{N}}}}s - \omega _{0{\rm{N}}}^2}}{{{s^2} - 2{\rm{i}}{\xi _{\rm{B}}}{\omega _{0{\rm{B}}}}s - \omega _{0{\rm{B}}}^2}} \text{,} $$ (3) 式中:ω0N和ω0B分别为窄频地震仪和宽频地震仪的自然角频率,ξN和ξB分别为相应的阻尼系数。
由于地震仪输出信号为离散信号,而式(3)为s域内的连续信号,因此利用双线性变换将连续信号映射为离散信号。首先利用
$$ \omega = {\frac{2}{T}}\arctan \left(\frac{{\varOmega T}}{2}\right) $$ (4) 限定频率范围,式中:
$\varOmega $ 为无限角频率,T为采样间隔,ω的范围为(−π/T,π/T).然后利用z变换求解s值。在频域中,z变换表示如下:$$ {\textit{z}} = {{\rm{e}}^{\rm{i}\omega }} \text{,} $$ (5) 式中s=iω,则s值可表示为
$$ s = \ln {\textit{z}} {\text{.}} $$ (6) 最后将式(6)代入式(3),应用式(4)的频率范围,得到频域内的双二阶传递函数:
$$ {H^{\rm{c}}} ( {{\rm{e}}^{{\rm{i}}\omega }} ) = \frac{{{a_0} + {a_1}{{{{\rm{e}}^{-{\rm{i}}\omega }}}} + {a_2}{{{{\rm{e}}^{-2{\rm{i}}\omega }}}}}}{{{b_0} + {b_1}{{{{\rm{e}}^{-{\rm{i}}\omega }}}} + {b_2}{{{{\rm{e}}^{-2{\rm{i}}\omega }}}}}} \text{,} $$ (7) 式中a,b为传递函数系数。利用式(7)的传递函数即可将窄频地震记录校正至宽频地震记录。
下面将以日本Hi-net速度记录为例,利用式(7)的传递函数对其进行校正。代入的Hi-net窄频地震仪的角频率和阻尼系数分别为ω0N=2π Hz和ξN=0.7。由于F-net地震仪记录低频的能力更强,精度更准确,所以采用F-net地震仪参数参与计算。取F-net地震仪的角频率和阻尼系数为ω0B=2π/135 rad/s和ξB=0.707。此时,式(7)中ai和bi (i=0,1,2)的数值列于表1。
表 1 校正窄频速度记录的传递函数系数Table 1. Transfer function coefficient for correcting narrow-band velocity recordingai bi i=0 1.044 984 1.000 370 i=1 −1.998 024 −1.999 999 i=2 0.956 990 0.999 629 2. 算例分析
选取日本Hi-net网站和KiK-net网站的四组地震记录(表2)作为算例,分别计算这四组数据的原始Hi-net速度记录和KiK-net加速度记录的信噪比。
表 2 本研究所用的日本同台站地震记录Table 2. Earthquake records at the same station in Japan编号 Hi-net
站点地震名称 发震时间(JST) 震中位置 震源
深度/kmM 方向 KiK-net
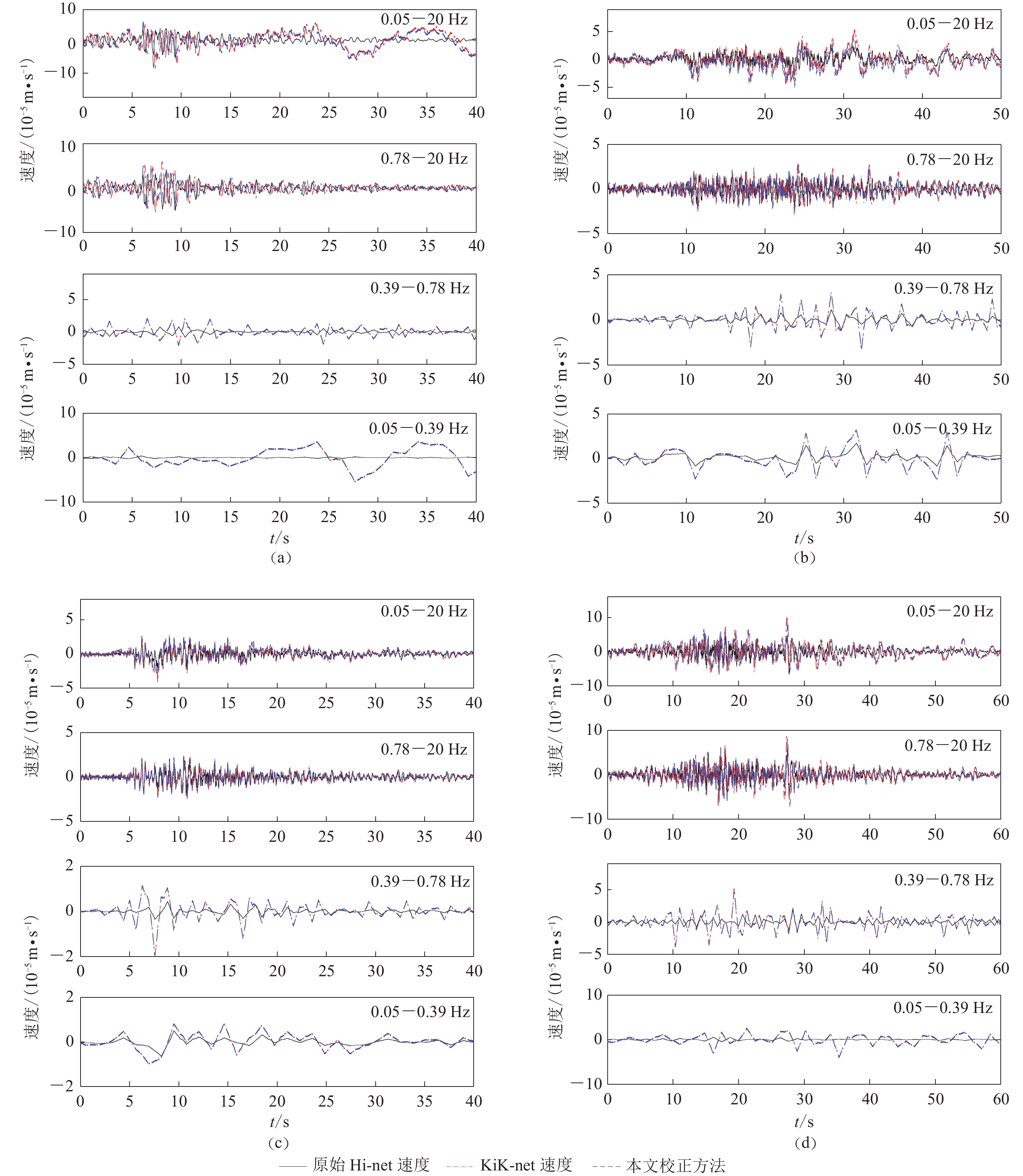
站点记录信噪比 年-月-日 时:分:秒 北纬/° 东经/° 速度 加速度 1 N.TSKH 茨城县地震 2008-05-08 01:45:00 36.227 141.607 51 7.0 EW IBRH19 72.45 48.55 2 N.TSKH 宫城地震 2011-04-07 03:54:00 38.20 141.92 66 7.1 EW IBRH19 23.97 12.10 3 N.TSKH 磐城县地震 2011-07-31 03:54:00 36.90 141.22 57 6.5 NS IBRH19 102.16 52.60 4 N.TSKH 福岛县地震 2021-02-13 08:00:00 37.73 141.70 55 7.3 EW IBRH19 85.30 55.51 选取与Hi-net地震仪同台布置的KiK-net地震仪(加速度)和F-net宽频地震仪(速度)输出的记录作为参考值,将其与校正后的速度记录进行对比。由于上述三个网站地震仪的采样频率有差别,F-net宽频地震仪记录的采样频率为20 Hz,Hi-net窄频地震仪记录的采样频率为100 Hz;KiK-net强震仪记录采样频率为100 Hz或200 Hz (冯静等,2013),因此对比算法精度时,按频率范围划分,即0.05—1 Hz范围内以F-net频谱值为标准值、1—20 Hz内以KiK-net加速度积分速度频谱值为标准值进行对比,原始Hi-net速度记录、校正后速度记录与标准值的对比结果如图1所示;在0.05—20 Hz范围内以KiK-net加速度积分速度记录作为参考,与校正后的速度记录进行对比,结果如图2和图3所示。
从图1和图3可以看出,原始Hi-net速度记录在低频部分存在明显失真,而校正后的Hi-net速度记录与KiK-net加速度积分速度记录的频率幅值相差不大。从分频段时程(图2)对比可以看出,原始Hi-net速度记录在0.05—0.78 Hz频段内的振幅远小于参考值,而使用本文方法校正得到的Hi-net速度记录与KiK-net加速度积分速度基本重合。
上述算例表明:对于信噪比大于12的地震记录,利用本文方法来校正低频成分缺失有效,且校正结果的精度较高;本文方法将窄频地震仪低频部分的可使用范围从1—20 Hz拓宽至0.05—20 Hz。
3. 与其它方法对比
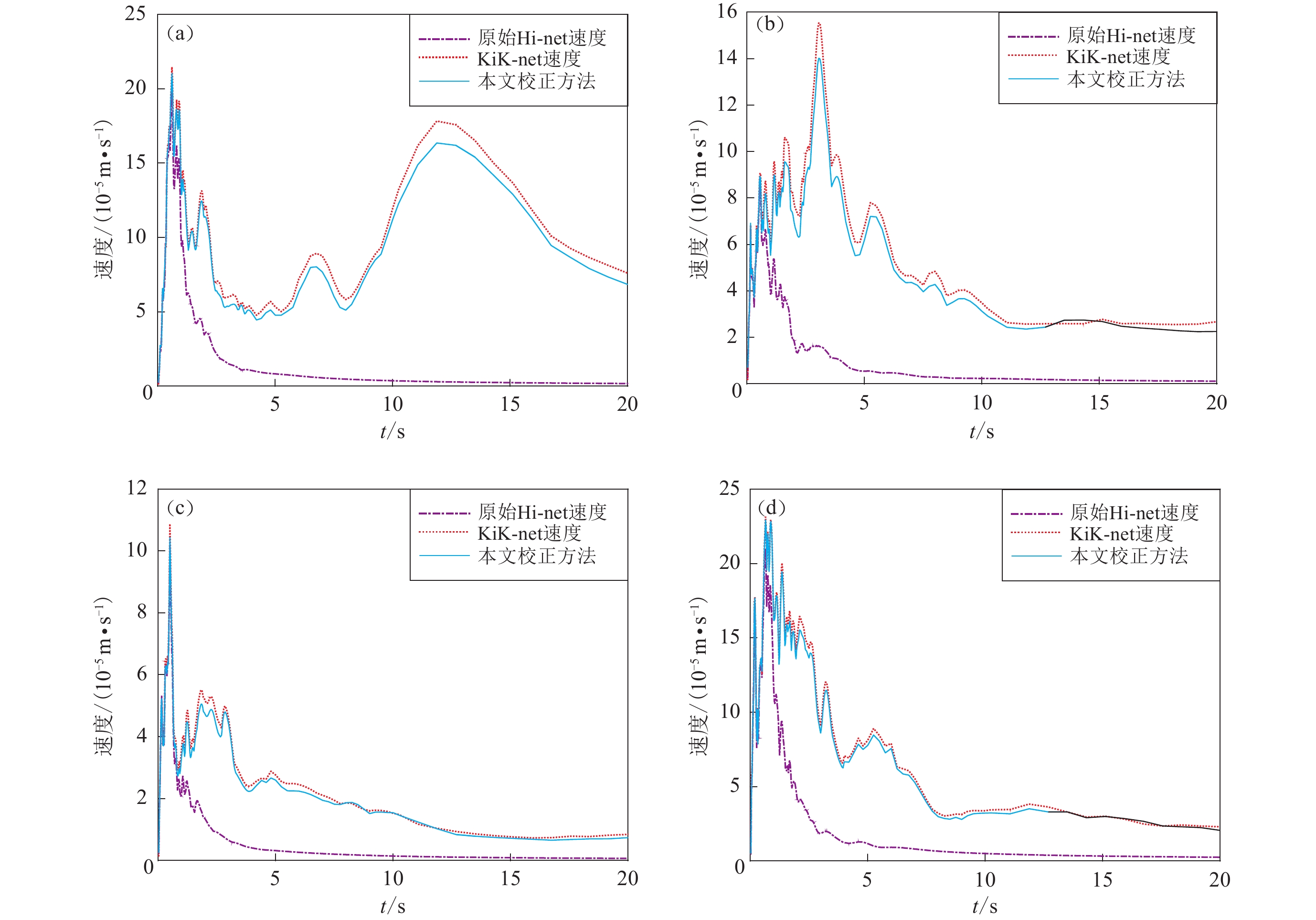
为验证本文方法与Nakata方法的计算精度差异,仍以表2中的四组数据为例,分别计算原始Hi-net速度记录、本文方法校正Hi-net速度记录、KiK-net积分速度记录和Nakata方法校正Hi-net速度记录的峰值,结果如表3所示。可以看出,以Nakata传递函数校正的速度记录峰值偏小,而以本文传递函数校正的速度记录峰值与同台记录的加速度积分速度峰值相差不大。
表 3 本文方法和Nakata方法校正速度记录峰值对比Table 3. Comparison of the peak value of the correction velocity recording by this method with that by the Nakata method地震 原始Hi-net速度记录
峰值/(10−5 m·s−1)KiK-net积分速度记录
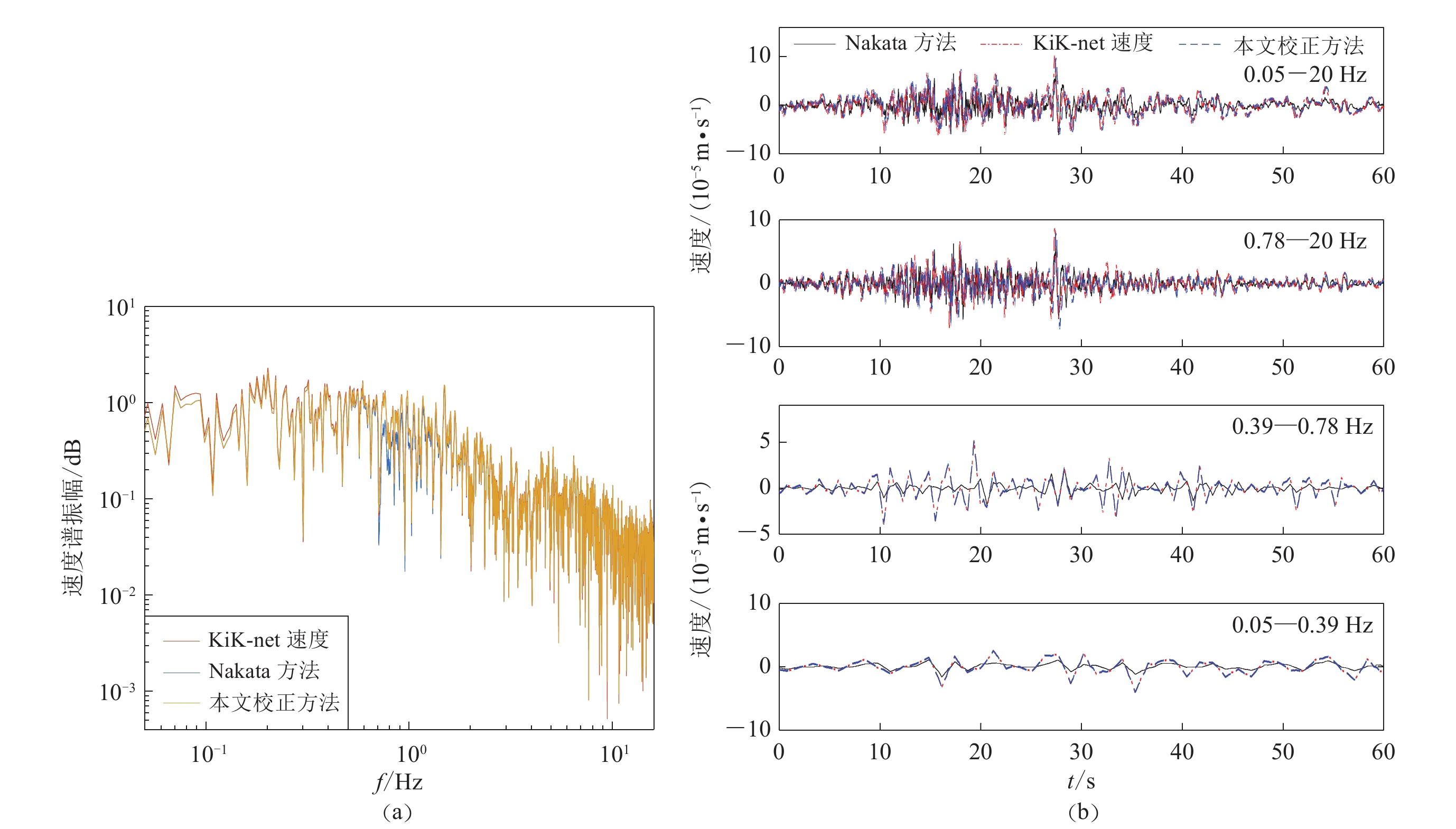
峰值/(10−5 m·s−1)校正的Hi-net速度记录峰值/(10−5 m·s−1) 本文方法 Nakata方法 茨城县地震 5.0 8.2 8.2 5.5 宫城地震 2.4 5.4 5.1 3.3 磐城县地震 3.9 4.3 4.1 3.5 福岛县地震 5.9 10.2 9.7 6.5 为了更直观地对比两种校正方法之间的差别,绘制Nakata方法、本文校正方法和KiK-net积分速度曲线的对比图。由于四组数据校正结果的差异规律相同,在此仅列出第四组数据的对比图(图4)。
从图4可以看出,在0.05—20 Hz范围内经本文方法校正后的结果与KiK-net加速度积分速度波形的重合度高,而Nakata方法校正所得结果与之偏差较大。按频段范围从大到小的顺序进行详细对比,可见:在0.78—20 Hz频段内,两种方法的校正结果基本一致;在0.05—0.39 Hz和0.39—0.78 Hz频段内,Nakata方法的校正结果存在较大误差。这说明Nakata方法校正低频信号的能力不足。综合表3和图4的结果可知,本文校正方法的计算精度更高,效果更好。
4. 讨论与结论
传递函数常用于恢复地面运动记录或者速度、加速度和位移记录在某一频段范围内的重构、仿真,可以采用傅里叶变换、脉冲响应不变法、拉普拉斯变换和双线性变换等建立。Nakata方法就是基于傅立叶变换建立的传递函数,但其局限于各个频率下的三角函数不随时间衰减,即复函数的幅值不随时间变化,所表述的信号必须满足狄利赫利(Dirichlet)条件,这就导致其应用范围的局限性较大;以脉冲响应不变法建立的传递函数需要求解的系数会减少,但应用此函数须限定频率范围,否则会出现频率混叠的现象(谢剑波,2014)。从理论上来看,以上述方法建立的传递函数均会存在计算误差。以拉普拉斯变换建立的复函数的幅值会随时间变化,所表达的函数范围更广,双线性变换可有效地解决频率混叠的现象,因此,基于拉普拉斯变换和双线性变换建立的传递函数,能避免上述缺陷。
利用本文给出的传递函数校正窄频地震记录,并与Nakata传递函数对比可知,经本文的传递函数校正后的速度记录波形与同台记录的KiK-net加速度积分速度记录波形具有高度一致性,且本文传递函数校正1 Hz以下频段范围内信号时准确性更高,能够将窄频地震记录的有效频段范围从1—20 Hz拓宽至0.05—20 Hz。由于窄频地震仪在我国台网中使用较少,且已有地震数据不公开,目前尚无法检验本文方法对我国窄频地震记录的适用性。
-
表 1 校正窄频速度记录的传递函数系数
Table 1 Transfer function coefficient for correcting narrow-band velocity recording
ai bi i=0 1.044 984 1.000 370 i=1 −1.998 024 −1.999 999 i=2 0.956 990 0.999 629 表 2 本研究所用的日本同台站地震记录
Table 2 Earthquake records at the same station in Japan
编号 Hi-net
站点地震名称 发震时间(JST) 震中位置 震源
深度/kmM 方向 KiK-net
站点记录信噪比 年-月-日 时:分:秒 北纬/° 东经/° 速度 加速度 1 N.TSKH 茨城县地震 2008-05-08 01:45:00 36.227 141.607 51 7.0 EW IBRH19 72.45 48.55 2 N.TSKH 宫城地震 2011-04-07 03:54:00 38.20 141.92 66 7.1 EW IBRH19 23.97 12.10 3 N.TSKH 磐城县地震 2011-07-31 03:54:00 36.90 141.22 57 6.5 NS IBRH19 102.16 52.60 4 N.TSKH 福岛县地震 2021-02-13 08:00:00 37.73 141.70 55 7.3 EW IBRH19 85.30 55.51 表 3 本文方法和Nakata方法校正速度记录峰值对比
Table 3 Comparison of the peak value of the correction velocity recording by this method with that by the Nakata method
地震 原始Hi-net速度记录
峰值/(10−5 m·s−1)KiK-net积分速度记录
峰值/(10−5 m·s−1)校正的Hi-net速度记录峰值/(10−5 m·s−1) 本文方法 Nakata方法 茨城县地震 5.0 8.2 8.2 5.5 宫城地震 2.4 5.4 5.1 3.3 磐城县地震 3.9 4.3 4.1 3.5 福岛县地震 5.9 10.2 9.7 6.5 -
冯静,高孟潭,胥广银,陈鲲. 2013. Hi-net速度微分与KiK-net加速度对比研究[J]. 地震学报,35(6):902–913. doi: 10.3969/j.issn.0253-3782.2013.06.013 Feng J,Gao M T,Xu G Y,Chen K. 2013. A comparative study on Hi-net velocity differential and KiK-net acceleration[J]. Acta Seismologica Sinica,35(6):902–913 (in Chinese).
金星,马强,李山有. 2004. 利用数字化速度记录实时仿真位移与加速度时程[J]. 地震工程与工程振动,24(6):9–14. doi: 10.3969/j.issn.1000-1301.2004.06.002 Jin X,Ma Q,Li S Y. 2004. Real-time simulation of ground displacement and acceleration using digital velocity record[J]. Earthquake Engineering and Engineering Vibration,24(6):9–14 (in Chinese).
李永振,金震,梁永朵,于沈平,刘琳婷. 2012. 利用数字化加速度记录仿真速度及位移时程应用研究[J]. 防灾减灾学报,28(3):32–36. doi: 10.3969/j.issn.1674-8565.2012.03.006 Li Y Z,Jin Z,Liang Y D,Yu S P,Liu L T. 2012. Study of simulation speed and displacement time history recorded by digital acceleration[J]. Journal of Disaster Prevention and Reduction,28(3):32–36 (in Chinese).
马强,金星,李山有. 2003. 单自由度系统地震动力反应的实时计算方法[J]. 地震工程与工程振动,23(5):61–68. doi: 10.3969/j.issn.1000-1301.2003.05.011 Ma Q,Jin X,Li S Y. 2003. Real-time algorithm of seismic dynamic response for SDOF system[J]. Earthquake Engineering and Engineering Vibration,23(5):61–68 (in Chinese).
潘岳怡,牟剑英,谢夜玉,符衡,张清. 2019. 广西地区测震记录与强震动记录一致性分析:以广西北流MS5.2地震为例[J]. 华北地震科学,37(增刊):38–42. Pan Y Y,Mou J Y,Xie Y Y,Fu H,Zhang Q. 2019. Consistency analysis of seismic records and strong motion records in Guangxi:Taking the Guangxi Beiliu MS5.2 earthquake as an example[J]. North China Earthquake Sciences,37(S1):38–42 (in Chinese).
任枭,刘瑞丰,杨辉,徐志国,孙丽,黄志斌,邹立晔. 2009. 中国数字地震台网所使用的仪器及其传递函数的计算方法[J]. 地震地磁观测与研究,30(5):113–119. Ren X,Liu R F,Yang H,Xu Z G,Sun L,Huang Z B,Zou L Y. 2009. The status and transfer function of instruments deployed in China Digital Seismological Networks[J]. Seismological and Geomagnetic Observation and Research,30(5):113–119 (in Chinese).
盛谦,崔臻,刘加进,冷先伦. 2012. 传递函数在地下工程地震响应研究中的应用[J]. 岩土力学,33(8):2253–2258. doi: 10.3969/j.issn.1000-7598.2012.08.003 Sheng Q,Cui Z,Liu J J,Leng X L. 2012. Application study of transfer function for seismic response analysis of underground engineering[J]. Rock and Soil Mechanics,33(8):2253–2258 (in Chinese).
文雪康,袁莹,唐建明,刘胜,陈明春. 2020. 速度、加速度检波器在超深层地震勘探中应用效果分析:以川南赤水地区为例[J]. 地球物理学进展,35(4):1489–1496. doi: 10.6038/pg2020DD0343 Wen X K,Yuan Y,Tang J M,Liu S,Chen M C. 2020. Analysis and application of velocity and acceleration geophone in ultra deep seismic exploration:A case study of Chishui area in south Sichuan basin[J]. Progress in Geophysics,35(4):1489–1496 (in Chinese).
谢剑波. 2014. 地震记录的时间域反褶积、仿真及在地震计方位角相对测量中的应用[J]. 地球物理学报,57(1):167–178. doi: 10.6038/cjg20140115 Xie J B. 2014. Deconvolution,simulation of seismic records in the time domain and application in the relative measurements of seismometer orientation[J]. Chinses Journal of Geophysics,57(1):167–178.
杨笑梅,郭钦华. 2007. 二维复杂工程场址设计地震动的修正分析方法[J]. 世界地震工程,23(2):112–117. doi: 10.3969/j.issn.1007-6069.2007.02.018 Yang X M,Guo Q H. 2007. The modified method for determining design ground motions of 2D complex engineering site[J]. World Earthquake Engineering,23(2):112–117 (in Chinese).
俞言祥, 汪素云, 胡聿贤. 1999. 用宽频带数字记录计算长周期地震动反应谱[C]//中国地震学会成立20周年纪念文集. 北京: 地震出版社: 113–121. Yu Y X, Wang S Y, Hu Y X. 1999. Calculation of long-period ground motion response spectrum using broadband digital records[C]//Celebration of the 20th Anniversary of the Founding of China Seismological Society. Beijing: Seismological Press: 113–121 (in Chinese).
张宁,徐国林. 2022. 基于Hi-net速度记录计算地震反应谱[J]. 地震工程与工程振动,42(1):243–249. Zhang N,Xu G L. 2022. Calculation of earthquake response spectrum based on Hi-net velocity records[J]. Earthquake Engineering and Engineering Dynamics,42(1):243–249 (in Chinese).
周云耀,蔡亚先,吕永清,程骏玲. 2002. CT-1地震计传递函数测试仪的设计[J]. 大地测量与地球动力学,22(3):117–121. doi: 10.14075/j.jgg.2002.03.028 Zhou Y Y,Cai Y X,Lü Y Q,Cheng J L. 2002. Design of CT-1 transfer function test system of seismometer[J]. Journal of Geodesy and Geodynamics,22(3):117–121 (in Chinese).
Maeda T,Obara K,Furumura T,Saito T. 2011. Interference of long-period seismic wavefield observed by the dense Hi-net array in Japan[J]. J Geophys Res:Solid Earth,116(B10):B10303. doi: 10.1029/2011JB008464
Nakata N. 2013. Combination of Hi-net and KiK-net data for deconvolution interferometry[J]. Bull Seismol Soc Am,103(6):3073–3082. doi: 10.1785/0120130101
-
期刊类型引用(4)
1. 李军红,崔宁. 基于拉普拉斯变换的常微分方程课程思政探究. 内江科技. 2025(01): 131-133 .  百度学术
百度学术
2. 吴文波,杨耀宁,禹谢华. 多层卷积神经网络在图像目标标注中的应用. 计算机仿真. 2025(02): 243-247 .  百度学术
百度学术
3. 宋小艳,刘菲菲,李彦超,刘伟东. 新能源汽车横摆角速度无静差跟踪鲁棒控制. 机械设计与制造. 2025(04): 163-168 .  百度学术
百度学术
4. 王锋. 港口桥式起重机械负载防摇控制方法研究. 自动化与仪表. 2024(01): 61-65 .  百度学术
百度学术
其他类型引用(0)















 下载:
下载: