Ground motion variability of a mountain-canyon site near a strike-slip fault considering uncertainty of source
-
摘要:
由于震源不确定性客观存在且对近地表地震动特性产生显著影响，尝试将乘子降维法应用至考虑震源不确定性的近断层复杂场地地震动变异性求解，将不确定性分析问题转换为有限次确定性分析，获得与蒙特卡洛模拟一致的地震动参数统计矩，其中单次确定性分析采用边界元法模拟断层破裂—传播路径—场地放大的整个物理过程。基于乘子降维法，以近走滑断层高山峡谷场地为例，分析了在近断层效应、场地效应和震源不确定性三者耦合作用下的场地地震动峰值加速度（PGA）、峰值速度（PGV）的空间分布变异性，以及关键地表点谱加速度（SA）的统计值。结果表明：乘子降维法适用于含震源参数不确定性的近断层复杂场地随机地震动求解；高山峡谷地形对地震波的散射效应叠加近断层效应后可引起断层上盘PGA和PGV均值的显著增大，可放大至两倍以上；震源不确定性经由场地传播，导致地表地震动变异性，PGA变异性强于PGV；考虑一倍均方差时，震源不确定性对结构反应的影响可达30%以上，大跨度工程结构还应考虑近断层地震动变异性的空间分布差异。
Abstract:A large number of railways and highways in the western part of China are located in near-fault mountain-canyon sites. The bridges and tunnels account for a large proportion due to the complex topography, and many important projects are faced with severe seismic risk. The ground motions in the near-fault mountain-canyon sites are very complex. On the one hand, velocity pulses and large vertical amplitudes are typical characteristics of the ground motions in near-fault regions; on the other hand, the topography of mountains and canyons leads to amplification and non-uniformity effects on ground motions. For example, the 1992 Hualien earthquake records show that the peak ground acceleration on the sidewalls of the Feitsui canyon in Taiwan is 2.69 times than that of the canyon bottom. In the 2008 Wenchuan earthquake, the peak ground acceleration in the east-west direction at the top of Xishan Mountain in Zigong is 1.77 times than that at the foot of the mountain. Theoretical and numerical analyses reveal that the physical essence of the amplification and non-uniformity effect is the scattering and local focusing of seismic waves by the topography of mountain-canyon sites.
In addition, the current technologies of geophysical prospecting make it difficult to finely determine the physical parameters of faults, interface slip characteristics, etc. It means that the fault rupture process has uncertainty. Based on the previous studies, the uncertainty of source existed objectively and had a significant impact on the characteristics of near-surface ground motions. In this study, it is an issue of quantifying uncertainty in ground motion parameters at near-fault mountain-canyon sites. Monte Carlo simulations and logic trees are commonly used to quantify the uncertainty in this problem. The main purpose is to construct different seismic scenarios, focusing on comparing the standard deviation of the spatial distribution of ground motions in the actual regional site with the standard deviation in the ground motion prediction model. It is worth pointing out that the Monte Carlo simulation has low efficiency to carry out the multidimensional uncertainty analysis. Besides, the simulation of ground motions in near-fault mountain-canyon sites needs to take into account near-fault and topography effects. Meanwhile, the uncertainty of the seismic source will cause random scattering of the seismic waves in mountain-canyon sites, which will lead to the variability of the ground motion parameters at various surface locations. However, the existing studies have not explored the propagation mechanism in depth.
In this paper, the multiplicative dimensional reduction method （M-DRM） is applied to solve the ground motion variability of complex sites near-fault considering the uncertainty of the source. The uncertainty analysis problem is converted into a finite deterministic analysis to obtain statistical moments of ground motion parameters consistent with Monte Carlo simulation. The deterministic analysis uses the boundary element method to simulate the entire physical process. Based on this method, the mountain-canyon site near a strike-slip fault was discussed as an example. The spatial distribution variability of the peak acceleration （PGA） and peak velocity （PGV） under the coupling of near-fault effect, site effect and source epistemic uncertainty was analyzed, as well as statistical values of spectral acceleration （SA） at some surface points.
The results indicated that the M-DRM is applied to the ground motion variability problem of near-fault mountain-canyon sites caused by seismic source uncertainty, which has higher computational efficiency compared with the conventional Monte Carlo simulation. This method can be used for the stochastic ground motion simulation of the complex sites based on the phylsical model and considering the uncertainty of seismic source. When there is a mountain-canyon topography in the near-fault region, the coupling of the near-fault effect and the local site effect causes a significant amplification of the mean values of the PGAs at the sites, which shows significant spatial variations, especially in the canyon. It can be up to 2.69 times that of the result without the local topography. The mean values of the variability of the PGVs at the different surface points are smaller than those of the PGAs. The structural periods corresponding to the maximum values of the surface ground motion acceleration response spectrum are basically the same under the conditions with and without mountain-canyon topography. The seismic source uncertainty is propagated through the site, which is finally manifested in the spatial distribution variability of PGAs and PGVs. Due to the different energy distributions of ground motion acceleration and velocity, there are differences in the variability of PGAs and PGVs. PGAs have larger coefficients of variation. The variability of PGAs and PGVs is different from that of a single parameter under different rupture scenarios when both the asperity intensity and the rupture velocity uncertainty are taken into account. However, the results of the acceleration response spectrum are more complicated. The variability of the structural response at different locations may be lower or higher than the superposition of single-parameter uncertainty variability, and it is affected by the location of the asperity.
-
Keywords:
- site effect /
- near-fault effect /
- source uncertainty /
- boundary element method
-
引言
我国西部大量铁路、公路位于近断层高山峡谷场地，由于地形复杂，桥隧比也相对较高，例如，川藏铁路雅林段和雅康高速公路桥隧比分别高达94%和82% （袁飞云，王剑波，2018；陈畅等，2019）。而西部大部分地区活动断层分布密集，近断层高山峡谷地区的诸多重要工程面临严峻的地震风险考验。如2008年汶川MS8.0地震，位于近断层高山峡谷地区的桥梁破坏尤其严重（雷涛等，2010）。2022年青海门源MS6.9地震中受损严重的硫磺沟大桥、大梁隧道同样位于近断层高山峡谷地区。因此，科学的构建地震动场是评估此类地区工程灾害风险的关键。
近断层高山峡谷场地的地震动场十分复杂。一方面，近断层地震动特性与远场地震动差异显著，存在上盘效应、方向性效应、大速度脉冲、强竖向地震动等特点（罗全波等，2019；陈笑宇等，2021；李宁等，2022）；另一方面，高山峡谷地区地震动呈现显著的空间变化和局部放大现象，如1992年花莲地震台站记录显示，台湾翡翠河谷侧壁的地震峰值加速度（peak ground acceleration，缩写为PGA）较谷底放大了2.69倍（Huang，Chiu，1995）；2008年汶川MS8.0地震，自贡西山山顶东西向PGA较山脚放大了1.77倍（王海云，谢礼立，2010；王伟等，2015）。理论分析表明，地震动放大效应的关键成因在于高山峡谷地形对地震波的散射和局部聚焦（巴振宁等，2020；李郑梁等，2021）。
此外，现有地球物理勘探技术还难以精细地确定断层物性参数、界面滑移特征等（张冬锋，2019）。Yamada等（2011）和Fortuño等（2021）分别基于有限差分法和离散波数法研究了震源不确定性对地表地震动空间分布变异性的影响，研究参数包括凹凸体位置、凹凸体面积、破裂点位置和破裂速度，但模拟频带为0—1 Hz，无法反映中高频地震动变异性。Ameri等（2009）、Iwaki等（2017）基于混合模拟法在宽频带内研究了震源不确定性在地震波传播过程中的传递过程，结果显示部分地表点地震动PGA和峰值速度（peak ground velocity，缩写为PGV）变异性可放大3倍（Iwaki et al，2017），且破裂速度对PGV的影响十分显著（Ameri et al，2009）。在Hartzell等（2011）和Cao等（2019）的研究中破裂速度同样作为关键参数被重点讨论。上述研究均采用蒙特卡洛法或逻辑树构建不同发震情景，侧重将实际区域场地地震动空间分布标准差与地震动衰减关系中标准差进行对比。然而，蒙特卡洛法开展多维不确定分析计算成本较高，且近断层高山峡谷场地地震动模拟需综合考虑近断层效应和地形效应，同时震源不确定性将引起高山峡谷场地对地震波的随机散射，导致地表空间变化地震动具有变异性，且此变异性与地表点位置有关，本质上仍为不确定性在系统中的传播问题，尚未有研究对于其传播机制进行过深入探讨。
鉴于此，本文以近断层高山峡谷场地（本文认为断层距小于20 km为近断层区域）、走滑断层类型为例，研究震源不确定性引起的此类场地地震动空间分布变异性，重点揭示不确定性在高山峡谷场地中的传递过程及其对近断层复杂场地效应耦合作用的影响规律。选择凹凸体位置、凹凸体强度和断层破裂速度作为不确定因素（Yamada et al，2011；Bjerrum et al，2013；Iwaki et al，2017），拟通过构建不同破裂情景考虑凹凸体位置不确定性，选择随机参数、采用乘子降维法考虑凹凸体强度、破裂速度不确定性以提高计算效率，将含震源不确定性的近走滑断层高山峡谷场地地震动模拟问题转化为一系列确定性分析，并基于运动学震源模型和边界元法求解断层破裂—近地表复杂场地物理模型，以PGA，PGV和谱加速度（spectral acceleration，缩写为SA）为指标考察高山峡谷场地地震动空间分布变异性。以期为近断层复杂场地随机地震动场模拟提供一种有效方法，为近断层高山峡谷场地效应评估提供部分依据。
1. 考虑震源不确定性的近走滑断层高山峡谷场地地震动模拟方法建立
1.1 计算模型
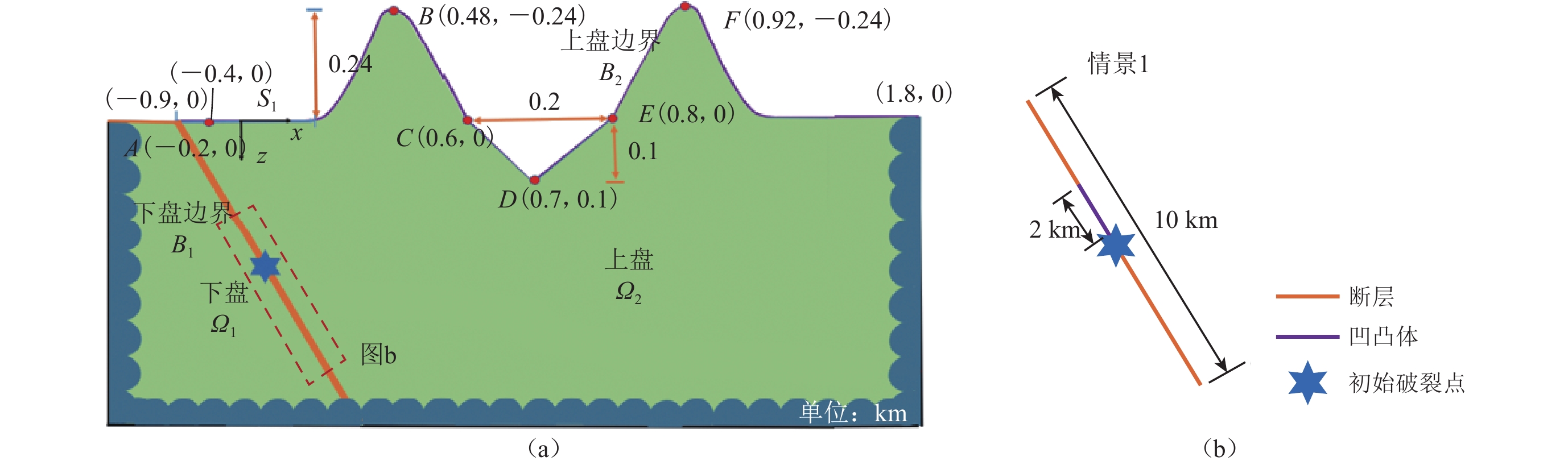
高山峡谷地形位于走滑断层上盘（图1），断层平行于y轴（出平面方向）。下盘、上盘分别记为Ω1和Ω2，高山峡谷地形与断层的距离记为S1；断层长度为Lf，垂直投影为Hf，断层倾角为α，山谷的宽度和深度分别为Wb和Hb，山的宽度和高度分别为Wm和Hm，本文所建立的走滑断层运动学计算模型如图1所示。考虑走滑断层存在不均匀错动（二维计算模型中走滑断层错动为出平面问题），地震波传播至近地表时，高山峡谷地形对其产生散射效应，导致地表地震动呈现非均匀空间分布。当进一步考虑震源不确定性时，此不确定性经由场地传播，导致地表地震动变异性也表现出非均匀特征。
![]()
图 1 近走滑断层高山峡谷场地计算模型示意图Figure 1. Calculation model of mountain-canyon site near a strike-slip fault1.2 高山峡谷场地对地震波的散射（频域分析）
在边界离散单元上施加满足波动方程基本解的虚拟荷载，间接构造地震波场。根据单层位势理论，离散边界元位移可由Somigliana积分公式导出（Sánchez-Sesma，Campillo，1991），表达式为
$$ {u_i} （ x ） ＝ \int_\varOmega {{G_{ij}} （ y \text{，} \xi ） } {f_i} （ \xi ） {\mathrm{d}}{\varOmega _\xi } ＋ \int_B {{G_{ij}} （ x \text{，} \xi ） } {\var\phi _j} （ \xi ） {\mathrm{d}}{B_\xi } \text{，}\qquad i\text{，} j＝x\text{，} {\textit{z}}\text{，} $$ (1) 式中：ui（x）表示任意点x处i方向上的位移；Gij（x，ξ）是位移的格林函数，即在坐标点ξ上施加单位力在j方向上所引起的坐标点x上i方向上的位移；fi （ξ）表示体力在i向的分量；ϕj（ξ）dBξ为各计算单元在边界处的分布力，即虚拟荷载密度值；$\varOmega $为上、下盘。
在弹性范围内，可结合其积分表达式和胡克定律求解应力，边界B上x点的i向应力可写为
$$ {\sigma _i} （ x ） ＝ \int_\varOmega {{T_{ij}} （ y \text{，} \xi ） } {f_j} （ \xi ） {\mathrm{d}}{\varOmega _\xi } ＋ \int_B {{T_{ij}} （ x \text{，} \xi ） } {\phi_j} （ \xi ） {\mathrm{d}}{B_\xi } ＋ \frac{1}{2}{\phi _i} （ x ） \text{，} $$ (2) 式中：Tij （x，ξ）为牵引力的格林函数，即在坐标点ξ上施加单位力j方向所引起的坐标点x上i方向的牵引力；fj（ξ）表示体力在j向的分量。
近断层高山峡谷场地边界条件为：① 半空间、山体、峡谷表面应力为零；② 半空间与峡谷交界面应力和位移连续；③ 断层面应力连续；④ 位移在y轴方向上差值为d0。具体数学表达式可写为（Liu et al，2022）
$$ \left\{ \begin{gathered} \sigma _i^{ {B_{1 \text{，} }}\rm{e} } （ x ） ＝ 0， \\ \sigma _i^{ {B_{2 \text{，} }}\rm{e} } （ x ） ＝ 0， \\ \sigma _i^{ {B_{1 \text{，} }}\rm{f} } （ x ） ＝ \sigma _i^{ {B_{2 \text{，} }}\rm{f} } （ x ） ， \\ u_x^{ {B_{1 \text{，} }}\rm{f} } （ x ） ＝ u_x^{ {B_{2 \text{，} }}\rm{f} } （ x ） ， \\ u_y^{ {B_{1 \text{，} }}\rm{f} } （ x ） － u_y^{ {B_{2 \text{，} }}\rm{f} } （ x ） ＝ {d_0}， \\ u_{\textit{z}}^{ {B_{1 \text{，} }}\rm{f} } （ x ） ＝ u_{\textit{z}}^{ {B_{2 \text{，} }}\rm{f} } （ x ） ， \\ \end{gathered} \right. $$ (3) 式中：上标B1和B2表示场点的子域边界，上标e和f表示自由表面和断层面，下标x，y，z表示方向。
结合式（1）—（3），可得到一个线性方程组。数值求解时，一般将连续积分转化为离散形式，即将每个计算域边界划分为有限个计算单元，并对每个计算单元施加虚拟均布荷载。当源点与场点不重合时，可采用高斯积分直接求解应力与位移的全空间动态格林函数；当源点与场点重合（x＝y）时，采用解析法求解，以避免高斯积分奇异性。由于篇幅有限，具体求解步骤请参考文献（Liu et al，2021），此处不再赘述。
1.3 运动学震源模型
本文采用运动学震源模型考虑走滑断层破裂过程，其表达式为（Haskell，1964）：
$$ D （ t ） ＝ {D_\infty }G \left( t － {{\frac{d_\xi }{v}} }\right)\text{，} $$ (4) 式中： D（t）为断层单元点在源点dξ处在t时刻的位错量；D∞为上下盘最终位错量；dξ为断层单元到初始破裂位置的距离；v为破裂速度；
$$ G （ t ） ＝ \left\{ {\begin{array}{*{20}{c}} 0&{t ＜ 0 \text{，} } \\ {{\dfrac {{\textit{t}}} {T}}}&{0 ＜ t ＜ 0 \text{，} } \\ 1&{t ＞ 0 \text{，} } \end{array}} \right. $$ (5) 其中，T为震源上升时间。
每个子断层破裂的时间差由频域中相应的相位差表示。为表达近断层地震动频谱特征，本文将初始位移引起的各子断层自由振动近似替换为均值为0、方差为1的高斯白噪声，并加入一定的衰减系数，表示为G1（t），最终断层滑移函数可表示为：
$$ D （ t ） ＝ {D_\infty }\left[ {G \left(t － {{\dfrac{d_\xi }{v}} } \right) ＋ {G_1} （ t ） } \right] . $$ (6) 对上述滑移时间函数进行傅里叶变换获得频域值，作为输入边界条件。根据单层势能理论，通过对各边界单元施加虚拟均匀荷载，间接构造出上下盘错位引起的地震波场。结合边界条件在频域内建立虚拟荷载的边界积分方程，求解各边界单元上的分布力。根据波的叠加原理，构建各计算单元在断层错位作用下的地震波场。在频域分析基础上，利用傅里叶逆变换可获得地表地震响应时域解。
1.4 不确定性分析方法
不确定震源参数可被视为一系列的随机变量，而具有震源参数不确定性的高山峡谷场地随机地震响应可写为
$$ \beta ＝ g （ \varPhi ） \text{，} $$ (7) 式中：Ф和β分别表示不确定震源参数和场地随机地震响应，g（•）表示Ф和β之间的映射关系，此关系通常具有显著的非线性和耦合特征。
基于乘子降维法，高山峡谷场地随机地震响应β可近似表示为（Zhang，Pandey，2013）：
$$ \beta ＝ g （ \varPhi ） {\text{≈}} g_0^{ （ 1 － n ） } \; { \prod\limits_{i ＝ 1}^n} {{g_i}} （ {\varPhi _i} ） \text{，} $$ (8) 式中：n代表震源不确定参数的数量；gi （Φi）为包含第i个随机变量的场地随机地震响应函数，可表示为
$$ {g_i} （ {\varPhi _i} ） ＝ g （ {c_1} \text{，} \cdots \text{，} {c_{i － 1}} \text{，} {\varPhi _i} \text{，} {c_{i ＋ 1}} \text{，} \cdots \text{，} {c_n} ） \text{，} $$ (9) 式中：ci-1为第i−1个随机变量的均值。g0表示所有随机变量取均值时场地地震响应，可写为
$$ {g_0} ＝ g （ {c_1} \text{，} {c_2} \text{，} ...{c_n} ） . $$ (10) 根据式（8）—（10），可将含n个随机变量的不确定性问题转化为n个含单一随机变量的不确定性问题和一个确定性问题。
当不确定震源参数相互独立时，场地随机地震响应β的m阶矩阵可写为（Zhang et al，2018）
$$ {\boldsymbol{E}} ［ {{\beta ^m}} ］ {\text{≈}} {\boldsymbol{E}}\left[ {{{ \left( {g_0^{ （ 1 - n ） } {\prod\limits _{i ＝ 1}^n {{g_i}}} （ {\phi _i} ） } \right)}^m}} \right] {\text{≈}} g_0^{m （ 1 - n ） } {\prod\limits_{i ＝ 1}^n} {{\boldsymbol{E}}\left[ {{{ {g^m_i} （ {\varPhi _i} ） }}} \right]}. $$ (11) 由式（11），场地随机地震响应β均值和均方差可分别表示为：
$$ {\mu _\beta } ＝ {\boldsymbol{E}} ［ \beta ］ ＝ g_0^{ （ 1 - n ） } {\prod\limits_{i ＝ 1}^n }{{\boldsymbol{E}} ［ {{g_i} （ {\varPhi _i} ） } ］ } \text{；} $$ (12) $$ \sigma _\beta ^2 ＝ {\boldsymbol{E}} ［ {{\beta ^2}} ］ － { （ {\boldsymbol{E}} ［ \beta ］ ） ^2} ＝ g_0^{2 （ 1 － n ） } {\prod\limits_{i ＝ 1}^n} {\boldsymbol{E}} {{{ ［ {g_i} （ {\varPhi _i} ） ］ }^2}} － {\left\{ {g_0^{ （ 1 － n ） } {\prod\limits_{i ＝ 1}^n }{{\boldsymbol{E}} ［ {{g_i} （ {\varPhi _i} ） } ］ } } \right\}^2} . $$ (13) β的m阶矩可基于高斯正交积分近似求解，其表达式为（Zhang，Pandey，2013）：
$$ {\boldsymbol{E}}\left[ {{{ （ {g_i} （ {\varPhi _i} ） ） }^m}} \right] {\text{≈}} \sum\limits_{j ＝ 1}^L {{w_j}} { （ {g_i} （ {\varPhi _j} ） ） ^m} \text{，} $$ (14) 式中：L为高斯正交点数量（一般取L＝5即可满足精度要求），Фj和wj （j＝1，2，3，···，L）分别为第i个随机变量取值和相应的权重，gi （Фj）为确定性震源参数下高山峡谷场地确定性地震响应。最终，具有n个不确定震源参数的近走滑断层高山峡谷场地随机地震动求解问题被转化为Ln＋1次确定性分析。
2. 方法验证
2.1 边界元法验证
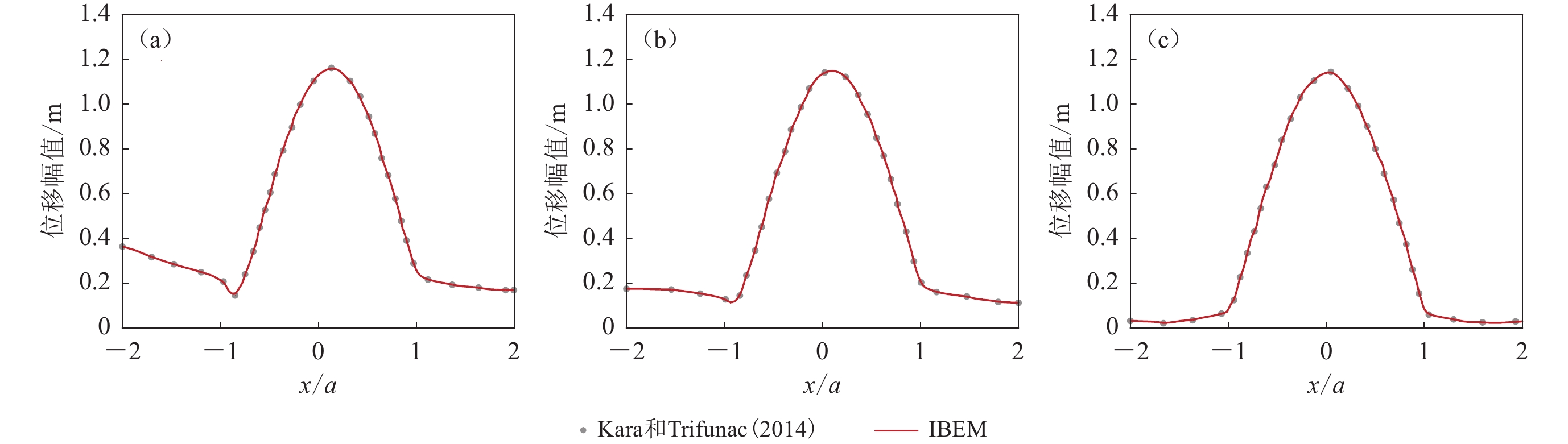
为了验证本研究方法模拟近走滑断层高山峡谷场地地震动的准确性，本节将1.2节方法所求结果与文献（Kara，Trifunac，2014）结果进行对比，计算模型包括a，b，c三个断层，断层形状为圆弧状，具体模型见文献（Kara，Trifunac，2014）。断层中心与半空间地表左侧的夹角分别为π/24，π/4，π/2，断层长度均为π/12，圆弧断层半径是沉积谷地半径的3倍。假设走滑断层错动量为1 m，基岩半空间部分与沉积谷地部分的波速比为3，密度比为1.2，无量纲频率为η＝2a1/λ＝0.25，其中a1和λ分别为半空间峡谷半径和剪切波波长。计算模型和参数取值参见文献（Kara，Trifunac，2014）。不同断层倾角条件下场地地表位移幅值对比如图2所示。由图可知，在三种断层倾角条件下，本文方法求取结果与Kara和Trifunac （2014）的结果具有良好的一致性，表明了本文方法的适用性。
![]()
图 2 不同断层角度下本文方法与Kara和Trifunac （2014）方法所得地表位移结果对比（a） 倾角为π/24；（b） 倾角为π/4；（c） 倾角为π/2Figure 2. Comparison of surface displacement results obtained by the proposed method and the method in reference Kara and Trifunac （2014） under different fault angles（a） Dip angle of π/24；（b） Dip angle of π/4；（c） Dip angle of π/22.2 边界元法数值稳定性验证
本节验证边界元方法的数值稳定性，验证模型与2.1节相同。假设断层破裂无量纲频率为η＝0.25，断层面离散点数N分别取为600，1 000和1 400，半空间地表离散点数L分别取为561，801和1 041。结果表明，断层面和地表离散点数增大时，地表各位置位移幅值收敛性良好（表1），相对误差可控制在10−3数量级以内，表现出良好的数值稳定性。
表 1 边界元法数值稳定性验证 （η＝0.25）Table 1. Numerical stability verification of boundary element method （η＝0.25）x/a 边界元法参数 解析解 N＝600，
L＝561N＝1 000，
L＝801N＝1 400，
L＝1 041−2.0 0.070 3 0.070 7 0.070 4 0.070 4 −1.5 0.091 3 0.090 9 0.090 8 0.090 8 −1.0 0.518 9 0.519 9 0.522 6 0.522 6 −0.5 0.495 0 0.516 7 0.551 2 0.551 2 0.5 0.744 8 0.749 8 0.754 7 0.754 7 1.0 0.494 2 0.547 7 0.551 2 0.551 2 1.5 0.097 8 0.099 2 0.100 3 0.100 3 2.0 0.083 3 0.084 0 0.084 6 0.084 6 2.3 不确定性分析方法验证
本节旨在验证乘子降维法代替蒙特卡洛模拟求解考虑震源不确定性的近走滑断层高山峡谷场地随机地震动的可靠性。定义凹凸体位错量与断层平均位错量的比值为凹凸体强度（Yamada et al，2011），将断层破裂速度、凹凸体强度取为随机变量。由2.3节可知，乘子降维法共需进行11次数值求解，远低于蒙特卡洛模拟所需计算样本数量（验证中蒙特卡洛模拟进行了1 000次数值求解）。
计算模型（图3）中，两侧山体形状符合高斯函数，高度为240 m；山间存在一宽度200 m、深度100 m的V型峡谷；断层延申至地表，长度为10 km，倾角为45°，断层距S1为1 km。假定该高山峡谷复杂场地为各向同性、均质的弹性体，密度ρ＝2 500 kg /m3，泊松比μ＝0.25，剪切波速CS＝3 200 m /s。断层初始破裂点位于断层中间位置，凹凸体位于初始破裂点之上，长度为2 km。凹凸体强度均值取为1，均方差为0.2，其余8 km位错均为0.5 m，破裂速度均值为2 720 m/s，均方差为136 m/s，假定两者均满足正态分布（Yamada et al，2011）。按张冬锋（2019）所给经验公式，当所有参数取均值时，地震矩约为7.76×1017 N·m，震级约为6.0。
![]()
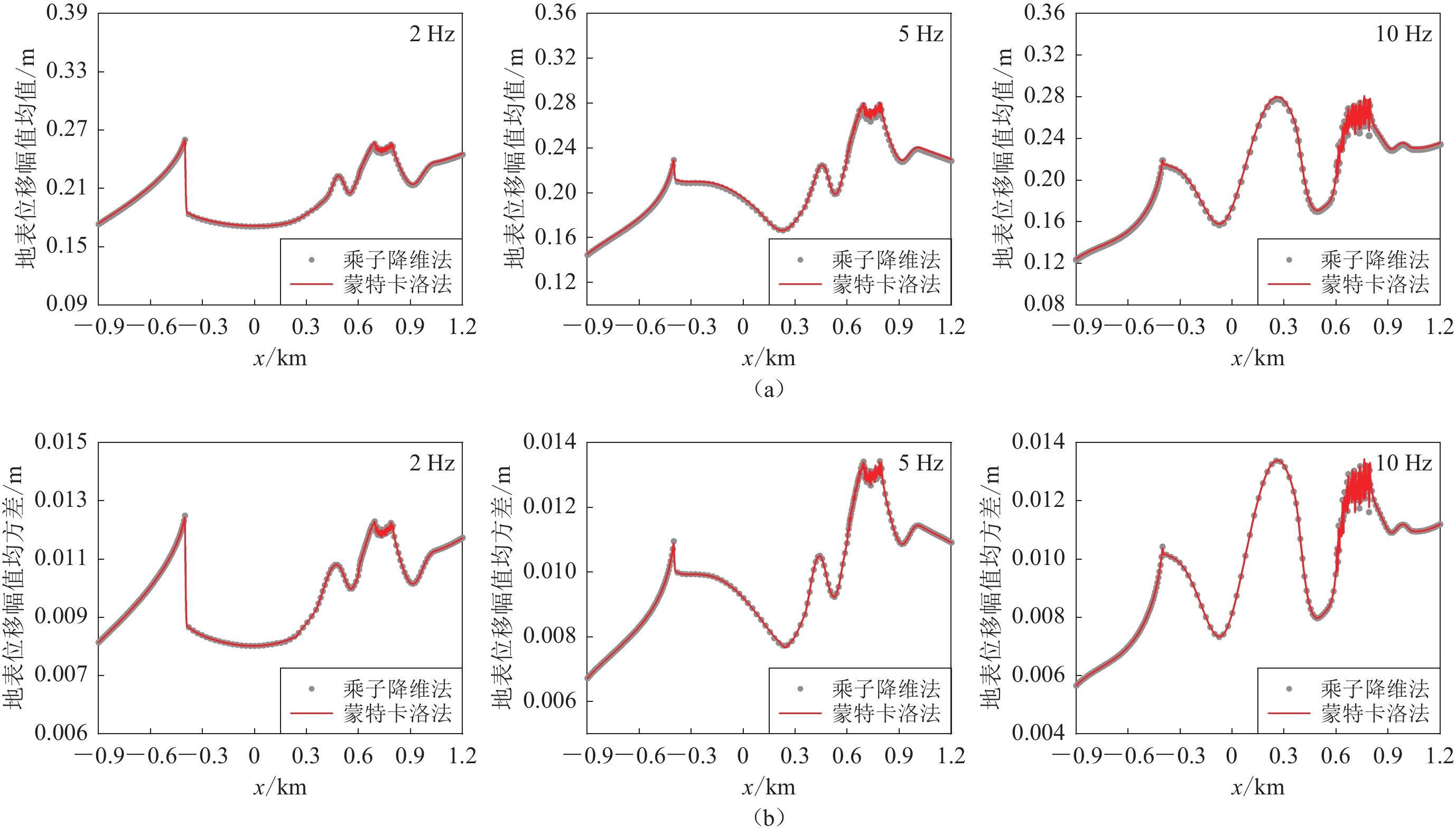
图 3 用于验证的计算模型（a） 场地计算模型，图中A，B，C，D，E，F为地表参考点；（b） 断层模型Figure 3. Calculation model for verification（a） Calulation model of sites，and A，B，C，D，E and F are the surface reference point；（b） Fault model图4给出了频率f为2 Hz，5 Hz和10 Hz时（频域分析）高山峡谷场地地表位移幅值均值和均方差，图中x表示水平向地表位置坐标，坐标轴位置见图3a。由图4可知，基于乘子降维法求解的场地地表位移幅值均值、均方差与蒙特卡洛模拟结果具有很好的一致性，表明乘子降维法可用于求解含不确定震源参数的复杂场地随机地震动，相较蒙特卡洛模拟，可更好地兼顾计算精度和计算效率。
![]()
图 4 不同入射频率波下高山峡谷场地位移幅值均值（a）及其均方差（b）Figure 4. Mean values of displacement amplitude of mountain-canyon site （a） and mean square deviation of displacement amplitude of mountain-canyon site （b） under different incident frequency waves3. 算例与分析
3.1 计算模型与参数
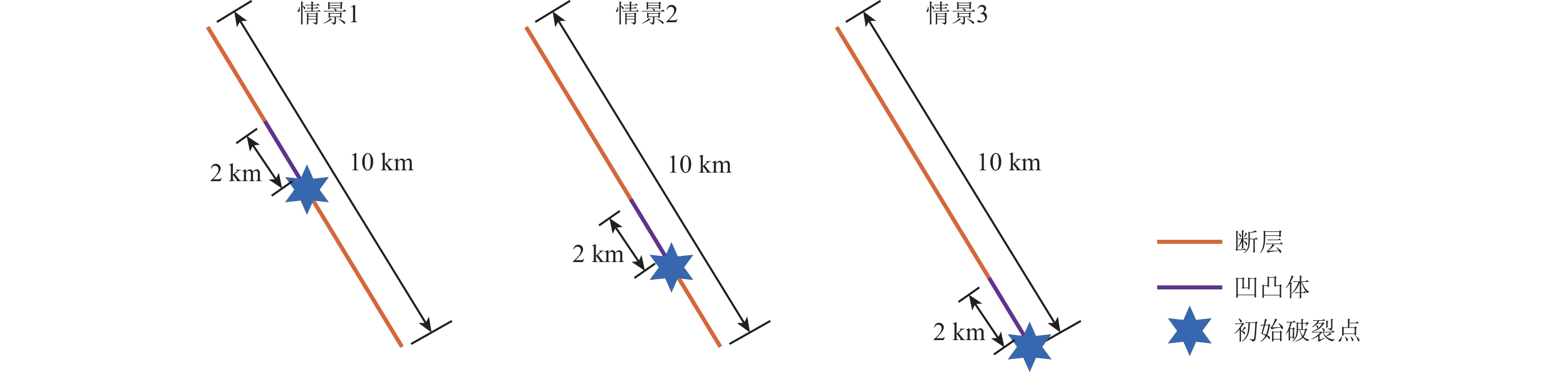
针对图3所示的二维计算模型，开展考虑震源不确定性的近走滑断层高山峡谷场地地震动变异性研究，考察指标包括地表PGA和PGV变异性空间分布、地表关键点谱加速度和速度脉冲，同时求解不存在高山峡谷地形地震动结果作为对比。考虑的震源不确定性包括破裂速度、凹凸体强度和位置，其中前两者作为随机变量考虑（同2.2节），通过构建三种破裂情景考虑凹凸体位置不确定性（图5），频率范围为0—10 Hz （Bjerrum et al，2013），初始破裂点位置均位于凹凸体下方（Yamada et al，2011）。其它计算模型参数取值与2.2节一致。
![]()
图 5 考虑凹凸体位置不确定性的破裂情景Figure 5. Rupture scenarios considering uncertainty of asperity positions3.2 PGA空间分布变异性
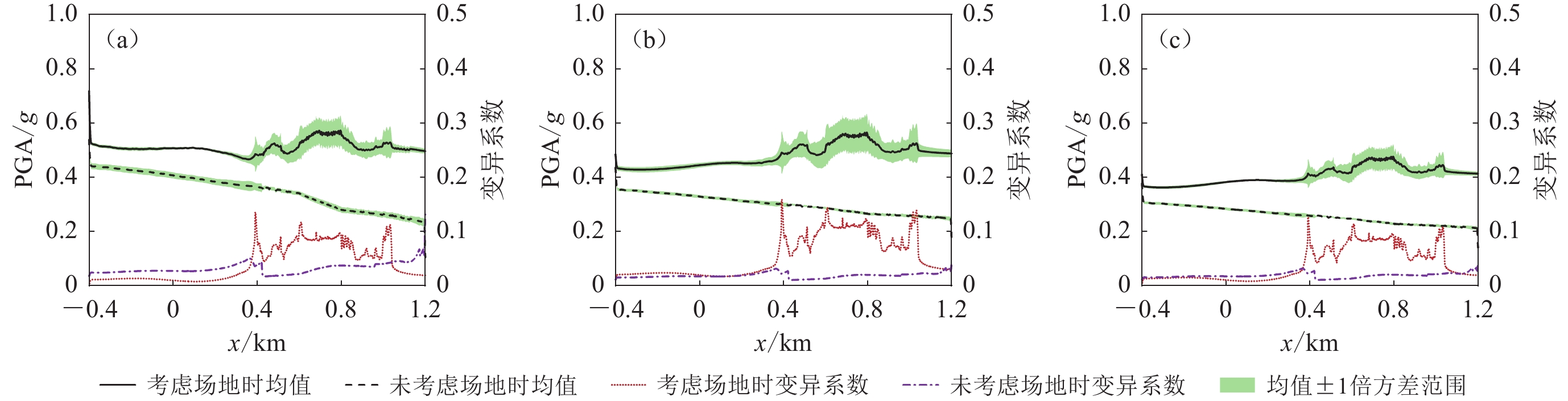
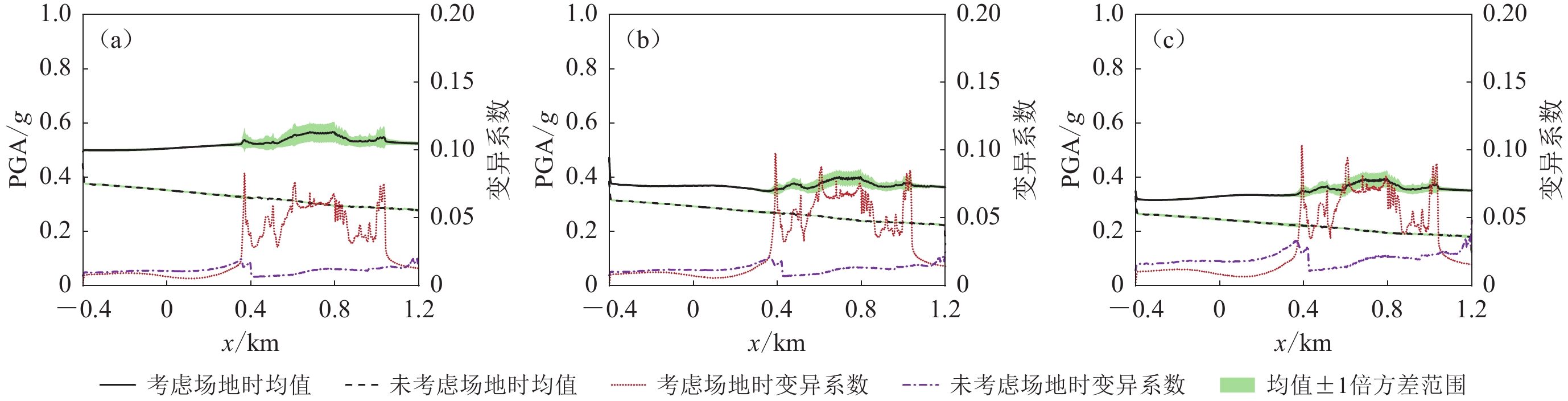
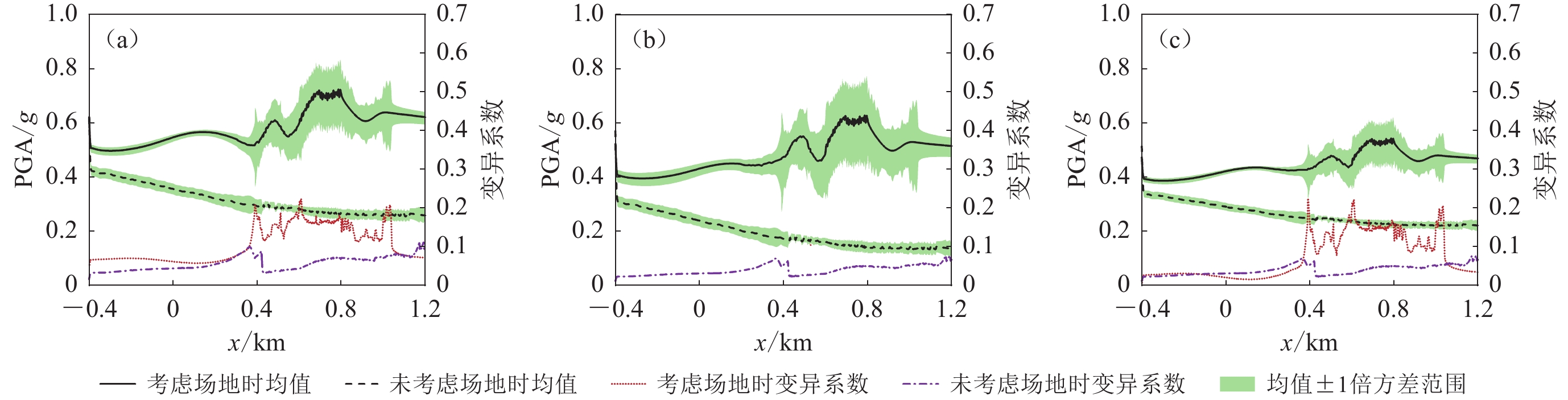
分别考虑凹凸体强度、破裂速度不确定性时近断层高山峡谷场地PGA空间分布的均值和均方差，如图6和图7所示，同时，定义变异系数为PGA均方差与均值之比，其空间分布也一并给出，以考察PGA空间分布变异性。由图可知，分别考虑凹凸体强度和破裂速度不确定性时，PGA均值分布存在差异，考虑凹凸体强度不确定性时场地的PGA振荡更为显著。而高山峡谷地形可导致PGA均值显著增大，且场地放大效应与地表空间位置有关，当考虑凹凸体强度不确定性时，三种破裂情景下峡谷谷底（D点）的PGA均值可放大至2.01，1.97，1.82倍，山顶（B点）的PGA均值可放大至1.76，1.68，1.47倍，表现出显著的空间差动效应，因此，近断层大跨结构抗震分析地震动参数确定应同时考虑近断层效应和场地效应。
![]()
图 6 考虑凹凸体强度不确定性时近断层场地PGA空间分布变异性（a） 破裂情景1；（b） 破裂情景2；（c） 破裂情景3Figure 6. Spatial distribution variability of PGAs in the near-fault site considering asperity intensity uncertainty（a） Rupture scenario 1；（b） Rupture scenario 2； （c） Rupture scenario 3![]()
图 7 考虑破裂速度不确定性时近断层场地PGA空间分布变异性（a） 破裂情景1；（b） 破裂情景2；（c） 破裂情景3Figure 7. Spatial distribution variability of PGAs in the near-fault site considering rupture velocity uncertainty（a） Rupture scenario 1；（b） Rupture scenario 2； （c） Rupture scenario 3当不存在局部场地时，近断层场地PGA变异性受地表点距断层位置影响较小；当考虑凹凸体强度不确定性时，三种破裂情景下大部分地表点PGA变异系数在0.02以内，最大值为0.05，均远小于凹凸体强度变异系数0.2；当考虑破裂速度不确定性时，地表点PGA变异系数亦小于破裂速度变异系数0.05。结果表明，凹凸体强度或破裂速度不确定性将引起场体的PGA不确定性，但该不确定性低于震源参数不确定性。
但当存在高山峡谷地形时，高山峡谷地表的PGA变异系数随地表点位置振荡剧烈，由图6和图7也整体可以看出，高山峡谷地表的PGA变异性较其它地表点结果更为显著。当破裂速度具有不确定性时，高山峡谷地表PGA变异系数可能大于破裂速度变异系数，尤其是峡谷地表，但凹凸体强度具有不确定性时，尽管高山峡谷地表的PGA变异系数较无起伏地形时明显增大，但仍低于设定的凹凸体强度变异系数，最大值为0.12，详见图6，7中破裂情景2的地表x＝0.4 km处；当考虑凹凸体强度或破裂速度不确定性时，不同破裂场景下PGA变异系数空间分布基本一致，数值接近；上述结果表明，震源不确定性经由场地传播，与起伏地形对地震波的散射效应综合作用后，将导致高山峡谷地表PGA出现了较为显著的变异性。
图8进一步给出了同时考虑凹凸体强度和破裂速度不确定性时近断层高山峡谷场地PGA空间分布均值、均方差和变异系数。结合图6，7和8可知，在无局部地形时，同时考虑凹凸体强度和破裂速度不确定性对应的PGA变异性空间分布趋势与单一参数不确定性结果类似，变异系数较单一参数不确定性对应的结果之和近似，例如，当初始破裂点深度为7.5 km （破裂情景2）时，峡谷谷底（D点）、山顶（B点、F点）PGA变异系数分别为0.054，0.028和0.053，而凹凸体强度（破裂速度）不确定性对应D点，B点和F点的结果为0.033 （0.013），0.018 （0.007），0.036 （0.013）。当近断层区域存在高山峡谷起伏地形时，三种破裂情景下，同时考虑凹凸体强度和破裂速度不确定性对应的PGA变异性与单一参数不确定性结果叠加也基本一致，例如，当初始破裂点深度为10 km （破裂情景3）时，峡谷谷底（D点）、山顶（B点、F点） PGA变异系数分别为0.158，0.137和0.124，而凹凸体强度、破裂速度不确定性对应的变异系数之和为0.162，0.134，0.120。
![]()
图 8 考虑凹凸体强度和破裂速度不确定性时近断层场地PGA空间分布变异性（a） 破裂情景1；（b） 破裂情景2；（c） 破裂情景3Figure 8. Spatial distribution variability of PGAs in the near-fault site considering asperity intensity and rupture velocity uncertainty（a） Rupture scenario 1；（b） Rupture scenario 2；（c） Rupture scenario 33.3 PGV空间分布变异性
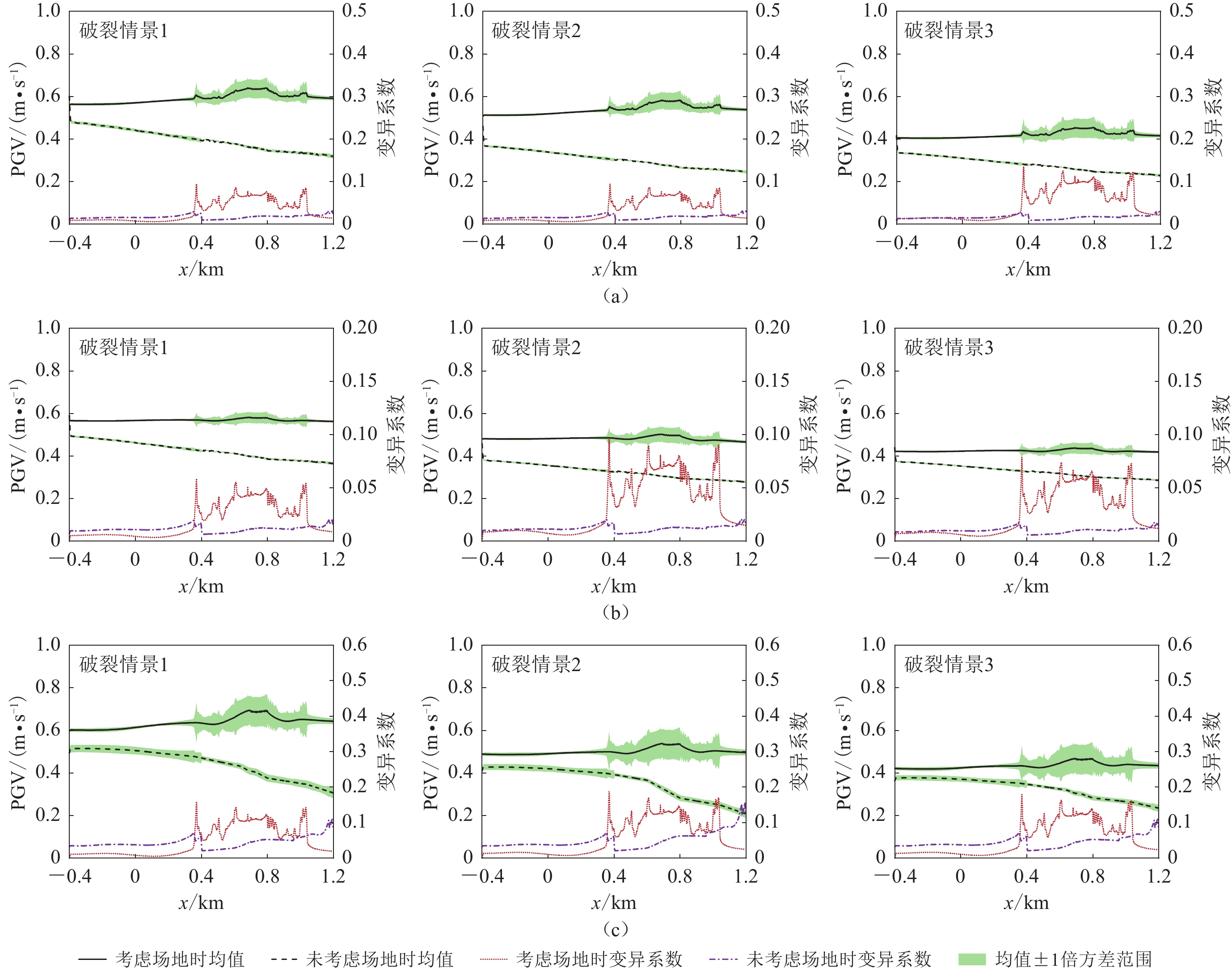
图9a和9b给出了分别考虑凹凸体强度、破裂速度不确定性时近断层高山峡谷场地PGV空间分布变异性。由图可知，当凹凸体强度或破裂速度具有不确定性时，高山峡谷地表的PGV均值振荡剧烈程度均比PGA弱，最大值出现在峡谷地表；高山峡谷地形对地震波的散射同样导致PGV均值增大，例如，三种破裂情景下，具有凹凸体强度的近断层高山峡谷场地PGV均值最大值分别为0.64 m/s，0.58 m/s和0.45 m/s，较无局部地形时放大了2.07，1.78和1.73倍。变异性方面，与PGA结果一致，无起伏地形时PGV变异性受单一不确定参数的影响非常有限，远小于凹凸体强度、破裂速度变异系数。存在高山峡谷地形时，高山峡谷地表PGV变异系数显著提高，其它地表点变异性仍较小。其中，当考虑凹凸体强度不确定性时，高山峡谷地表PGV变异系数最大值约为凹凸体强度变异系数62.4%，表现出场地传播后不确定性的弱化；当考虑破裂速度不确定性时，高山峡谷地表PGV变异系数可较破裂速度变异系数增大80%，表现出场地传播后不确定性的放大。
![]()
图 9 近断层场地PGV空间分布变异性（a） 考虑凹凸体强度不确定性；（b） 考虑破裂速度不确定性；（c） 同时考虑凹凸体强度和破裂速度不确定性Figure 9. Spatial distribution variability of PGVs in the near-fault site（a） Considering asperity intensity uncertainty ；（b） Considering rupture velocity uncertainty；（c） Considering asperity intensity and rupture velocity uncertainty此外，同时考虑凹凸体强度和破裂速度不确定性时近断层高山峡谷场地PGV空间分布均值、均方差和变异系数，如图9c所示。结果表明，当近断层高山峡谷场地具有凹凸体强度和破裂速度不确定性时，三种破裂情景下，峡谷地表均较其它地表点出现更大的PGV均值，与单一参数不确定性分析结果一致；存在高山峡谷地形时，三种破裂情景下，同时考虑凹凸体强度和破裂速度不确定性对应的PGV变异性与单一参数不确定性结果叠加基本一致，此结论与3.2节PGA变异性相同，但各地表点PGV变异系数均小于0.2 （凹凸体强度变异系数），说明整体上场地PGV受凹凸体强度和破裂速度不确定性影响较小，这可能是由于近断层场地地震动加速度和速度时程的能量分布不同导致。
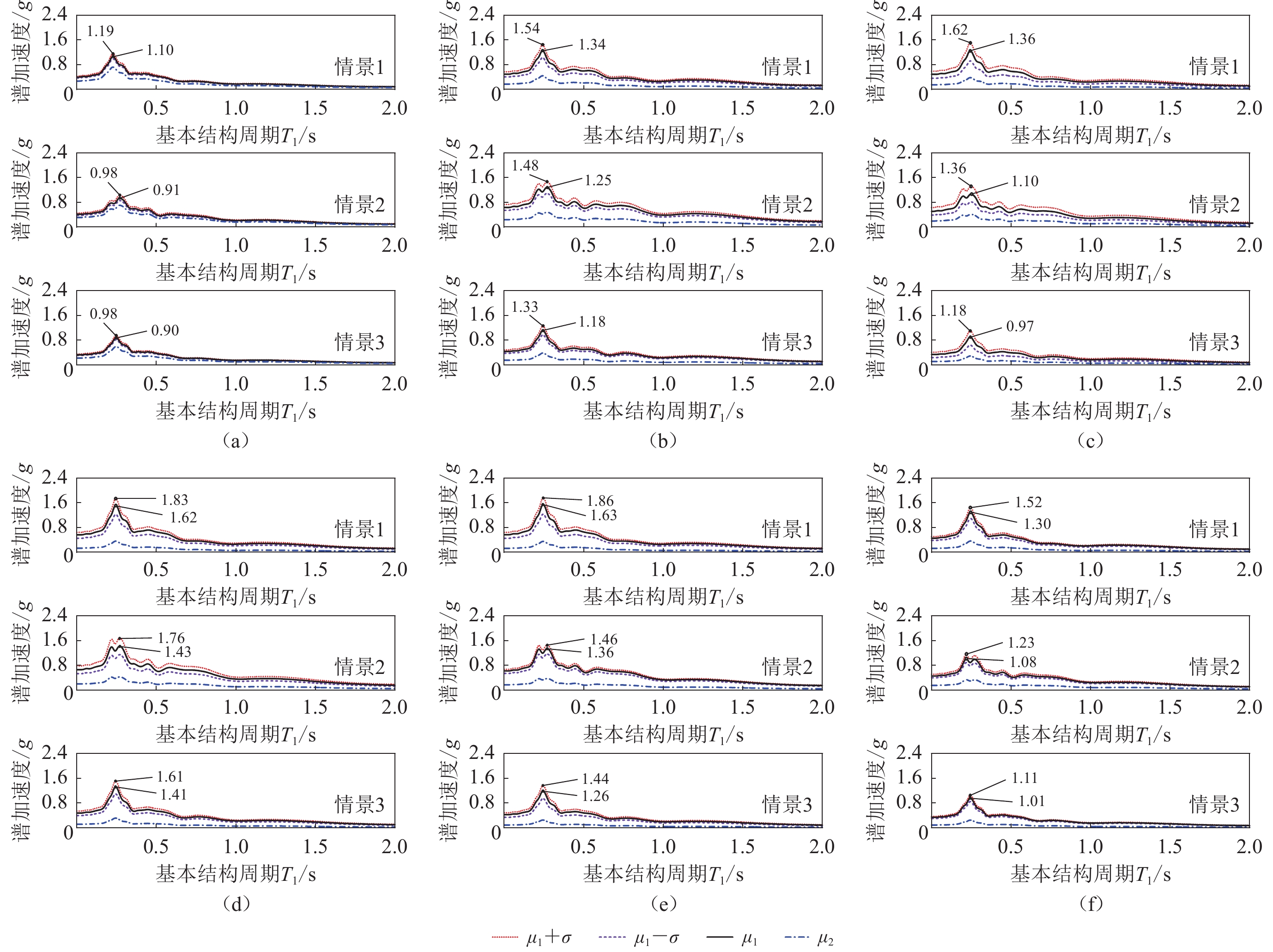
3.4 高山峡谷场地观测点SA变异性
图10—12分别给出了考虑凹凸体强度不确定性、破裂速度不确定性及同时考虑两者不确定性时有无高山峡谷地形时近断层场地观测点（A—F点）SA均值，同时给出了存在局部地形时考虑一倍均方差后对应的观测点SA统计值。
![]()
图 10 考虑凹凸体强度不确定性时近断层场地SA空间分布变异性（a） A点；（b） B点；（c） C点；（d） D点；（e） E点；（f） F点。μ1和μ2分别代表有、无高山峡谷地形时观测点SA均值，σ为存在高山峡谷地形时观测点SA均方差，阻尼比取为0.05，下同Figure 10. Spatial distribution variability of SAs in the near fault site considering asperity intensity uncertainty（a） Point A；（b） Point B；（c） Point C ；（d） Point D；（e） Point E；（f） Point F. μ1 and μ2 represent mean SAs at observed points with and without the mountain-canyon site， σ represents the root mean squares of SAs at observed points with the mountain-canyon site，and the damping ratio is 0.05，the same below![]()
图 12 同时考虑凹凸体强度和破裂速度不确定性时近断层场地SA空间分布变异性（a） A点；（b） B点；（c） C点；（d） D点；（e） E点；（f） F点Figure 12. Spatial distribution variability of SAs in the near-fault site considering asperity intensity and rupture velocity uncertainty（a） Point A；（b） Point B； （c） Point C ；（d） Point D；（e） Point E；（f） Point F由图10—12可知，无高山峡谷地形时，SA最大值对应的结构基本周期约为0.25 s，存在起伏地形时，SA最大值对应的结构基本周期基本不变，但可能存在其它峰值；与场地PGA均值类似，近断层效应耦合场地效应后，不同位置点的SA均值增大显著，特别是基本周期在0.1—0.5 s的结构受地形影响较大，峡谷内C，D，E点结构反应最大值均值增幅有所不同，可放大1.24，1.23和1.14倍。
靠近断层、处于局部地形之外的A点SA受震源不确定性影响较小，其它观测点考虑一倍均方差后，SA较均值变化明显。例如：三种破裂情景下，考虑凹凸体强度不确定性时，峡谷中心点（D点）结构反应最大值（均值与一倍均方差之和）较最大值均值分别增大12%，23%和14%；考虑破裂速度不确定性时，增幅分别为2%，17%和8%；同时考虑两者的不确定性时，增幅为24%，33%和25%。而考虑凹凸体强度（破裂速度）不确定性时，峡谷内C点结构反应最大值（均值与一倍均方差之和）较均值分别增大19% （12%），24% （17%）和22% （15%）；同时考虑两者的不确定性时，增幅变为29%，33%和30%。而破裂情景2下，同时考虑凹凸体强度和破裂速度不确定性时峡谷内E点结构反应最大值均值与一倍均方差之和仅较均值增大18%。结果表明：同时考虑凹凸体强度和破裂速度不确定性时，结构反应变异性可能高于或低于单一参数不确定性变异性的叠加，这与高山峡谷场地PGA和PGV变异性结果不同（近似相等）；此外，结构反应变异性受凹凸体位置影响也十分复杂，与地表点位置有关。对于建造于高山峡谷区域的工程结构，震源不确定性引起的结构反应变异性不可忽略，仅考虑地震动一倍均方差后，结构反应最大值可增大超30%。
4. 结论
1） 本文将乘子降维法应用至震源不确定性引起的近断层高山峡谷场地地震动变异性问题中，与常规蒙特卡洛模拟相比具有更高的计算效率，可兼顾计算精度，此方法可用于基于物理模型、考虑震源不确定性的复杂场地随机地震动模拟。
2） 当近断层区域存在高山峡谷地形时，近断层效应和局部场地效应的耦合作用引起场地PGA均值显著放大，表现出显著的空间变化特征，尤其是峡谷内，可达无起伏地形的2.69倍，不同地表点PGV均值差异性小于PGA；有无高山峡谷地形时，地表地震动加速度反应谱最大值对应的结构周期基本一致。
3） 震源不确定性经由场地传播，最终表现为场地PGA和PGV空间分布变异性，由于地震动加速度和速度的能量分布不同，PGA和PGV变异性存在差异，PGA均有更大的变异系数；对于PGA和PGV，不同破裂情景下，同时考虑凹凸体强度和破裂速度不确定性时其变异性与单一参数不确定性变异性的叠加近似，但加速度反应谱结果较为复杂，不同位置处的结构反应变异性可能低于或高于单一参数不确定性变异性的叠加结果，且受凹凸体位置影响；考虑一倍均方差后，结构反应最大值可较均值变化30%以上。
-
![]()
图 9 近断层场地PGV空间分布变异性
（a） 考虑凹凸体强度不确定性；（b） 考虑破裂速度不确定性；（c） 同时考虑凹凸体强度和破裂速度不确定性
Figure 9. Spatial distribution variability of PGVs in the near-fault site
（a） Considering asperity intensity uncertainty ；（b） Considering rupture velocity uncertainty；（c） Considering asperity intensity and rupture velocity uncertainty
![]()
图 1 近走滑断层高山峡谷场地计算模型示意图
Figure 1. Calculation model of mountain-canyon site near a strike-slip fault
![]()
图 2 不同断层角度下本文方法与Kara和Trifunac （2014）方法所得地表位移结果对比
（a） 倾角为π/24；（b） 倾角为π/4；（c） 倾角为π/2
Figure 2. Comparison of surface displacement results obtained by the proposed method and the method in reference Kara and Trifunac （2014） under different fault angles
（a） Dip angle of π/24；（b） Dip angle of π/4；（c） Dip angle of π/2
![]()
图 3 用于验证的计算模型
（a） 场地计算模型，图中A，B，C，D，E，F为地表参考点；（b） 断层模型
Figure 3. Calculation model for verification
（a） Calulation model of sites，and A，B，C，D，E and F are the surface reference point；（b） Fault model
![]()
图 4 不同入射频率波下高山峡谷场地位移幅值均值（a）及其均方差（b）
Figure 4. Mean values of displacement amplitude of mountain-canyon site （a） and mean square deviation of displacement amplitude of mountain-canyon site （b） under different incident frequency waves
![]()
图 5 考虑凹凸体位置不确定性的破裂情景
Figure 5. Rupture scenarios considering uncertainty of asperity positions
![]()
图 6 考虑凹凸体强度不确定性时近断层场地PGA空间分布变异性
（a） 破裂情景1；（b） 破裂情景2；（c） 破裂情景3
Figure 6. Spatial distribution variability of PGAs in the near-fault site considering asperity intensity uncertainty
（a） Rupture scenario 1；（b） Rupture scenario 2； （c） Rupture scenario 3
![]()
图 7 考虑破裂速度不确定性时近断层场地PGA空间分布变异性
（a） 破裂情景1；（b） 破裂情景2；（c） 破裂情景3
Figure 7. Spatial distribution variability of PGAs in the near-fault site considering rupture velocity uncertainty
（a） Rupture scenario 1；（b） Rupture scenario 2； （c） Rupture scenario 3
![]()
图 8 考虑凹凸体强度和破裂速度不确定性时近断层场地PGA空间分布变异性
（a） 破裂情景1；（b） 破裂情景2；（c） 破裂情景3
Figure 8. Spatial distribution variability of PGAs in the near-fault site considering asperity intensity and rupture velocity uncertainty
（a） Rupture scenario 1；（b） Rupture scenario 2；（c） Rupture scenario 3
![]()
图 10 考虑凹凸体强度不确定性时近断层场地SA空间分布变异性
（a） A点；（b） B点；（c） C点；（d） D点；（e） E点；（f） F点。μ1和μ2分别代表有、无高山峡谷地形时观测点SA均值，σ为存在高山峡谷地形时观测点SA均方差，阻尼比取为0.05，下同
Figure 10. Spatial distribution variability of SAs in the near fault site considering asperity intensity uncertainty
（a） Point A；（b） Point B；（c） Point C ；（d） Point D；（e） Point E；（f） Point F. μ1 and μ2 represent mean SAs at observed points with and without the mountain-canyon site， σ represents the root mean squares of SAs at observed points with the mountain-canyon site，and the damping ratio is 0.05，the same below
![]()
图 12 同时考虑凹凸体强度和破裂速度不确定性时近断层场地SA空间分布变异性
（a） A点；（b） B点；（c） C点；（d） D点；（e） E点；（f） F点
Figure 12. Spatial distribution variability of SAs in the near-fault site considering asperity intensity and rupture velocity uncertainty
（a） Point A；（b） Point B； （c） Point C ；（d） Point D；（e） Point E；（f） Point F
表 1 边界元法数值稳定性验证 （η＝0.25）
Table 1 Numerical stability verification of boundary element method （η＝0.25）
x/a 边界元法参数 解析解 N＝600，
L＝561N＝1 000，
L＝801N＝1 400，
L＝1 041−2.0 0.070 3 0.070 7 0.070 4 0.070 4 −1.5 0.091 3 0.090 9 0.090 8 0.090 8 −1.0 0.518 9 0.519 9 0.522 6 0.522 6 −0.5 0.495 0 0.516 7 0.551 2 0.551 2 0.5 0.744 8 0.749 8 0.754 7 0.754 7 1.0 0.494 2 0.547 7 0.551 2 0.551 2 1.5 0.097 8 0.099 2 0.100 3 0.100 3 2.0 0.083 3 0.084 0 0.084 6 0.084 6 
下载: 导出CSV
-
巴振宁,吴孟桃,梁建文,喻志颖. 2020. 高山—峡谷复合地形对入射平面P-SV波的散射[J]. 应用数学和力学,41(7):695–712. Ba Z N,Wu M T,Liang J W,Yu Z Y. 2020. Scattering and diffraction by the hill-canyon composite topography for incident plane P- and SV-waves[J]. Applied Mathematics and Mechanics,41(7):695–712 (in Chinese).
陈畅,吴军,黄炬斌,代劲松. 2019. 新建川藏铁路雅安至林芝段出渣综合利用及处置对策研究[C]//川藏铁路工程建造技术研讨会论文集. 北京:中国铁建股份有限公司:25−28. Chen C,Wu J,Huang J B,Dai J S. 2019. Study on comprehensive utilization and disposal countermeasures of slag from Ya'an to Linzhi section of newly constructed Sichuan−Tibet railway[C]//Proceedings of Sichuan-Tibet Railway Engineering Construction Technology Seminar. Beijing:China Railway Construction Corporation:25−28 (in Chinese).
陈笑宇,王东升,付建宇,国巍. 2021. 近断层地震动脉冲特性研究综述[J]. 工程力学,38(8):1–14. Chen X Y,Wang D S,Fu J Y,Guo W. 2021. State-of-the-art review on pulse characteristics of near-fault ground motions[J]. Engineering Mechanics,38(8):1–14 (in Chinese).
雷涛,李碧雄,曹鹏杰,顺远坤. 2010. 汶川地震近断层附近桥梁震害浅析[J]. 重庆交通大学学报(自然科学版),29(3):358–362. Lei T,Li B X,Cao P J,Shun Y K. 2010. Analysis on damage of near-faultage bridges in Wenchuan earthquake[J]. Journal of Chongqing Jiaotong University (Natural Science),29(3):358–362 (in Chinese).
李宁,刁泽民,李忠献. 2022. 考虑震源和场地特征的近断层地区竖向地震动合成研究[J]. 工程力学,39(6):181–190. Li N,Diao Z M,Li Z X. 2022. Study on synthesis method of vertical ground motions for near-fault regions considering the characteristics of source and site condition[J]. Engineering Mechanics,39(6):181–190 (in Chinese).
李郑梁,李建春,刘波,聂萌萌. 2021. 浅切割的高山峡谷复杂地形的地震动放大效应研究[J]. 工程地质学报,29(1):137–150. Li Z L,Li J C,Liu B,Nie M M. 2021. Seismic motion amplification effect of shallow-cutting hill-canyon composite topography[J]. Journal of Engineering Geology,29(1):137–150 (in Chinese).
罗全波,陈学良,高孟潭,李宗超,李铁飞. 2019. 近断层速度脉冲地震动的三维有限差分模拟[J]. 地震工程学报,41(6):1630–1636. Luo Q B,Chen X L,Gao M T,Li Z C,Li T F. 2019. Three-dimensional finite-difference simulation of near-fault velocity pulse-like ground motions[J]. China Earthquake Engineering Journal,41(6):1630–1636 (in Chinese).
王海云,谢礼立. 2010. 自贡市西山公园地形对地震动的影响[J]. 地球物理学报,53(7):1631–1638. Wang H Y,Xie L L. 2010. Effects of topography on ground motion in the Xishan park,Zigong city[J]. Chinese Journal of Geophysics,53(7):1631–1638 (in Chinese).
王伟,刘必灯,刘欣,杨明亮,周正华. 2015. 基于汶川MS8.0地震强震动记录的山体地形效应分析[J]. 地震学报,37(3):452–462. Wang W,Liu B D,Liu X,Yang M L,Zhou Z H. 2015. Analysis on the hill topography effect based on the strong ground motion records of Wenchuan MS8.0 earthquake[J]. Acta Seismologica Sinica,37(3):452–462 (in Chinese).
袁飞云,王剑波. 2018. 雅康高速长下坡高桥隧比路段交通安全设施优化设计研究[J]. 交通科技,(5):114–117. Yuan F Y,Wang J B. 2018. Research on optimum design of traffic safety facilities for Ya’an-Kangding expressway at long downhill and high ratio of bridge tunnel section[J]. Transportation Science and Technology,(5):114–117 (in Chinese).
张冬锋. 2019. 随机有限断层法参数不确定性分析及其在近场地震动模拟中的工程应用研究[D]. 北京:中国地震局地球物理研究所:26−29. Zhang D F. 2019. Parameter Uncertainty Analysis of Stochastic Finite-Fault Method and Its Application in Near-Field Ground Motion Simulation[D]. Beijing:Institute of Geophysics,China Earthquake Administration:26−29 (in Chinese).
Ameri G,Gallovic F,Pacor F,Emolo A. 2009. Uncertainties in strong ground-motion prediction with finite-fault synthetic seismograms:An application to the 1984 M5.7 Gubbio,Central Italy,Earthquake[J]. Bull Seismol Soc Am,99(2A):647–663. doi: 10.1785/0120080240
Bjerrum L W,Sørensen M B,Ottemöller L,Atakan K. 2013. Ground motion simulations for İzmir,Turkey:Parameter uncertainty[J]. J Seismol,17(4):1223–1252. doi: 10.1007/s10950-013-9389-9
Cao Z L,Tao X X,Tao Z R,Tang A P. 2019. Kinematic source modeling for the synthesis of broadband ground motion using the f-κ approach[J]. Bull Seismol Soc Am,109(5):1738–1757.
Fortuño C,de la Llera J C,González G,González J,Aguirre P. 2021. Rupture parameter sensitivity of low frequency ground motion response spectra using synthetic scenarios in North Chile[J]. Bull Earthq Eng,19(12):4833–4864. doi: 10.1007/s10518-021-01113-0
Hartzell S,Frankel A,Liu P C,Zeng Y H,Rahman S. 2011. Model and parametric uncertainty in source-based kinematic models of earthquake ground motion[J]. Bull Seismol Soc Am,101(5):2431–2452. doi: 10.1785/0120110028
Haskell N A. 1964. Total energy and energy spectral density of elastic wave radiation from propagating faults[J]. Bull Seismol Soc Am,54(6A):1811–1841. doi: 10.1785/BSSA05406A1811
Huang H C,Chiu H C. 1995. The effect of canyon topography on strong ground motion at Feitsui damsite:Quantitative results[J]. Earthq Engin Struct Dyn,24(7):977–990. doi: 10.1002/eqe.4290240705
Iwaki A,Morikawa N,Maeda T,Fujiwara H. 2017. Spatial distribution of ground-motion variability in broadband ground-motion simulations[J]. Bull Seismol Soc Am,107(6):2963–2979. doi: 10.1785/0120170150
Kara H F,Trifunac M D. 2014. Two-dimensional earthquake vibrations in sedimentary basins:SH waves[J]. Soil Dyn Earthq Eng,63:69–82. doi: 10.1016/j.soildyn.2014.03.010
Liu Z X,Liu Y,Huang L,Li Y R,Zhao R B. 2021. Indirect boundary element method solution to the seismic ground motion of near-fault sedimentary valley[J]. Eng Anal Bound Elem,132:289–308.
Liu Z X,Zhou T,Meng S B,Jin L G. 2022. 2-D FM-IBEM simulation of broadband ground motions on near-fault mountain-valley coupling site[J]. Eng Anal Bound Elem,145:224–241. doi: 10.1016/j.enganabound.2022.09.020
Sánchez-Sesma F J,Campillo M. 1991. Diffraction of P,SV,and Rayleigh waves by topographic features:A boundary integral formulation[J]. Bull Seismol Soc Am,81(6):2234–2253.
Yamada M,Senna S,Fujiwara H. 2011. Statistical analysis of ground motions estimated on the basis of a recipe for strong-motion prediction:Approach to quantitative evaluation of average and standard deviation of ground motion distribution[J]. Pure Appl Geophys,168(1/2):141–153.
Zhang J,Bi K M,Zheng S X,Jia H Y,Zhang D Y. 2018. Seismic system reliability analysis of bridges using the multiplicative dimensional reduction method[J]. Struct Infrastruct E,14(11):1455–1469. doi: 10.1080/15732479.2018.1450428
Zhang X F,Pandey M D. 2013. Structural reliability analysis based on the concepts of entropy,fractional moment and dimensional reduction method[J]. Struct Saf,43:28–40. doi: 10.1016/j.strusafe.2013.03.001
计量
- 文章访问数: 299
- HTML全文浏览量: 31
- PDF下载量: 147
