Magnetic field distribution in geomagnetic observation bin based on equivalent magnetic charge method
-
摘要:
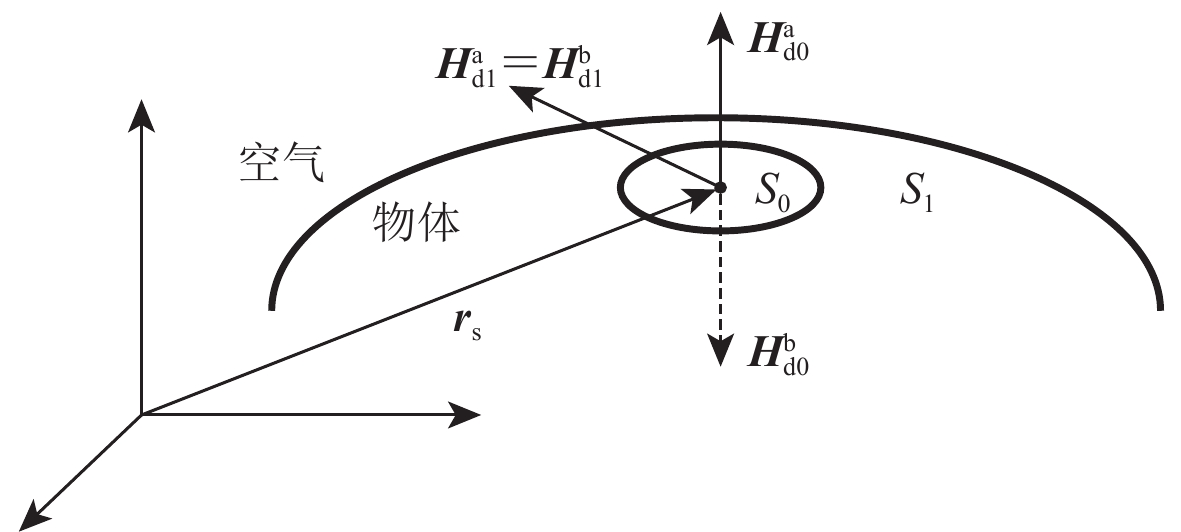
利用等效磁荷方法计算了非轴向磁化圆柱形有底仓体表面的磁荷密度分布和仓内的磁场分布，并与计算机仿真和实测结果进行了对比，结果显示其相对差值＜0.001%。在此基础上，利用该方法计算了无底仓体及不同底部厚度、高度、直径、磁化率的仓体的内部磁场梯度分布情况。结果表明：仓体底部厚度和磁化率对仓体内部磁场梯度的影响较小，增大仓体高度和直径可有效地增加内部的低磁场梯度空间，当仓体内高≥4 m、仓体内径≥1.0 m时，仓体内部水平方向和垂直方向的有效空间占比平均在85%以上。
Abstract:Geomagnetic field observation constitutes a vital part of geophysical data acquisition. In recent years, the employment of smaller and more compact bins for geomagnetic observations has emerged as a novel trend. A geomagnetic observation bin typically takes the form of a cylindrical structure with a bottom. By utilizing the equivalent magnetic charge （EMC） method, a system of equations is initially solved at the boundaries between the object and the surrounding air. This facilitates the determination of the surface magnetic charge density on N discrete elements, which is subsequently employed to compute the magnetic field at any point in space. When applying this method to a cylindrical bottomed bin with non-axial magnetization, we obtain the distribution of surface magnetic charge density and the magnetic field within the bin, followed by the calculation of the magnetic field gradient inside.
In the calculations, the magnetic susceptibility of typical concrete was referred to, and the background magnetic field characteristics were acquired using the International Geomagnetic Reference Field （IGRF）. The results reveal that, due to the small geomagnetic declination angle D, the magnetic field intensity inside the observation bin exhibits approximate axial symmetry in the east-west direction, and near-central symmetry in the north-south direction. Near the bottom of the bin, the magnetic field intensity mainly increases, with a maximum increment of approximately 1.2 nT compared to the background field. Near the top of the bin, the magnetic field intensity predominantly decreases, with a maximum decrease of around 1.5 nT. The largest magnetic field gradients are concentrated at the edges of the top and bottom of the bin.
Finite element simulations along three measuring lines were compared with actual measurement data. These measuring lines include: ① a vertical line inside the bin, ② a north-south line one meter above the bin’s bottom, and ③ an east-west line one meter below the bin’s top, with a measurement interval of 0.1 meters. The results demonstrate that the magnetic field calculated using the EMC method deviates from the measured results by less than 0.1 nT, with a relative error of less than
0.000 1 %. In the region near the bottom of the bin along the vertical line, the magnetic field intensity increases by approximately 0.7 nT, while near the top of the bin, it decreases by about 0.2 nT.The effects of the bin’s bottom thickness, the ratio of its height to diameter, and the magnetic susceptibility of the material on the internal magnetic field gradient were also explored. The results indicate that as the bottom thickness of the bin decreases, the effective horizontal space inside the bin enlarges. When the bin is bottomless, it offers the maximum horizontal effective space. However, a bottomless structure does not augment the vertical effective space inside the bin. When the external and internal heights of the bin are kept constant, an increase in the inner diameter leads to more effective vertical space inside the bin, without significant alteration in the horizontal direction. When the inner and outer diameters of the bin are fixed, increasing the internal height results in more effective horizontal space inside the bin, with no pronounced impact on the vertical direction. The highest magnetic field gradients inside the bin are concentrated near the edges of the top and bottom. As the bin’s dimensions expand, the proportion of effective space inside the bin also rises. Moreover, when the bin size remains unchanged, the influence of varying magnetic susceptibilities on the magnetic field gradient inside the bin was analyzed. The results suggest that for magnetic susceptibilities ≤800×10−6, increasing the susceptibility does not trigger significant changes in the effective space inside the bin.
-
-
![]()
图 1 物体与空气的边界上的磁场关系
Figure 1. Magnetic field relationship at the boundary between an object and air
![]()
图 3 仓体内部沿南北向（a）、东西向（b）剖面的磁场分布
Figure 3. Distribution of magnetic field along N-S （a） and E-W （b） profiles in the bin
![]()
图 4 仓体内部剖面的磁场梯度分布
（a） 南北向剖面的水平梯度；（b） 南北向剖面的垂直梯度；（c） 东西向剖面的水平梯度；（d） 东西向剖面的垂直梯度
Figure 4. Distribution of magnetic field gradient along the internal profiles of the bin
（a） Horizontal gradient along the N-S profile；（b） Vertical gradient along the N-S profile； （c） Horizontal gradien along the E-W profile；（d） Vertical gradient along the E-W profile
![]()
图 5 利用三种方法得到的仓体内部沿三条测线的磁场分布对比
（a） 仓体内部的垂直测线；（b） 距离仓体底部1 m处的南北向测线；（c） 距离仓体顶部1 m处的东西向测线
Figure 5. Comparison of magnetic field distribution along three measuring lines in the bin by the three methods
（a） Vertical line inside the bin；（b） N-S line 1 m from the bottom of the bin；（c） E-W line 1 m from the top of the bin
表 1 不同仓体底厚对应的仓体内部磁场梯度分布特征
Table 1 The distribution characteristics of the magnetic field gradient inside the bin with different bottom thickness of the bin
仓体底部
厚度/m仓体内高与
底厚之比水平方向最小内径
与仓体内径之比垂直方向最小高度
与仓体内高之比2.25 1.00 0.82 0.78 1.13 3.00 0.83 0.86 0.75 5.00 0.84 0.75 0.56 7.00 0.84 0.75 0.45 9.00 0.84 0.75 0 ∞ 0.87 0.83 
下载: 导出CSV
表 2 仓体高度和直径对应的仓体内部磁场梯度的分布特征
Table 2 The distribution characteristics of the magnetic field gradient inside the bin with different height and diameter of the bin
内高
/m不同内径情况下水平方向最小内径与仓体内径之比 不同内径情况下垂直方向最小高度与仓体内高之比 1 m 2 m 3 m 4 m 5 m 1 m 2 m 3 m 4 m 5 m 1 0.70 0.70 0.80 0.85 0.88 0.80 0.90 0.90 0.90 0.90 2 0.95 0.80 0.80 0.85 0.88 0.60 0.80 0.95 0.95 0.95 3 0.95 0.90 0.87 0.85 0.88 0.73 0.73 0.90 0.97 0.97 4 0.96 0.97 0.97 0.90 0.88 0.80 0.80 0.85 0.93 0.98 5 0.98 0.98 0.98 0.92 0.89 0.84 0.84 0.91 0.97 0.99
下载: 导出CSV
表 3 不同仓体材料磁化率对应的仓体内部磁场梯度的分布特征
Table 3 The distribution characteristics of the magnetic field gradient inside the bin with magnetic susceptibility of different bin materials
磁化率χ
/10−6水平方向最小内径与
仓体内径之比垂直方向最小高度与
仓体内高之比100 0.82 0.78 200 0.82 0.78 400 0.84 0.78 600 0.84 0.77 800 0.86 0.77
下载: 导出CSV
-
郭友钊,李祥新,董杰,李磊. 2001. 河北省沉积岩磁化率特征及古温度意义的初探[J]. 物探与化探,25(5):368–371. Guo Y Z,Li X X,Dong J,Li L. 2001. A preliminary study on features and paleotemperature implications of sedimentary magnetic susceptibility in Hebei Province[J]. Geophysical &Geochemical Exploration,25(5):368–371 (in Chinese).
单红丹,卢升高. 2005. 火电厂粉煤灰的矿物磁性及其环境意义[J]. 矿物学报,25(2):141–146. doi: 10.3321/j.issn:1000-4734.2005.02.009 Shan H D,Lu S G. 2005. Mineral magnetism of power-plant fly ash and its environmental implication[J]. Acta Mineralogica Sinica,25(2):141–146 (in Chinese).
王树萱,贾义侠. 1990. 磁场计算中的等效磁荷法[J]. 太原重型机械学院学报,11(3):84–91. Wang S X,Jia Y X. 1990. Equivalent magnetic monopoles model for calculation of magnetic field[J]. Journal of Taiyuan Heavy Machinery Institute,11(3):84–91 (in Chinese).
吴攀,郭智勇,徐伟,陈文辉. 2020. 磁偶极子构造法球形磁性矿体磁异常正演[J]. 地球物理学进展,35(3):893–900. doi: 10.6038/pg2020DD0189 Wu P,Guo Z Y,Xu W,Chen W H. 2020. Magnetic dipole reconstruction method for forward modeling of magnetic anomaly of spherical magnetic body[J]. Progress in Geophysics,35(3):893–900 (in Chinese).
夏志成,马晓进,毕亚军,周健南,吴鹏. 2004. 硅酸盐类水泥的磁性试验研究[J]. 水泥工程,(4):17–20. doi: 10.3969/j.issn.1007-0389.2004.04.004 Xia Z C,Ma X J,Bi Y J,Zhou J N,Wu P. 2004. Trial study on magnetism of Portland cement[J]. Cement Engineering,(4):17–20 (in Chinese).
张秀霞,刘高川,蒋延林,郭宇鑫,赵卫红,张骞,潘颖,高熹微,李玉. 2022. 地埋式地磁观测装置的设计与实现[J]. 中国地震,38(1):131–141. doi: 10.3969/j.issn.1001-4683.2022.01.013 Zhang X X,Liu G C,Jiang Y L,Guo Y X,Zhao W H,Zhang Q,Pan Y,Gao X W,Li Y. 2022. Design and test of a buried geomagnetic observation device[J]. Earthquake Research in China,38(1):131–141 (in Chinese).
张朝阳,肖昌汉,高俊吉,周国华. 2010. 磁性物体磁偶极子模型适用性的试验研究[J]. 应用基础与工程科学学报,18(5):862–868. doi: 10.3969/j.issn.1005-0930.2010.05.016 Zhang Z Y,Xiao C H,Gao J J,Zhou G H. 2010. Experiment research of magnetic dipole model applicability for a magnetic object[J]. Journal of Basic Science and Engineering,18(5):862–868 (in Chinese).
中国地震局. 2004. DB/T 9—2004 地震台站建设规范:地磁台站[S]. 北京:中国地震局:2−4. China Earthquake Administration. 2004. DB/T 9−2004 Specification for the Construction of Seismic Station:Geomagnetic Station[S]. Beijing:China Earthquake Administration:2−4 (in Chinese).
周耀忠,张国友. 2004. 舰船磁场分析计算[M]. 北京:国防工业出版社:135−136. Zhou Y Z,Zhang G Y. 2004. Ship Magnetic Field Analysis and Calculation[M]. Beijing:National Defense Industry Press:135−136 (in Chinese).
Guo Z Y,Liu D J,Chen Z,Meng H. 2012. Modeling on ground magnetic anomaly detection of underground ferromagnetic metal pipeline[C]//ICPTT 2012:Better Pipeline Infrastructure for a Better Life. Wuhan:American Society of Civil Engineers:1011−1024.
Guo Z Y,Liu D J,Pan Q,Zhang Y Y. 2015. Forward modeling of total magnetic anomaly over a pseudo-2D underground ferromagnetic pipeline[J]. J Appl Geophys,113:14–30. doi: 10.1016/j.jappgeo.2014.12.011
Huang X J,Chen S L,Guo S X,Zhao W,Jin S J. 2013. Magnetic charge and magnetic field distributions in ferromagnetic pipe[J]. ACES J,28(8):737–746.
Soda N,Kobayashi M,Rowlands G. 2004. Charge densities and inclination angles of magnetization on various surfaces of rotational symmetry[J]. IEEE Trans Magn,40(4):1763–1768. doi: 10.1109/TMAG.2004.828160
计量
- 文章访问数: 39
- HTML全文浏览量: 11
- PDF下载量: 10
