Dynamic source rupture process of the 2022 Menyuan MW6.6 earthquake，Qinghai Province
-
摘要:
2022年1月8日青海门源MW6.6地震在地表产生了较强的破坏，为研究门源地震产生强地表破裂及阻碍托莱山东段应力释放的构造背景，本文采用谱元法模拟了门源地震曲面分支断层在实际地形下的动力学破裂过程。结果显示，破裂自初始破裂点沿断层上倾方向同时发生双侧破裂；受震源区上方高速P波异常体的影响，断层产生非连续破裂。在破裂传播到托莱山东段时，断层的滑动速度和滑动量发生明显的阶跃式下降；此外，近地表处滑动速率约3.6 m/s的区域可能为强地面运动生成区。以上两者产生的高频辐射共同作用可能是此次门源地震同震地表形变较强的主要原因。由动力学模拟结果计算得到的应变空间分布显示，托莱山东段南西侧与冷龙岭西段北东侧的应变以拉张为主，而冷龙岭西段南西侧的应变以挤压为主，这与门源地震震源机制解结果、门源地震所处的青藏高原东北缘北东向主压转为南西向迁移所处的构造背景以及ENE和WSW的震源区应力场方向一致。托莱山断裂东段受分支断层破裂过程的强烈抑制作用，其累积应力未完全释放，残留震级约为MW5.1，在门源地震库仑应力的触发作用下，未来有进一步破裂的可能。
Abstract:On January 8, 2022, a significant earthquake with a magnitude of 6.6 struck Menyuan, Qinghai, which resulted in substantial surface damage. To investigate the geological context behind the strong surface rupture generated by the Menyuan earthquake and its impact on the inhibition of stress release in the eastern section of the Tuolaishan fault, the spectral element method was employed in this study to simulate the dynamic rupture process of the branching fault on actual terrain. The dynamic rupture simulation revealed that the rupture was initiated bilaterally along an upward direction from the initial rupture point. Under the influence of a high-speed P-wave anomaly located above the source area, the rupture displayed a non-continuous pattern. With the progression of the rupture into the eastern section of Tuolaishan, a significantly abrupt decrease occurred in both slip rate and slip. Furthermore, the area with a slip rate of around 3.6 m/s near the surface of the Earth could be considered as a strong motion generation zone. The combined influence of these factors, along with their high-frequency radiation, might have played a pivotal role in the pronounced coseismic surface deformation during the MW6.6 earthquake in Menyuan. As calculated from the dynamic simulation results, the spatial distribution of strain suggested that the southwestern side of the eastern section of Tuolaishan and the northeastern side of the western section of Lenglongling experienced predominantly tensile stress, with corresponding areas subjected to compression. This observation aligns with the focal mechanism solution and the geological context of the northeastern margin of the Qinghai-Tibet Plateau, where the direction of principal compressive stress transitions from north-south to southwest-northeast. Furthermore, the dynamic rupture process in the eastern section of Tuolaishan was strongly inhibited by the rupture of the branching fault. This led to incomplete stress release and a residual seismic magnitude of approximately MW5.1. Under the trigger of Coulomb stress from the Menyuan earthquake, further rupture in the future is possible.
-
Keywords:
- 2022 Menyuan earthquake /
- dynamic rupture /
- spectral element method /
- Coulomb stress
-
引言
2022年1月8日青海门源MW6.6地震震中（37.77°N，101.26°E）位于青藏高原东北缘的前缘、冷龙岭断裂西段（图1），中国地震台网中心（2022）最终确定其震源深度约为10 km，此地震为冷龙岭断裂带自1986年发生两次M6地震（图1）后发生的地表破裂最强的地震（李智敏等，2022）。门源地震余震重定位结果（Fan et al，2022）显示，余震深度主要介于7—12 km，在主震震中东西两侧分别沿冷龙岭断裂的北西向和托莱山断裂的东西向分布；采用地震观测资料反演的门源地震主震和余震的震源机制解（万永革等，2022）表明，门源地震主震为近直立断层面上左旋走滑事件。此外，大地电磁剖面和体波成像结果（王琼等，2022；赵凌强等，2022）进一步揭示了门源地震深部构造和孕震环境的复杂性：此次门源地震震中位于以冷龙岭断裂带为高-低阻边界的高阻一侧，该区域地壳厚度和体波速度也具有明显的空间变化特征，这与震源区的构造背景相对应，青藏高原东北缘应力场表现为北东向挤压和南东向拓展。震后野外应急考察和InSAR同震干涉结果显示本次地震形成的同震地表变形约27 km，地表破裂以左旋走滑为主，并兼有局部逆冲，整体走向WNW-ESE，最大同震左旋位错约3 m （韩帅等，2022；李智敏等，2022；梁宽等，2022；潘家伟等，2022；薛善余等，2022）。门源MW6.6地震在产生强地表破裂的同时，仍存在残留应力，尤其是托莱山东段，库仑应力明显升高，具有较大的地震危险性（许英才等，2022；余鹏飞等，2022；冯万鹏等，2023）。因此，本次地震的震源破裂过程的研究尤为重要。
![]()
图 1 门源MW6.6地震和研究区内历史强震分布（GCMT，2022）黑点为余震震中分布（数据源自Fan et al，2022）；红色实线为区域内活断层在地表的投影（数据源自徐锡伟等，2016）；绿框为进行强地面运动模拟的范围Figure 1. Distribution of the Mengyuan MW6.6 earthquake and historical strong earthquakes in the region （GCMT，2022 ）The black dots are the distribution of aftershock epicenters （data from Fan et al，2022）；the red solid line is the projection of active faults on the surface in the region （data from Xu et al，2016）；the green box is the range wherestrong ground motion simulations were performed门源地震发生后，不同学者利用远震、GPS和InSAR观测资料（李振洪等，2022；Liao et al，2022；Yang et al，2022；冯万鹏等，2023）反演得到了门源地震的震源运动学破裂过程，其结果均显示此次地震的破裂过程表现为沿WNW-ESE的双侧破裂，破裂持续时间约15 s，同震滑动主要集中在震源上方4—7 km处，最大滑动量约为3.5 m。这些运动学模型描述了门源地震的震源破裂过程，但目前尚未有研究从物理学角度分析门源地震震源破裂形成的机制。从物理学角度分析认为，构造运动引起的应力变化在震源区累积，逐渐加载到介质强度后发生破裂并产生地震，在断层面上形成同震滑动。因此，为了从物理学角度解释此次门源MW6.6地震震源破裂机制及产生强地表破裂的成因，分析破裂过程中阻碍托莱山东段应力释放的构造背景，本文依据青海门源MW6.6地震的运动学震源模型（Yang et al，2022）构建包含实际地形的曲面分支断层模型，采用谱元法对门源地震进行动力学模拟。同时，研究断层面几何对动力学破裂过程的影响，并探讨应力、构造和震源破裂之间的关系，分析门源地震动力学破裂过程及强地表破裂产生的物理机制。
1. 断层模型建立和数值模拟方法
门源地震余震定位（Fan et al，2022）、InSAR同震形变（余鹏飞等，2022；Liao et al，2022）以及野外考察（李智敏等，2022；潘家伟等，2022）和高分卫星影像解译（薛善余等，2022； Yang et al，2022；冯万鹏等，2023）的结果表明，此次门源地震地表破裂带主要包括两部分。Yang等（2022）采用Sentinel-1卫星的升、降轨数据和差分干涉方法得到了门源地震的同震形变场，解译和构建了多断层段的运动学震源模型，其结果与野外实地调查显示的地表破裂带一致。因此，本文采用Yang 等（2022）的运动学断层数据，构建包含实际地形的三维曲面分支断层模型（图2）：沿托莱山断裂东段的分支断层F1和沿冷龙岭断裂西段的主断层F2，F3。其中：F1断层的曲面长度约18.0 km，F2和 F3断层的曲面长度分别约为18.6 km和30.0 km，断层面倾角均为82°；断层上边界与起伏地形的最低点相切。震源深度（初始破裂点）设置为由中国地震台网中心（2022）确定的10 km （图2），基于此模型进行了门源地震动力学破裂过程的模拟。速度结构采用Fan等（2022）计算门源地震余震精定位结果的一维层状速度模型，P波和S波波速比设为1.73 （表1）。
![]()
图 2 数值计算空间和包含实际地形的曲面分支断层模型（Yang et al，2022）F1为托莱山断裂东段的分支断层；F2和F3为冷龙岭断裂的西段主断层Figure 2. Numerical computational space and a curved surface branch fault model （Yang et al，2022） that includes actual terrainF1 is the branch fault of easternTuolaishan fault；F2 and F3 are the main faults of the western Lenglongling fault表 1 门源地震动力学破裂模拟采用的层状速度 结构（Fan et al，2022）Table 1. Layered velocity structure used in the Menyuan earthquake dynamics rupture simulation （Fan et al，2022）层数 vP/（km·s−1） vS/（km·s−1） 深度上边界/km 1 5.0 2.9 0 2 5.2 3.0 2.5 3 6.1 3.5 5.8 4 6.6 3.8 16.3 5 6.9 4.0 28.1 6 8.2 4.7 57.0 考虑到断层模型的复杂性，本研究采用基于谱元法（spectral element method，缩写为SEM）的动力学模拟程序Specfem3D（Komatitsch，Vilotte，1998；Komatitsch，Tromp，1999，2002a，b）对门源地震的破裂过程进行数值模拟。谱元法是一种基于有限元法（finite element method，缩写为FEM）和谱方法（spectral method，缩写为SM）的高阶数值计算方法（Komatitsch et al，2005），不仅具有有限元法计算复杂断层几何结构时的灵活性，同时还具备伪谱法高精度以及快速收敛的特性。Komatitsch和Vilotte （1998）与Komatitsch 和Tromp （1999，2002a，b）构建了基于谱元法的三维波动方程的数值模拟程序Specfem3D，该程序已经在南加州动力学破裂代码项目中得到验证（Harris et al，2009），且已广泛应用于地震动力学破裂过程的研究（Kaneko et al，2008；Galvez et al，2014；Weng，Ampuero，2019；谢张迪等，2024）。
2. 震源动力学参数设置
在震源动力学模拟过程中，断层面上动力学参数（初始应力、屈服应力和滑动弱化距离）和摩擦准则决定震源破裂过程。除初始应力外，其它震源破裂特征，包括地震的起始、传播和停止的方式，由摩擦准则确定（Olsen et al，1997）。目前，在动力学破裂过程模拟中主要采用三种摩擦准则：即滑动弱化摩擦准则（Ida，1972；Andrews，1976）、速率弱化摩擦准则（Carlson，Langer，1989）和速率状态摩擦准则（Dieterich，1978，1979；Ruina，1983）。基于实际地震的动力学参数计算结果，一些研究通过滑动量、滑动速度与应力之间的函数关系确定本构关系，其结果表明断层面上应力与滑动量之间存在与滑动弱化摩擦准则相近的函数关系（Bouchon，1997；Ide，Takeo，1997；Zhang et al，2003），即在破裂开始后，断层面上的剪切应力，随着滑动量的增加，由屈服应力线性减小到剩余应力。本文参考前人研究结果，采用滑动弱化摩擦准则（图3）进行动力学破裂过程的数值模拟。
![]()
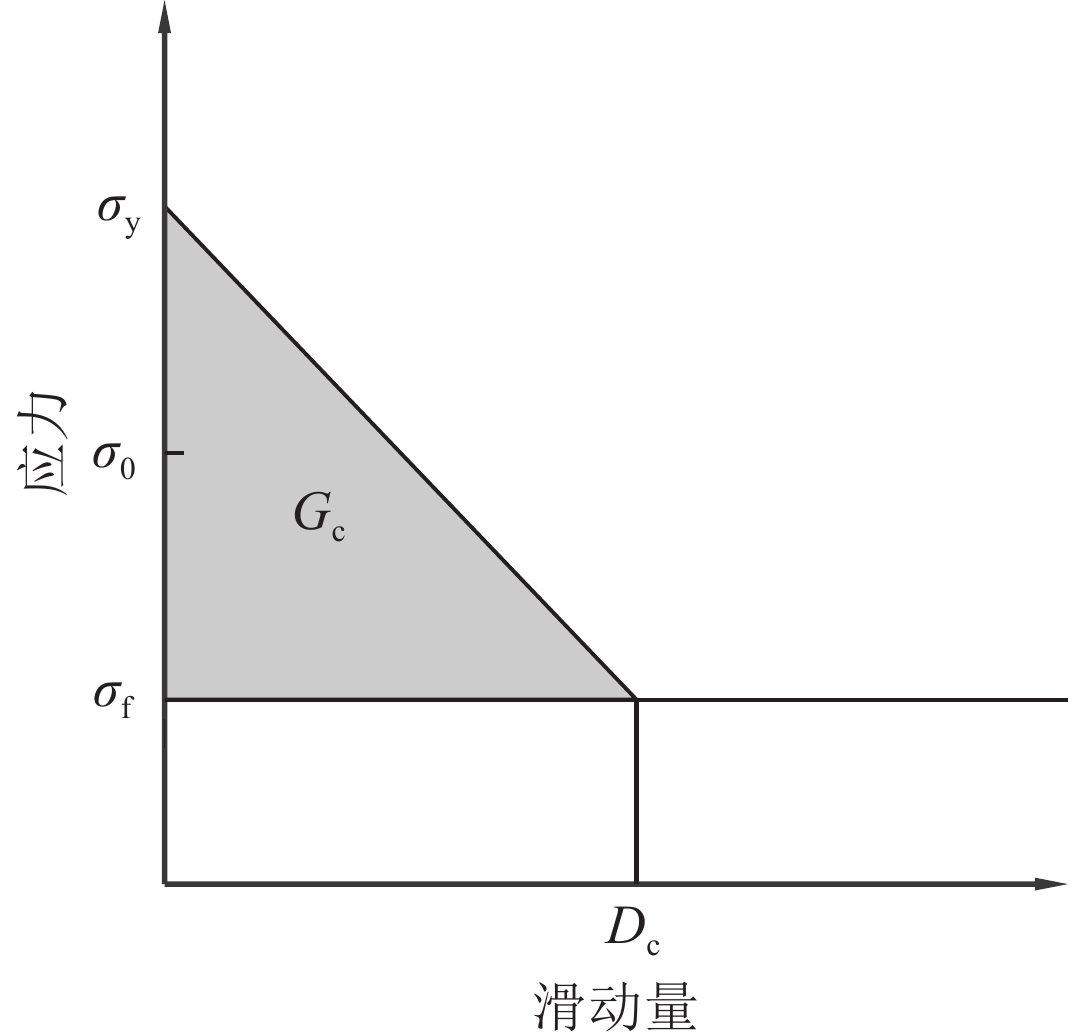
图 3 滑动弱化摩擦准则$ {\sigma }_{\mathrm{y}} $：屈服应力；$ {\mathrm{\sigma }}_{0} $：初始应力；$ \mathrm{\sigma}\mathrm{_f} $：最终应力；Dc：滑动弱化距离；Gc：破裂能Figure 3. A simple and typical slip-weakening friction law$ {\mathrm{\sigma }}_{{\mathrm{y}}} $：yield stress；$ {\mathrm{\sigma }}_{0} $：initial stress level；$ {\mathrm{\sigma }}_{{\mathrm{f}}} $：final stress；$ {{D}}_{\mathrm{c}} $：critical slip-weakening distance；$ {{G}}_{\mathrm{c}} $：fracture energy在滑动弱化摩擦准则（图3）中，滑动弱化距离Dc定义为断层面上剪切应力强度随滑动量减小到剩余应力时的滑动量。受断层面介质状态的影响（Guatteri，2001），不同地震Dc的取值存在较大差别：例如Ide和Takeo （1997）由波形反演结果计算得到的1995年Kobe地震的Dc范围在0.5—1.0 m；Olsen 等（1997）由试错法计算的1992年Landers地震的滑动弱化距离为0.8 m；Pulido和Irikura （2000）由断层面滑动速率函数计算得到的视应力空间分布估计Landers地震的Dc范围在1.0—3.5 m。随后的一些研究（Guatteri，2001；Mikumo et al，2003）结果显示，Dc与最终滑动量的比值在0.2—0.9之间；而结合实际地震的计算结果（Mikumo et al，2003；Zhang et al，2004）显示，Dc与最终滑动量的比值约为0.2—0.6。由于运动学反演采用重叠三角形或箱型函数作为震源时间函数时，存在应力缓慢弱化的现象，使最终的Dc值偏大，综合考虑，本文在动力学模拟过程中采用的Dc为非均匀分布模式，参考运动学反演模型的结果（Yang et al，2022），将Dc设为运动学最终滑动量的0.15倍。
在动力学模拟过程中，当断层面上初始应力场$ {\mathrm{\sigma }}_{0} $设为0时，应力降可以根据运动学滑动量由Andrews （1980）提出的在波数域里的沿断层面滑动方向剪切应力变化与滑动分布之间的关系计算得到。依据震源表示定理（Aki，Richards，1980），在无限均匀各向同性弹性介质中，在断层系坐标中（x1：走向，x2：倾向，x3：法向）对于给定断层面${\boldsymbol{x}} $上的滑动分布$ {D}_{n} （ \mathit{x} ） $（其中${\boldsymbol{D}} $为滑动矢量，n＝1，2，3），当滑动位移仅沿断层面走向方向（$ {x}_{1} $）时（位于$ {x}_{3}＝0 $平面内），Andrews （1974，1978，1980）及Ripperger 和Mai （2004）采用2D傅里叶变换给出了频率域中剪切应力与滑动量之间的关系：
$$ {\Delta \tau }_{21} （ k ） ＝{K}_{21} （ k ） D （ k ） \text{，} $$ (1) $$ {\Delta \tau }_{23} （ k ） ＝{K}_{23} （ k ） D （ k ） \text{，} $$ (2) 式中，$ {\Delta \tau }_{21} （ k ） $和$ {\Delta \tau }_{23} （ k ） $分别为沿平行和垂直断层走向方向的剪切应力改变量，$ k $为波数，$ D （ k ） $为滑动谱，$ {K}_{21} （ k ） $和$ {K}_{23} （ k ） $为仅与波数和Lamé常数（$ \lambda $，$ \mu $）有关的刚度函数：
$$ {K}_{21} （ k ） ＝－\dfrac{\mu }{2}\dfrac{1}{k}\left[\dfrac{2 （ \lambda ＋\mu ） }{\lambda ＋2\mu }{k}_{21}^{2}＋{k}_{23}^{2}\right] \text{，} $$ (3) $$ {K}_{23} （ k ） ＝－\dfrac{\mu }{2}\dfrac{1}{k}\left[\dfrac{2 （ \lambda ＋\mu ） }{\lambda ＋2\mu }－1\right]{k}_{21}{k}_{23} \text{，} $$ (4) 式中，$ {k}_{21} $和$ {k}_{23} $分别为沿平行和垂直滑动方向的波数，$ {k＝ （ {k}_{21}^{2}＋{k}_{23}^{2} ） }^{1/2} $。由式（1）和式（2）进行逆傅里叶变换到时间域即可得到应力降。图4给出了由Yang等（2022）的运动学滑动量计算得到主断层（F2和F3）和分支断层（F1）的静态应力降。
![]()
图 4 由运动学滑动量计算得到的静态应力降（a，b）和随深度变化的屈服应力（c，d）图（a）和（c）为主断层F2、F3；图（b）和（d）为分支断层F1；黑色虚线为分支断层交切的位置，下同Figure 4. Distribution of static stress drop （a，b） and yield stress （c，d） on the faultFigs.（a） and （c） are main faults F2，F3；Figs.（b） and （d） are branching faults F1. The black dashed line shows the location of the branching fault intersection，the same below基于水力压裂（Zoback et al，1977）和应变释放法（Haimson，1978）测量的上地壳构造应力的近似值显示，场地应力的绝对值随着深度的增加而增加；谢张迪等（2024）的研究结果显示，在初始应力和滑动弱化距离Dc确定的条件下，整体改变屈服应力进行动力学模拟可以调整震源破裂时间。假设屈服应力$ {\sigma }_{{\mathrm{y}}} $随深度发生变化（Aochi，Madariaga，2003）：
$$ \sigma_{\mathrm{y}}＝\delta H\text{，} $$ (5) 式中：$ {\sigma }_{y} $为屈服应力，单位为MPa；H为深度，单位为km；$ \delta $为常数转换因子。本文将Yang 等（2022）的运动学反演结果（断层面最终滑动量、地震矩、震源破裂时间）作为约束，采用试错法确定$ \delta $值。基于破裂相图（Madariaga，Olsen，2000；Xu et al，2015）可知，当应力降和Dc确定时，$ {\sigma }_{{\mathrm{y}}} $值从小到大分别会产生超剪切破裂、亚剪切破裂和自停止破裂，且超剪切的震源破裂时间小于次剪切；Zhang等（2003）采用三维有限差分法解弹性动力学方程得到的1999年集集地震的屈服应力和谢张迪等（2024）采用Andrews （1980）方法计算的2018年日本北海道地震的屈服应力均显示，屈服应力最大值约为静态应力降最大值的10%；由本文计算的应力降（图4）可知，当$ \delta $＝0.1时，与之前的研究结果相近，即$ {\sigma }_{{\mathrm{y}}}－{\sigma }_{0} $的最大值约为$ {\sigma }_{0}－{\mathrm{\sigma }}_{{\mathrm{f}}} $最大值的10 %；因此，自$ \delta $＝0.1开始取值，当动力学的震源破裂时间大于运动学结果时，说明屈服应力偏小，需要增大$ \delta $值，反之则减小$ \sigma\mathrm{_y} $值。当动力学模拟得到的门源地震震源破裂时间与Yang 等（2022）采用反射投影方法（Wang et al，2016）得到的主震能量释放时间、断层面滑动量和矩震级相近时，得到断层面上合适的屈服应力（图4c-d）。同时，为了产生初始破裂，在震源处设置半径为1.5 km的圆形区域作为成核区，对成核区内部的屈服应力单独降低0.1 MPa，使初始应力超过屈服应力。动力学模拟过程中的时间阶步设置为0.000 5 s，模拟总时间为25 s。
3. 动力学模拟结果
采用上文确定的动力学参数，并以Yang等（2022）的运动学结果（地震矩、断层面滑动量和震源破裂时间）为约束进行动力学模拟后，可以得到半空间实际地形条件下、断层面上滑动量和滑动速率最大值的空间分布（图5）及滑动速率随时间的演化过程（图6）。动力学模拟产生的最大滑动量约为3.6 m，位于震源上方约5 km （图5a），矩震级为MW6.6，与运动学结果（Yang et al，2022）较为相似；在断层分支处，滑动速率最大值由主断层（F2，F3）的约3.6 m/s下降到分支断层（F1）的约2.5 m/s （图5c-d），且滑动量也存在明显的阶跃下降现象（图5a-b）。由动力学破裂过程（图6）可知：动力学破裂自震中开始，先沿断层面下倾方向破裂，产生较小的滑动速率；自第4.5 s开始向断层两侧及上倾方向破裂，与前人研究的双侧破裂结果一致（韩帅等，2022；许英才等，2022）；由第5.5 s至第7.5 s的破裂过程可知，在断层F2和F3的连接处存在高强度障碍体，从而产生非连续破裂（第6 s红色方框区域），在累积应力达到障碍体的屈服应力后，未破裂的区域重新发生破裂（第6.5—7.5 s），这一非连续破裂过程与数值模拟（Das，Aki，1977）中显示的破裂跳过障碍体继续传播的现象相似，Zhang等（2004）在模拟1999年集集地震动力学破裂过程中也揭示出相似的动力学跳跃破裂特征。门源地震动力学破裂过程中产生该非连续破裂的现象与震源区构造具有重要关系：门源地震体波速度成像（左可桢，陈继峰，2018；王琼等，2022；尹欣欣等，2022）显示，震源区上方存在高速异常，大地电磁成像也揭示了相似的结果（赵凌强等，2022）；由于高速异常区内介质强度的增加，其产生破裂所需的能量也随之增加（吴建平等，2009），在累积应力达到介质强度时，重新发生破裂，正如本文动力学模拟过程中第6.5—7.5 s （图6），高强度的应力积累得到释放。
![]()
图 5 动力学模拟得到的断层面上滑动量和最大滑动速率空间分布图（a）和（c）表示主断层F2和 F3；图（b）和（d）表示分支断层F1Figure 5. Spatial distribution of fault plane slip and maximum slip rate obtained from dynamic simulationFigs. （a） and （c） denote main faults F2and F3；Figs. （b） and （d） denote branching faults F1![]()
图 6 主断层（F2，F3）（a）与分支断层（F1）（b）的动力学滑动速率快照Figure 6. Snapshots of slip rate for the main faults （F2，F3） （a） and the branch fault （F1） （b）地震产生的强地面破裂与动力学破裂过程密切相关，且受强运动生成区的大小和上升时间控制（Miyake et al，2003）。由图5和图6的动力学破裂结果可知，断层面上侧较下侧滑动速率大，且震源区位于体波速度剧烈变化的区域（孙安辉等，2022；王琼等，2022），与日本2011年3月11日地震断层面强运动生成区的构造背景及动力学破裂特征（Yoshida et al，2012；Galvez et al，2020）相似，由此产生的高频辐射可能在地表产生较强的破坏性；另一方面，Madariaga （1977）和Day （1982）的研究表明，高频辐射与滑动速率的突然改变有关，如在本文模拟过程中托莱山断裂带与冷龙岭断裂带交会处分支断层的抑制作用使震源破裂过程产生滑动速率的突然下降（图5c-d）。在门源地震强运动生成区和分支断层处滑动速率改变产生的高频辐射的共同作用下，动力学模拟得到的综合PGV结果及与烈度的对应关系（GB/T 17742—2020）确定的烈度分布表明（图7a）：最大地震烈度为Ⅷ度，位于震中附近，北西向地震动衰减小于南东向，该结果与门源地震仪器烈度（图7b）的等震线长轴为北西走向的结果相近。
![]()
Figure 7. PGV obtained from dynamic simulation （a） and instrumental seismic intensity distribution of MenyuanMS6.9 earthquake （Strong Motion Observation Group，Institute of Engineering Mechanics，China Earthquake Administration，2022；Yin et al，2022）（b）4. 讨论与结论
4.1 断层几何对动力学模拟结果的影响
北东向挤压和南东向拓展，但之前的两次地震震源机制均为逆冲型（徐纪人等，1986；梁姗姗等，2017），与本次地震的左旋走滑（万永革等，2022；许英才等，2022）具有明显的差异，因此，2022年青海门源地震的发震机制可能是由断层几何控制（冯万鹏等，2023）。张丽芬（2016）与董森和张海明（2019）采用边界积分方法研究Y型分叉断层的动力学破裂过程时发现，分叉断层之间的夹角对破裂传播过程具有较强的影响，如果分叉断层存在较小的夹角，可能会抑制破裂的传播，即“应力影区效应”（Yamashita，Umeda，1994）：当破裂传播到分叉断层时，如果分支的一侧发生破裂，其断层周围的累积应力得到释放，另一侧断层的应力强度也随之下降，很难再次发生破裂。这与本文采用夹角约20°的曲面分支断层动力学模拟存在的滑动量和滑动速率的阶跃下降（图5）现象相似。
为确定本文动力学模拟过程中出现的滑动速率、滑动量和应力降的阶跃下降现象是否与断层几何形状有关，本文参考上述所设动力学参数，分别单独模拟F2和F3断层与F1和F3断层的动力学破裂过程。图8给出了这两种模型动力学初始应力（图8a，c）和模拟得到的滑动量（图8b，d）：在不存在分支断层的情况下，滑动量（图8b，d）并未出现分支断层中的阶跃下降现象。由此可知，门源地震的破裂过程中产生的“Y”型分支断层，抑制了托莱山东段累积应力的释放，分支断层条件下动力学模拟结果（图5）与野外实地调查（潘家伟等，2022）显示的托莱山断裂东段较冷龙岭断裂西段地表破裂较小的现象一致。
![]()
图 8 两种断层模型及其动力学模拟结果图（a）和 （b）分别为F1，F3的初始应力和模拟结果；图（c）和 （d）分别为F2，F3的初始应力和模拟结果Figure 8. Two fault models and their dynamic simulation resultsFigs.（a） and （b） are the initial stresses and simulation results for F1 and F3，respectively；Figs.（c） and （d） are the initial stresses and simulation results for F2 and F3，respectively4.2 托莱山断裂东段地震危险性
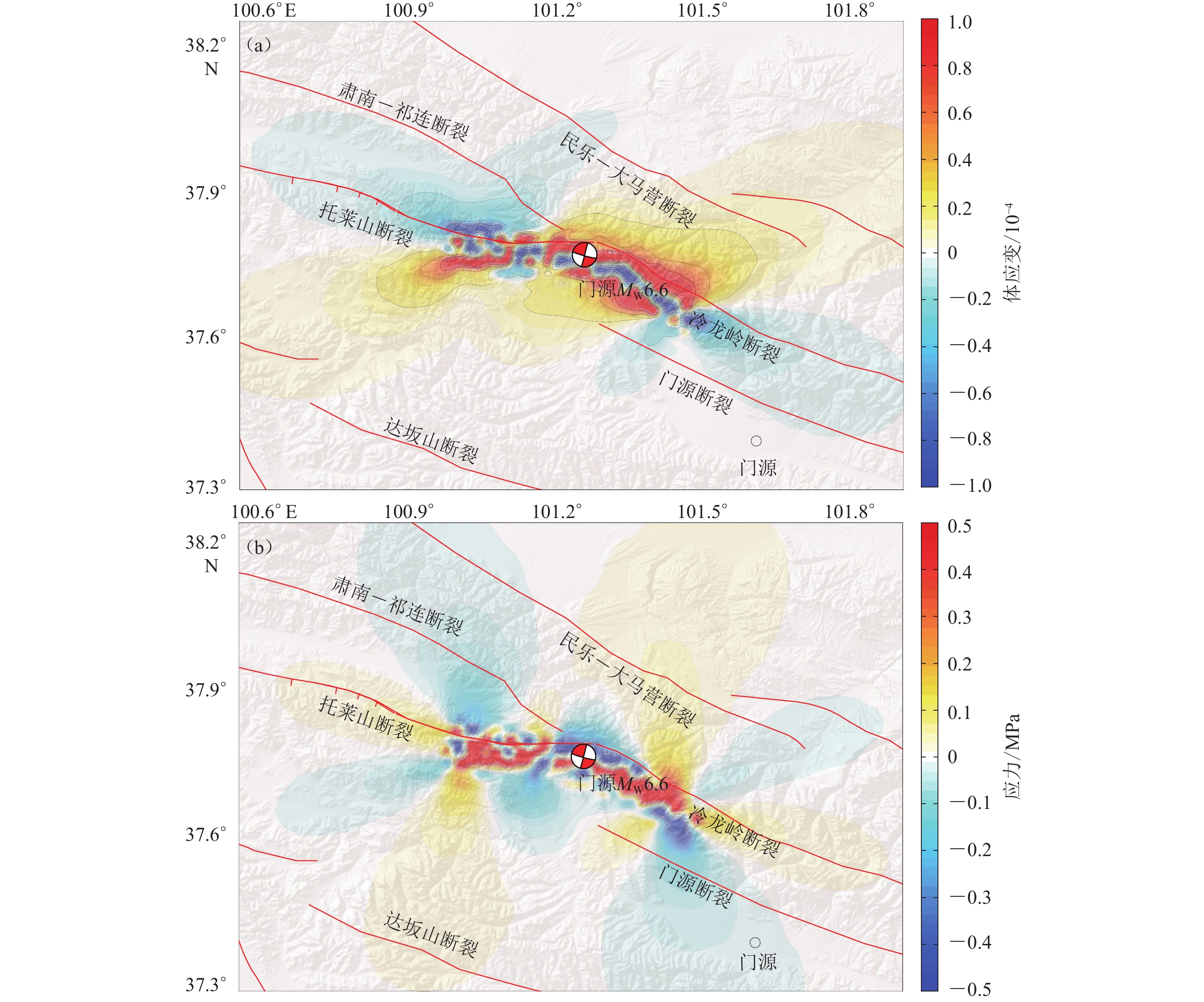
为了更好地研究门源地震对周围地区的影响，揭示托莱山断裂东段的地震危险性，采用动力学结果和Coulomb3.3软件（Lin，Stein，2004；Toda et al，2005），在有效摩擦系数0.4、计算深度10 km的条件下，得到了门源地震在震源区产生的体应变和库仑应力变化量（图9）。体应变结果（图9a）显示，震中位置NE和SW以拉张应力为主，对应侧为挤压，具有明显的走滑断层四象限分布特征。该结果与门源地震所处的青藏高原东北缘北东向挤压、南东向拓展的构造背景（Gan et al，2007；赵凌强等，2022）及震源区应力ENE—WSW向拉张、NNW−SSE向挤压应力场（万永革等，2022）一致。此外，门源地震产生的库仑应力结果表明（图9b）：震中西侧处于应力加载状态，尤其是托莱山断裂东段，库仑破裂应力变化约为0.5 MPa，远大于0.01 MPa的触发后继地震阈值（Harris，1998），同震库仑应力和剪应力分析也得到了相似的结果（许英才等，2022；余鹏飞等，2022；冯万鹏等，2023）。刘雷等（2023）采用跨断层GPS数据的分析结果显示，F1断层（托莱山断裂东段）存在长约50 km的地震空区，具有强闭锁特征，地震危险性较强。结合上文的动力学计算结果可知，分支断层对破裂过程的抑制主要存在于F1断层。因此，F1断层上动力学破裂的两种情况得到的滑动量差值，即为F1断层残留应力的释放量，采用均匀介质模型（vP＝6.4 km/s，vS＝3.5 km/s，密度为
2800 kg/m3）可估算得到相当于矩震级为MW5.1的能量（标量地震矩为5.0×1016 N·m），由于该值仅采用了F1断层的面积，其可能为托莱山断裂东段未来震级的下限值。![]()
图 9 门源地震动力学模型计算的体应变（a）和库仑破裂应力（b）Figure 9. Volumetric strain （a） and Coulomb rupture stress （b） produced by the Menyuan earthquake dynamic model4.3 结论
本文采用谱元法模拟了2022年青海门源MW6.6地震在实际地形下的动力学破裂过程，其结果显示，破裂自初始破裂点开始向两侧传播。受震源区上方高速P波异常区的影响，在托莱山断裂东段和冷龙岭断裂西段交界处产生非连续破裂且近地表的滑动速率达到约3.6 m/s；同时，由于分支断层之间夹角较小，托莱山断裂东段的破裂受到强烈的抑制作用，滑动量和滑动速率在分支断层交界处存在阶跃下降的现象。因此，分支断层处滑动速率的阶跃下降和近地表的强运动生成区产生的高频辐射共同作用，是门源地震产生强地表破裂的主因。
门源地震产生的各分量应变结果表明：托莱山断裂东段的南西侧和冷龙岭断裂西段的北东侧以拉张应力为主，对应侧为挤压，与震源区所处的青藏高原东北缘北东向挤压、南东向拓展的构造背景及震源区ENE−SWS向拉张、NNW−SSE向挤压应力场一致，说明此次门源地震破裂过程主要受区域应力场的控制。
此外，门源地震动力学破裂过程显示，冷龙岭断裂西段积累的应力已在地震中基本释放，但托莱山断裂东段残留震级的下限约为MW5.1，且托莱山断裂东段的库仑应力处于加载状态，最大库仑应力约为0.5 MPa，远大于触发阈值0.01 MPa （Harris，1998）。由此，托莱山断裂东段处于较大的地震危险性。
文中所使用门源地震运动学模型来源于Yang 等（2022），震源机制解数据来源于GCMT （2022）；动力学数值模拟代码为Specfem3D_Cartesian，文中图件采用Matplotlib和GMT绘制。两位匿名审稿专家对本文提出的宝贵意见，作者在此一并表示感谢。
-
![]()
图 8 两种断层模型及其动力学模拟结果
图（a）和 （b）分别为F1，F3的初始应力和模拟结果；图（c）和 （d）分别为F2，F3的初始应力和模拟结果
Figure 8. Two fault models and their dynamic simulation results
Figs.（a） and （b） are the initial stresses and simulation results for F1 and F3，respectively；Figs.（c） and （d） are the initial stresses and simulation results for F2 and F3，respectively
![]()
图 1 门源MW6.6地震和研究区内历史强震分布（GCMT，2022）
黑点为余震震中分布（数据源自Fan et al，2022）；红色实线为区域内活断层在地表的投影（数据源自徐锡伟等，2016）；绿框为进行强地面运动模拟的范围
Figure 1. Distribution of the Mengyuan MW6.6 earthquake and historical strong earthquakes in the region （GCMT，2022 ）
The black dots are the distribution of aftershock epicenters （data from Fan et al，2022）；the red solid line is the projection of active faults on the surface in the region （data from Xu et al，2016）；the green box is the range wherestrong ground motion simulations were performed
![]()
图 2 数值计算空间和包含实际地形的曲面分支断层模型（Yang et al，2022）
F1为托莱山断裂东段的分支断层；F2和F3为冷龙岭断裂的西段主断层
Figure 2. Numerical computational space and a curved surface branch fault model （Yang et al，2022） that includes actual terrain
F1 is the branch fault of easternTuolaishan fault；F2 and F3 are the main faults of the western Lenglongling fault
![]()
图 3 滑动弱化摩擦准则
$ {\sigma }_{\mathrm{y}} $：屈服应力；$ {\mathrm{\sigma }}_{0} $：初始应力；$ \mathrm{\sigma}\mathrm{_f} $：最终应力；Dc：滑动弱化距离；Gc：破裂能
Figure 3. A simple and typical slip-weakening friction law
$ {\mathrm{\sigma }}_{{\mathrm{y}}} $：yield stress；$ {\mathrm{\sigma }}_{0} $：initial stress level；$ {\mathrm{\sigma }}_{{\mathrm{f}}} $：final stress；$ {{D}}_{\mathrm{c}} $：critical slip-weakening distance；$ {{G}}_{\mathrm{c}} $：fracture energy
![]()
图 4 由运动学滑动量计算得到的静态应力降（a，b）和随深度变化的屈服应力（c，d）
图（a）和（c）为主断层F2、F3；图（b）和（d）为分支断层F1；黑色虚线为分支断层交切的位置，下同
Figure 4. Distribution of static stress drop （a，b） and yield stress （c，d） on the fault
Figs.（a） and （c） are main faults F2，F3；Figs.（b） and （d） are branching faults F1. The black dashed line shows the location of the branching fault intersection，the same below
![]()
图 5 动力学模拟得到的断层面上滑动量和最大滑动速率空间分布
图（a）和（c）表示主断层F2和 F3；图（b）和（d）表示分支断层F1
Figure 5. Spatial distribution of fault plane slip and maximum slip rate obtained from dynamic simulation
Figs. （a） and （c） denote main faults F2and F3；Figs. （b） and （d） denote branching faults F1
![]()
图 6 主断层（F2，F3）（a）与分支断层（F1）（b）的动力学滑动速率快照
Figure 6. Snapshots of slip rate for the main faults （F2，F3） （a） and the branch fault （F1） （b）
![]()
图 7 动力学模拟计算得到的PGV （a）和门源地震仪器烈度（中国地震局工程力学研究所强震观测组，2022；尹晓菲等，2022）（b）
Figure 7. PGV obtained from dynamic simulation （a） and instrumental seismic intensity distribution of MenyuanMS6.9 earthquake （Strong Motion Observation Group，Institute of Engineering Mechanics，China Earthquake Administration，2022；Yin et al，2022）（b）
![]()
图 9 门源地震动力学模型计算的体应变（a）和库仑破裂应力（b）
Figure 9. Volumetric strain （a） and Coulomb rupture stress （b） produced by the Menyuan earthquake dynamic model
表 1 门源地震动力学破裂模拟采用的层状速度 结构（Fan et al，2022）
Table 1 Layered velocity structure used in the Menyuan earthquake dynamics rupture simulation （Fan et al，2022）
层数 vP/（km·s−1） vS/（km·s−1） 深度上边界/km 1 5.0 2.9 0 2 5.2 3.0 2.5 3 6.1 3.5 5.8 4 6.6 3.8 16.3 5 6.9 4.0 28.1 6 8.2 4.7 57.0 
下载: 导出CSV
-
董森,张海明. 2019. 角度对于Y型分叉断层自发破裂传播过程的影响[J]. 地球物理学报,62(11):4156–4169. doi: 10.6038/cjg2019M0572 Dong S,Zhang H M. 2019. Effects of angles on dynamic ruptures on Y-type branched faults[J]. Chinese Journal of Geophysics,62(11):4156–4169 (in Chinese).
冯万鹏,何骁慧,张逸鹏,房立华,Samsonov S,张培震. 2023. 2022年青海门源MW6.6地震的发震断层及孕震构造模式[J]. 科学通报,68(2/3):254–270. Feng W P,He X H,Zhang Y P,Fang L H,Samsonov S,Zhang P Z. 2023. Seismic faults of the 2022 MW6.6 Menyuan,Qinghai earthquake and their implication for the regional seismogenic structures[J]. Chinese Science Bulletin,68(2/3):254–270 (in Chinese).
韩帅,吴中海,高扬,卢海峰. 2022. 2022年1月8日青海门源MS6.9地震地表破裂考察的初步结果及对冷龙岭断裂活动行为和区域强震危险性的启示[J]. 地质力学学报,28(2):155–168. Han S,Wu Z H,Gao Y,Lu H F. 2022. Surface rupture investigation of the 2022 Menyuan MS6.9 Earthquake,Qinghai,China:Implications for the fault behavior of the Lenglongling fault and regional intense earthquake risk[J]. Journal of Geomechanics,28(2):155–168 (in Chinese).
李振洪,韩炳权,刘振江,张苗苗,余琛,陈博,刘海辉,杜静,张双成,朱武. 2022. InSAR数据约束下2016年和2022年青海门源地震震源参数及其滑动分布[J]. 武汉大学学报•信息科学版,47(6):887–897. Li Z H,Han B Q,Liu Z J,Zhang M M,Yu C,Chen B,Liu H H,Du J,Zhang S C,Zhu W. 2022. Source parameters and slip distributions of the 2016 and 2022 Menyuan,Qinghai earthquakes constrained by InSAR observations[J]. Geomatics and Information Science of Wuhan University,47(6):887–897 (in Chinese).
李智敏,盖海龙,李鑫,袁道阳,谢虹,姜文亮,李永生,苏琦. 2022. 2022年青海门源MS6.9级地震发震构造和地表破裂初步调查[J]. 地质学报,96(1):330–335. Li Z M,Gai H L,Li X,Yuan D Y,Xie H,Jiang W L,Li Y S,Su Q. 2022. Seismogenic fault and coseismic surface deformation of the Menyuan MS6.9 earthquake in Qinghai,China[J]. Acta Geologica Sinica,96(1):330–335 (in Chinese).
梁宽,何仲太,姜文亮,李永生,刘泽民. 2022. 2022年1月8日青海门源MS6.9地震的同震地表破裂特征[J]. 地震地质,44(1):256–278. doi: 10.3969/j.issn.0253-4967.2022.01.016 Liang K,He Z T,Jiang W L,Li Y S,Liu Z M. 2022. Surface rupture characteristics of the Menyuan MS6.9 earthquake on January 8,2022,Qinghai Province[J]. Seismology and Geology,44(1):256–278 (in Chinese).
梁姗姗,雷建设,徐志国,邹立晔,刘敬光. 2017. 2016年1月21日青海门源MS6.4余震序列重定位和主震震源机制解[J]. 地球物理学报,60(6):2091–2103. doi: 10.6038/cjg20170606 Liang S S,Lei J S,Xu Z G,Zou L Y,Liu J G. 2017. Relocation of the aftershock sequence and focal mechanism solutions of the 21 January 2016 Menyuan,Qinghai,MS6.4 earthquake[J]. Chinese Journal of Geophysics,60(6):2091–2103 (in Chinese).
刘雷,朱良玉,季灵运,庄文泉,刘传金. 2023. 2022年门源MS6.9地震前祁连—海原断裂带闭锁程度及地震危险性研究[J]. 地震研究,46(1):88–98. Liu L,Zhu L Y,Ji L Y,Zhuang W Q,Liu C J. 2023. Study of the locking degree of the Qilian−Haiyuan fault zone before the 2022 Menyuan MS6.9 earthquake and its seismic risk in future[J]. Journal of Seismological Research,46(1):88–98 (in Chinese).
潘家伟,李海兵,Chevalier M L,刘栋梁,李超,刘富财,吴琼,卢海建,焦利青. 2022. 2022年青海门源MS6.9地震地表破裂带及发震构造研究[J]. 地质学报,96(1):215–231. doi: 10.3969/j.issn.0001-5717.2022.01.018 Pan J W,Li H B,Chevalier M L,Liu D L,Li C,Liu F C,Wu Q,Lu H J,Jiao L Q. 2022. Coseismic surface rupture and seismogenic structure of the 2022 MS6.9 Menyuan earthquake,Qinghai Province,China[J]. Acta Geologica Sinica,96(1):215–231 (in Chinese).
孙安辉,高原,赵国峰,任超,梁姗姗. 2022. 2022年1月8日青海门源6.9级地震的震源区结构特征和b值意义初探[J]. 地球物理学报,65(3):1175–1183. doi: 10.6038/cjg2022Q0030 Sun A H,Gao Y,Zhao G F,Ren C,Liang S S. 2022. Seismic structure and b-value in the focal area of the 8th January 2022 Menyuan,Qinghai MS6.9 earthquake[J]. Chinese Journal of Geophysics,65(3):1175–1183 (in Chinese).
万永革,许鑫,黄少华,崔华伟,冯淦,李枭. 2022. P波极性资料确定的2022青海门源MS6.9地震序列震源机制及应力场[J]. 地震工程学报,44(3):670–679. Wan Y G,Xu X,Huang S H,Cui H W,Feng G,Li X. 2022. Focal mechanisms and stress field of the 2022 Menyuan,Qinghai MS6.9 earthquake sequence determined by P-wave polarity data[J]. China Earthquake Engineering Journal,44(3):670–679 (in Chinese).
王琼,肖卓,武粤,李抒予,高原. 2022. 2022年1月8日青海门源MS6.9地震深部构造背景浅析[J]. 地震学报,44(2):211–222. doi: 10.11939/jass.20220010 Wang Q,Xiao Z,Wu Y,Li S Y,Gao Y. 2022. The deep tectonic background of the MS6.9 Menyuan earthquake on January 8,2022 in Qinghai Province[J]. Acta Seismologica Sinica,44(2):211–222 (in Chinese).
吴建平,黄媛,张天中,明跃红,房立华. 2009. 汶川MS8.0级地震余震分布及周边区域P波三维速度结构研究[J]. 地球物理学报,52(2):320–328. Wu J P,Huang Y,Zhang Z T,Ming Y H,Fang L H. 2009. Aftershock distribution of the MS8.0 Wenchuan earthquake and three dimensional P-wave velocity structure in and around source region[J]. Chinese Journal of Geophysics,52(2):320–328 (in Chinese).
谢张迪,于湘伟,章文波. 2024. 2018年日本北海道MW6.6地震震源动力学破裂过程[J]. 地球物理学报,67(8):2972–2989. doi: 10.6038/cjg2023Q0942 Xie Z D,Yu X W,Zhang W B. 2024. Dynamic rupture process of the 2018 Hokkaido MW6.6 earthquake,Japan[J]. Chinese Journal of Geophysics,67(8):2972–2989 (in Chinese).
徐纪人,姚立珣,汪进. 1986. 1986年8月26日门源6.4级地震及其强余震的震源机制解[J]. 西北地震学报,8(4):82–84. Xu J R,Yao L X,Wang J. 1986. Earthquake source mechanisms of Menyuan earthquake (MS=6.4 on Aug.26,1986) and its strong aftershocks[J]. Northwestern Seismological Journal,8(4):82–84 (in Chinese).
徐锡伟,韩竹军,杨晓平,张世民. 2016. 中国及邻近地区地震构造图[M]. 北京:地震出版社. Xu X W,Han Z J,Yang X P,Zhang S M. 2016. Seismic Tectonic Map of China and Its Adjacent Areas[M]. Beijing:Seismological Press (in Chinese).
许英才,郭祥云,冯丽丽. 2022. 2022年1月8日青海门源MS6.9 地震序列重定位和震源机制解研究[J]. 地震学报,44(2):195–210. doi: 10.11939/jass.20220008 Xu Y C,Guo X Y,Feng L L. 2022. Relocation and focal mechanism solutions of the MS6.9 Menyuan earthquake sequence on January 8,2022 in Qinghai Province[J]. Acta Seismologica Sinica,44(2):195–210 (in Chinese).
薛善余,谢虹,袁道阳,李智敏,苏瑞欢,文亚猛. 2022. 2022门源MS6.9地震地表破裂带震害特征调查[J]. 地震工程学报,44(2):458–467. Xue S Y,Xie H,Yuan D Y,Li Z M,Su R H,Wen Y M. 2022. Seismic disaster characteristics of the surface rupture of Menyuan MS6.9 earthquake in 2022[J]. China Earthquake Engineering Journal,44(2):458–467 (in Chinese).
尹晓菲,王芃,张伟,邵志刚,曹文忠,熊仁伟. 2022. 2022年1月8日青海门源MS6.9地震强地面运动模拟及烈度分布估计[J]. 地震学报,44(2):237–244. doi: 10.11939/jass.20220011 Yin X F,Wang P,Zhang W,Shao Z G,Cao W Z,Xiong R W. 2022. Strong ground motion simulation and intensity distribution estimation for the MS6.9 Menyuan,Qinghai,earthquake on 8 January 2022[J]. Acta Seismologica Sinica,44(2):237–244 (in Chinese).
尹欣欣,邱江涛,李敏娟,许康生,张波,张胜霞. 2022. 2022年门源MS6.9地震震区三维速度与发震机制研究[J]. 地震工程学报,44(2):360–369. Yin X X,Qiu J T,Li M J,Xu K S,Zhang B,Zhang S X. 2022. Three-dimensional velocity structure and seismogenic mechanism of Menyuan MS6.9 earthquake in 2022[J]. China Earthquake Engineering Journal,44(2):360–369 (in Chinese).
余鹏飞,陈威,乔学军,赵斌,李刚,熊维. 2022. 基于多源SAR数据的2022年门源MS6.9地震同震破裂模型反演研究[J]. 武汉大学学报•信息科学版,47(6):898–906. Yu P F,Chen W,Qiao X J,Zhao B,Li G,Xiong W. 2022. Slip model of the 2022 Menyuan MS6.9 earthquake constrained by mulit-source SAR data[J]. Geomatics and Information Science of Wuhan University,47(6):898–906 (in Chinese).
张丽芬. 2016. 不同因素对于断层动力学破裂过程复杂性的影响研究[D]. 北京:中国地震局地球物理研究所:49−53. Zhang L F. 2016. Influences of Different Factors on the Complexities of Fault Dynamic Rupture Propagation[D]. Beijing:Institute of Geophysics,China Earthquake Administration :49−53(in Chinese).
赵凌强,孙翔宇,詹艳,杨海波,王庆良,郝明,刘雪华. 2022. 2022年1月8日青海门源MS6.9地震孕震环境和冷龙岭断裂分段延展特征[J]. 地球物理学报,65(4):1536–1546. doi: 10.6038/cjg2022Q0051 Zhao L Q,Sun X Y,Zhan Y,Yang H B,Wang Q L,Hao M,Liu X H. 2022. The seismogenic model of the Menyuan MS6.9 earthquake on January 8,2022,Qinghai Province and segmented extensional characteristics of the Lenglongling fault[J]. Chinese Journal of Geophysics,65(4):1536–1546 (in Chinese).
中国地震局工程力学研究所强震观测组. 2022. 2022年01月08日青海门源6.9级地震仪器烈度分布图[EB/OL]. [2022−01−20]. https://mp.weixin.qq.com/s/IGp1dw7KfFY3PiwWT2WY6A. Strong Motion Observation Group,Institute of Engineering Mechanics,China Earthquake Administration. 2022. Instrument intensity distribution map of the Menyuan,Qinghai MS6.9 earthquake on January 8th,2022[EB/OL]. [2022−01−20]. https://mp.weixin.qq.com/s/IGp1dw7KfFY3PiwWT2WY6A (in Chinese).
中国地震台网中心. 2022. 青海海北州门源县6.9级地震[EB/OL]. [2022−01−10]. https://news.ceic.ac.cn/CC20220108014528.html. China Earthquake Networks Center. 2022. MS6.9 Menyuan earthquake in Haibei Prefecture,Qinghai Province[EB/OL]. [2022−01−10]. https://news.ceic.ac.cn/CC20220108014528.html (in Chinese).
左可桢,陈继锋. 2018. 门源地区地壳三维体波速度结构及地震重定位研究[J]. 地球物理学报,61(7):2788–2801. doi: 10.6038/cjg2018L0537 Zuo K Z,Chen J F. 2018. 3D body-wave velocity structure of crust and relocation of earthquakes in the Menyuan area[J]. Chinese Journal of Geophysics,61(7):2788–2801 (in Chinese).
Aki K,Richards P G. 1980. Quantitative Seismology:Theory and Methods[M]. San Francisco:W. H. Freeman & Co:28−30.
Andrews D J. 1974. Evaluation of static stress on a fault plane from a Green's function[J]. Bull Seismol Soc Am,64(6):1629–1633. doi: 10.1785/BSSA0640061629
Andrews D J. 1976. Rupture velocity of plane strain shear cracks[J]. J Geophys Res,81(32):5679–5687. doi: 10.1029/JB081i032p05679
Andrews D J. 1978. Coupling of energy between tectonic processes and earthquakes[J]. J Geophys Res:Solid Earth,83(B5):2259–2264. doi: 10.1029/JB083iB05p02259
Andrews D J. 1980. A stochastic fault model:1. Static case[J]. J Geophys Res:Solid Earth,85(B7):3867–3877. doi: 10.1029/JB085iB07p03867
Aochi H,Madariaga R. 2003. The 1999 İzmit,Turkey,earthquake:Nonplanar fault structure,dynamic rupture process,and strong ground motion[J]. Bull Seismol Soc Am,93(3):1249–1266. doi: 10.1785/0120020167
Bouchon M. 1997. The state of stress on some faults of the San Andreas system as inferred from near-field strong motion data[J]. J Geophys Res:Solid Earth,102(B6):11731–11744. doi: 10.1029/97JB00623
Carlson J M,Langer J S. 1989. Mechanical model of an earthquake fault[J]. Phys Rev A,40(11):6470–6484. doi: 10.1103/PhysRevA.40.6470
Das S,Aki K. 1977. Fault plane with barriers:A versatile earthquake model[J]. J Geophys Res,82(36):5658–5670. doi: 10.1029/JB082i036p05658
Day S M. 1982. Three-dimensional simulation of spontaneous rupture:The effect of nonuniform prestress[J]. Bull Seismol Soc Am,72(6A):1881–1902. doi: 10.1785/BSSA07206A1881
Dieterich J H. 1978. Time-dependent friction and the mechanics of stick-slip[J]. Pure Appl Geophys,116(4):790–806.
Dieterich J H. 1979. Modeling of rock friction:1. Experimental results and constitutive equations[J]. J Geophys Res:Solid Earth,84(B5):2161–2168. doi: 10.1029/JB084iB05p02161
Fan L P,Li B R,Liao S R,Jiang C,Fang L H. 2022. High-precision relocation of the aftershock sequence of the January 8,2022,MS6.9 Menyuan earthquake[J]. Earthquake Science,35(2):138–145. doi: 10.1016/j.eqs.2022.01.021
Galvez P,Petukhin A,Irikura K,Somerville P. 2020. Dynamic source model for the 2011 Tohoku earthquake in a wide period range combining slip reactivation with the short-period ground motion generation process[J]. Pure Appl Geophys,177(5):2143–2161. doi: 10.1007/s00024-019-02210-7
Galvez P,Ampuero J P,Dalguer L A,Somala S N,Nissen-Meyer T. 2014. Dynamic earthquake rupture modelled with an unstructured 3-D spectral element method applied to the 2011 M9 Tohoku earthquake[J]. Geophys J Int,198(2):1222–1240. doi: 10.1093/gji/ggu203
Gan W J,Zhang P Z,Shen Z K,Niu Z J,Wang M,Wan Y G,Zhou D M,Cheng J. 2007. Present day crustal motion within the Tibetan Plateau inferred from GPS measurements[J]. J Geophys Res:Solid Earth,112(B8):B08416.
GCMT. 2022. Global CMT Catalog Search[EB/OL]. [2022−06−30]. https://www.globalcmt.org/CMTsearch.html.
Guatteri M. 2001. Inferring Fault Rupture Dynamics from Strong Motion Data[D]. Palo Alto:Stanford University.
Haimson B C. 1978. Crustal stress in the Michigan basin[J]. J Geophys Res:Solid Earth,83(B12):5857–5863. doi: 10.1029/JB083iB12p05857
Harris R A. 1998. Introduction to special section:Stress triggers,stress shadows,and implications for seismic hazard[J]. J Geophys Res:Solid Earth,103(B10):24347–24358. doi: 10.1029/98JB01576
Harris R A,Barall M,Archuleta R,Dunham E,Aagaard B,Ampuero J P,Bhat H,Cruz-Atienza V,Dalguer L,Dawson P,Day S,Duan B,Ely G,Kaneko Y,Kase Y,Lapusta N,Liu Y,Ma S,Oglesby D,Olsen K,Pitarka A,Song S,Templeton E. 2009. The SCEC/USGS dynamic earthquake rupture code verification exercise[J]. Seismol Res Lett,80(1):119–126. doi: 10.1785/gssrl.80.1.119
Ida Y. 1972. Cohesive force across the tip of a longitudinal-shear crack and Griffith's specific surface energy[J]. J Geophys Res,77(20):3796–3805. doi: 10.1029/JB077i020p03796
Ide S,Takeo M. 1997. Determination of constitutive relations of fault slip based on seismic wave analysis[J]. J Geophys Res:Solid Earth,102(B12):27379–27391. doi: 10.1029/97JB02675
Kaneko Y,Lapusta N,Ampuero J P. 2008. Spectral element modeling of spontaneous earthquake rupture on rate and state faults:Effect of velocity-strengthening friction at shallow depths[J]. J Geophys Res:Solid Earth,113(B9):B09316.
Komatitsch D,Vilotte J P. 1998. The spectral element method:An efficient tool to simulate the seismic response of 2D and 3D geological structures[J]. Bull Seismol Soc Am,88(2):368–392. doi: 10.1785/BSSA0880020368
Komatitsch D,Tromp J. 1999. Introduction to the spectral element method for three-dimensional seismic wave propagation[J]. Geophys J Int,139(3):806–822. doi: 10.1046/j.1365-246x.1999.00967.x
Komatitsch D,Tromp J. 2002a. Spectral-element simulations of global seismic wave propagation: Ⅰ . Validation[J]. Geophys J Int,149(2):390–412. doi: 10.1046/j.1365-246X.2002.01653.x
Komatitsch D,Tromp J. 2002b. Spectral-element simulations of global seismic wave propagation: Ⅱ . Three-dimensional models,oceans,rotation and self-gravitation[J]. Geophys J Int,150(1):303–318. doi: 10.1046/j.1365-246X.2002.01716.x
Komatitsch D,Tsuboi S,Tromp J. 2005. The spectral-element method in seismology[M]//Seismic Earth:Array Analysis of Broadband Seismograms. Washington:American Geophysical Union:205.
Liao J T,Liang C T,Wang C L,Cao F H,Ye C M,Yang Y H. 2022. Source model for the 2022 Qinghai Menyuan MS6.9 earthquake based on D-InSAR[J]. Front in Earth Science,10:948661. doi: 10.3389/feart.2022.948661
Lin J,Stein R S. 2004. Stress triggering in thrust and subduction earthquakes and stress interaction between the southern San Andreas and nearby thrust and strike-slip faults[J]. J Geophys Res:Solid Earth,109(B2):B02303.
Madariaga R. 1977. High-frequency radiation from crack (stress drop) models of earthquake faulting[J]. Geophys J Int,51(3):625–651. doi: 10.1111/j.1365-246X.1977.tb04211.x
Madariaga R,Olsen K B. 2000. Criticality of rupture dynamics in 3-D[M]//Microscopic and Macroscopic Simulation:Towards Predictive Modelling of the Earthquake Process. Basel:Birkhäuser:1981−2001.
Mikumo T,Olsen K B,Fukuyama E,Yagi Y. 2003. Stress-breakdown time and slip-weakening distance inferred from slip-velocity functions on earthquake faults[J]. Bull Seismol Soc Am,93(1):264–282. doi: 10.1785/0120020082
Miyake H,Iwata T,Irikura K. 2003. Source characterization for broadband ground-motion simulation:Kinematic heterogeneous source model and strong motion generation area[J]. Bull Seismol Soc Am,93(6):2531–2545. doi: 10.1785/0120020183
Olsen K B,Madariaga R,Archuleta R J. 1997. Three-dimensional dynamic simulation of the 1992 Landers earthquake[J]. Science,278(5339):834–838. doi: 10.1126/science.278.5339.834
Pulido N,Irikura K. 2000. Estimation of dynamic rupture parameters from the radiated seismic energy and apparent stress[J]. Geophys Res Lett,27(23):3945–3948. doi: 10.1029/2000GL011658
Ripperger J,Mai P M. 2004. Fast computation of static stress changes on 2D faults from final slip distributions[J]. Geophys Res Lett,31(18):L18610.
Ruina A. 1983. Slip instability and state variable friction laws[J]. J Geophys Res:Solid Earth,88(B12):10359–10370. doi: 10.1029/JB088iB12p10359
Toda S,Stein R S,Richards-Dinger K,Bozkurt S B. 2005. Forecasting the evolution of seismicity in southern California:Animations built on earthquake stress transfer[J]. J Geophys Res:Solid Earth,110(B5):B05S16.
Wang D,Kawakatsu H,Mori J,Ali B,Ren Z,Shen X. 2016. Backprojection analyses from four regional arrays for rupture over a curved dipping fault:the MW7.7 24 September 2013 Pakistan earthquake[J]. J Geophys Res:Solid Earth,121(3):1948–1961.
Weng H H,Ampuero J P. 2019. The dynamics of elongated earthquake ruptures[J]. J Geophys Res:Solid Earth,124(8):8584–8610. doi: 10.1029/2019JB017684
Xu J K,Zhang H M,Chen X F. 2015. Rupture phase diagrams for a planar fault in 3-D full-space and half-space[J]. Geophys J Int,202(3):2194–2206. doi: 10.1093/gji/ggv284
Yamashita T,Umeda Y. 1994. Earthquake rupture complexity due to dynamic nucleation and interaction of subsidiary faults[J]. Pure Appl Geophys,143(1):89–116.
Yang H F,Wang D,Guo R M,Xie M Y,Zang Y,Wang Y,Yao Q,Cheng C,An Y R,Zhang Y Y. 2022. Rapid report of the 8 January 2022 MS6.9 Menyuan earthquake,Qinghai,China[J]. Earthquake Research Advances,2(1):100113. doi: 10.1016/j.eqrea.2022.100113
Yoshida K,Petukhin A,Miyakoshi K,Hada K,Okazaki A. 2012. Source process and constitutive relations of the 2011 Tohoku earthquake inferred from near-field strong-motion data[C]//Proceedings of the 15th World Conference on Earthquake Engineering. Lisbon:Curran Associates, Inc:3876.
Zhang W B,Iwata T,Irikura K,Sekiguchi H,Bouchon M. 2003. Heterogeneous distribution of the dynamic source parameters of the 1999 Chi-Chi,Taiwan,earthquake[J]. J Geophys Res:Solid Earth,108(B5):2232.
Zhang W B,Iwata T,Irikura K,Pitarka A,Sekiguchi H. 2004. Dynamic rupture process of the 1999 Chi-Chi,Taiwan,earthquake[J]. Geophys Res Lett,31(10):L10605.
Zoback M D,Healy J H,Roller J C. 1977. Preliminary stress measurements in central California using the hydraulic fracturing technique[J]. Pure Appl Geophys,115(1):135–152.
计量
- 文章访问数: 69
- HTML全文浏览量: 25
- PDF下载量: 35
