Morphological structure of stagnant slab under southern Japan and Ryukyu Island Arc based on seismic waveform fitting
-
摘要:
在日本南部及琉球岛弧地区，太平洋板块和菲律宾海板块共同向欧亚板块下方俯冲，形成复杂的双板块俯冲系统。滞留板片在地幔过渡带内的形态特征及其与地幔物质的相互作用影响着东海陆架盆地及中国大陆边缘的构造演化过程。本研究基于中国国家数字台网记录的发生于伊豆—小笠原地区的一次深源地震的宽频带波形资料，通过P波三重震相拟合，获得了日本南部及琉球岛弧下方660 km间断面附近的速度结构特征。结果显示，研究区域660 km间断面上方普遍存在高速异常体，其深度范围约为490—720 km，P波速度异常1.0%—3.0%；660 km间断面下沉深度为20—60 km，呈现出由南向北逐渐减小的趋势，速度跃变1.5%—3.0%。相较于前人基于地震活动性和层析成像的结果，本文高速异常体的存在位置要深于菲律宾海板块俯冲所能到达的地幔转换区上边界附近，因而其应为俯冲滞留的西太平洋俯冲板片物质，与菲律宾海板块俯冲物质无关；此外，660 km间断面较大的南北下沉深度变化应由俯冲板块带来的滞留物质及其引起的温度异常和相关的相变所导致。
Abstract:Under southern Japan and Ryukyu Island Arc, the Pacific plate and the Philippine Sea plate both subducted under the Eurasian plate, forming a complex double-plate subduction system. Statistical results of seismic activity reflect that there are significant differences in the influence of the two subducted plates on the mantle transition zone. It is currently unclear whether the stagnant slab beneath this region is a single Pacific plate or a mixed stagnant body consisting of the Pacific plate and the Philippine Sea plate. The properties, geometric characteristics, and morphological changes, as well as its interaction with the mantle material, collectively affect the tectonic evolution of the East China Sea Shelf Basin and the Chinese continental margin. The accumulation of the stagnant slab within the mantle transition zone and its interaction with the mantle material can cause variations in the depth of the 660 km discontinuity at the bottom of the mantle transition zone. Cold stagnant slab material induces depression of the 660 km discontinuity. By studying the depth of the 660 km discontinuity and its nearby velocity structure, we can explore the depth variation characteristics of the stagnant slab in the mantle transition zone beneath the southern Japan and Ryukyu Island Arc, obtain the lateral differences of the plate subduction process. This will deepen our understanding of the integrity of plate subduction in the Northwest Pacific Ocean and contribute to the study of the geodynamic processes of plate subduction. When the seismic wave passes through the 660 km discontinuity, the triplicated seismic phases will be generated. Utilizing the arrival times and amplitude characteristics of the triplicated waveforms recorded by a dense seismic network, we can effectively constrain the morphology of the discontinuity and the velocity structure near it.
In this study, we selected a deep earthquake that occurred in the Izu-Bonin recorded by the China National Seismic Network （CNSN）. The earthquake had a focal depth of 472 km, which avoided the influence of the triplicated waveforms associated with the 410 km discontinuity. The surface projections of the P-wave turning points are located in the southern Japan and Ryukyu Island Arc, and the characteristics of the triplicated waveforms can be used to constrain the depth of the 660 km discontinuity and its nearby velocity structure. Through identification and analysis of the morphological characteristics in P-wave triplicated waveforms, we divided the study area into 13 profiles, delineating horizontal differences in velocity structures based on distinct features of triplicated waveforms features. Building upon previous research findings and the characteristics of observed waveforms, we constructed a foundational velocity structure model. Employing a grid search methodology, we generated various parameter models. Theoretical seismic waveforms were subsequently computed using reflectivity method and compared against observed waveforms. The optimal velocity structure was determined via maximization of cross-correlation coefficients. Specifically, during the fitting process of P-wave triplicated waveforms, we refined waveform selection to minimize interference from non-triplicated waveforms, thereby augmenting fitting precision.
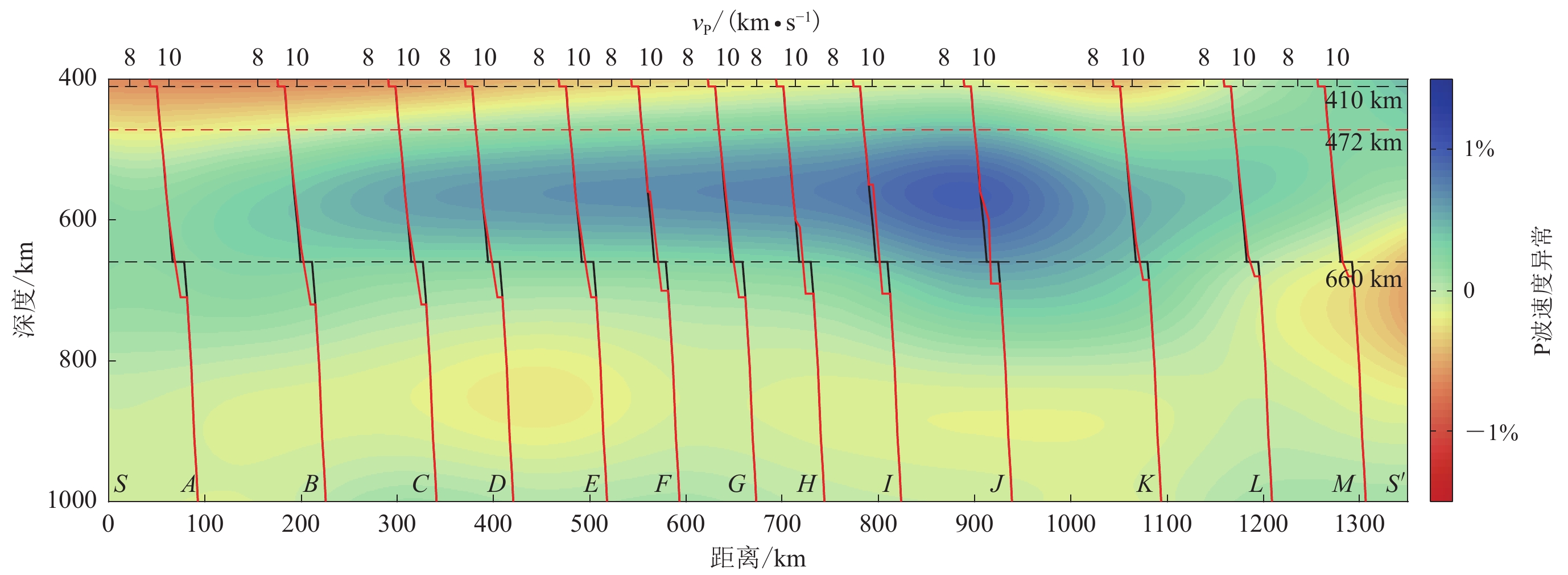
Our results reveal that: ① There is a high-velocity layer above the 660 km discontinuity with depth of 490−720 km and P-wave velocity anomaly of 1.0%−3.0%; ② The 660 km discontinuity depressed 20−60 km with velocity increment of 1.5%−3.0%, and the depression depth gradually decreases from south to north.
Drawing on insights gleaned from seismic tomography and tele-seismic data, we propose that the thickness of the high-velocity anomaly layer above the 660 km discontinuity significantly surpasses that of the subducting Pacific slab. This discrepancy likely arises from the halting of the downward motion of the subducted oceanic slab within the mantle transition zone by the 660 km discontinuity. This interruption triggers a lateral shift in motion, leading to the entrapment and subsequent buildup of the slab material within the mantle transition zone. Moreover, compared to previous studies based on seismic activity and tomography, our findings position the high-velocity anomaly layer at a depth deeper than typically reachable by the subduction of the Philippine Sea Plate, suggesting its composition primarily comprises material from the stagnant western Pacific slab rather than from the Philippine Sea Plate. Additionally, the depression of the 660 km discontinuity ranges from 20 to 80 km, exceeding depths attributed solely to thermal anomalies. We suggest that this depression may also involve phase transformations within the stagnant material brought by the subducted slab. The gradual reduction in depression depth from south to north likely reflects variations in subduction dynamics along the north-south direction.
-
Campbell （1982，1983）将贝叶斯概率理论和极值概率模型相结合，发展出一种估算地震发生概率的贝叶斯极值分布模型。在此模型中，地震活动的先验估计值是基于地震矩、滑动速率、地震复发率和震级等数据计算得到的，而后将估计值用于贝叶斯理论的后验估算，或者用于研究区的历史地震活动性的评估方面（李拴虎等，2016）。
假设极端地震发生的贝叶斯概率遵循时间和震级指数的泊松分布（Campbell，1982），在周期T内，贝叶斯估算的最大震级Mmax的概率有时会超过限定的震级幅度m，其基本方程为
$P\left( {{M_{{\rm{max}}}}{\text{＞}} m{\rm{|}}T} \right) {\text{＝}} 1 - {\left( {\frac{{t''}}{{t'' {\text{＋}} T\left[ {1 - F\left( m \right)} \right]}}} \right)^{n''}},$
(1) 式中：P（Mmax＞m|T）表示在周期T内，贝叶斯估算的最大震级Mmax大于阈值震级m的概率；n″为地震发生次数的后验贝叶斯估算值；t″为地震发生时间的后验贝叶斯估算值；F（m）为震级的贝叶斯分布。
一般情况下，假定地震是独立的随机事件，且同一时刻不会发生两次以上地震，则地震发生的时间符合泊松（函数）分布，即
$P\left( {N {\text{＝}} n{\rm{|}}v,t} \right) {\text{＝}} \frac{{{{\left( {vt} \right)}^n}{{\rm{e}}^{ - vt}}}}{{n!}},$
(2) 式中，P（N＝ n|v，t）表示在时间t内发生n次地震的概率，v为地震的平均发生率。考虑到估计值v的不确定性，使用式（3）能更准确地表示贝叶斯的分布函数（Benjamin，1968；Benja-min，Cornell，1970；Campbell，1982），并采用积分方程的形式表示，即
$P\left( {N {\text{＝}} n{\rm{|}}t} \right) {\text{＝}} \mathop \smallint \nolimits_0^\infty P\left( {N {\text{＝}} n{\rm{|}}v} \right)f''\left( v \right){\rm{d}}v,$
(3) 式中f″（v）表示v的后验概率密度函数，由地震发生的前验分布计算得到。假设地震的发生是一个泊松过程，不确定的v可以用伽马分布表示，Mortgat和Shah （1979）把另一个伽马分布应用于后验概率密度函数f″（v）的计算，即
$f''\left( v \right) {\text{＝}} {K_1}{v^{n'' - 1}}{{\rm{e}}^{ - vt''}},$
(4) 式中，标准常数K1可以表示为K1＝ t″n″/Γ（n″），Γ（n″）为参数n″的伽马函数，Campbell （1982）在式（3）的基础上得到了泊松-伽马分布函数，即
$P\left( {N {\text{＝}} n{\rm{|}}n'',t'',t} \right) {\text{＝}} \frac{{\Gamma \left( {n {\text{＋}} n''} \right)}}{{n!\ \Gamma \left( {n''} \right)}}{\left( {\frac{{t''}}{{t {\text{＋}} t''}}} \right)^{n''}}{\left( {\frac{t}{{t {\text{＋}} t''}}} \right)^n}.$
(5) 式（5）是依据地震发生的泊松分布和v的伽马分布推导出来的，给出了在时间t内发生n次地震事件的概率，且地震发生率的不确定性影响着泊松分布的参数（Galanis et al，2002 ）。参数n″和t″可用下列关系式计算得到，即
${n'' {\text{＝}} {n_0} {\text{＋}} {{\left( {\displaystyle\frac{{v'}}{{\sigma _{{v}}'}}} \right)}^2}},\quad {t'' {\text{＝}} {t_0} {\text{＋}} \displaystyle\frac{{v'}}{{{{\left( {\sigma _{{v}}'} \right)}^2}}}},$
(6) 式中，t0为有记录的历史地震的时间长度，n0为在时间t0内观测到的地震次数，σv ′为参数v的标准偏差的先验值。Campbell （1982）提出了预测地震震级的最终表达式，即双截断贝叶斯指数-伽马分布。
$F\left( {m{\rm{|}}{m_{\rm{l}}},{m_{\rm{u}}}} \right) {\text{＝}} K''\left[ {1 - {{\left( {\frac{{m''}}{{m'' {\text{＋}} m - {m_{\rm{l}}}}}} \right)}^{\eta ''}}} \right],$
(7) $K'' {\text{＝}} {\left[ {1 - {{\left( {\frac{{m''}}{{m'' {\text{＋}} {m_{\rm{u}}} - {m_{\rm{l}}}}}} \right)}^{\eta ''}}} \right]^{ - 1}},$
(8) 式中，mu和ml分别为研究区地震震级的高值和低值，η″为震级大于ml的地震事件数的后验贝叶斯评价，m″为震级介于m与ml之间的地震事件数的后验贝叶斯评价。
先验估计：为了评价震级大于ml的地震发生率v的先验值v′，Campbell （1982）及Stavrakakis和Drakopoulos （1995）推荐了v′的计算方法，即
$v' {\text{＝}} \frac{{\mu uA}}{{{M_0}\left( {{m_{\rm{u}}}} \right)}} \frac{{{C_2} - b'}}{{b'}}{10^{b'\left( {{m_{\rm{u}}} - {m_{\rm{l}}}} \right)}},$
(9) 式中：μ为剪切模量；u为滑动速率；A为断层总面积；M0（mu）为震级上限值的地震矩；参数b′为b的先验估计值，即lgN＝a−bM中的b值；系数C2的定义来自于表达式lgM0＝ C1+mC2。震级-频率参数的先验估计β′可以用下式计算，即
$\beta ' {\text{＝}} b\ln 10.$
(10) 后验估计：v″为震级M＞ml的地震平均发生率v的后验估计；震级-频率参数的后验估计β″的计算公式为
$\left\{ \begin{array}{l}v'' {\text{＝}} \displaystyle\frac{{n''}}{{t''}};\quad V_v^{''} {\text{＝}} \displaystyle\frac{1}{{\sqrt {n''} }};\quad V_v' {\text{＝}} \displaystyle\frac{{{\sigma _v}\!\!'}}{{v'}},\\\beta '' {\text{＝}} \displaystyle\frac{{\eta ''}}{{m''}};\quad V_\beta ^{''}{\text{＝}} \displaystyle\frac{1}{{\sqrt {\eta ''} }};\quad V_\beta ' {\text{＝}} \displaystyle\frac{{{\sigma _\beta }\!\!'}}{{\beta '}};\quad m'' {\text{＝}} {n_0}\left( {\overline m - {m_{\rm l}}} \right) {\text{＋}} \displaystyle\frac{{\beta '}}{{{{\left( {\sigma _\beta '} \right)}^2}}};\quad \eta '' {\text{＝}} {n_0} {\text{＋}} {\left( {\frac{{\beta '}}{{\sigma _\beta '}}} \right)^2}, \end{array} \right.$
(11) This page contains the following errors:
error on line 1 at column 1: Start tag expected, '<' not foundBelow is a rendering of the page up to the first error.
研究范围选取河套断陷带区域（106.3°E—110.5°E，40.1°N—41.4°N），该地区位于鄂尔多斯地块北缘，主要活动断裂有狼山山前断裂、正谊关断裂、乌拉山山前断裂和包头断裂等，具有较强的发震背景，同时河套平原又是人口和经济比较集中的地区，所以研究此区域的地震发震概率具有重大的科学意义。本研究所用的地震目录来源于中国地震台网中心，选取时段为1970—2017年，中国地震台网中心的地震目录提供了完整的地震信息，包括发震的地点、时间和震级，这是贝叶斯极值分布评估地震发生概率的基础数据。目前，经验性的公式MS＝0.8ML＋0.83被认为是适合中国的震级转换公式（Bormann et al，2007 ），但Wang等（2014a）通过对龙门山断裂带的研究，认为公式MS＝1.01ML－1.02更符合南北地震带的实际情况，由于本文研究区域处于南北地震带的北段，所以采用此经验公式来进行震级转换。研究区内特定震级的贝叶斯概率，分别使用时段t为5，20，100年来计算。
岩石力学测试表明，应力差Δσ与b值之间存在反比关系，所以b值被认为是地壳的“应力计”（Schorlemmer et al，2005 ），区域的应力水平可以通过b值来得以反映（Wiemer，Schorlem-mer，2007）。根据古登堡-里克特（Gutenberg-Richter，简写为G-R）公式lgN＝a−bM来计算b值，考虑到研究区地震目录的完整性，选取MS≥2.0的地震事件，通过地震目录分布情况拟合出G-R公式中的b值（图1）。
滑动速率u在平均地震发生率的估算中起着重要作用，Brune （1968）的模型尤其适合计算接近块体边界区域的滑动速率（Tselentis et al，1988 ），即
$u {\text{＝}} \frac{{\mathop \sum \nolimits {M_0}}}{{\mu {t_0}A}},$
(12) 式中，∑M0为过去t0时段内所发生地震的地震矩之和，A为断层滑动区域的面积，μ为剪切模量（Stavrakakis，Drakopoulos，1995）。地震矩的计算使用Hanks和Kanamori （1979）提出的公式lgM0＝16.1＋1.5M，剪切模量μ一般采用默认值3×104 MPa，此值通用于整个地壳。有多种方法可以计算A值，不同的计算方法会导致不同的结果，一般由多边形顶点测量出的A值可能是最大的（Wang et al，2014a ），但地震区域的边界不可能是直线，所以此测量方法只是一种近似计算。在本文中，较低震级为MS5.0，较大震级为目录时段内发生的最大地震的震级，贝叶斯计算所需参数值列于表1。
表 1 河套断陷带的贝叶斯估计参数Table 1. Parameters of the Bayesian estimate for the Hetao riftu/（cm·a–1） A/km2 历史最大震级MS mu $\scriptstyle{\overline m}$ 
n0 b 0.22 38 875 7.0 7.0 7.5 8.0 8.5 5.3 37 0.76 假设Vv′和Vβ′的3个变异系数均为0.10，0.25，1.0，河套断陷带的地震活动参数的后验估计列于表2。变异系数Vv是一个非常重要的参数（Campbell，1983；Stavrakakis，Drakopoulos，1995），会导致历史数据先验估计值出现偏差，分析Vv′＝0.1，0.25，1.0等3种情况下的结果可知：当Vv′＝1.0时，地震事件的发生主要由历史地震事件控制；当Vv′＝0.1时，地震事件的发生主要基于先验值的估计值（Campbell，1983；Parvez，2007）。贝叶斯分布的一个重要特点就是条件关联性，即把地震活动先验估计与历史地震事件结合起来，但是贝叶斯极值的灵敏度不会随着参数数量的增加而降低，不同来源的信息均应纳入到现有的统计范畴，以便更全面地评估孕震区内的地震危险性（Wang et al，2014b ，2015）。
表 2 研究区内参数v和β的先验估计和后验估计Table 2. Prior and posterior estimates of parameters v and β for the studied areamu 先验估计 后验估计 v′ β′ Vv′，Vβ′ v″ β″ Vv″，Vβ″ 7.0 4.84 1.54 0.10 0.36 1.64 0.09 4.84 1.54 0.25 0.15 1.84 0.14 4.84 1.54 1.00 0.11 1.98 0.16 7.5 1.86 1.54 0.10 0.33 1.64 0.09 1.86 1.54 0.25 0.15 1.84 0.14 1.86 1.54 1.00 0.11 1.98 0.16 8.0 0.72 1.54 0.10 0.28 1.64 0.09 0.72 1.54 0.25 0.14 1.84 0.14 0.72 1.54 1.00 0.11 1.98 0.16 8.5 0.28 1.54 0.10 0.19 1.64 0.09 0.28 1.54 0.25 0.13 1.84 0.14 0.28 1.54 1.00 0.11 1.98 0.16 在Vv′和Vβ′的3个变异系数分别为0.10，0.25，1.0的条件下，分别预测t为5，20，100年这3个时段内MS≥5.0地震的发生概率（图2）。地震发生概率随着震级的增大而不断衰减，当接近最大震级时，衰减最为严重。随着mu的减小和t的增大，地震事件的发生概率增大。研究中使用的起始震级为MS5.0，此震级通常被认为是破坏性地震的震级阈值（Parvez，2007）。在t＝5 a时，研究区内MS5.0地震的发震概率小于0.36，MS8.0地震的发震概率小于0.000 05。地震发生概率在MS＝5.0，Vv′＝0.1的条件下最小；在Vv′＝1.0，mu＝7.0，7.5，8.0，8.5时最大，与t值的相关性不大。当t＝5，20，100 a时，MS5.0地震的发震概率分别为0.06—0.36，0.20—0.82，0.68—1.0。
![]()
图 2 不同条件下的地震发生概率Figure 2. Probabilities of earthquake occurrence under different conditions基于极值分布的贝叶斯概率理论将各种不确定性的参数用于地震活动性的量化分析，其重要特点是当有新的信息加入模型时，当前的概率值也会随着变化，这将有利于地震活动、断裂构造、地质资料和历史观测资料等有用信息的整合，进而对地震发生的概率进行综合判定。这也体现出，当历史资料不是很完整、时间覆盖相对较短或数据量不充足时，贝叶斯概率理论则具有明显的优势。
本文地震目录采用中国地震台网中心的最新数据，成都理工大学王莹博士提供了计算程序并对计算进行了指导，作者在此一并表示感谢。
-
![]()
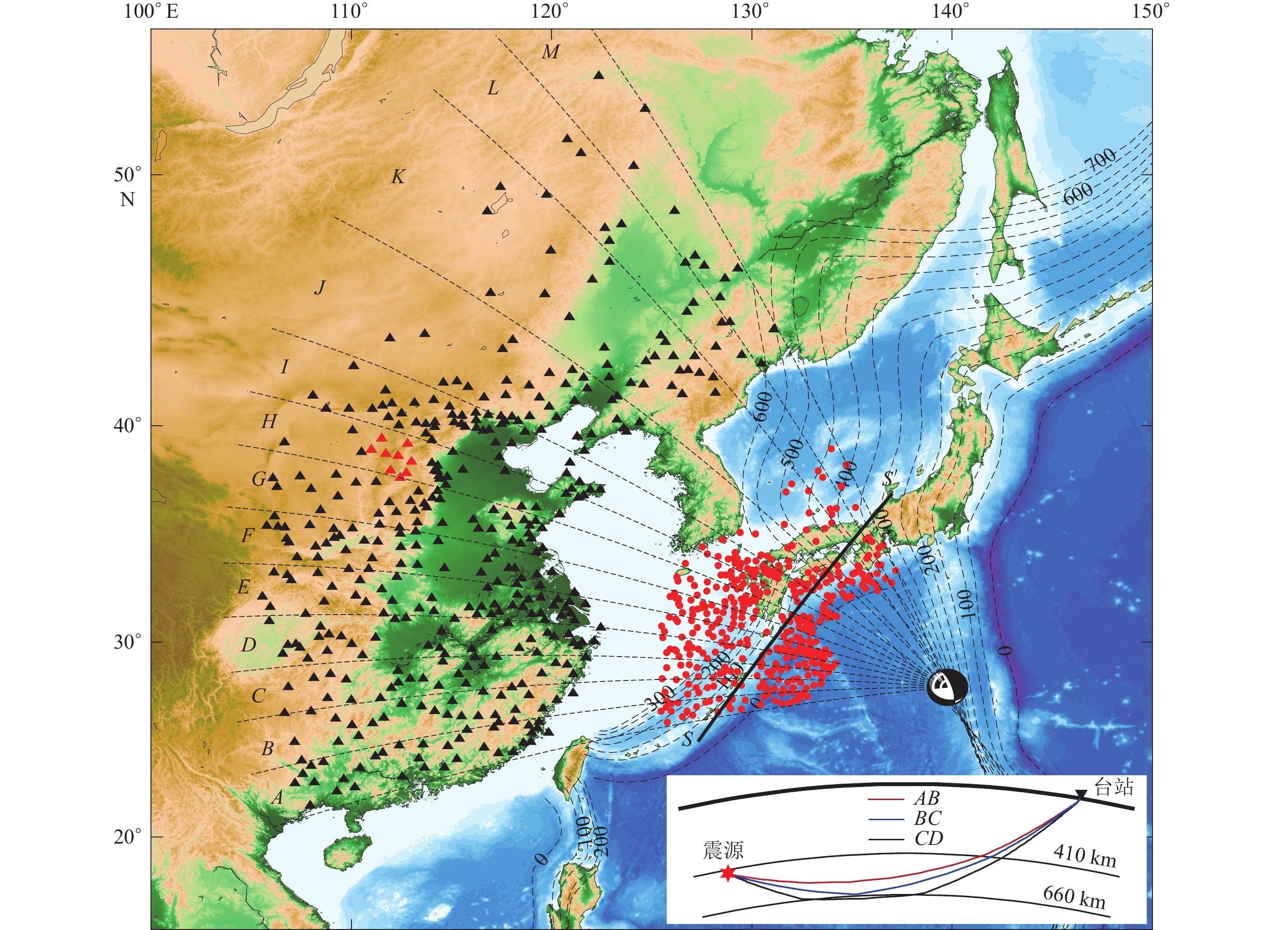
图 1 本文所用地震事件位置和台站分布图
黑色三角形为台站位置，按方位分布划分为13个剖面（A，B，···，M），红色三角形为剖面H上观测到到时延迟的台站；红色圆点为P波射线拐点的地表投影，黑色虚线为俯冲太平洋板片与菲律宾海板片等深线（Gudmundsson，Sambridge，1998）。右下角插图为660 km间断面附近三重震相射线路径图，包括地震射线AB，BC和CD
Figure 1. Location of the earthquake event and stations used in this study
Black triangles denote the used stations，and the studied region is divided into 13 profiles （A，B，···，M）. Red triangles in the profile H are the stations with obvious observed time delay. Red dots are the surface projections of P-wave turning points. Contours shown in black dashed lines represent the Pacific and Philippine Sea slabs （Gudmundsson，Sambridge，1998）. The bottom right inset shows ray paths of the triplication near the 660 km discontinuity，including the seismic rays AB，BC and CD
![]()
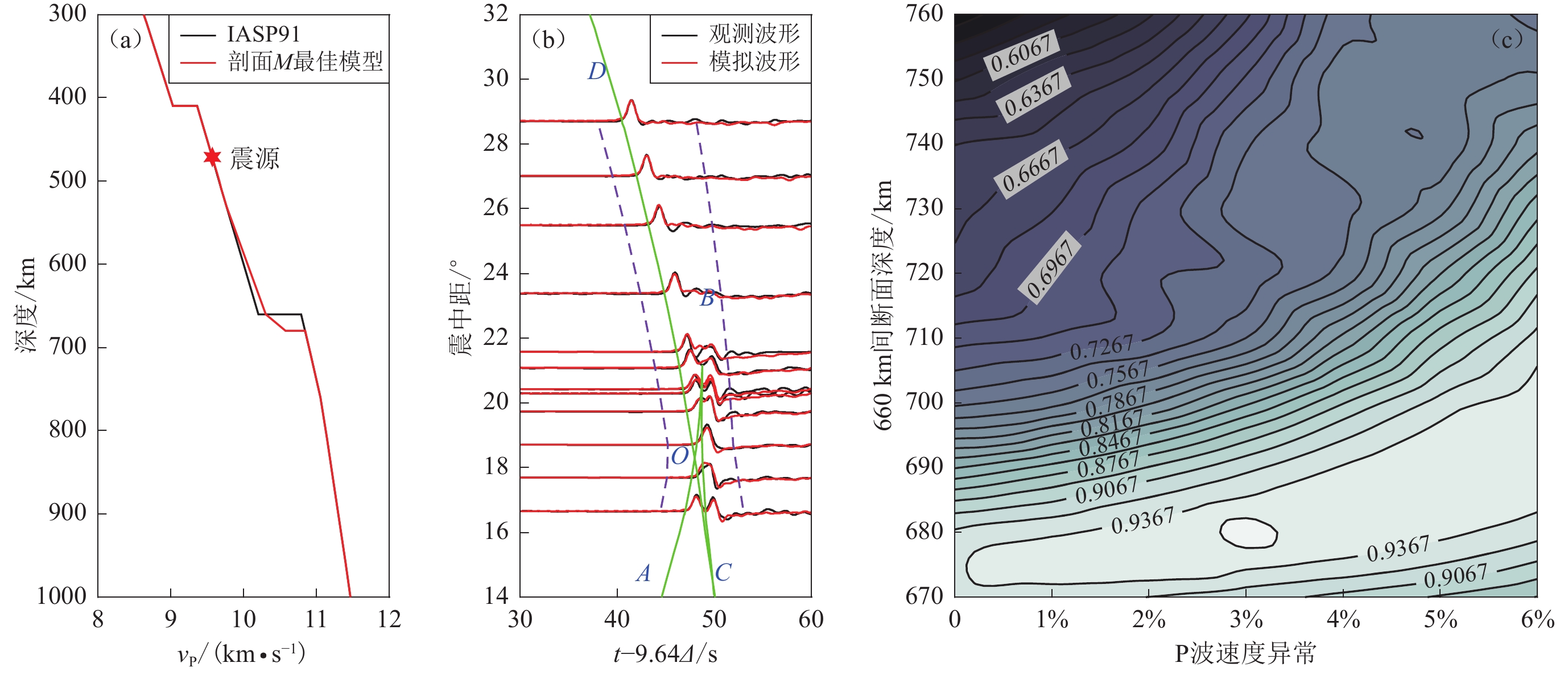
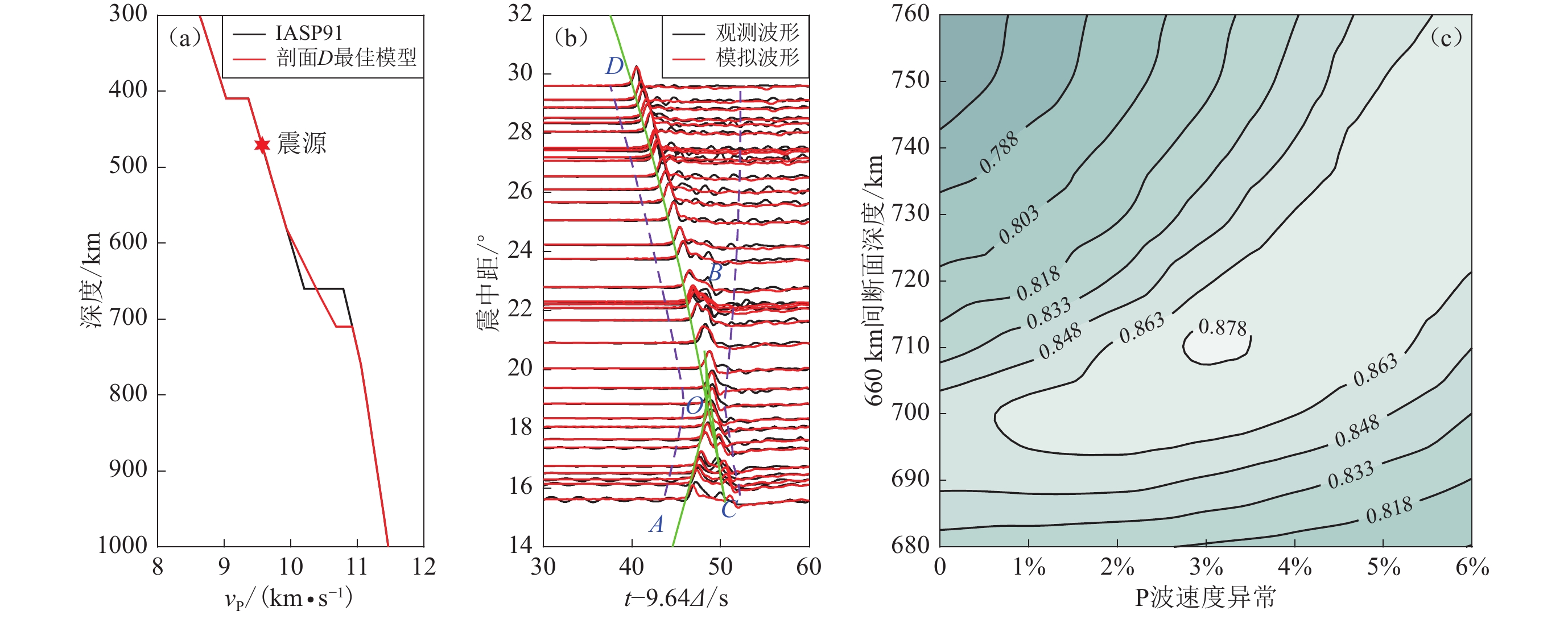
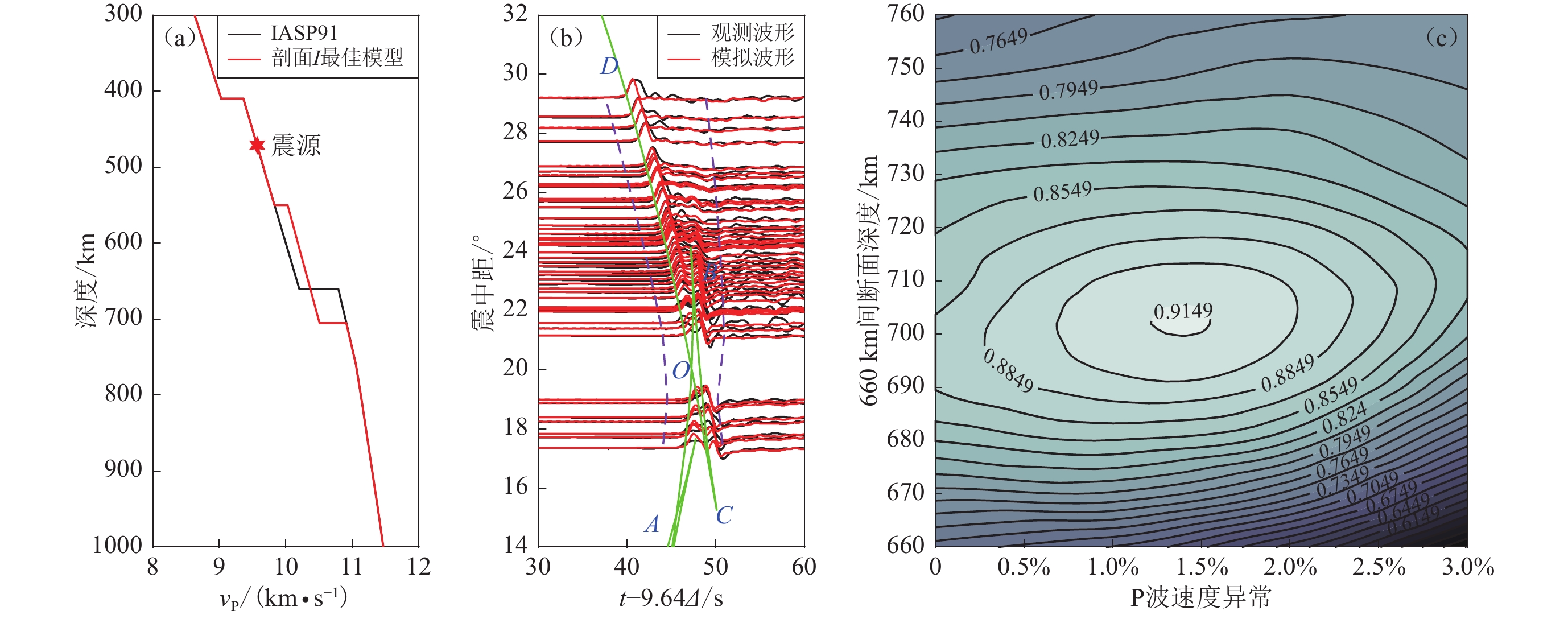
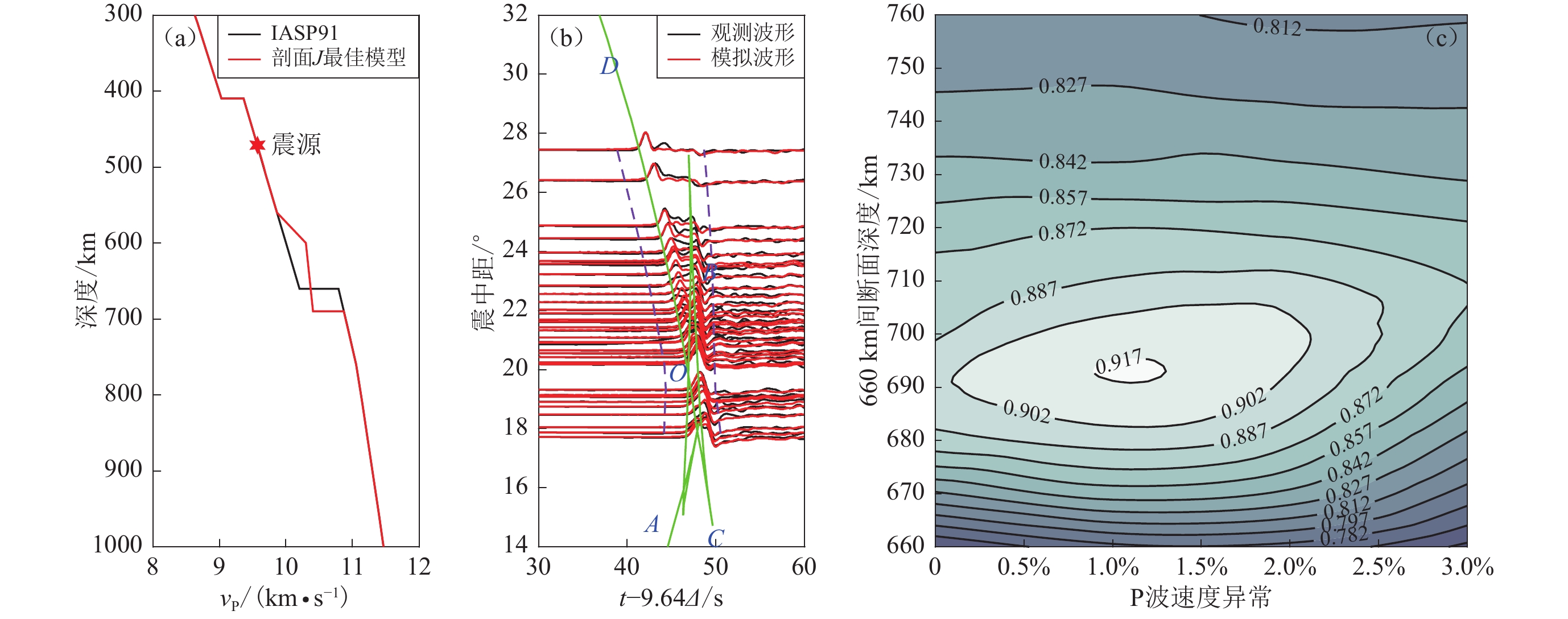
图 2 剖面A观测波形与模型参数定义图
（a） 剖面A上的观测波形及与IASP91模型对应的走时曲线；（b） 前人参考模型M3.11 （Tajima，Grand，1995）及本文构建模型参数定义图。d1 （480—600 km）表示660上方高速异常体起始深度，d2 （490—720 km）表示最大速度异常所处深度，d3 （660—760 km）表示660深度，s2 （0—4%）表示高速异常最大速度异常扰动量，s3 （0—5%）表示660的速度异常跃变值
Figure 2. The observed waveforms of the profile A and the parameter definition diagrams of the model
（a） The observed waveforms of the profile A and the travel time curves calculated with the IASP91；（b） Predecessor reference model M3.11 （Tajima，Grand，1995） and the parameter definition diagrams of the model used in the grid search procedure. d1 （480—600 km） is the starting depth of the high-velocity layer above the 660，d2 （490—720 km） is the depth at which the maximum velocity anomaly occurs，d3 （660—760 km） is the depth of the 660，s2 （0—4%） is the maximum velocity anomaly of the high-velocity layer，s3 （0—5%） is the velocity anomaly of the 660
![]()
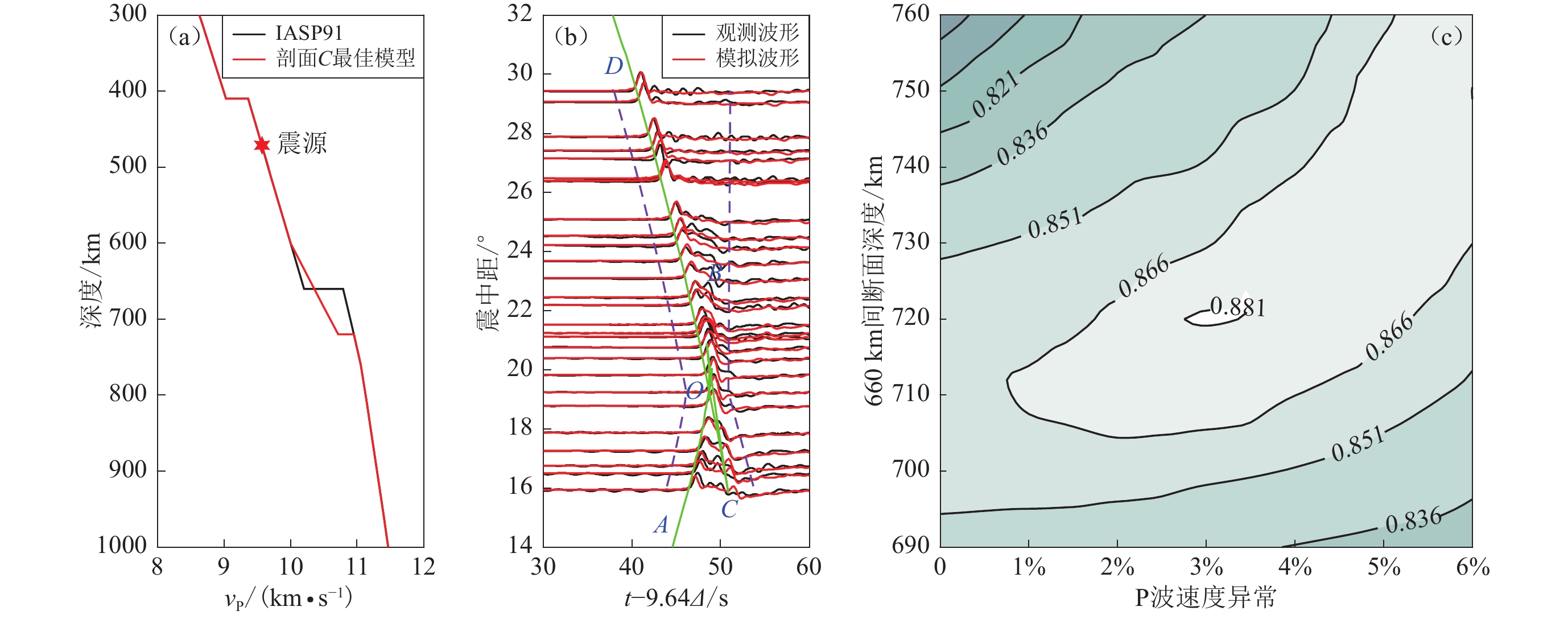
图 3 剖面A波形拟合结果及互相关系数等值线图
（a） P波速度结构；（b） 波形拟合结果。绿线表示由最佳模型计算得到的走时曲线，紫虚线框标记用于计算互相关系数的波形；（c） 660 km间断面深度与速度异常的互相关系数等值线图。下同
Figure 3. Waveform fitting results and cross-correlation coefficients contour map of profile A
（a） P-wave velocity structure；（b） Waveform fitting results. The green line represents travel time curve calculated with the best model. The purple dotted frame marks the waveforms used for calculating the cross-correlation coefficients；（c） Contour map of cross-correlation coefficients of the 660 km depth and velocity anomaly. The same below
![]()
图 15 剖面M波形拟合结果及互相关系数等值线图
Figure 15. Waveform fitting results and cross-correlation coefficients contour map of profile M
![]()
![]()
图 4 剖面B波形拟合结果及互相关系数等值线图
Figure 4. Waveform fitting results and cross-correlation coefficients contour map of profile B
![]()
图 5 剖面C波形拟合结果及互相关系数等值线图
Figure 5. Waveform fitting results and cross-correlation coefficients contour map of profile C
![]()
图 6 剖面D波形拟合结果及互相关系数等值线图
Figure 6. Waveform fitting results and cross-correlation coefficients contour map of profile D
![]()
图 7 剖面E波形拟合结果及互相关系数等值线图
Figure 7. Waveform fitting results and cross-correlation coefficients contour map of profile E
![]()
图 8 剖面F波形拟合结果及互相关系数等值线图
Figure 8. Waveform fitting results and cross-correlation coefficients contour map of profile F
![]()
图 9 剖面G波形拟合结果及互相关系数等值线图
Figure 9. Waveform fitting results and cross-correlation coefficients contour map of profile G
![]()
图 11 剖面I波形拟合结果及互相关系数等值线图
Figure 11. Waveform fitting results and cross-correlation coefficients contour map of profile I
![]()
图 12 剖面J波形拟合结果及互相关系数等值线图
Figure 12. Waveform fitting results and cross-correlation coefficients contour map of profile J
![]()
图 13 剖面K波形拟合结果及互相关系数等值线图
Figure 13. Waveform fitting results and cross-correlation coefficients contour map of profile K
![]()
图 14 剖面L波形拟合结果及互相关系数等值线图
Figure 14. Waveform fitting results and cross-correlation coefficients contour map of profile L
![]()
图 10 剖面H波形拟合结果及互相关系数等值线图
Figure 10. Waveform fitting results and cross-correlation coefficients contour map of profile H
表 1 各剖面速度模型
Table 1 The velocity models of each profile
剖面 高速异常体
起始深度/km高速异常体最大
速度异常深度/km高速异常体最大
速度异常660 km间断面
深度/km660 km间断面
速度跃变A 600 650 1.0% 710 2.0% B 540 640 1.0% 720 3.0% C 600 680 2.0% 720 3.0% D 580 670 2.0% 710 3.0% E 580 680 2.0% 710 3.0% F 560 560 1.0% 700 2.0% G 490 640 1.0% 710 2.0% H 600 610 2.0% 705 1.5% I 550 550 2.0% 705 1.5% J 560 600 3.0% 690 1.0% K 490 630 1.0% 685 2.5% L 530 650 1.0% 680 3.0% M 530 660 1.0% 680 3.0% 
下载: 导出CSV
-
崔辉辉,周元泽. 2016. 基于三重震相方法探测日本海俯冲区地幔转换带的速度结构[J]. 地震学报,38(5):659–670. doi: 10.11939/jass.2016.05.001 Cui H H,Zhou Y Z. 2016. Detecting the structure of the mantle transition zone in Japan subduction zone from the waveform triplications[J]. Acta Seismologica Sinica,38(5):659–670 (in Chinese).
崔辉辉,周元泽,石耀霖,王晓冉,李国辉. 2016. 华北克拉通东部滞留板块下方低速异常的地震三重震相探测[J]. 地球物理学报,59(4):1309–1320. Cui H H,Zhou Y Z,Shi Y L,Wang X R,Li G H. 2016. Seismic detection of a low-velocity anomaly under the stagnant slab beneath the eastern North China Craton with P-wave triplication[J]. Chinese Journal of Geophysics,59(4):1309–1320 (in Chinese).
地震科学国际数据中心. 2022. 地震科学国际数据中心[DB/OL]. [2023−11−25]. http://www.esdc.ac.cn:8080/static/frontSharePage.html. International Earthquake Science Data Center. 2022. International Earthquake Science Data Center[DB/OL]. [2023−11−25]. http://www.esdc.ac.cn:8080/static/frontSharePage.html (in Chinese).
瞿辰,周蕙兰,赵大鹏. 2007. 使用纵波和横波走时层析成像研究菲律宾海板块西边缘带和南海地区的深部结构[J]. 地球物理学报,50(6):1757–1768. Qu C,Zhou H L,Zhao D P. 2007. Deep structure beneath the west margin of Philippine Sea Plate and South China Sea from P and S wave travel time tomography[J]. Chinese Journal of Geophysics,50(6):1757–1768 (in Chinese).
苏慧,魏荣强,周元泽,崔清辉,李国辉. 2023. 东北亚边缘地区地幔过渡带内滞留太平洋板片上界面的三重震相研究[J]. 地球物理学报,66(6):2431–2444. Su H,Wei R Q,Zhou Y Z,Cui Q H,Li G H. 2023. Upper interface of the Pacific slab stagnant in the mantle transition zone beneath Northeast Asia edge derived from triplicated waveforms[J]. Chinese Journal of Geophysics,66(6):2431–2444 (in Chinese).
眭怡,周元泽. 2015. 利用三重震相探测中国东部海域410km深度低速层[J]. 地震学报,37(1):1–14. Sui Y,Zhou Y Z. 2015. Low-velocity anomaly around 410 km beneath the Yellow and East China Seas with P wave triplications[J]. Acta Seismologica Sinica,37(1):1–14 (in Chinese).
叶玲玲,李娟. 2012. 东北地区660km间断面附近波速结构研究[J]. 地震学报,34(2):137–146. Ye L L,Li J. 2012. Detecting velocity structure around 660-km discontinuity beneath northeastern China[J]. Acta Seismologica Sinica,34(2):137–146 (in Chinese).
张炎,钮凤林,宁杰远. 2022. 基于程函方程与三维速度模型的中国东北地区地幔过渡带接收函数研究[J]. 地球物理学报,65(8):2945–2959. Zhang Y,Niu F L,Ning J Y. 2022. Mantle transition zone beneath northeast China imaged by receiver function data using fast marching Eikonal solver based 3-D migration[J]. Chinese Journal of Geophysics,65(8):2945–2959 (in Chinese).
周春银,金振民,章军锋. 2010. 地幔转换带:地球深部研究的重要方向[J]. 地学前缘,17(3):90–113. Zhou C Y,Jin Z M,Zhang J F. 2010. Mantle transition zone:An important field in the studies of Earth’s deep interior[J]. Earth Science Frontiers,17(3):90–113 (in Chinese).
Bird P. 2003. An updated digital model of plate boundaries[J]. Geochem Geophys Geosyst,4(3):1027.
Buland R,Chapman C H. 1983. The computation of seismic travel times[J]. Bull Seismol Soc Am,73(5):1271–1302.
Cammarano F,Goes S,Vacher P,Giardini D. 2003. Inferring upper-mantle temperatures from seismic velocities[J]. Phys Earth Planet Inter,138(3/4):197–222.
Chu R S,Schmandt B,Helmberger D V. 2012. Upper mantle P velocity structure beneath the Midwestern United States derived from triplicated waveforms[J]. Geochem Geophys Geosyst,13(2):Q0AK04.
Chu R S,Zhu L P,Ding Z F. 2019. Upper-mantle velocity structures beneath the Tibetan Plateau and surrounding areas inferred from triplicated P waveforms[J]. Earth and Planetary Physics,3(5):445–459. doi: 10.26464/epp2019045
Courtier A M,Bagley B,Revenaugh J. 2007. Whole mantle discontinuity structure beneath Hawaii[J]. Geophys Res Lett,34(17):L17304.
Deuss A. 2007. Seismic observations of transition-zone discontinuities beneath hotspot locations[M]//Plates,Plumes,and Planetary Processes. Boulder:Geological Society of America:121−136.
Dziewoński A M,Chou T A,Woodhouse J H. 1981. Determination of earthquake source parameters from waveform data for studies of global and regional seismicity[J]. J Geophys Res Solid Earth,86(B4):2825–2852. doi: 10.1029/JB086iB04p02825
Ekström G,Nettles M,Dziewoński A M. 2012. The global CMT project 2004−2010:Centroid-moment tensors for 13,017 earthquakes[J]. Phys Earth Planet Inter,200-201:1–9. doi: 10.1016/j.pepi.2012.04.002
Engdahl E R,Van Der Hilst R,Buland R. 1998. Global teleseismic earthquake relocation with improved travel times and procedures for depth determination[J]. Bull Seismol Soc Am,88(3):722–743. doi: 10.1785/BSSA0880030722
Fei Y,Van Orman J,Li J,van Westrenen W,Sanloup C,Minarik W,Hirose K,Komabayashi T,Walter M,Funakoshi K. 2004. Experimentally determined postspinel transformation boundary in Mg2SiO4 using MgO as an internal pressure standard and its geophysical implications[J]. J Geophys Res Solid Earth,109(B2):B02305.
Fossen H. 2010. Structural Geology[M]. Cambridge:Cambridge University Press:79-83.
Fukao Y,Widiyantoro S,Obayashi M. 2001. Stagnant slabs in the upper and lower mantle transition region[J]. Rev Geophys,39(3):291–323. doi: 10.1029/1999RG000068
Fukao Y,Obayashi M. 2013. Subducted slabs stagnant above,penetrating through,and trapped below the 660 km discontinuity[J]. J Geophys Res Solid Earth,118(11):5920–5938. doi: 10.1002/2013JB010466
Gao Y,Suetsugu D,Fukao Y,Obayashi M,Shi Y T,Liu R F. 2010. Seismic discontinuities in the mantle transition zone and at the top of the lower mantle beneath eastern China and Korea:Influence of the stagnant Pacific slab[J]. Phys Earth Planet Inter,183(1/2):288–295.
Goes S,Agrusta R,Van Hunen J,Garel F. 2017. Subduction-transition zone interaction:A review[J]. Geosphere,13(3):644–664. doi: 10.1130/GES01476.1
Gorbatov A,Kennett B L N. 2003. Joint bulk-sound and shear tomography for Western Pacific subduction zones[J]. Earth Planet Sci Lett,210(3/4):527–543.
Gudmundsson Ó,Sambridge M. 1998. A regionalized upper mantle (RUM) seismic model[J]. J Geophys Res Solid Earth,103(B4):7121–7136. doi: 10.1029/97JB02488
Hall R,Ali J R,Anderson C D,Baker S J. 1995. Origin and motion history of the Philippine Sea Plate[J]. Tectonophysics,251(1/2/3/4):229–250.
Huang J L,Zhao D P. 2006. High-resolution mantle tomography of China and surrounding regions[J]. J Geophys Res Solid Earth,111(B9):B09305.
Ito E,Takahashi E. 1989. Postspinel transformations in the system Mg2SiO4-Fe2SiO4 and some geophysical implications[J]. J Geophys Res Solid Earth,94(B8):10637–10646. doi: 10.1029/JB094iB08p10637
Kennett B L N,Engdahl E R. 1991. Traveltimes for global earthquake location and phase identification[J]. Geophys J Int,105(2):429–465. doi: 10.1111/j.1365-246X.1991.tb06724.x
Lei J S. 2012. Upper-mantle tomography and dynamics beneath the North China Craton[J]. J Geophys Res Solid Earth,117(B6):B06313.
Li C,Van Der Hilst R D,Engdahl E R,Burdick S. 2008. A new global model for P wave speed variations in Earth’s mantle[J]. Geochem Geophys Geosyst,9(5):Q05018.
Li G H,Bai L,Zhou Y Z,Wang X R,Cui Q H. 2017. Velocity structure of the mantle transition zone beneath the southeastern margin of the Tibetan Plateau[J]. Tectonophysics,721:349–360. doi: 10.1016/j.tecto.2017.08.009
Li G H,Bai L,Zhang H,Xu Q,Zhou Y Z,Gao Y,Wang M J S,Li Z H. 2022. Velocity anomalies around the mantle transition zone beneath the Qiangtang Terrane,Central Tibetan Plateau from triplicated P waveforms[J]. Earth Space Sci,9(2):e2021EA002060. doi: 10.1029/2021EA002060
Li J,Wang X,Wang X J,Yuen D A. 2013. P and SH velocity structure in the upper mantle beneath Northeast China:Evidence for a stagnant slab in hydrous mantle transition zone[J]. Earth Planet Sci Lett,367:71–81. doi: 10.1016/j.jpgl.2013.02.026
Li W L,Wei R Q,Cui Q H,Li G H,Zhou Y Z. 2020. P-wave velocity anomalies atop and in the mantle transition zone beneath the northern South China Sea from triplicated waveforms[J]. J Asian Earth Sci,197:104379. doi: 10.1016/j.jseaes.2020.104379
Miller M S,Kennett B L N,Toy V G. 2006. Spatial and temporal evolution of the subducting Pacific plate structure along the western Pacific margin[J]. J Geophys Res Solid Earth,111(B2):B02401.
Niu F L,Levander A,Ham S,Obayashi M. 2005. Mapping the subducting Pacific slab beneath southwest Japan with Hi-net receiver functions[J]. Earth Planet Sci Lett,239(1/2):9–17.
Obayashi M,Sugioka H,Yoshimitsu J,Fukao Y. 2006. High temperature anomalies oceanward of subducting slabs at the 410-km discontinuity[J]. Earth Planet Sci Lett,243(1/2):149–158.
Tajima F,Grand S P. 1995. Evidence of high velocity anomalies in the transition zone associated with southern Kurile subduction zone[J]. Geophys Res Lett,22(23):3139–3142. doi: 10.1029/95GL03314
Tajima F,Grand S P. 1998. Variation of transition zone high-velocity anomalies and depression of 660 km discontinuity associated with subduction zones from the southern Kuriles to Izu-Bonin and Ryukyu[J]. J Geophys Res Solid Earth,103(B7):15015–15036. doi: 10.1029/98JB00752
Tonegawa T,Hirahara K,Shibutani T. 2005. Detailed structure of the upper mantle discontinuities around the Japan subduction zone imaged by receiver function analyses[J]. Earth Planets Space,57(1):5–14. doi: 10.1186/BF03351801
Wang B S,Niu F L. 2010. A broad 660 km discontinuity beneath northeast China revealed by dense regional seismic networks in China[J]. J Geophys Res,115(B6):B06308.
Wang B S,Niu F L. 2011. Spatial variations of the 660-km discontinuity in the western Pacific subduction zones observed from CEArray triplication data[J]. Earthquake Science,24(1):77–85. doi: 10.1007/s11589-011-0771-9
Wang R J. 1999. A simple orthonormalization method for stable and efficient computation of Green's functions[J]. Bull Seismol Soc Am,89(3):733–741. doi: 10.1785/BSSA0890030733
Wang T,Chen L. 2009. Distinct velocity variations around the base of the upper mantle beneath northeast Asia[J]. Phys Earth Planet Inter,172(3/4):241–256.
Wang Y,Wen L X,Weidner D,He Y M. 2006. SH velocity and compositional models near the 660-km discontinuity beneath South America and northeast Asia[J]. J Geophys Res,111(B7):B07305.
Wang Z,Huang R Q,Huang J L,He Z H. 2008. P-wave velocity and gradient images beneath the Okinawa Trough[J]. Tectonophysics,455(1/2/3/4):1–13.
Wei W,Zhao D P,Xu J D,Wei F X,Liu G M. 2015. P and S wave tomography and anisotropy in Northwest Pacific and East Asia:Constraints on stagnant slab and intraplate volcanism[J]. J Geophys Res Solid Earth,120(3):1642–1666. doi: 10.1002/2014JB011254
Weston J,Engdahl E R,Harris J,Di Giacomo D,Storchak D A. 2018. ISC-EHB:Reconstruction of a robust earthquake data set[J]. Geophys J Int,214(1):474–484. doi: 10.1093/gji/ggy155
Yamamoto Y,Takahashi T,Ishihara Y,Kaiho Y,Arai R,Obana K,Nakanishi A,Miura S,Kodaira S,Kaneda Y. 2018. Modeling the geometry of plate boundary and seismic structure in the southern Ryukyu trench subduction zone,Japan,using amphibious seismic observations[J]. J Geophys Res Solid Earth,123(2):1793–1809. doi: 10.1002/2017JB015330
Yao Z W,Li C F,He G Y,Tao T S,Zheng X L,Zhang T,Tang X J,Zhao T L. 2020. Cenozoic sill intrusion in the central and southern East China Sea Shelf Basin[J]. Mar Petrol Geol,119:104465. doi: 10.1016/j.marpetgeo.2020.104465
Zhang R Q,Gao Z Y,Wu Q J,Xie Z X,Zhang G C. 2016. Seismic images of the mantle transition zone beneath Northeast China and the Sino-Korean Craton from P-wave receiver functions[J]. Tectonophysics,675:159–167. doi: 10.1016/j.tecto.2016.03.002
Zhao D P. 2004. Global tomographic images of mantle plumes and subducting slabs:Insight into deep Earth dynamics[J]. Phys Earth Planet Inter,146(1/2):3–34.
Zhao D P,Tian Y,Lei J S,Liu L,Zheng S H. 2009. Seismic image and origin of the Changbai intraplate volcano in East Asia:Role of big mantle wedge above the stagnant Pacific slab[J]. Phys Earth Planet Inter,173(3/4):197–206.
Zhao D P,Yanada T,Hasegawa A,Umino N,Wei W. 2012. Imaging the subducting slabs and mantle upwelling under the Japan Islands[J]. Geophys J Int,190(2):816–828. doi: 10.1111/j.1365-246X.2012.05550.x
Zhao D P,Liu X,Hua Y Y. 2018. Tottori earthquakes and Daisen volcano:Effects of fluids,slab melting and hot mantle upwelling[J]. Earth Planet Sci Lett,485:121–129. doi: 10.1016/j.jpgl.2017.12.040
Zhao D P,Wang J,Huang Z C,Liu X. 2021. Seismic structure and subduction dynamics of the western Japan arc[J]. Tectonophysics,802:228743. doi: 10.1016/j.tecto.2021.228743
计量
- 文章访问数: 89
- HTML全文浏览量: 11
- PDF下载量: 25
