Fast estimation of fault rupture length and direction based on FinDer method
-
摘要:
为了快速计算震后断层的破裂参数、适应场地特征和减少模板匹配的时间,对美国加州ShakeAlert地震预警系统中断层破裂参数实时测定方法FinDer 方法中的台站性质判别和模板匹配两个环节进行了改进和优化,并基于我国大陆的六次M4.5—8.0地震对改进后的FinDer方法进行了验证。计算结果表明:对于4.5≤M≤5.5地震,利用FinDer方法能够快速得到断层的破裂方向和长度,但由于断层破裂长度有限,所计算的断层方向准确度不高;对于M>5.5地震,在首台触发后开始使用FinDer方法计算断层的长度和方向,计算过程持续数秒至1分钟左右,断层破裂长度和方向与野外调查结果基本一致;当地震引发多条断层破裂时,该方法只能计算一个综合的断层方向和长度。
-
关键词:
- FinDer方法 /
- 大震断层破裂参数 /
- 地震预警系统 /
- 国家地震烈度速报与预警工程
Abstract:The distribution direction and rupture length of large earthquake faults will affect the prediction of the city intensity in the earthquake early warning system and the subsequent outputs in the intensity rapid reporting system, such as the intensity of cities and towns, spatial distribution maps of intensity, etc. For large earthquake catastrophes, the accurate fault location will effectively improve the comprehensive processing ability of the earthquake early warning system and the intensity rapid reporting system for major earthquakes. In 2012, Bose proposed the finite fault identification rupture detector (short for FinDer), which used image recognition and template matching techniques to calculate the rupture length and direction of large earthquakes (MW>6.0) in real time. In 2015, the FinDer method was used in the ShakeAlert system in California, USA. In 2018, Bose expanded the warning range of fault parameters and proposed the second-generation algorithm, namely FinDer 2. For earthquakes with M>2.0, the peak ground acceleration (PGA) threshold was set based on the earthquake magnitudes in FinDer 2.
When a station data records exceed the set PGA threshold, the station is determined as a near-source station. By identifying the distribution range of near-source stations, the near-field area with strong ground motion is obtained, which is then matched with a pre-made template with fault direction and length. Here, the length and direction of the fault are calculated using the template with the highest matching degree.
The FinDer method has been running online in California for nearly ten years, and hence it provides a good demonstration for the discrimination of major earthquake faults in the earthquake early warning system, and provides a foundation for the construction of the large earthquake early warning system in the National Seismic Intensity Rapid Reporting and Early Warning project. What is more, through the construction of the national project, the current monitoring station network provides a favorable data support for the implementation of the FinDer method. In order to adapt to local site conditions and improve the calculation efficiency of the direction and length of fault rupture of major earthquakes, this study optimized the two steps: station property discrimination and template matching, and the details are as follows:
1) Select earthquakes with magnitude M4.0 or above that occurred in China from January 2022 to July 2023, as well as the 1999 ML7.3 Chi-Chi and 2008 MS8.0 Wenchuan earthquakes, and recount the initial thresholds corresponding to different magnitudes.
2) Perform an interpolation calculation on the distribution range of the near-source stations to determine the boundary of the surface rupture area of the fault: ① calculate the PGA value of each interpolation point every second; ② compare PGA of the interpolation points with the initial triggering threshold and determine the classification of the interpolation points; ③ determine the edge of rupture projection on the surface through the envelope of the near-source stations and the near-source interpolation points.
3) Select the minimum enclosing rectangle of the fault surface rupture edge as the optimal template and select the length and direction of the long side of the rectangle as the length and direction of the surface projection of the fault rupture so as to quickly obtain the length and direction of the fault rupture.
Taking the Chi-Chi ML7.3 earthquake in Taiwan region in 1999 as an example, the real-time calculation process by FinDer method is introduced in detail. Then, the improved FinDer method is verified by six earthquakes of M4.5−8.0 in Chinese mainland. The verification results show that:
1) By selecting an appropriate PGA threshold, the calculated length of the fault will be around a reasonable theoretical value. For earthquakes with larger magnitude, especially those with M>7.0, the calculation results are consistent with the field investigation results. For earthquakes with 4.5<M<5.5, the length and direction of rupture is calculated in an extremely short time, with a relatively short rupture length. Although the FinDer method can calculate direction, its accuracy is not high.
2) The calculation of fault direction and length is related to the distribution of the station network. When there are near-source stations around the fault, there are better constraints on the calculation of fault length. When the near-source stations around the fault are densely distributed, the accuracy of fault direction will be improved.
3) Based on the existing network conditions, the fault rupture calculations are generally completed within one minute after the first station is triggered, meeting the timeliness requirements of the earthquake early warning system. However, when a single earthquake causes multiple faults to rupture simultaneously, the FinDer method can only calculate the comprehensive rupture length and direction of multiple faults, but cannot calculate the rupture length and direction of multiple faults.
-
引言
国家地震烈度速报与预警工程项目(下文简称“国家工程”)自2023年9月起,各省地震局陆续通过验收,但实时计算大震断层破裂参数的功能尚未完全实现。大震断层的分布方向和破裂长度会影响地震预警系统城市预测烈度及后续烈度速报系统产出的结果,如城市和乡镇烈度、烈度空间分布图等(金星,2021)。对于大震巨灾,准确的断层位置将有效地提高地震预警和烈度速报对大震的综合处理能力,大震预警模块亟需稳定可靠的断层破裂长度及方向的算法。
目前,已有很多适用于地震预警的大震断层破裂参数反演方法,依据观测数据来源这些方法主要分为两大类:一类是基于传统地震观测数据进行断层参数实时计算,另一类是基于近场全球导航卫星系统(Global Navigation Satellite System,缩写为GNSS)数据得到断层破裂参数。近年来高频GNSS数据已经应用于地震预警领域(Allen,Ziv,2011;Colombelli et al,2013;Shan et al,2021;高志钰,2022)。GNSS数据能够得到地表的永久位移,拥有其它监测数据难以替代的优势,可以有效地计算地震能量的释放过程及断层的主破裂方向(Geng et al,2013;郑佳伟,2022),但高频GNSS测震台站相对稀疏,近断层处触发的台站数量有限,目前在反演速度和精度上都难以超越基于传统地震观测数据的反演方法。基于传统地震监测数据反演大震断层破裂参数的方法,包括:将大震断层分解,通过子断层的加速度外包络合成大震断层的破裂参数(Cua,2005;Yamada,Heaton,2008);利用贝叶斯理论,通过观测值与预测值的比较快速得到断层破裂参数(Ben-Menahem,1961;Boatwright,2007;Convertito et al,2012);基于线性判别理论,通过判别函数对台站性质进行判定,加上近断层处的台站分布,快速得到断层破裂区域的范围(Yamada et al,2007;于伟恒,2016)。
上述大震断层快速反演方法大多停留在理论研究阶段,正式实时在线运行的是Böse等(2012)提出的有限断层识别算法(finite fault identification rupture detector,缩写为FinDer),利用图像识别及模板匹配技术实时计算大震(MW>6.0)的断层破裂长度和方向。2015年,FinDer方法在美国加州ShakeAlert系统中运行,之后Böse等(2018)扩展了断层参数的预警范围,开发了第二代FinDer方法,即:对于M>2.0地震,基于不同震级的地震设置峰值加速度(peak ground acceleration,缩写为PGA)阈值,通过超过PGA阈值台站的分布区域与模板进行匹配,求取最佳断层参数。
在密集台网条件下,FinDer方法能够较为准确地计算正在发生地震的断层破裂长度和方向(卢建旗,李山有,2021)。2023年底我国国家工程台站建设完成后,现有的台网条件为FinDer方法的实现提供了有利的数据基础;另一方面,由于在线运行的生产系统随时可能遇到波形异常等未知问题,对算法的要求更为严格,而FinDer方法已经在线稳定运行多年,有很好的在线运行基础,为地震预警系统中大震断层的判别提供了良好的示范,因此可为我国国家工程中大震预警模块的建设提供参考。
鉴于此,本文拟对FinDer方法中PGA参数进行重新拟合,使其更加符合本地的场地条件,对模板匹配环节进行改进,减少模板匹配的时间,为大震预警后续处理过程提供断层参数,提高预警系统对大震参数的计算准确度,以期为国家工程中预警系统的相关算法提供研发基础。
1. FinDer方法
断层附近地震动的空间分布呈一定规律性,即:断层附近的台站记录PGA明显较高,且随着断层距的增大,PGA迅速衰减。利用PGA的这一特点,可判断该台站距离断层的远近。FinDer方法即利用地震波的这一特性,设置台站记录的PGA阈值,当台站记录的PGA超过这一阈值后,便判定该台站为靠近断层的台站即近源台站,否则判定为远源台站。
利用插值计算对台站分布空间进行均匀化处理,插值点的PGA记为f (x,y),该值根据周围台站的PGA线性插值获得。同台站性质判别相同,当插值点的PGA超过设定阈值,判断该网格点为近源插值点,否则为远源插值点。根据近源台站和近源插值点的分布可得到断层破裂在地表的分布范围,生成实际近场分布图。
FinDer方法的核心是模板匹配,模板记为g (x,y/L,θ),模板根据断层长度及展布方向设定,自带断层长度L和方向θ的属性。Böse等 (2012)提出的FinDer方法中,断层长度设置为5—350 km,间隔5 km递增,断层走向为0°—180°,间隔1°递增,共计制作断层模板180×$ [ $(350-5)/5+1$ ] $=12 600 个,将生成模板与实际近场分布图像进行匹配,求取二者相差最小的目标参数,该参数表达如下:
$$\ \sigma_ {{\mathrm{SSE}}}= \sum_x \sum_y\left[f ( x\text{,} y ) -g\left( x\text{,} \frac{{y}}{{L}} \text{,} \theta \right) \right] \text{,} $$ (1) 最小的目标参数即为所求模板,模板长轴的长度为断层的长度,长轴方向与正北方向的夹角即为断层的方向。
2. 算法优化与改进
FinDer方法中,台站性质的判别与模板匹配两个环节直接影响计算结果的准确性和时效性。为适应本地场地条件,提高大震断层破裂方向和长度的计算效率,对台站性质判别和模板匹配两个步骤进行优化。
2.1 依据震级范围设置不同的PGA阈值
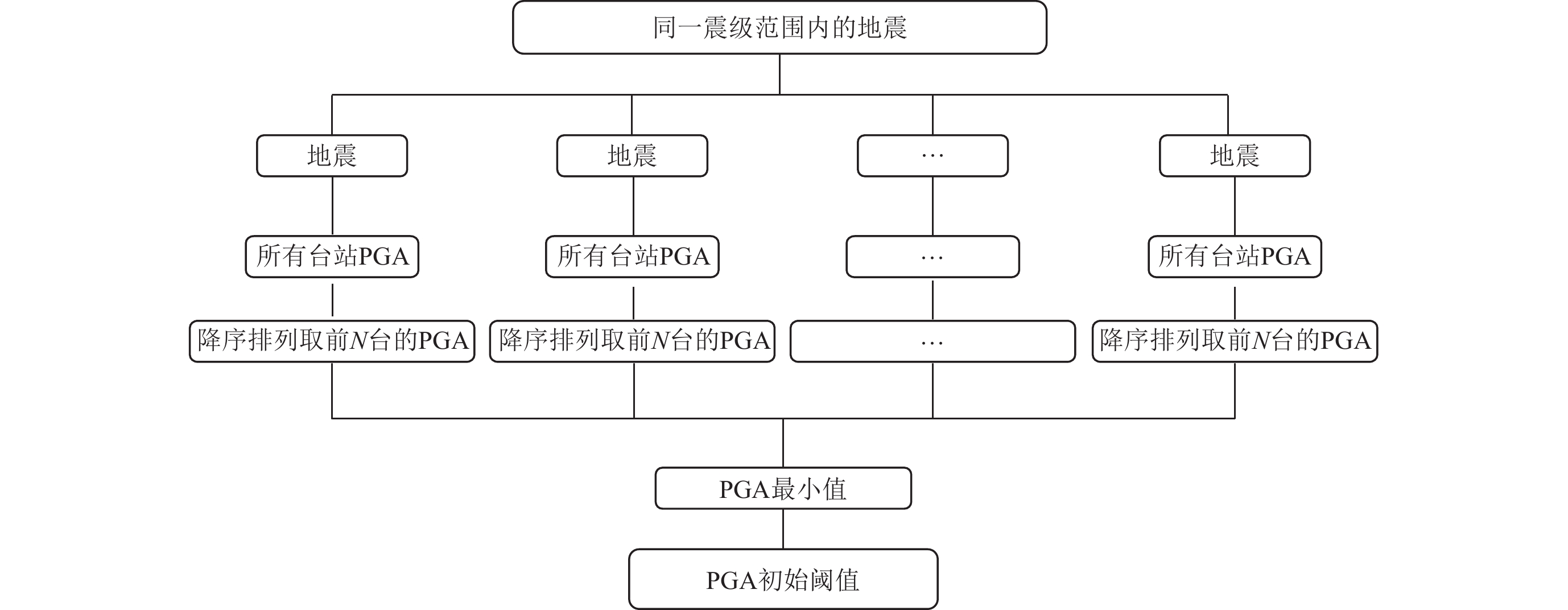
Böse等 (2018)提出九组震级对应的台站性质判别的PGA阈值,但这九组阈值是基于美国加州台网条件下的统计所得,并不完全适用于我国现有的高密度台网。强震台站的台基噪声不同,不同地区的台站分布情况也存在差异,对于相同震级范围内的地震,相同震中距的台站记录到的PGA值并不相同,故需选取合适的PGA阈值,以提高台站判别的准确性。本文选取2022年1月—2023年7月我国发生的M4.0以上地震及1999 年9 月21 日台湾集集ML7.3 地震、2008 年5 月12 日四川汶川MS8.0 地震进行统计。PGA初始阈值的确定流程如图1所示。对于相同震级范围内的地震,选取每个地震PGA最大的前N台(N根据震级和台间距进行设定),其中最小的PGA作为该震级范围内的PGA初始阈值。最终得到不同震级地震对应的PGA初始阈值结果,如表1所示。
表 1 我国不同震级台站性质判别的PGA初始阈值Table 1. Initial threshold of PGA for station property discrimination corresponding to different magnitudesM PGA阈值
/(cm⋅s−2)M PGA阈值
/(cm⋅s−2)4.0—4.5 44 6.0—6.5 173 4.5—5.0 100 6.5—7.0 180 5.0—5.5 160 7.0—7.5 195 5.5—6.0 168 7.5—8.0 250 分别对三分向加速度记录进行基线校正和带通滤波,计算三分向波形数据每秒钟的累计PGA,取三分向PGA的最大值与PGA初始阈值比较,超过阈值则判定该台站为近源台站。一般情况下,近源台站较少。为确定断层地表破裂区域的边界,需要对台站分布范围进行插值计算,插值范围为台站分布范围,插值网格为5 km×5 km。利用插值点周围四个台站的PGA线性插值计算各插值点的PGA。不同插值方法会造成插值点的地震动参数偏差,但影响该方法准确性的主要因素是近源台站性质判别是否准确。插值偏差影响较小,线性插值能够简化计算流程,每秒钟每个台站的计算量更小,节省计算时间,更加适用于地震预警系统对时效性的需求。计算各台站及插值点每秒的PGA,并与初始触发阈值进行对比,以判定插值点是否属于近源台站。
2.2 模板匹配算法优化
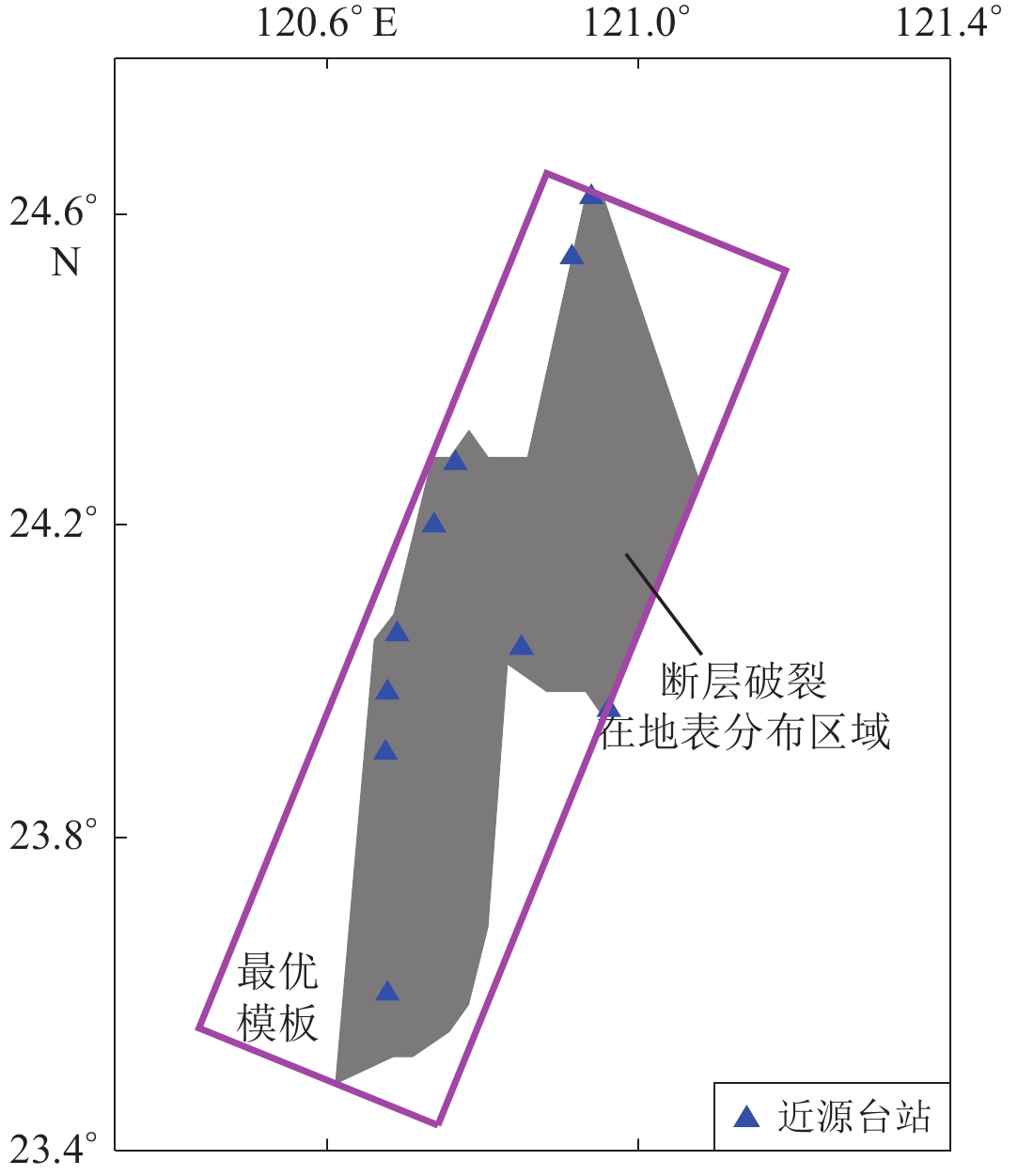
得到近源台站和近源插值点后,可利用二者分布的外包络线生成断层破裂在地表的分布区域,如图2灰色分布区域所示。
除了多次匹配需要花费较多时间外,模板匹配过程中也会遇到计算所得断层破裂区域与断层模型不收敛的情况。为了快速得到断层破裂的长度和方向,选取断层地表破裂边缘的最小外接矩形作为最优模板,选取矩形长轴的长度和方向作为断层破裂地表投影的长度和方向。最优模板选取如图2矩形框所示。
3. 震例计算及结果分析
为了验证改进后的FinDer方法对不同震级地震的计算效果,选取1999年9月21日台湾集集ML7.3地震、2008年5月12日四川汶川MS8.0地震、2022年9月5日四川泸定M6.8地震、2023年3月8日广东河源M4.5地震、2023年12月18日甘肃积石山M6.2地震以及2024年11月25日四川康定M5.8地震这六次处于不同震级范围内的地震作为计算震例,模拟实时计算断层参数。
3.1 集集地震断层破裂过程计算
利用表1中不同震级范围对应的PGA初始阈值对台站记录进行判定,并根据近源台站的外包络矩形计算断层的长度和方向。限于篇幅,以1999年9月21日台湾地区集集ML7.3地震为例,详细介绍FinDer方法的计算过程。
集集地震的断层长度和方向在首台触发后启动计算。集集地震首台触发后1 s,断层破裂长度为8 km,走向为1°,随着时间推移,断层的长度和走向不断变化,直至震后18 s,断层方向趋于稳定,最终计算于首台触发后38 s完成,此时计算得到的断层长度为132 km,走向为18°,详细计算过程展示于图3中。
![]() 图 3 1999年9月21日集集地震断层破裂过程的反演(a) 首台触发后1 s;(b) 首台触发后5 s;(c) 首台触发后10 s;(d) 首台触发后15 s;(e) 首台触发后20 s;(f) 首台触发后25 s;(g) 首台触发后30 s;(h) 首台触发后35 s;(i) 首台触发后38 sFigure 3. Inversion for fault rupture process of Chi-Chi earthquake on September 21,1999(a) 1 second after the first station is triggered;(b) 5 seconds after the first station is triggered;(c) 10 seconds after the first station is triggered;(d) 15 seconds after the first station is triggered;(e) 20 seconds after the first station is triggered;(f) 25 seconds after the first station is triggered;(g) 30 seconds after the first station is triggered;(h) 35 seconds after the first station is triggered;(i) 38 seconds after the first station is triggered
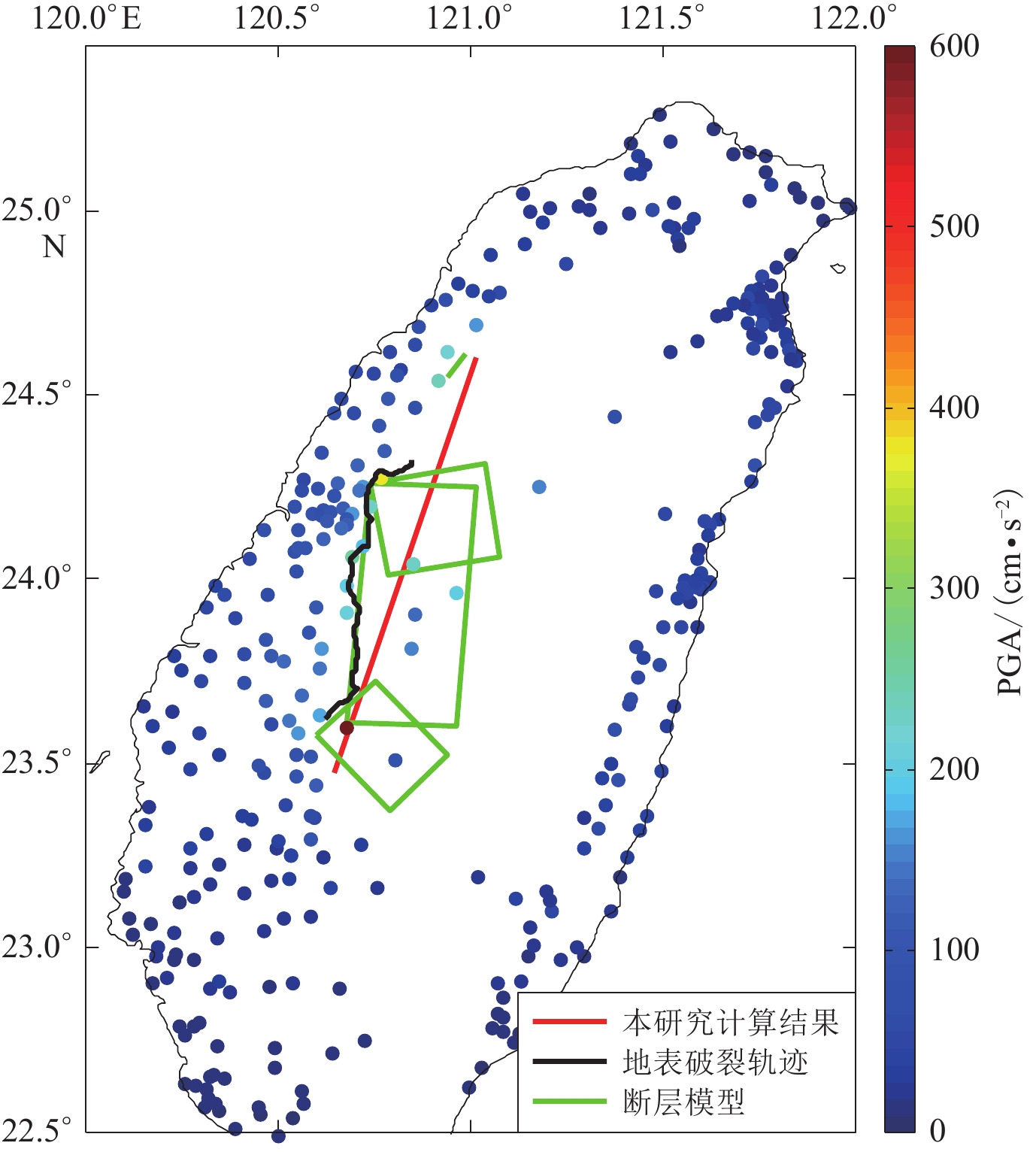
图 3 1999年9月21日集集地震断层破裂过程的反演(a) 首台触发后1 s;(b) 首台触发后5 s;(c) 首台触发后10 s;(d) 首台触发后15 s;(e) 首台触发后20 s;(f) 首台触发后25 s;(g) 首台触发后30 s;(h) 首台触发后35 s;(i) 首台触发后38 sFigure 3. Inversion for fault rupture process of Chi-Chi earthquake on September 21,1999(a) 1 second after the first station is triggered;(b) 5 seconds after the first station is triggered;(c) 10 seconds after the first station is triggered;(d) 15 seconds after the first station is triggered;(e) 20 seconds after the first station is triggered;(f) 25 seconds after the first station is triggered;(g) 30 seconds after the first station is triggered;(h) 35 seconds after the first station is triggered;(i) 38 seconds after the first station is triggered野外调查结果显示,集集地震沿着已有的车笼埔断裂带造成多条断层破裂,南北向主断层的地表破裂长度约为80 km,走向为3°,破裂走向在南北两侧均发生变化,总断裂长度为140 km,走向介于0°—26°之间(卢建旗,李山有,2021)。Ji等(2001)利用速度计和GPS近震源的观测数据联合反演得到集集地震的三个平面断层几何图形。本研究计算结果与野外调查的主断层方向之间有15°的偏差,但与总断裂走向和破裂长度基本一致。与Ji等(2001)的断层几何图形相比,断层长度基本一致,如图4所示。本文方法只能得到一个综合的断层走向和长度,无法计算多个断层的长度和走向。
3.2 其它震例的断层破裂过程计算
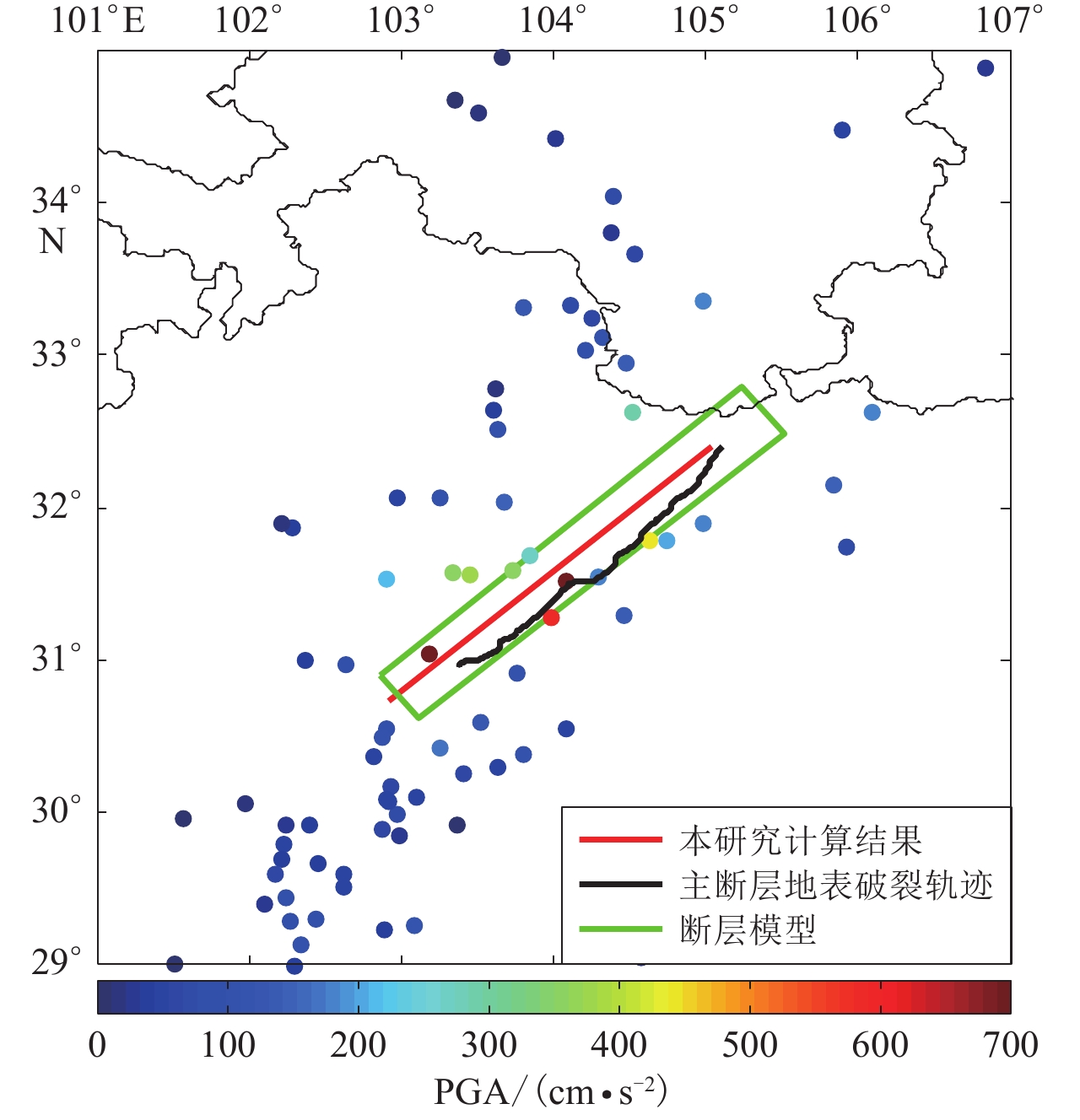
汶川地震首台触发后54 s计算结果趋向稳定,最终计算结果显示断层长度为300 km,断层走向为N51°E。野外地质调查结果表明,此次地震位于龙门山推覆构造带上,主断层北川—映秀断层的地表破裂长约240 km,走向为(42°± 5°)(徐锡伟等,2008)。汶川地震发生后,许多学者对其发震构造进行了研究(Ji et al,2001;徐锡伟等,2008;Zhang et al,2009;于伟恒,2016),其中Zhang等(2009)得到了断层破裂在地表投影的矩形模型。图5给出了本文方法与野外调查主断层及Zhang等(2009)的断层结果的对比,可见:本文方法得到的断层长度稍长于野外调查主断层长度,较Zhang等(2009)得到的断层模型稍短;断层走向与两者基本一致。汶川地震断层展布方向的东北端,台站分布相对稀疏,近源台站数量较少,对断层东北段的约束较弱,导致最终计算结果与野外调查结果及Zhang等(2009)的断层模型稍有偏差。
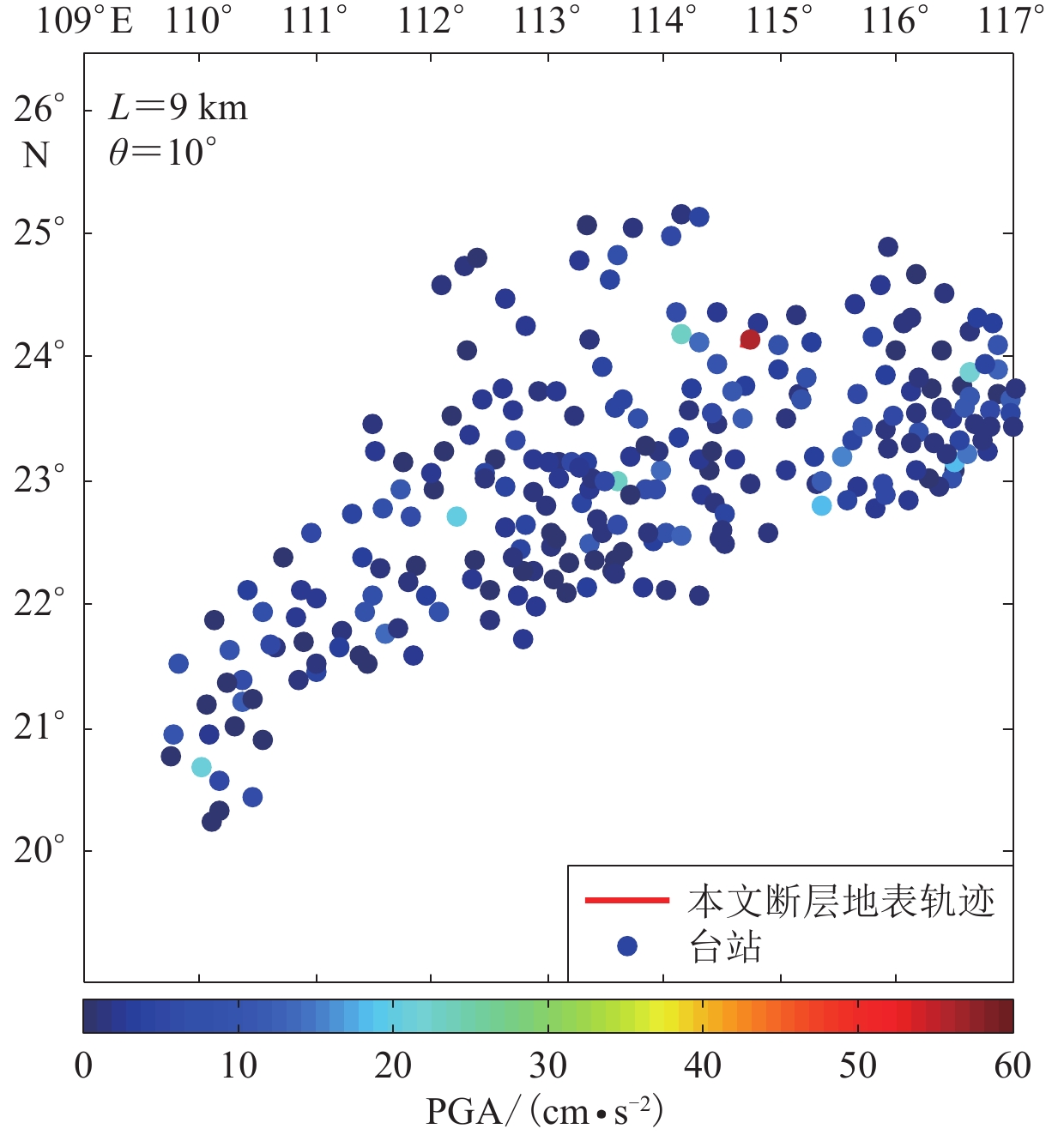
对于震级较大的地震,由于断层破裂尺度较大,计算结果一般比较好(Böse et al,2012;卢建旗,李山有,2021)。此外,Böse等(2018)提出FinDer二代可以计算M>2.0地震的断层破裂方向和长度,但小震断层破裂几乎在瞬间完成,计算的破裂方向不明显,计算结果的准确性尚待验证。利用该方法对2023年3月8日广东河源M4.5地震进行计算,此次地震中PGA大于20 cm/s2的台站分布分散,无明显规律,计算结果如图6所示。
广东河源地震计算得到的断层长度为9 km,断层走向为10°,与国家地震科学数据中心公布此次地震的震源机制解(杨志高等,2023)的两个断层面走向(330°和62°)相差较大。由于最终达到阈值的近源台站只有一个,计算结果并不可靠,因此当近源台站及近源插值点的数量低于2时,对断层方向的约束不足,计算得到的断层方向有很大的不确定性,判定结果不可靠。
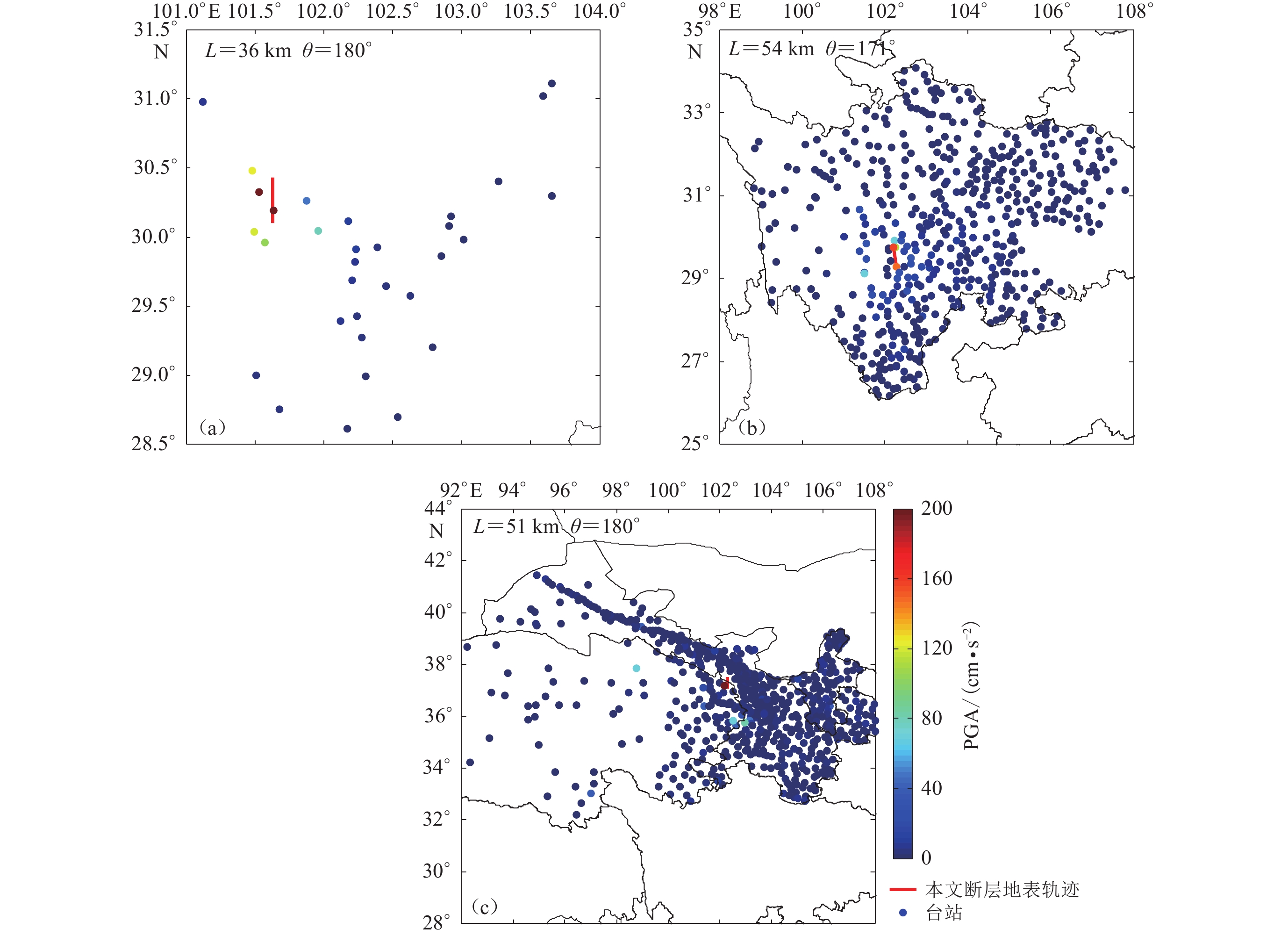
为了进一步验证改进后FinDer方法的适用性,选取2014年11月25日四川康定M5.8地震、2022年9月5日四川甘孜州泸定M6.8地震和2023年12月18日甘肃临夏州积石山M6.2地震进行计算。康定地震在首台触发后开始进行计算,此时断层还未发育完全,断层破裂长度为10 km,断层走向为90°,断层方向并不稳定,在首台触发后3 s计算得到稳定的断层长度为36 km和走向为180°。四川泸定地震首台触发后7 s计算结果稳定,最终计算的断层长度为54 km,走向为171°和351° (断层两个走向之间相差180°)。甘肃积石山地震在震后5 s得到稳定的断层长度为51 km,走向为180°。上述结果如图7所示。
根据国家地震科学数据中心公布的四川康定M5.8、四川泸定M6.8、甘肃积石山M6.2这三次地震的震源机制解结果(杨志高等,2023):四川康定地震为走滑型地震,断层走向为152°。本文最终计算的断层走向结果180°与其相差28°;甘肃积石山地震为逆冲型地震,断层走向为170°,本文最终计算结果180°与其相差10°;四川泸定地震为走滑型地震,断层走向为343°,本文最终计算结果171° (351°)与其相差8°。经专家现场考察及泸定地震余震序列精定位,确定泸定地震位于鲜水河断裂带磨西段,整体沿NNW向展布,该段处于鲜水河断裂与龙门山断裂的交会处(申星等,2024),利用FinDer方法计算得到的断层走向位于鲜水河断裂带与龙门山断裂带之间(图7),与余震序列走向基本一致。
三次地震最终计算的断层走向的准确度与震源机制类型并无明显关系(表2)。若断层四周有近源台站的分布,则对整个断层的长度约束更好,若四周近源台站分布密集,则断层走向的计算结果就会更加准确。
表 2 利用改进的FinDer方法计算结果与断层机制解结果对比Table 2. The calculation results using improved FinDer method compared with large earthquake mechanism CMT products震例 M 平均台间距/km 计算断层走向/° 断层走向/° 走向偏差/° 震源机制 四川康定地震 5.8 57.1 180 152 28 走滑断层地震 甘肃积石山地震 6.2 50.7 180 170 10 逆断层地震 四川泸定地震 6.8 43.9 171 343 8 走滑断层地震 注:断层走向来自杨志高等(2 023)。 4. 讨论与结论
实时在线运行的地震预警系统在计算过程中并未提前假定地震的位置及震级的大小,因而初始阈值无法确定。在模拟预警计算的过程中,依据预警震级设置PGA初始阈值,当近源台站和近源插值点较多时,提高阈值,减少近源台站和近源插值点;当近源台站和近源插值点较少时降低阈值,增加近源台站和近源插值点,循环计算直到显示的近源插值点和台站数合理。
对于震级较小的地震(M≤5.5),如广东河源地震,断层破裂引起的地震动并不强烈,且方向性不明显,靠近断层处的地震波幅值未明显偏大,近源台站和远源台站记录到的PGA并无明显偏差,这可能会导致近源台站的性质判定存在误差,尤其当判定出的近源台站数量较小时(一般当近源台站及近源插值点总数少于2时),断层方向的计算结果可靠性较低,不能作为最终判别结果。
断层方向和长度计算稳定前,受台站分布及近源台站判别时效的影响,断层方向可能会发生较大的变化,如集集地震计算中显示,在首台触发后1—15 s,断层方向并不稳定。此次地震造成多条断层破裂,利用FinDer方法只能得到一个综合的断层展布方向和长度。六个震例的计算结果如表3所示,选用合适的PGA阈值,计算所得的断层长度会在合理的理论值附近,震级越大的地震,尤其是M>7.0地震,计算结果与野外调查结果基本一致。实时计算方法不仅对判别准确性有较高要求,得到稳定结果的时效性也需要满足发布要求。从表3中可以看出,对于M<8.0地震,能够在首台触发后的秒级时间范围内得到断层破裂方向和长度,可满足实时运行的系统对时效性的要求,也为后续城市和乡镇烈度及烈度空间分布的计算奠定较好的基础。
表 3 利用本文方法计算的六个震例的断层参数结果Table 3. Fault parameters of six earthquake examples by using improved FinDer method in this study震例 M 理论断层长度/km 计算断层长度*/km 计算断层走向/° 断层参数稳定时间(首台触发后)/s 广东河源地震 4.5 1.6 9 10 0 四川康定地震 5.8 10.6 36 180 3 甘肃积石山地震 6.2 22.1 51 180 5 四川泸定地震 6.8 44 54 171 7 台湾集集地震 7.3 90.4 132 18 38 四川汶川地震 8.0 245 300 51 54 *断层长度由lgL=0.62M-2.5计算。 FinDer方法作为实时在线运行的大震破裂计算方法,为大震预警模块中断层破裂参数的计算提供了思路。通过对其进行改进和优化,利用我国六次中强地震对其进行了验证,结果表明:
1) 对于M5.5以下地震,断层破裂在极短的时间内完成,破裂长度较短,FinDer方法虽能计算方向,但准确性不高;
2) 在现有的台网条件下,一般在首台触发后一分钟内完成计算,满足地震预警系统对时效性的需求;
3) 一次地震引发多条断层同时破裂,FinDer方法只能计算多条断层的综合破裂长度和方向,不能计算多条断层各自的破裂长度和方向。
不同地区场地条件不同,全国采用统一的PGA阈值对结果的准确性有较大影响。国家工程建设完成后,我国M5.5以上地震的观测数据积累较少,随着高密度台网观测资料的积累,在今后的工作中,可分别统计各地区PGA初始阈值,以便得到更加准确合理的结果。
-
图 3 1999年9月21日集集地震断层破裂过程的反演
(a) 首台触发后1 s;(b) 首台触发后5 s;(c) 首台触发后10 s;(d) 首台触发后15 s;(e) 首台触发后20 s;(f) 首台触发后25 s;(g) 首台触发后30 s;(h) 首台触发后35 s;(i) 首台触发后38 s
Figure 3. Inversion for fault rupture process of Chi-Chi earthquake on September 21,1999
(a) 1 second after the first station is triggered;(b) 5 seconds after the first station is triggered;(c) 10 seconds after the first station is triggered;(d) 15 seconds after the first station is triggered;(e) 20 seconds after the first station is triggered;(f) 25 seconds after the first station is triggered;(g) 30 seconds after the first station is triggered;(h) 35 seconds after the first station is triggered;(i) 38 seconds after the first station is triggered
表 1 我国不同震级台站性质判别的PGA初始阈值
Table 1 Initial threshold of PGA for station property discrimination corresponding to different magnitudes
M PGA阈值
/(cm⋅s−2)M PGA阈值
/(cm⋅s−2)4.0—4.5 44 6.0—6.5 173 4.5—5.0 100 6.5—7.0 180 5.0—5.5 160 7.0—7.5 195 5.5—6.0 168 7.5—8.0 250 表 2 利用改进的FinDer方法计算结果与断层机制解结果对比
Table 2 The calculation results using improved FinDer method compared with large earthquake mechanism CMT products
震例 M 平均台间距/km 计算断层走向/° 断层走向/° 走向偏差/° 震源机制 四川康定地震 5.8 57.1 180 152 28 走滑断层地震 甘肃积石山地震 6.2 50.7 180 170 10 逆断层地震 四川泸定地震 6.8 43.9 171 343 8 走滑断层地震 注:断层走向来自杨志高等(2 023)。 表 3 利用本文方法计算的六个震例的断层参数结果
Table 3 Fault parameters of six earthquake examples by using improved FinDer method in this study
震例 M 理论断层长度/km 计算断层长度*/km 计算断层走向/° 断层参数稳定时间(首台触发后)/s 广东河源地震 4.5 1.6 9 10 0 四川康定地震 5.8 10.6 36 180 3 甘肃积石山地震 6.2 22.1 51 180 5 四川泸定地震 6.8 44 54 171 7 台湾集集地震 7.3 90.4 132 18 38 四川汶川地震 8.0 245 300 51 54 *断层长度由lgL=0.62M-2.5计算。 -
高志钰. 2022. 基于高频GNSS与合成地震的强震破裂及预警研究[D]. 北京:中国地震局地质研究所:44−55. Gao Z Y. 2022. Research on Rupture and Early Warning of Strong Earthquakes Based on High-Rate GNSS and Synthetic Earthquakes[D]. Beijing:Institute of Geology,China Earthquake Administration:44−55 (in Chinese).
金星. 2021. 地震预警与烈度速报:理论与实践[M]. 北京:科学出版社:192−228. Jin X. 2021. Earthquake Early Warning and Intensity Rapid Report:Theory and Practice[M]. Beijing:Science Press:192−228 (in Chinese).
卢建旗,李山有. 2021. 地震预警断层参数实时识别方法(FinDer)详解及其性能初步评价[J]. 世界地震工程,37(1):152–164. Lu J Q,Li S Y. 2021. Detailed analysis and preliminary performance evaluation of the FinDer:A real-time finite fault rupture detector for earthquake early warning[J]. World Earthquake Engineering,37(1):152–164 (in Chinese).
申星,梁洪宝,宋成科. 2024. 泸定MS6.8地震震前变形特征及鲜水河断裂南东段地震活动性[J]. 大地测量与地球动力学,44(1):69–74. Shen X,Liang H B,Song C K. 2024. Deformation characteristics before the Luding MS6.8 earthquake and seismicity of the southeast section of the Xianshuihe fault[J]. Journal of Geodesy and Geodynamics,44(1):69–74 (in Chinese).
徐锡伟,闻学泽,叶建青,马保起,陈杰,周荣军,何宏林,田勤俭,何玉林,王志才,孙昭民,冯希杰,于贵华,陈立春,陈桂华,于慎鄂,冉勇康,李细光,李陈侠,安艳芬. 2008. 汶川 MS8.0 地震地表破裂带及其发震构造[J]. 地震地质,30(3):597–629. doi: 10.3969/j.issn.0253-4967.2008.03.003 Xu X W,Wen X Z,Ye J Q,Ma B Q,Chen J,Zhou R J,He H L,Tian Q J,He Y L,Wang Z C,Sun Z M,Feng X J,Yu G H,Chen L C,Chen G H,Yu S E,Ran Y K,Li X G,Li C X,An Y F. 2008. The MS8.0 Wenchuan earthquake surface ruptures and its seismogenic structure[J]. Seismology and Geology,30(3):597–629 (in Chinese).
杨志高,徐泰然,梁建宏,邓文泽,张建勇,徐佳静. 2023. 中国地震台网中心大震震源机制CMT产品[EB/OL]. [2023−12−20]. https://data.earthquake.cn/datashare/report.shtml?PAGEID=earthquake_dzzyjz. Yang Z G,Xu T R,Liang J H,Deng W Z,Zhang J Y,Xu J J. 2023. Large earthquake mechanism (CMT) of China Earthquake Networks Center[EB/OL]. [2023−12−20]. https://data.earthquake.cn/datashare/report.shtml?PAGEID=earthquake_dzzyjz (in Chinese).
于伟恒. 2016. 基于Fisher线性识别方法反演大震破裂尺度的研究[D]. 哈尔滨:中国地震局工程力学研究所:26−38. Yu W H. 2016. A Study of Inversion of Large Earthquake Rupture Based on Fisher Linear Identification Methods[D]. Harbin:Institute of Engineering Mechanics,China Earthquake Administration:26−38 (in Chinese).
郑佳伟. 2022. 基于高频GNSS和加速度计信息的大震破裂特征快速确定研究[D]. 武汉:武汉大学:42−54. Zheng J W. 2022. Rapid Determination of Rupture Characteristic of Large Earthquakes Based on High-Rate GNSS and Accelerometer[D]. Wuhan:Wuhan University:42−54 (in Chinese).
Allen R M,Ziv A. 2011. Application of real-time GPS to earthquake early warning[J]. Geophys Res Lett,38(16):L16310.
Ben-Menahem A. 1961. Radiation of seismic surface-waves from finite moving sources[J]. Bull Seismol Soc Am,51(3):401–435. doi: 10.1785/BSSA0510030401
Boatwright J. 2007. The persistence of directivity in small earthquakes[J]. Bull Seismol Soc Am,97(6):1850–1861. doi: 10.1785/0120050228
Böse M,Heaton T H,Hauksson E. 2012. Real-time Finite Fault Rupture Detector (FinDer) for large earthquakes[J]. Geophys J Int,191(2):803–812. doi: 10.1111/j.1365-246X.2012.05657.x
Böse M,Smith D E,Felizardo C,Meier M A,Heaton T H,Clinton J F. 2018. FinDer v.2:Improved real-time ground-motion predictions for M2−M9 with seismic finite-source characterization[J]. Geophys J Int,212(1):725–742. doi: 10.1093/gji/ggx430
Colombelli S,Allen R M,Zollo A. 2013. Application of real-time GPS to earthquake early warning in subduction and strike-slip environments[J]. J Geophys Res:Solid Earth,118(7):3448–3461. doi: 10.1002/jgrb.50242
Convertito V,Caccavale M,De Matteis R,Emolo A,Wald D,Zollo A. 2012. Fault extent estimation for near-real-time ground-shaking map computation purposes[J]. Bull Seismol Soc Am,102(2):661–679. doi: 10.1785/0120100306
Cua G B. 2005. Creating the Virtual Seismologist:Developments in Ground Motion Characterization and Seismic Early Warning[D]. Pasadena:California Institute of Technology:182−211.
Geng J H,Bock Y,Melgar D,Crowell B W,Haase J S. 2013. A new seismogeodetic approach applied to GPS and accelerometer observations of the 2012 Brawley seismic swarm:Implications for earthquake early warning[J]. Geochem Geophys Geosyst,14(7):2124–2142. doi: 10.1002/ggge.20144
Ji C,Helmberger D V,Alex Song T R,Ma K F,Wald D J. 2001. Slip distribution and tectonic implication of the 1999 Chi-Chi,Taiwan,earthquake[J]. Geophys Res Lett,28(23):4379–4382. doi: 10.1029/2001GL013225
Shan X J,Li Y C,Wang Z J,Yin H,Liu X D,Liu Y H,Zhang G H,Zhang Y F,Qu C Y. 2021. GNSS for quasi-real-time earthquake source determination in eastern Tibet:A prototype system toward early warning applications[J]. Seismol Res Lett,92(5):2988–2997. doi: 10.1785/0220190244
Yamada M,Heaton T,Beck J. 2007. Real-time estimation of fault rupture extent using near-source versus far-source classification[J]. Bull Seismol Soc Am,97(6):1890–1910. doi: 10.1785/0120060243
Yamada M,Heaton T. 2008. Real-time estimation of fault rupture extent using envelopes of acceleration[J]. Bull Seismol Soc Am,98(2):607–619. doi: 10.1785/0120060218
Zhang Y,Feng W P,Xu L S,Zhou C H,Chen Y T. 2009. Spatio-temporal rupture process of the 2008 great Wenchuan earthquake[J]. Science in China:Series D,52(2):145–154. doi: 10.1007/s11430-008-0148-7




 下载:
下载: