Model for H/V acceleration response spectral ratio for non-scenario earthquakes from the subduction interface
-
摘要:
基于日本KiK-net和K-NET台网所获取的59次俯冲带板间地震的3 048条强震记录，采用随机效应算法和数理统计方法，分析了地震类型、谱周期、场地条件等因素对水平与竖向加速度（H/V）反应谱比的影响，并建立了俯冲带板间非设定地震（不包含震源项、路径项等参数）的H/V反应谱比模型，用于调整无震源、无路径信息时的工程抗震设计反应谱。研究结果表明：① 地震类型和场地类别对H/V反应谱比均存在显著影响，H/V反应谱比的峰值周期与各类别场地的平均场地周期接近，预测模型的H/V反应谱比峰值周期与场地的卓越周期具有一定的相关性；② 路径效应项和场地效应项的随机误差对H/V反应谱比离散程度的贡献随谱周期的增加而不断增大，场地效应的贡献在各类场地的平均场地周期处最大。为便于工程应用，模型中未加入震源和路径参数，残差分析表明震源特性和传播路径仍会对H/V反应谱比产生影响，当有明确的地震信息时，引入震级、断层距、断层深度等因素可进一步提高模型预测精度。
Abstract:Vertical ground motions acceleration response spectrum is an important part of the structural seismic design, and the domestic and foreign seismic design codes use the H/V response spectrum ratio to calculate the vertical ground motion response spectrum indirectly, which is a simple and feasible method, but the current seismic codes are still too rough for the vertical ground motions response spectrum, which will underestimate the role of vertical component in the engineering application or will result in redundancy in the application. In addition, the geological structure of the subduction zone is complex and earthquakes occur frequently, and the interface earthquakes have the characteristics of high magnitude and high intensity, so it is necessary to study the interface earthquakes in the subduction zone separately.
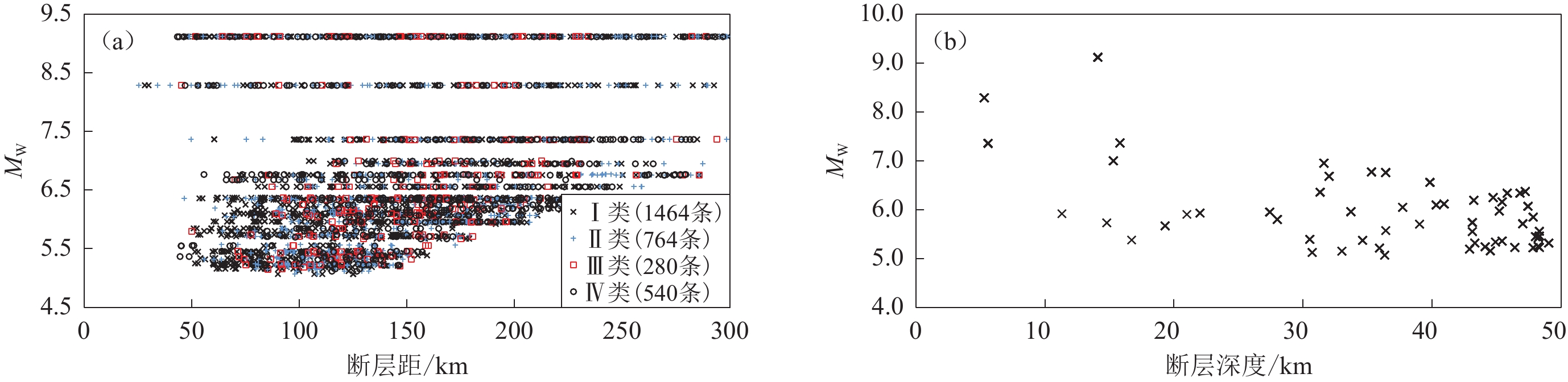
In this paper, based on the surface observation data obtained from the KiK-net and K-NET in Japan, the data are first preprocessed so as to improve the accuracy of the model and to eliminate the seismic records that affect the fitting effect, which involves filtering the magnitude and the fault distance of the data, checking the acceleration time history, then correcting the instrumental response and filtering the waveforms. Finally, 3 048 seismic records from 59 interface earthquakes in subduction zones are selected for the study, and the acceleration response spectra are calculated for each record with 5% damping ratio. Random-effects algorithms and mathematical statistics were used to analyze the effects of spectral period, earthquake type and site conditions on the H/V response spectral ratio. Based on the site period Ts division of site classes, the H/V response spectrum ratio model for non-scenario earthquakes （without source and path parameters） from the subduction interface is established, the model coefficients are smoothed and adjusted to facilitate engineering applications, and the standard deviation of model residuals is evaluated and analyzed to explore the magnitude and source of random residuals and to improve the accuracy and reliability of the model. The model can be used to adjust the response spectrum of engineering seismic design when there is no earthquake source and no path information.
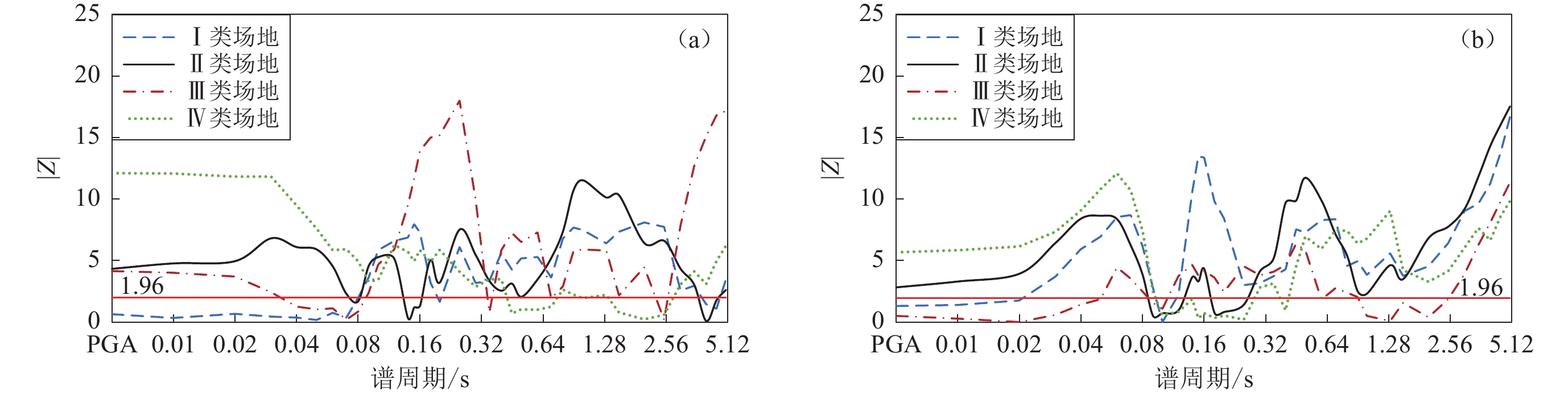
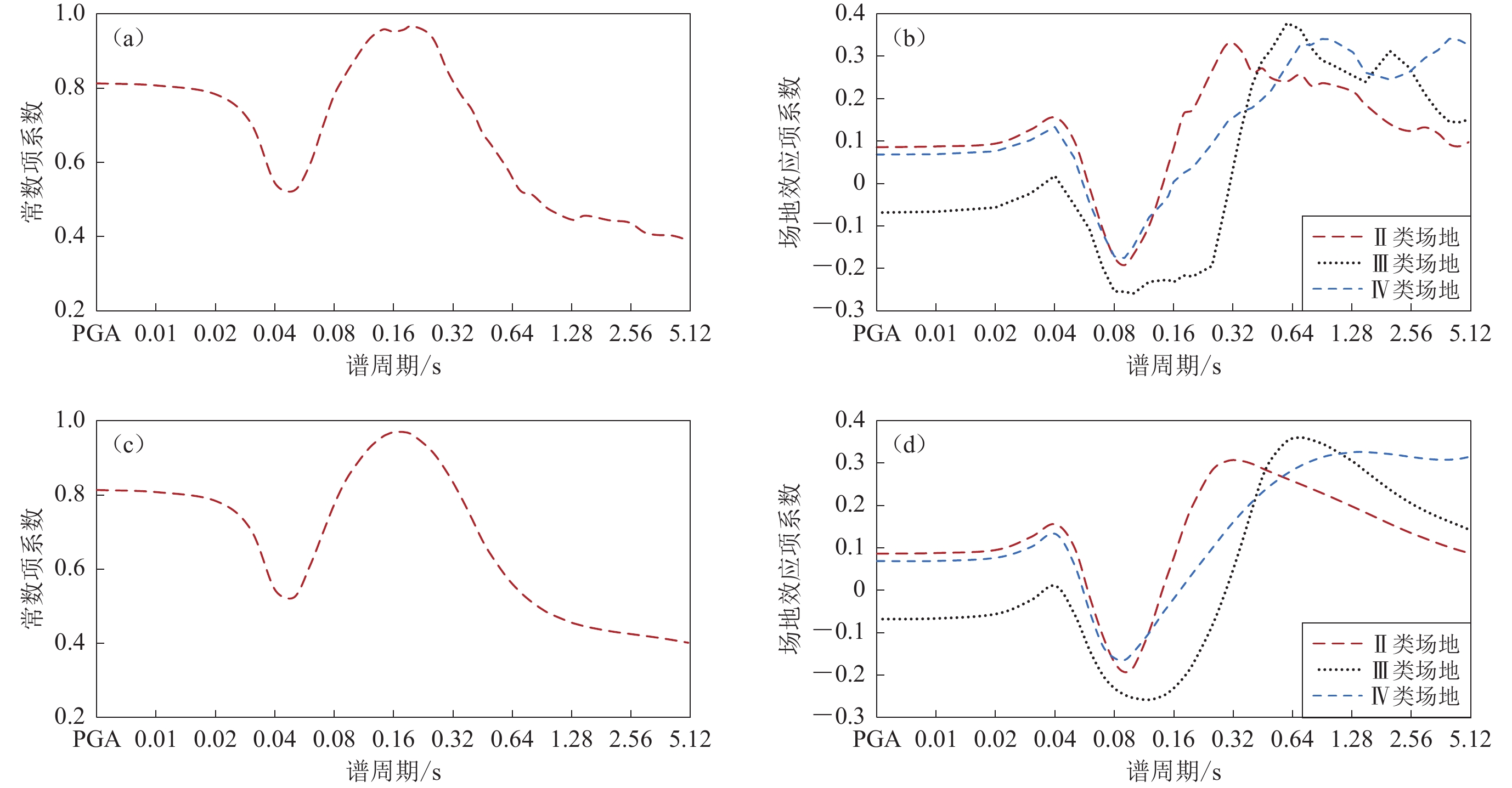
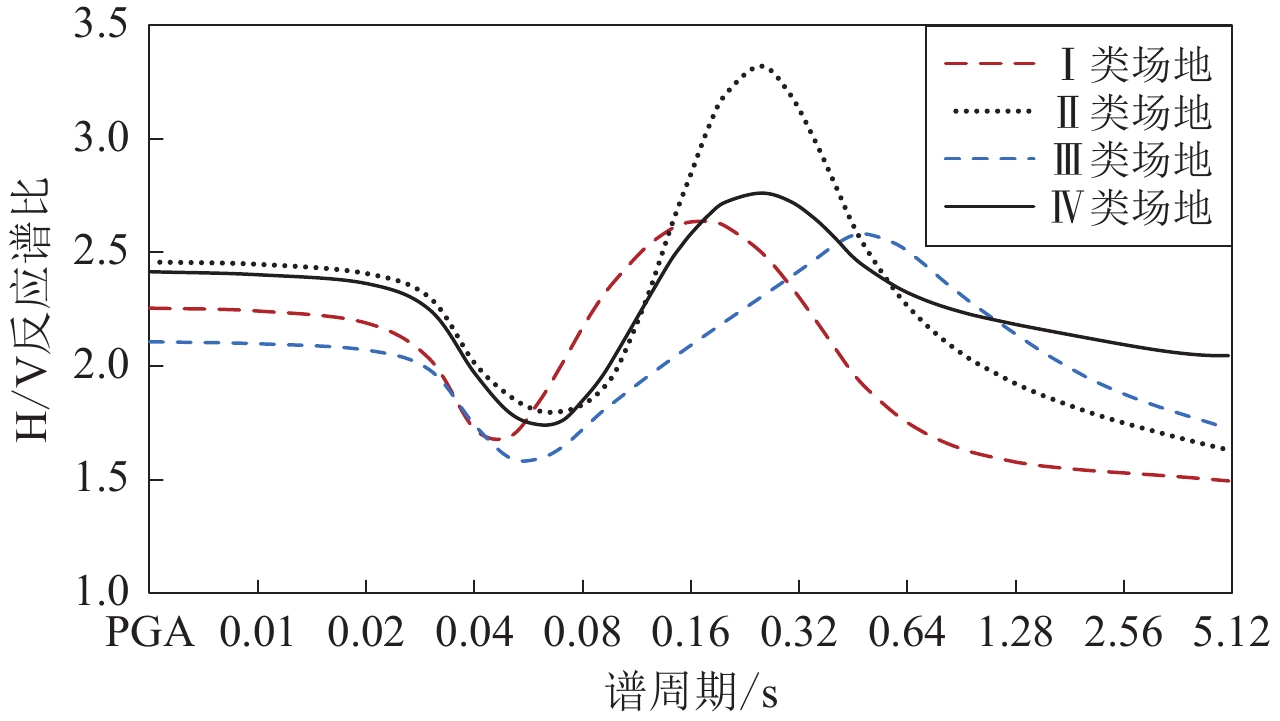
The study shows that: ① t-test method verifies that the type of earthquake and the site classes have a significant effect on the H/V response spectral ratio, so it is necessary to establish the corresponding response spectral ratio models according to different types of earthquakes and site classes; ② The peak period of the site effect term coefficients is close to the average period for each type of site, indicating that the site term coefficients are reasonably well taken to elicit a resonant site response. In the prediction model, the peak period of the H/V response spectral ratio is 0.2 s for classⅠsites, 0.27 s for class Ⅱ sites, and 0.5 s for class Ⅲ sites. The peak period of the H/V response spectral ratio in the prediction model has a certain correlation with the site predominant period, which can prove that the H/V response spectral ratio model is well fitted by using the random-effects method; ③ The standard deviation analysis shows that the dispersion generated by the source effect is smaller than that generated by the path effect and the site effect, the random residuals of the path effect term and the site effect term contribute more to the model variability, indicating that the random residuals of the horizontal and vertical components of the seismic records have strong correlation. Different site classes produce the largest dispersion in response spectral ratios at their corresponding mean site period. The increasing dispersion of the H/V response spectral ratio due to path effects with increasing spectral period demonstrates that the correlation between the horizontal and vertical component random path residuals decreases with increasing period.
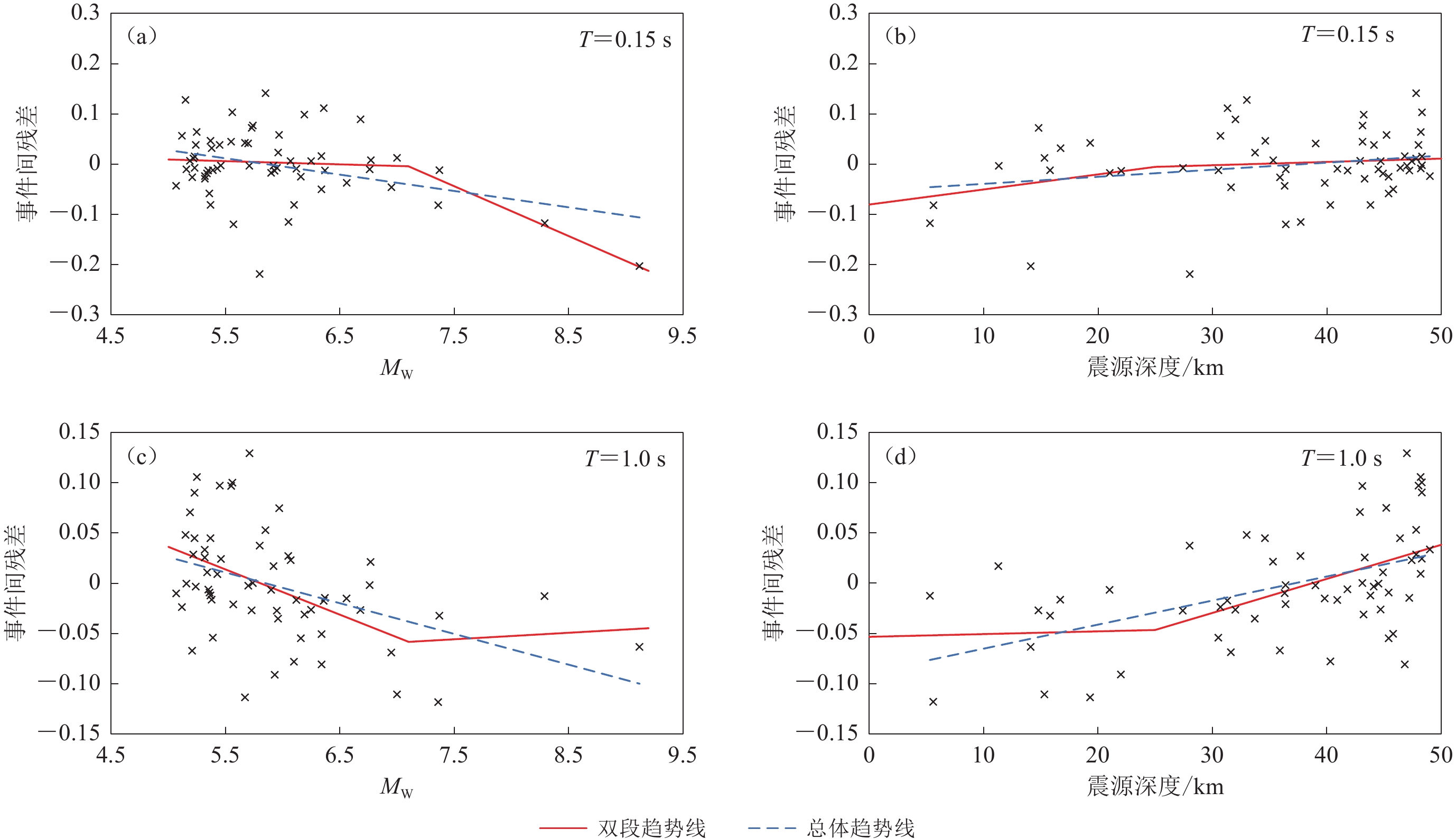
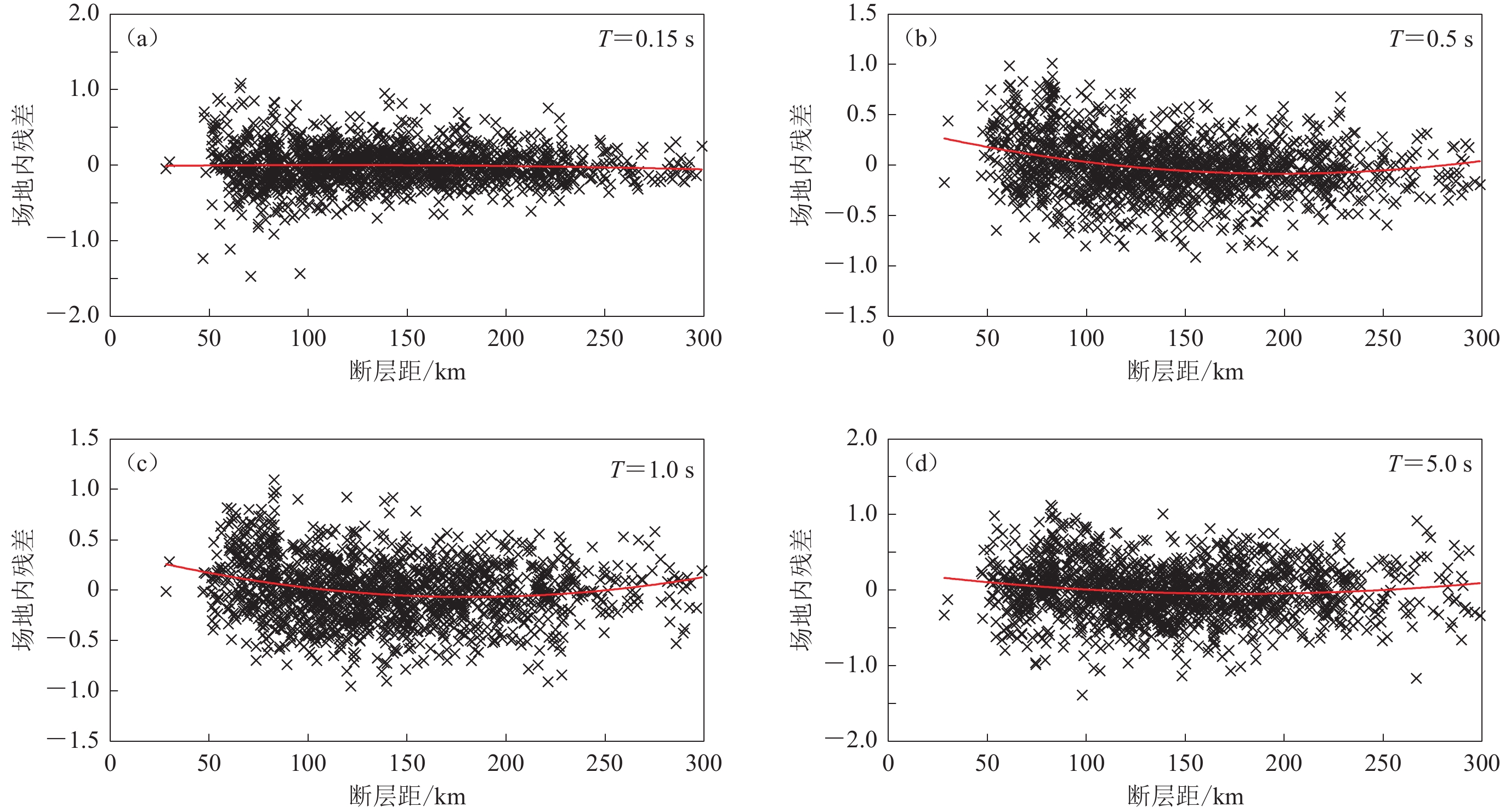
The H/V response spectral ratio model for non-scenario earthquakes established in this study has the characteristics of simplicity and convenience for engineering application, and the source and path parameters are not added into the model. Residual analysis shows that the between-event residuals are correlated with the magnitude and fault depth, and the effects of magnitude and fault depth on the between-event residuals are clearly segmented at magnitude 7.1 and fault depth of 25 km. There is a correlation between the within-site residuals and the fault distance. Moreover, the effects of magnitude, source depth and fault distance on the residuals change with the spectral period, the seismic source characteristics and propagation paths still affect the H/V response spectral ratio. Therefore, when there is clear seismic information, the introduction of magnitude, fault distance, fault depth and other factors can further improve the model prediction accuracy, and will be committed to constructing a more accurate H/V response spectral ratio model in the future research. The results of this paper are of reference value for the seismic fortification of offshore engineering.
-
-
![]()
图 3 四种场地类别之间的统计值|Z|随谱周期的变化
（a） Ⅱ类与Ⅰ类之比；（b） Ⅲ类与Ⅰ类之比；（c） Ⅳ类与Ⅰ类之比；（d） Ⅲ类与Ⅱ类之比；（e） Ⅳ类与Ⅱ类之比；（f） Ⅳ类与Ⅲ类之比
Figure 3. The variations of |Z| values versus spectral periods for each pair of four site classes
（a） The ratio of class Ⅱ to class Ⅰ ；（b） The ratio of class Ⅲ to class Ⅰ ；（c） The ratio of class Ⅳ to class Ⅰ ；（d） The ratio of class Ⅲ to class Ⅱ ；（e） The ratio of class Ⅳ to class Ⅱ ；（f） The ratio of class Ⅳ to class Ⅲ
![]()
图 1 地震数据分布图
（a） 矩震级MW与断层距；（b） 矩震级MW与断层深度
Figure 1. The distribution of earthquake records
（a） Magnitude MW and fault distance；（b） Magnitude MW and fault depth
![]()
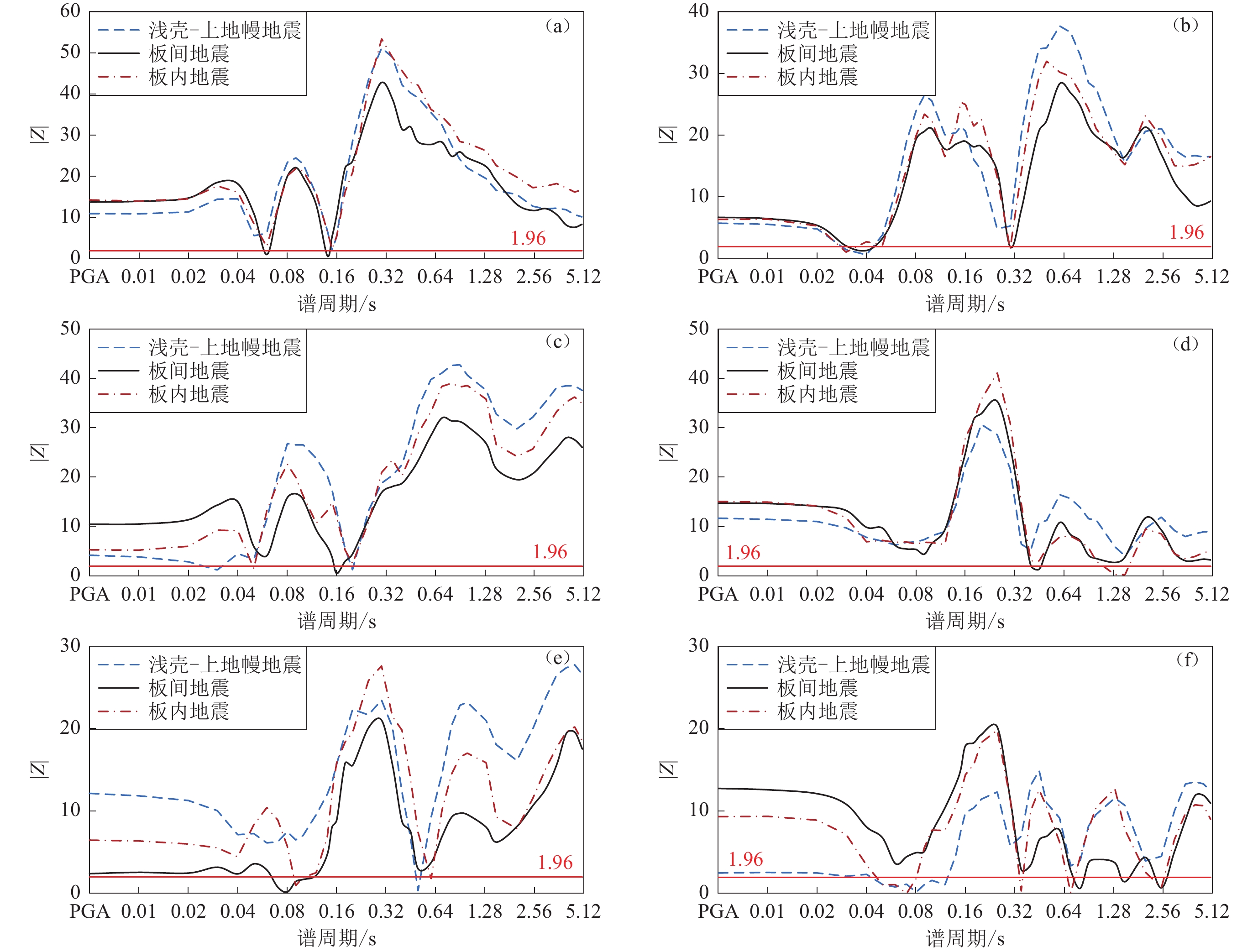
图 2 不同类型地震间的显著性检验统计值|Z|
（a） 板间地震与浅壳-上地幔地震之比；（b） 板间地震与板内地震之比
Figure 2. The variation of |Z| values versus spectral period for each pair of three types of earthquakes with four site classes
（a） The ratio of subduction interface earthquakes to shallow crustal and upper mantle earthquakes；（b） The ratio of subduction interface earthquakes and slab earthquakes
![]()
图 4 平滑前后模型系数随谱周期的分布图
（a） 平滑前常数项；（b） 平滑前场地效应项；（c） 平滑后常数项；（b） 平滑后场地效应项
Figure 4. Distribution of model coefficients versus spectral period before and after smoothing
（a） Constant term before smoothing；（b） Site effect terms before smoothing；（c） Constant term after smoothing；（d） Site effect terms after smoothing
![]()
图 5 预测H/V反应谱比随谱周期的变化
Figure 5. The variation of the predicted H/V spectral ratios with spectral periods
![]()
图 6 H/V反应谱比模型标准差随谱周期的分布图
Figure 6. Distribution of standard deviations for H/V response spectral ratio model，including between-event τ，within-event σ，and total standard deviations σT with spectral period
![]()
图 7 四类场地的场地间标准差τs （a）和场地内标准差σs （b）随谱周期的分布
Figure 7. The distribution of between-site standard deviations τs （a） and within-site standard deviations σs （b） with spectral period for four-class sites
![]()
图 8 谱周期T为0.15 s （上）和1.0 s （下）时事件间残差随MW （a，c）和震源深度（b，d）的分布图
Figure 8. Distribution of between-event residuals with MW （a，c） and source depth （b，d） for the spectral period T of 0.15 s （upper） and 1.0 s （lower）
![]()
图 9 四个谱周期T下Ⅰ类场地模型的场地内残差随断层距的分布图
Figure 9. Distribution of within-site residuals of Ⅰ -class site model with fault distance for four spectral periods T
（a） T＝0.15 s；（b） T＝0.5 s；（c） T＝1.0 s；（d） T＝5.0 s
表 1 场地分类标准与数据量
Table 1 Site classification criteria and number of records
场地类别 场地周期Ts /s 平均剪切波速vS30/（m·s−1） 记录数量 Ⅰ类（岩石） Ts＜0.2 vS30＞600 1 464 Ⅱ类（硬土） 0.2 ≤Ts＜0.4 300＜vS30≤600 764 Ⅲ类 （中硬土） 0.4 ≤Ts＜0.6 200＜vS30≤300 280 Ⅳ类（软土） Ts ≥0.6 vS30≤200 540 
下载: 导出CSV
表 2 俯冲带板间非设定地震H/V反应谱比模型平滑后的系数取值
Table 2 The smoothed coefficients of the H/V response spectral ratio model for non-scenario earthquakes from the subduction interface
T/s c S2 S3 S4 T/s c S2 S3 S4 PGA 0.813 0.086 −0.068 0.069 0.35 0.796 0.305 0.106 0.181 0.01 0.808 0.088 −0.066 0.069 0.40 0.735 0.298 0.194 0.209 0.02 0.784 0.095 −0.056 0.077 0.45 0.679 0.287 0.267 0.231 0.03 0.709 0.125 −0.024 0.102 0.50 0.637 0.278 0.310 0.249 0.04 0.545 0.156 0.011 0.134 0.60 0.578 0.263 0.352 0.276 0.05 0.524 0.102 −0.055 0.062 0.70 0.539 0.250 0.360 0.293 0.06 0.607 −0.016 −0.142 −0.051 0.80 0.512 0.239 0.355 0.305 0.07 0.697 −0.108 −0.201 −0.132 0.90 0.493 0.229 0.346 0.314 0.08 0.775 −0.170 −0.231 −0.160 1.00 0.479 0.220 0.336 0.319 0.09 0.832 −0.193 −0.246 −0.164 1.30 0.455 0.197 0.303 0.326 0.10 0.871 −0.181 −0.254 −0.145 1.50 0.446 0.183 0.281 0.326 0.12 0.925 −0.097 −0.258 −0.100 2.00 0.433 0.156 0.237 0.321 0.14 0.956 0 −0.249 −0.055 2.50 0.426 0.137 0.208 0.315 0.15 0.964 0.041 −0.241 −0.037 3.00 0.420 0.122 0.188 0.311 0.16 0.969 0.077 −0.230 −0.019 3.50 0.415 0.111 0.173 0.308 0.18 0.969 0.149 −0.204 0.013 4.00 0.411 0.101 0.162 0.308 0.20 0.961 0.201 −0.173 0.041 4.50 0.406 0.094 0.153 0.310 0.25 0.918 0.283 −0.084 0.099 5.00 0.402 0.088 0.144 0.315 0.30 0.859 0.305 0.012 0.145 注：表中参数为文中式（4）$ {{\mathrm{ln}}R}_{{\mathrm{HV}}}＝c ＋ {S}_{ k} ＋ {\xi }_{i\text{，} j} ＋ {\eta }_{i} $中的参数。
下载: 导出CSV
表 3 不同谱周期下各场地类别的模型标准差
Table 3 Standard deviations for models with different site class in different spectral periods
谱周期
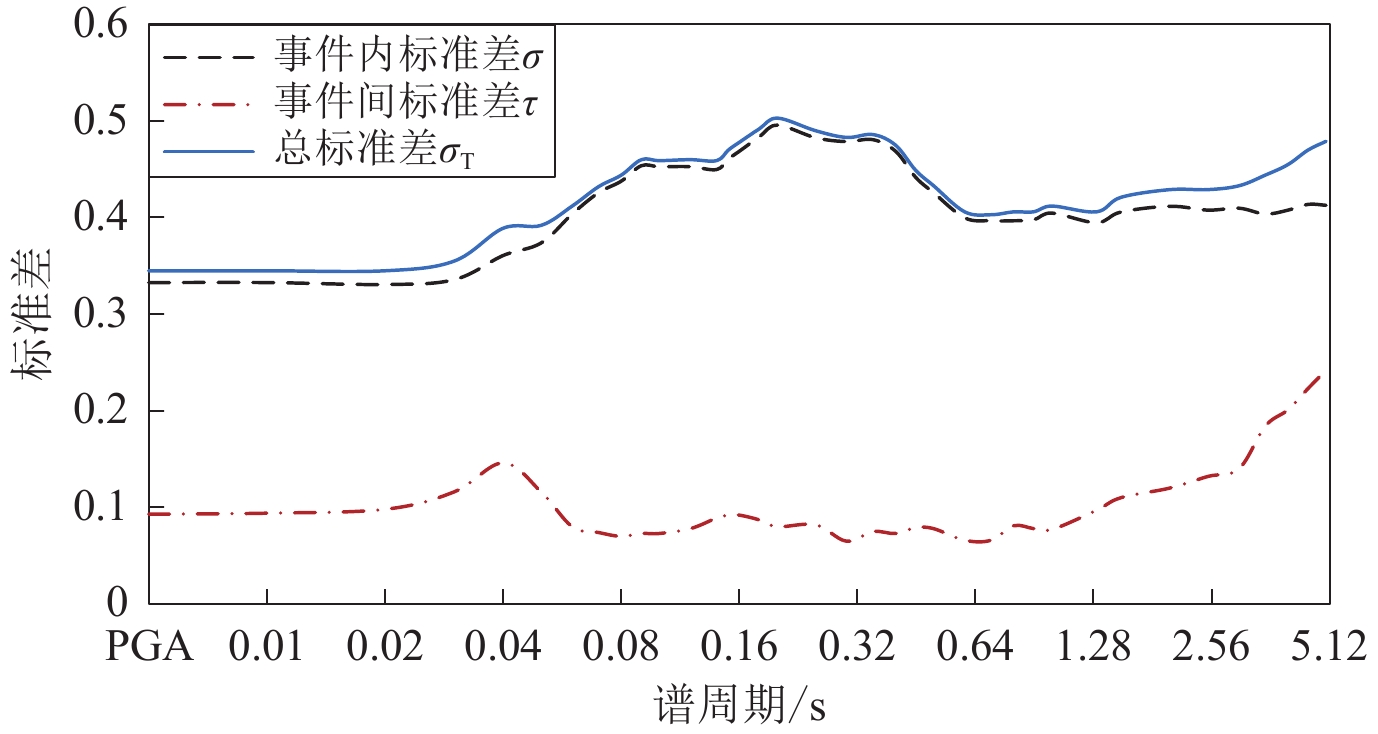
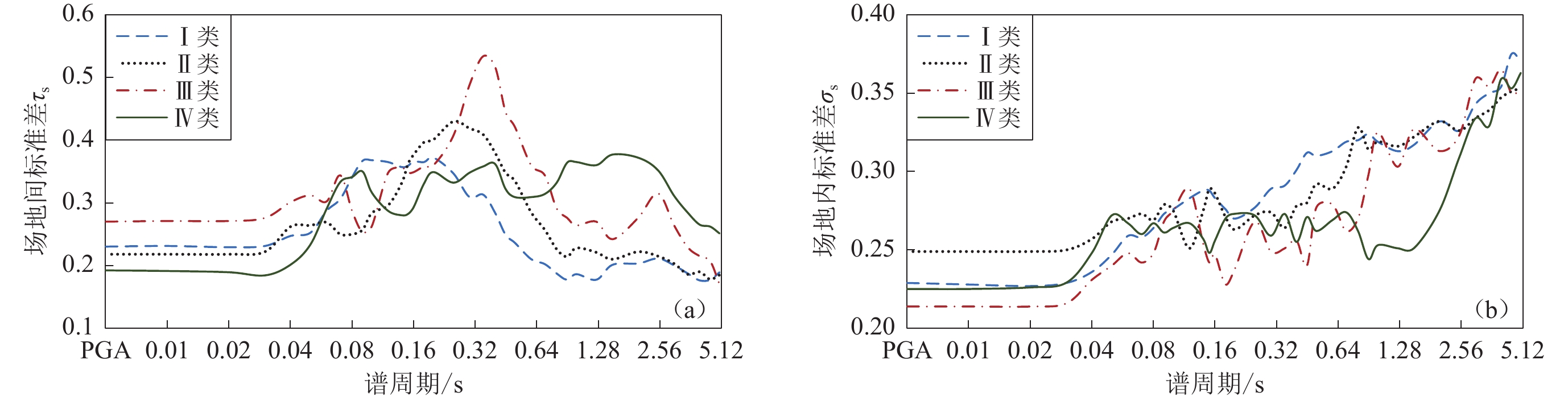
/sⅠ类场地 Ⅱ类场地 Ⅲ类场地 Ⅳ类场地 总体标准差 σs τs σST σs τs σST σs τs σST σs τs σST σ τ σT PGA 0.229 0.230 0.324 0.249 0.218 0.331 0.214 0.270 0.345 0.225 0.192 0.296 0.333 0.093 0.345 0.01 0.228 0.231 0.324 0.249 0.218 0.331 0.214 0.271 0.345 0.225 0.191 0.295 0.333 0.094 0.345 0.02 0.227 0.229 0.323 0.249 0.218 0.331 0.214 0.271 0.346 0.226 0.189 0.294 0.331 0.098 0.345 0.03 0.229 0.231 0.325 0.250 0.222 0.334 0.216 0.276 0.351 0.229 0.184 0.294 0.336 0.116 0.355 0.04 0.236 0.247 0.342 0.257 0.262 0.367 0.231 0.301 0.380 0.248 0.201 0.319 0.361 0.146 0.389 0.05 0.247 0.252 0.353 0.268 0.264 0.376 0.240 0.312 0.394 0.272 0.233 0.358 0.374 0.116 0.392 0.06 0.259 0.287 0.387 0.270 0.269 0.381 0.248 0.302 0.391 0.267 0.290 0.394 0.404 0.080 0.412 0.07 0.258 0.306 0.401 0.273 0.250 0.369 0.242 0.344 0.421 0.260 0.333 0.423 0.426 0.074 0.432 0.08 0.264 0.341 0.431 0.269 0.250 0.368 0.248 0.287 0.379 0.267 0.341 0.433 0.438 0.070 0.444 0.09 0.273 0.367 0.457 0.279 0.257 0.379 0.268 0.253 0.368 0.261 0.350 0.436 0.454 0.073 0.460 0.10 0.276 0.368 0.460 0.275 0.284 0.395 0.275 0.263 0.381 0.264 0.316 0.412 0.453 0.073 0.459 0.12 0.283 0.365 0.462 0.251 0.296 0.388 0.289 0.348 0.453 0.267 0.287 0.392 0.453 0.078 0.460 0.14 0.288 0.357 0.458 0.275 0.332 0.431 0.254 0.356 0.437 0.257 0.280 0.380 0.450 0.089 0.459 0.15 0.288 0.359 0.461 0.289 0.362 0.463 0.242 0.348 0.424 0.248 0.282 0.375 0.461 0.092 0.470 0.16 0.283 0.366 0.463 0.286 0.377 0.474 0.247 0.349 0.428 0.256 0.293 0.389 0.469 0.092 0.478 0.18 0.276 0.365 0.457 0.271 0.397 0.481 0.228 0.358 0.425 0.270 0.331 0.427 0.484 0.087 0.492 0.20 0.270 0.372 0.460 0.263 0.401 0.479 0.238 0.360 0.432 0.273 0.349 0.443 0.496 0.080 0.503 0.25 0.277 0.347 0.444 0.272 0.430 0.509 0.268 0.407 0.487 0.272 0.332 0.429 0.483 0.082 0.490 0.30 0.289 0.309 0.423 0.274 0.419 0.500 0.249 0.488 0.548 0.259 0.348 0.434 0.479 0.065 0.483 0.35 0.291 0.313 0.427 0.264 0.409 0.487 0.251 0.534 0.590 0.273 0.358 0.450 0.481 0.075 0.486 0.40 0.301 0.284 0.414 0.278 0.380 0.471 0.256 0.517 0.577 0.255 0.362 0.443 0.469 0.073 0.475 0.45 0.312 0.249 0.399 0.280 0.348 0.447 0.240 0.445 0.506 0.271 0.322 0.420 0.442 0.079 0.449 0.50 0.310 0.236 0.389 0.292 0.335 0.445 0.277 0.421 0.504 0.262 0.309 0.405 0.426 0.078 0.433 0.60 0.313 0.210 0.377 0.289 0.285 0.406 0.279 0.360 0.455 0.269 0.309 0.410 0.400 0.066 0.406 0.70 0.319 0.202 0.378 0.303 0.257 0.398 0.262 0.344 0.433 0.274 0.314 0.416 0.397 0.066 0.403 0.80 0.320 0.187 0.370 0.328 0.226 0.398 0.270 0.291 0.398 0.262 0.332 0.423 0.397 0.081 0.406 0.90 0.324 0.177 0.369 0.314 0.214 0.380 0.298 0.276 0.406 0.244 0.364 0.439 0.398 0.078 0.406 1.00 0.319 0.186 0.369 0.319 0.228 0.393 0.325 0.264 0.419 0.253 0.365 0.444 0.405 0.077 0.412 1.25 0.313 0.177 0.360 0.316 0.221 0.385 0.303 0.270 0.406 0.251 0.360 0.439 0.395 0.097 0.406 1.50 0.317 0.201 0.375 0.322 0.210 0.384 0.327 0.242 0.407 0.251 0.377 0.453 0.406 0.110 0.421 2.00 0.332 0.203 0.389 0.332 0.222 0.399 0.313 0.276 0.417 0.275 0.372 0.463 0.412 0.120 0.429 2.50 0.326 0.211 0.388 0.326 0.215 0.390 0.323 0.315 0.451 0.309 0.352 0.468 0.408 0.132 0.429 3.00 0.343 0.200 0.397 0.334 0.203 0.390 0.359 0.264 0.445 0.334 0.311 0.456 0.410 0.140 0.433 3.50 0.350 0.187 0.396 0.339 0.186 0.387 0.354 0.229 0.422 0.329 0.284 0.434 0.404 0.184 0.444 4.00 0.354 0.176 0.396 0.347 0.190 0.396 0.365 0.215 0.423 0.359 0.265 0.446 0.408 0.201 0.455 4.50 0.375 0.177 0.414 0.351 0.178 0.394 0.350 0.207 0.406 0.353 0.262 0.439 0.414 0.223 0.470 5.00 0.370 0.189 0.415 0.353 0.185 0.399 0.352 0.170 0.391 0.363 0.251 0.441 0.413 0.242 0.479 注：σs为场地内标准差，τs为场地间标准差，σST为总场地标准差，σ为事件内标准差，τ为事件间标准差，σT为总标准差。
下载: 导出CSV
-
薄景山,李琪,齐文浩,王玉婷,赵鑫龙,张毅毅. 2021. 场地条件对地震动和震害影响的研究进展与建议[J]. 吉林大学学报(地球科学版),51(5):1295–1305. Bo J S,Li Q,Qi W H,Wang Y T,Zhao X L,Zhang Y Y. 2021. Research progress and discussion of site condition effect on ground motion and earthquake damage[J]. Journal of Jilin University (Earth Science Edition),51(5):1295–1305 (in Chinese).
耿淑伟,陶夏新. 2004. 地震动加速度反应谱竖向分量与水平分量的比值[J]. 地震工程与工程振动,24(5):33–38. Geng S W,Tao X X. 2004. The ratios of vertical to horizontal acceleration response spectra[J]. Earthquake Engineering and Engineering Vibration,24(5):33–38 (in Chinese).
蒋飞. 2018. 日本俯冲带地区竖向地震动衰减关系研究[D]. 成都:西南交通大学:16−78. Jiang F. 2018. Ground-Motion Prediction Equations for the Vertical Component From Subduction Zone Earthquakes in Japan[D]. Chengdu:Southwest Jiaotong University:16−78 (in Chinese).
姜明秀,张潇男,康莉莉. 2019. 俯冲带板内地震水平加速度谱的阻尼比修正系数模型研究[J]. 地震工程与工程振动,39(4):126–138. Jiang M X,Zhang X N,Kang L L. 2019. A study on damping correction factor model for acceleration response spectrum for the horizontal components from subduction slab earthquakes[J]. Earthquake Engineering and Engineering Dynamics,39(4):126–138 (in Chinese).
李恒,秦小军. 2010. 竖向与水平向地震动加速度反应谱比特性分析[J]. 地震工程与工程振动,30(1):8–14. Li H,Qin X J. 2010. Study on characteristics of vertical to horizontal acceleration response spectral ratio[J]. Journal of Earthquake Engineering and Engineering Vibration,30(1):8–14 (in Chinese).
李小军,陈苏,任治坤,吕悦军,童华炜,温增平. 2020. 海域地震区划关键技术研究项目及研究进展[J]. 地震科学进展,50(1):2–19. Li X J,Chen S,Ren Z K,Lü Y J,Tong H W,Wen Z P. 2020. Project plan and research progress on key technologies of seismic zoning in sea areas[J]. Progress in Earthquake Sciences,50(1):2–19 (in Chinese).
石磐. 2017. 俯冲带地区的基岩反应谱及横竖向反应谱比研究[D]. 成都:西南交通大学:21−22. Shi P. 2017. A Study on the Rock Site Spectrum and its H/V Ratio of Earthquake in Subduction Zone[D]. Chengdu:Southwest Jiaotong University:21−22 (in Chinese).
谢俊举,温增平,高孟潭,胡聿贤,何少林. 2010. 2008年汶川地震近断层竖向与水平向地震动特征[J]. 地球物理学报,53(8):1796–1805. Xie J J,Wen Z P,Gao M T,Hu Y X,He S L. 2010. Characteristics of near-fault vertical and horizontal ground motion from the 2008 Wenchuan earthquake[J]. Chinese Journal of Geophysics,53(8):1796–1805 (in Chinese).
徐龙军,谢礼立. 2007. 竖向地震动加速度反应谱特性[J]. 地震工程与工程振动,27(6):17–23. Xu L J,Xie L L. 2007. Characteristics of acceleration response spectra for vertical ground motions[J]. Journal of Earthquake Engineering and Engineering Vibration,27(6):17–23 (in Chinese).
张齐. 2016. 地震动衰减关系的区域性差异研究[D]. 哈尔滨:中国地震局工程力学研究所:93−131. Zhang Q. 2016. Study on Regional Differentiation of Ground Motion Attenuation Relationship[D]. Harbin:Institute of Engineering Mechanics,China Earthquake Administration:93−131 (in Chinese).
中国建筑科学研究院. 2010. GB/T 50011—2010 建筑抗震设计标准[S]. 北京:中国建筑工业出版社:25. China Academy of Building Research. 2010. GB/T 50011−2010 Code for Seismic Design of Buildings[S]. Beijing:China Architecture & Building Press:25 (in Chinese).
周锡元,徐平,王国权,闫维明. 2006. 1999年台湾集集地震近断层竖向与水平反应谱比值的研究[J]. 地震地质,28(3):325–335. Zhou X Y,Xu P,Wang G Q,Yan W M. 2006. A case study on near fault vertical to horizontal response spectral ratio from the 1999,Chi-Chi,Taiwan,earthquake[J]. Seismology and Geology,28(3):325–335 (in Chinese).
周正华,周雍年,卢滔,杨程. 2003. 竖向地震动特征研究[J]. 地震工程与工程振动,23(3):25–29. Zhou Z H,Zhou Y N,Lu T,Yang C. 2003. Study on characteristics of vertical ground motion[J]. Earthquake Engineering and Engineering Vibration,23(3):25–29 (in Chinese).
Abrahamson N A,Youngs R R. 1992. A stable algorithm for regression analyses using the random effects model[J]. Bull Seismol Soc Am,82(1):505–510. doi: 10.1785/BSSA0820010505
Bozorgnia Y,Niazi M,Campbell K W. 1995. Characteristics of free-field vertical ground motion during the Northridge earthquake[J]. Earthq Spectra,11(4):515–525. doi: 10.1193/1.1585825
Bozorgnia Y,Campbell K W. 2004. The vertical-to-horizontal response spectral ratio and tentative procedures for developing simplified V/H and vertical design spectra[J]. J Earthq Eng,8(2):175–207.
Cultrera G,Cornou C,Di Giulio G,Bard P Y. 2021. Indicators for site characterization at seismic station:Recommendation from a dedicated survey[J]. Bull Earthq Eng,19(11):4171–4195. doi: 10.1007/s10518-021-01136-7
Xie J J,Li K W,Li X J,An Z,Wang P F. 2023. vS30-based relationship for Chinese site classification[J]. Eng Geol,324:107253. doi: 10.1016/j.enggeo.2023.107253
Zhao J X,Zhang J,Asano A,Ohno Y,Oouchi T,Takahashi T,Ogawa H,Irikura K,Thio H K,Somerville P G,Fukushima Y,Fukushima Y. 2006a. Attenuation relations of strong ground motion in Japan using site classification based on predominant period[J]. Bull Seismol Soc Am,96(3):898–913. doi: 10.1785/0120050122
Zhao J X,Irikura K,Zhang J,Fukushima Y,Somerville P G,Asano A,Ohno Y,Oouchi T,Takahashi T,Ogawa H. 2006b. An empirical site-classification method for strong-motion stations in Japan using H/V response spectral ratio[J]. Bull Seismol Soc Am,96(3):914–925. doi: 10.1785/0120050124
Zhao J X,Xu H. 2013. A comparison of vS30 and site period as site-effect parameters in response spectral ground-motion prediction equations[J]. Bull Seismol Soc Am,103(1):1–18. doi: 10.1785/0120110251
Zhao J X,Hu J S,Jiang F,Zhou J,Zhang Y B,An X W,Lu M,Rhoades D A. 2015a. Nonlinear site models derived from 1D analyses for ground-motion prediction equations using site class as the site parameter[J]. Bull Seismol Soc Am,105(4):2010–2022. doi: 10.1785/0120150019
Zhao J X,Zhou S L,Gao P J,Long T,Zhang Y B,Thio H K,Lu M,Rhoades D A. 2015b. An earthquake classification scheme adapted for Japan determined by the goodness of fit for ground-motion prediction equations[J]. Bull Seismol Soc Am,105(5):2750–2763. doi: 10.1785/0120150013
Zhao J X,Zhou S L,Zhou J,Zhao C,Zhang H,Zhang Y B,Gao P J,Lan X W,Rhoades D,Fukushima Y,Somerville P G,Irikura K. 2016a. Ground-motion prediction equations for shallow crustal and upper-mantle earthquakes in Japan using site class and simple geometric attenuation functions[J]. Bull Seismol Soc Am,106(4):1552–1569. doi: 10.1785/0120150063
Zhao J X,Liang X,Jiang F,Xing H,Zhu M,Hou R B,Zhang Y B,Lan X W,Rhoades D A,Irikura K,Fukushima Y,Somerville P G. 2016b. Ground-motion prediction equations for subduction interface earthquakes in Japan using site class and simple geometric attenuation functions[J]. Bull Seismol Soc Am,106(4):1518–1534. doi: 10.1785/0120150034
Zhao J X,Jiang F,Shi P,Xing H,Huang H F,Hou R B,Zhang Y B,Yu P C,Lan X W,Rhoades D A,Somerville P G,Irikura K,Fukushima Y. 2016c. Ground-motion prediction equations for subduction slab earthquakes in Japan using site class and simple geometric attenuation functions[J]. Bull Seismol Soc Am,106(4):1535–1551. doi: 10.1785/0120150056
Zhu C B,Pilz M,Cotton F. 2020. Which is a better proxy,site period or depth to bedrock,in modelling linear site response in addition to the average shear-wave velocity?[J]. Bull Earthq Eng,18(3):797–820. doi: 10.1007/s10518-019-00738-6
-
期刊类型引用(14)
1. 黄志强，付铭威，席御僖，李刚，王若豪，蒲伟. 基于拓扑优化方法的BV500型可控震源振动器平板激振性能改善研究. 机械强度. 2025(02): 75-84 . 
百度学术
2. Xinjuan He，Hua Pan. A modified stochastic finite-fault method for estimating strong ground motion:Validation and application. Earthquake Science. 2024(01): 36-50 . 必应学术
3. 范业昊，王宏伟，温瑞智，任叶飞. 利用经验格林函数谱比法估计2013年芦山地震序列的应力降. 地震学报. 2023(01): 98-106 . 本站查看
4. 李琴，蒲伟，黄志强，席御僖，李刚，王若豪. 川渝地区可控震源道路激振效果分析. 工程设计学报. 2022(06): 766-775 . 百度学术
5. 俞瑞芳，时洪涛，孙吉泽，张冬锋，俞言祥. 基于随机有限断层法的坝址地震动参数综合评价方法. 土木工程学报. 2020(07): 1-11+27 . 百度学术
6. 傅磊，李小军，陈苏. 基于经验参考场地的广义线性反演方法. 地震工程学报. 2019(05): 1290-1298 . 百度学术
7. 傅磊，李小军，荣棉水，陈苏，周越. 基于强震动数据的龙门山地区地震动预测模型参数反演. 地震学报. 2018(03): 374-386 . 本站查看
8. 孙晓丹，曾程，杨成，李永恒，常志旺. 基于有限断层随机地震动合成的高速路网地震危险性分析. 工程力学. 2018(04): 186-199 . 百度学术
9. 卢婷，苏金蓉，孙玮. 四川近震震级确定及地震波衰减特征的区域性差异. 大地测量与地球动力学. 2017(06): 655-660 . 百度学术
10. 王宏伟，任叶飞，温瑞智. 地震动震源、路径及场地效应分析的广义反演方法研究进展. 地球物理学进展. 2017(01): 78-86 . 百度学术
11. L.Fu，X.J.Li，傅磊. 龙门山及其相邻区域的剪切波高频衰减特性. 世界地震译丛. 2017(06): 524-541 . 百度学术
12. 姜治军，胡进军，张齐，谢礼立. 考虑土层非线性效应的四川地区场地放大系数模型. 岩土工程学报. 2016(09): 1650-1659 . 百度学术
13. 魏晓刚，麻凤海，刘书贤. 煤矿采空区的地震动力灾变及安全防控的研究进展与挑战. 地震研究. 2015(03): 495-507 . 百度学术
14. 温瑞智，王宏伟，任叶飞，冀昆. 芦山余震震源参数及震源区品质因子反演. 哈尔滨工业大学学报. 2015(04): 58-63 . 百度学术
其他类型引用(19)
计量
- 文章访问数: 145
- HTML全文浏览量: 25
- PDF下载量: 31
- 被引次数: 33
