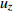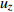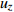Application of an optimized transmitting boundary with multiple artificial wave velocities in spectral-element simulation of seismic wave propagation
-
摘要: 将作者最近发展的多人工波速优化透射边界（记为ca j-MTF）应用于高精度谱元法的地震波动模拟中，并与经典的廖氏透射（MTF）边界、完美匹配层（PML）边界、黏弹性边界以及一阶旁轴近似边界进行了比较分析。结果显示：① ca j-MTF边界与MTF边界在形式上非常接近，它继承了后者公式简单、易于实现、精度可控、计算量低以及通用性好的优点；② 不同于传统MTF边界的单一人工波速参数配置，ca j-MTF边界所具有的多个人工波速参数可以分别取为P波和S波波速，此时边界计算波速与介质物理波速相匹配，能够大幅度提高复杂波动情形下的边界精度；③ ca j-MTF边界的精度略低于PML边界，不过要明显优于MTF边界、黏弹性边界以及一阶旁轴近似边界；④ ca j-MTF边界相比于PML边界的优势在于形式简洁且普遍适用。本研究为谱元法的地震波动模拟提供了一种便捷、高效的人工边界条件（即吸收边界条件）实现方法。Abstract: This paper applied an optimized transmitting boundary with multiple artificial velocities （denoted as caj-MTF） that is recently proposed by the authors to the high-accuracy spectral-element simulation of seismic wave propagation, and made a comparison study with several other classical artificial （or absorbing） boundary conditions including Liao’s multi-transmitting formula （MTF） boundary, perfectly matched layer （PML） boundary, viscous-spring boundary and the first-order Clayton-Engquist paraxial-approximation boundary. The results obtained from theoretical analysis and numerical tests are as follows: ① The formulation of ca j-MTF is very similar to that of MTF, so it has most of the advantages of the latter, i.e., very simple expressions, easy to be implemented, adjustable accuracy, minimal computation cost, and general applicability. ② Unlike the traditional MTF boundary that has only a single artificial wave velocity （i.e., computational wave velocity）, ca j-MTF has multiple artificial wave velocities. In the simulation of elastic waves, the computational wave velocity parameters of ca j-MTF can be set to be P- and S-wave velocities, respectively. On this situation, the consistency between computational and physical wave velocities makes a significant improvement in the boundary accuracy. ③ ca j-MTF boundary has an slightly lower accuracy than that of PML boundary, whereas it is significantly superior to MTF, viscous-spring boundary and the first-order paraxial-approximation boundary. ④ ca j-MTF is superior to PML as it has much simpler formulations and better versatility. This work provides a convenient and high-efficient artificial boundary （or absorbing boundary） for spectral-element simulation of seismic wave propagation.
-
-
![]()
图 1 多人工波速优化透射边界（
$c_{{\rm{a}}j}$ -MTF）示意图 （s0t为定义人工边界条件的局部坐标系）Figure 1. Schematic diagram of optimized transmitting boundary with multiple artificial wave velocities （
$c_{{\rm{a}}j}$ -MTF） （s0t is a local coordinate system that is used to define the artificial boundary condition）![]()
图 2 优化透射边界的谱元计算格式
Figure 2. Spectral-element computational scheme of the optimized transmitting boundary
![]()
图 3 人工边界性能分析模型
（a） 体波大角度透射问题；（b） 面波透射问题
Figure 3. Analytical models of artificial boundary performance
（a） The problem of body wave transmission over large incident angles；（b） Surface wave transmission problem
![]()
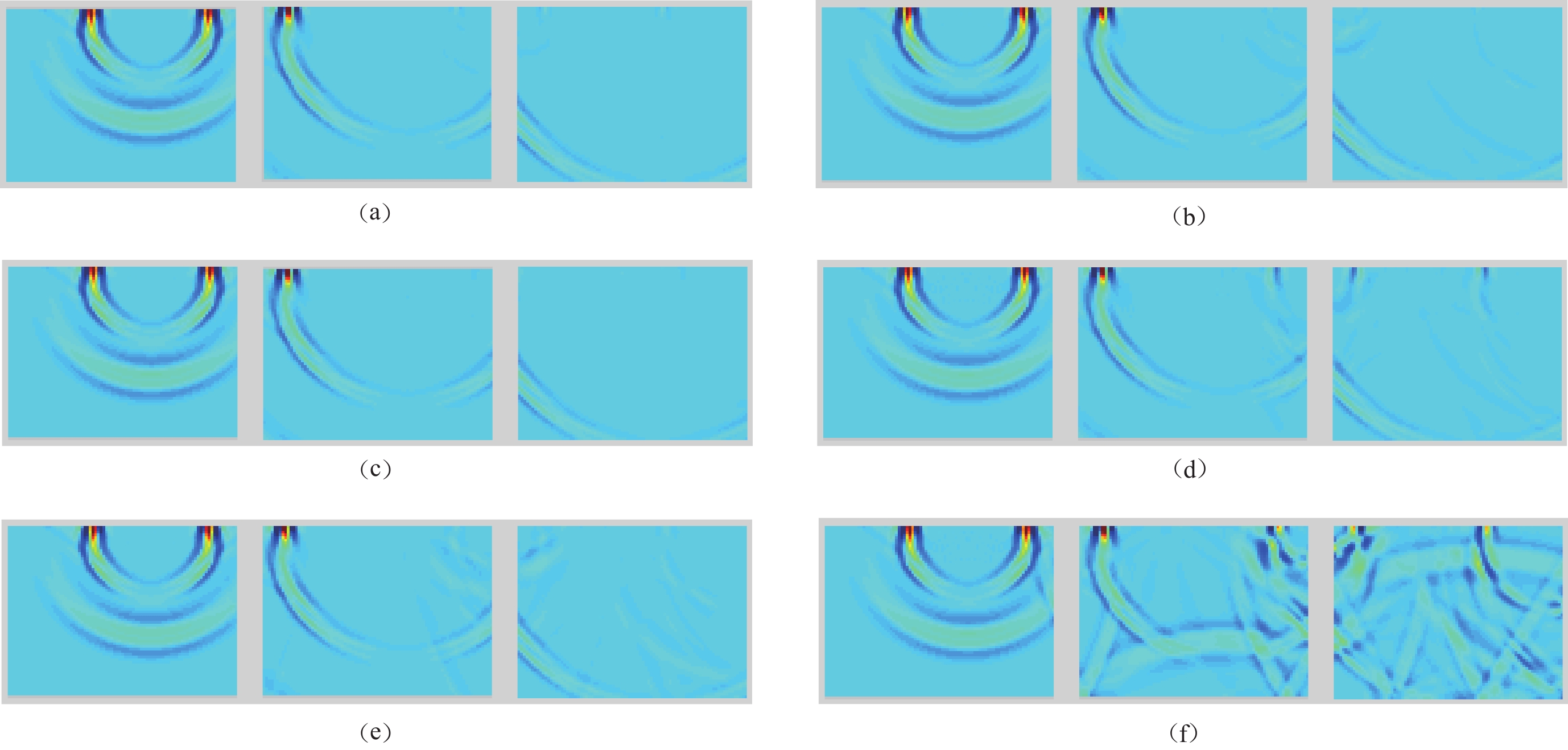
图 5 不同边界条件模拟体波大角度透射问题的波场快照（时刻依次为0.3 s，0.4 s，0.5 s，0.7 s）
（a） $c_{{\rm{a}}j} $-MTF边界；（b） MTF边界；（c） PML边界；（d） 黏弹性边界；（e） 一阶旁轴近似边界；（f） 自由边界（物理边界）
Figure 5. Wave field snapshots of body wave propagating over large incident angles obtained from different boundary conditions （time instants are 0.3 s，0.4 s，0.5 s，0.7 s）
（a） $c_{{\rm{a}}j} $-MTF boundary；（b） MTF boundary；（c） PML boundary；（d） Viscous-spring boundary；（e） The first-order paraxial-approximation boundary；（f） Free boundary （physical boundary）
![]()
图 6 观察点A （a），B （b）和C （c）处z方向位移
$u_z $ 的时程及误差曲线Figure 6. Time histories and error curves of the displacement
$u_z $ at the observation points A （a），B （b） and C （c）![]()
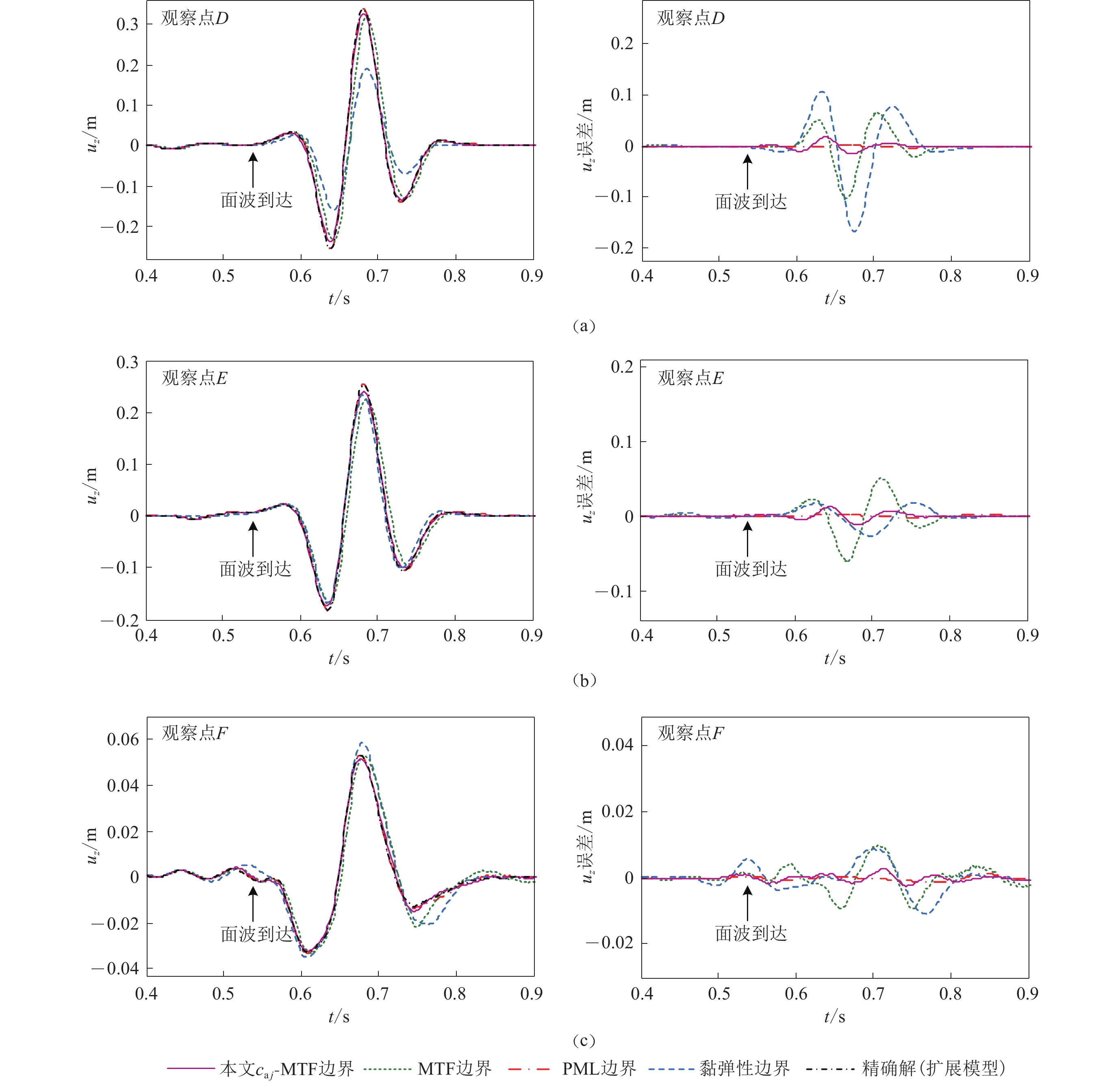
图 7 不同边界条件模拟面波问题的波场快照（时刻依次为0.5 s，0.9 s，1.2 s）
（a） $ c_{{\rm{a}}j} $-MTF边界；（b） MTF边界；（c） PML边界；（d） 黏弹性边界；（e） 一阶旁轴近似边界；（f） 自由边界（物理边界）
Figure 7. Wave field snapshots of surface waves obtained by different boundary conditions （time instants are 0.5 s，0.9 s，1.2 s）
（a） $ c_{{\rm{a}}j} $-MTF boundary；（b） MTF boundary；（c） PML boundary；（d） Viscous-spring boundary；（e） The first-order paraxial-approximation boundary；（f） Free boundary （physical boundary）
![]()
图 8 观察点D （a），E （b）和F （c）处z方向位移
$u_z $ 的时程及误差曲线Figure 8. Time history and error curves of the displacement
$u_z $ at the observation points D （a），E （b） and F （c）表 1 体波情形下A，B和C点误差曲线峰值（绝对值）以及每种边界条件下误差峰值与参考解峰值的百分比
Table 1 The peaks （absolute value） of error curves of the points A，B，C，and the percentages between those error peaks and reference solution peaks on different boundary conditions：Body wave case
边界条件 ux误差峰值/m uz误差峰值/m 误差峰值与参考
解峰值的百分比A点 B点 C点 A点 B点 C点 ca j-MTF边界 0.006 5 0.002 1 0.005 1 0.013 9 0.004 4 0.003 9 15.3% MTF边界 0.021 9 0.013 1 0.008 0 0.033 0 0.008 5 0.009 8 37.0% PML边界 0.001 0 0.000 9 0.001 5 0.001 5 0.001 1 0.000 6 3.2% 黏弹性边界 0.019 3 0.028 7 0.016 9 0.038 3 0.025 3 0.012 4 62.1% 一阶旁轴近似边界 0.022 3 0.004 0 0.010 0 0.008 3 0.011 0 0.012 9 33.7% 自由边界 0.058 5 0.036 4 0.016 2 0.097 6 0.076 4 0.013 7 119.2% 参考解峰值 0.062 6 0.045 8 0.021 1 0.075 3 0.028 2 0.021 3 − 
下载: 导出CSV
表 2 面波情形下D，E和F点误差曲线峰值（绝对值）以及每种边界条件下误差峰值与参考解峰值的百分比
Table 2 The peaks （absolute value） of error curves of the points D， E，F， and the percentages between those error peaks and reference solution peaks under different boundary conditions：Surface wave case
边界条件 ux 误差峰值/m uz误差峰值/m 误差峰值与参考
解峰值的百分比D点 E点 F点 D点 E点 F点 ca j-MTF边界 0.019 6 0.012 2 0.002 5 0.018 1 0.012 5 0.002 8 11.7% MTF边界 0.102 0 0.049 7 0.017 8 0.103 2 0.061 0 0.009 9 59.7% PML边界 0.005 0 0.002 5 0.002 5 0.002 8 0.002 3 0.001 4 4.8% 黏弹性边界 0.086 4 0.014 0 0.004 5 0.168 5 0.026 8 0.011 0 30.4% 一阶旁轴近似边界 0.075 4 0.034 8 0.009 8 0.069 9 0.059 6 0.009 6 41.0% 自由边界 0.552 3 0.196 5 0.204 9 0.447 7 0.153 4 0.154 9 425.4% 参考解峰值 0.233 3 0.039 8 0.015 3 0.336 7 0.252 2 0.053 1 −
下载: 导出CSV
-
陈少林,孙杰,柯小飞. 2020. 平面波输入下海水-海床-结构动力相互作用分析[J]. 力学学报,52(2):578–590. doi: 10.6052/0459-1879-19-354 Chen S L,Sun J,Ke X F. 2020. Analysis of water-seabed-structure dynamic interaction excited by plane waves[J]. Chinese Journal of Theoretical and Applied Mechanics,52(2):578–590 (in Chinese).
戴志军,李小军,侯春林. 2015. 谱元法与透射边界的配合使用及其稳定性研究[J]. 工程力学,32(11):40–50. doi: 10.6052/j.issn.1000-4750.2014.03.0196 Dai Z J,Li X J,Hou C L. 2015. A combination usage of transmitting formula and spectral element method and the study of its stability[J]. Engineering Mechanics,32(11):40–50 (in Chinese).
杜修力,赵密,王进廷. 2006. 近场波动模拟的人工应力边界条件[J]. 力学学报,38(1):49–56. doi: 10.3321/j.issn:0459-1879.2006.01.007 Du X L,Zhao M,Wang J T. 2006. A stress artificial boundary in FEA for near-field wave problem[J]. Chinese Journal of Theoretical and Applied Mechanics,38(1):49–56 (in Chinese).
景立平. 2004. 多次透射公式实用形式稳定性分析[J]. 地震工程与工程振动,24(4):20–24. doi: 10.3969/j.issn.1000-1301.2004.04.004 Jing L P. 2004. Stability analysis of practical formula for multi-transmitting boundary[J]. Earthquake Engineering and Engineering Vibration,24(4):20–24 (in Chinese).
李宁,谢礼立,翟长海. 2007. 基于混合有限元格式的完美匹配层与多次透射公式人工边界比较研究[J]. 地震学报,29(6):643–653. doi: 10.3321/j.issn:0253-3782.2007.06.009 Li N,Xie L L,Zhai C H. 2007. Comparison of perfectly matched layer and multi-transmitting formula artificial boundary conditions based on hybrid finite element formulation[J]. Acta Seismologica Sinica,29(6):643–653 (in Chinese).
李小军,廖振鹏. 1996. 时域局部透射边界的计算飘移失稳[J]. 力学学报,28(5):627–632. doi: 10.3321/j.issn:0459-1879.1996.05.016 Li X J,Liao Z P. 1996. The drift instability of local transmitting boundary in time domain[J]. Acta Mechanica Sinica,28(5):627–632 (in Chinese).
李小军,廖振鹏,关慧敏. 1995. 粘弹性场地地形对地震动影响分析的显式有限元-有限差分方法[J]. 地震学报,17(3):362–369. Li X J,Liao Z P,Guan H M. 1995. An explicit finite element-finite difference method for the analysis of the influence of viscous-elastic site topography on seismic wave motion[J]. Acta Seismologica Sinica,17(3):362–369 (in Chinese).
廖振鹏. 2002. 工程波动理论导论[M]. 第二版. 北京: 科学出版社: 156–181. Liao Z P. 2002. Introduction to Wave Motion Theories in Engineering[M]. 2nd ed. Beijing: Science Press: 156–181 （in Chinese）.
廖振鹏,李小军. 1995. 推广的多次透射边界:标量波情形[J]. 力学学报,27(1):69–78. Liao Z P,Li X J. 1995. Generalized multi-transmitting boundary:Scalar wave case[J]. Acta Mechanica Sinica,27(1):69–78 (in Chinese).
廖振鹏,黄孔亮,杨柏坡,袁一凡. 1984. 暂态波透射边界[J]. 中国科学:A辑,(6):556–564. Liao Z P,Huang K L,Yang B P,Yuan Y F. 1984. A transmitting boundary for transient wave analyses[J]. Science in China:Series A,27(10):1063–1076.
廖振鹏,周正华,张艳红. 2002. 波动数值模拟中透射边界的稳定实现[J]. 地球物理学报,45(4):533–545. doi: 10.3321/j.issn:0001-5733.2002.04.011 Liao Z P,Zhou Z H,Zhang Y H. 2002. Stable implementation of transmitting boundary in numerical simulation of wave motion[J]. Chinese Journal of Geophysics,45(4):533–545 (in Chinese).
刘晶波,李彬. 2005. 三维黏弹性静-动力统一人工边界[J]. 中国科学:E辑,35(9):966–980. Liu J B,Li B. 2005. A unified viscous-spring artificial boundary for 3-D static and dynamic applications[J]. Science in China:Series E,48(5):570–584. doi: 10.1360/04ye0362
谢志南,章旭斌. 2017. 弱形式时域完美匹配层[J]. 地球物理学报,60(10):3823–3831. doi: 10.6038/cjg20171012 Xie Z N,Zhang X B. 2017. Weak-form time-domain perfectly matched layer[J]. Chinese Journal of Geophysics,60(10):3823–3831 (in Chinese).
邢浩洁,李鸿晶. 2017a. 透射边界条件在波动谱元模拟中的实现:一维波动[J]. 力学学报,49(2):367–379. Xing H J,Li H J. 2017a. Implementation of multi-transmitting boundary condition for wave motion simulation by spectral element method:One dimension case[J]. Chinese Journal of Theoretical and Applied Mechanics,49(2):367–379 (in Chinese).
邢浩洁,李鸿晶. 2017b. 透射边界条件在波动谱元模拟中的实现:二维波动[J]. 力学学报,49(4):894–906. Xing H J,Li H J. 2017b. Implementation of multi-transmitting boundary condition for wave motion simulation by spectral element method:Two dimension case[J]. Chinese Journal of Theoretical and Applied Mechanics,49(4):894–906 (in Chinese).
邢浩洁,李小军,刘爱文,李鸿晶,周正华,陈苏. 2021. 波动数值模拟中的外推型人工边界条件[J]. 力学学报,53(5):1480–1495. doi: 10.6052/0459-1879-20-408 Xing H J,Li X J,Liu A W,Li H J,Zhou Z H,Chen S. 2021. Extrapolation-type artificial boundary conditions in the numerical simulation of wave motion[J]. Chinese Journal of Theoretical and Applied Mechanics,53(5):1480–1495 (in Chinese).
于彦彦,丁海平,刘启方. 2017. 透射边界与谱元法的结合及对波动模拟精度的改进[J]. 振动与冲击,36(2):13–22. Yu Y Y,Ding H P,Liu Q F. 2017. Integration of transmitting boundary and spectral-element method and improvement on the accuracy of wave motion simulation[J]. Journal of Vibration and Shock,36(2):13–22 (in Chinese).
章旭斌,廖振鹏,谢志南. 2015. 透射边界高频耦合失稳机理及稳定实现:SH波动[J]. 地球物理学报,58(10):3639–3648. doi: 10.6038/cjg20151017 Zhang X B,Liao Z P,Xie Z N. 2015. Mechanism of high frequency coupling instability and stable implementation for transmitting boundary:SH wave motion[J]. Chinese Journal of Geophysics,58(10):3639–3648 (in Chinese).
Clayton R,Engquist B. 1977. Absorbing boundary conditions for acoustic and elastic wave equations[J]. Bull Seismol Soc Am,67(6):1529–1540. doi: 10.1785/BSSA0670061529
Higdon R L. 1986. Absorbing boundary conditions for difference approximations to the multi-dimensional wave equation[J]. Math Comput,47(176):437–459.
Higdon R L. 1991. Absorbing boundary conditions for elastic waves[J]. Geophysics,56(2):231–241. doi: 10.1190/1.1443035
Huang J J. 2018. An incrementation-adaptive multi-transmitting boundary for seismic fracture analysis of concrete gravity dams[J]. Soil Dyn Earthq Eng,110:145–158. doi: 10.1016/j.soildyn.2017.12.002
Liao Z P. 1996. Extrapolation non-reflecting boundary conditions[J]. Wave Motion,24(2):117–138. doi: 10.1016/0165-2125(96)00010-8
Liao Z P,Wong H L. 1984. A transmitting boundary for the numerical simulation of elastic wave propagation[J]. Int J Soil Dyn Earthq Eng,3(4):174–183.
Liao Z P,Liu J B. 1992. Numerical instabilities of a local transmitting boundary[J]. Earthq Eng Struct Dyn,21(1):65–77. doi: 10.1002/eqe.4290210105
Liu Y S,Teng J W,Lan H Q,Si X,Ma X Y. 2014. A comparative study of finite element and spectral element methods in seismic wavefield modeling[J]. Geophysics,79(2):T91–T104. doi: 10.1190/geo2013-0018.1
Shi L,Wang P,Cai Y Q,Cao Z G. 2016. Multi-transmitting formula for finite element modeling of wave propagation in a saturated poroelastic medium[J]. Soil Dyn Earthq Eng,80:11–24. doi: 10.1016/j.soildyn.2015.09.021
Xie Z N,Zhang X B. 2017. Analysis of high-frequency local coupling instability induced by multi-transmitting formula:P-SV wave simulation in a 2D waveguide[J]. Earthq Eng Eng Vib,16(1):1–10. doi: 10.1007/s11803-017-0364-2
Xing H J,Li X J,Li H J,Liu A W. 2021a. Spectral-element formulation of multi-transmitting formula and its accuracy and stability in 1D and 2D seismic wave modeling[J]. Soil Dyn Earthq Eng,140:106218. doi: 10.1016/j.soildyn.2020.106218
Xing H J,Li X J,Li H J,Xie Z N,Chen S L,Zhou Z H. 2021b. The theory and new unified formulas of displacement-type local absorbing boundary conditions[J]. Bull Seismol Soc Am,111(2):801–824. doi: 10.1785/0120200155
Zeng C,Xia J H,Miller R D,Tsoflias G P. 2011. Application of the multiaxial perfectly matched layer (M-PML) to near-surface seismic modeling with Rayleigh waves[J]. Geophysics,76(3):T43–T52. doi: 10.1190/1.3560019
-
期刊类型引用(3)
1. 丁逸凡 ，吴立新 ，齐源 ，毛文飞 . 2022年6月阿富汗帕克提卡M6.0级地震前微波亮温异常的多态性及其归因辨析. 遥感学报. 2024(10): 2621-2631 . 
百度学术
2. 刘善军，纪美仪，宋丽美，魏恋欢. 基于改进两步差法的玛多M_S7.4地震微波异常研究. 地震学报. 2023(02): 328-340 . 本站查看
3. 吴立新，齐源，毛文飞，刘善军，丁逸凡，荆凤，申旭辉. 多波段多极化被动微波遥感地震应用研究进展与前沿方向探索. 测绘学报. 2022(07): 1356-1371 . 百度学术
其他类型引用(3)
计量
- 文章访问数: 394
- HTML全文浏览量: 161
- PDF下载量: 88
- 被引次数: 6
