The periodic and aperiodic slip during earthquake faulting and aseismic faulting slip: 1D fault model analysis
-
摘要: 本文首先根据Dieterich和Ruina提出的含速率和状态的摩擦定律(Dieterich-Ruina定律), 基于一维弹簧-滑块模型推导了地震复发周期的解析表达式, 然后将该近似解与数值模拟结果以及Barbot等的相关研究进行了对比分析. 此外, 本文还利用数值模拟与理论分析研究了断层周期和非周期演化的力学成因机制以及非地震滑移形成的另类力学机制, 并讨论了一维弹簧-滑块模型的优点及其局限性. 结果表明: ① 震后滑移和自加速/成核阶段的持续时间在整个演化过程中不能被忽略; ② 在修正后的复发周期模型中, 复发周期的长短除了与断层特征尺度、 作用于断层面上的有效正应力和远场加载速率相关外, 还受Dieterich-Ruina定律中摩擦参数的取值以及临界滑移距离的影响; ③ 当给定各个物理参数和几何参数时, 目前所得到的解析近似解可以很好地估计地震的复发周期, 其相对误差可小于5%; ④ 在断层演化过程中, 施加剪切应力加载会产生非周期的地震滑移, 而在自加速/成核阶段后期或震后滑移阶段早期, 施加较大的剪切应力加载, 则会出现非地震滑移.
-
关键词:
- 含速率和状态的摩擦定律 /
- 地震循环 /
- 地震复发周期 /
- 非地震滑移
Abstract: With a simple 1D spring-slide block model governed by Dieterich-Ruina law, this study derived an approximate analytical solution used to calculate the earthquake recurrence time and compared its approximate solution with previous results given by Barbot et al. The comparison between approximate solutions and the results by numerical simulation also demonstrated that current solution gives us a better approximation compared with previous result. Furthermore, it should be emphasized that, because only 40% to 80% of earthquake recurrence time belongs to interseismic stage, therefore, the duration of postseismic and nucleation/preseismic stage could not be ignored in the whole faulting process. Specifically, the time scale of earthquake recurrence in current earthquake recurrence model is not only related to the characteristic dimension of fault, the effective normal stress and remote loading rate, but also strongly depends on the values of the frictional parameters in the Dieterich-Ruina law and the critical distance obtained from laboratory. It is necessary to point out that, if all parameters we used represent a real situation in the earth, the approximate solution proposed in the present paper can give us an excellent estimation of earthquake recurrence time for a given tectonic region, of which relative error is less than 5%. Moreover, numerical simulation and theory are also used to study the mechanical mechanisms which cause the periodic and aperiodic evolution of the earthquake faulting and aseismic faulting slip. In addition, this paper also discussed the limitations and advantages of using 1D spring-slide block model to describe the elasticity in modeling evolution of fault. And it is found that applying shear stress loading during evolution of earthquake faulting can cause aperiodic evolution. Specially, applying shear stress loading at late nucleation/preseismic stage or early postseismic stage will lead to aseismic faulting slip. -
-
图 1 地震复发模型示意图(引自Shimazaki,Nakata,1980)
(a)周期模型;(b)时间可预测模型;(c)滑动可预测模型τ1和τ2分别为地震发生后和地震发生前的应力,均为常数(虚线),Sc为累积同震位移
Figure 1. Schematic diagram of earthquake recurrence models(after Shimazaki,Nakata,1980)
(a)Strictly periodic model;(b)Time-predictable model;(c)Slip-predictable model τ1 and τ2 are stresses after and before an earthquake,respectively,and they are constants denoted by dashed lines. Sc represents the cumulative coseismic slip
图 2 库仑模型断层失稳示意图
(a)静态应力扰动引起的断层失稳(修改自仲秋,史保平,2012);(b)断层摩擦强度降低引起的断层失稳虚线为演化状态变化后的断层演化图,Δt为断层演化状态变化后第一次失稳发生时间的提前量
Figure 2. Schematic diagram of the fault instability in Coulomb failure model
(a)Fault instability caused by applying static stress perturbation(modified from Zhong,Shi,2012);(b)Fault instability caused by lowering the fault frictional strength. Dashed lines represent the fault evolution after changing state. Δt represents the time advance when the first instability occurs after changing the evolution state of fault
图 3 一维弹簧-滑块模型
Figure 3. 1D spring-slide block model
τ and σ represent shear and normal stress on the fault plane,respectively; δ is the displacement of the block along fault plane; vpl is the remote loading rate; δpl is the remote loading displacement; k is the effective stiffness coefficient of spring
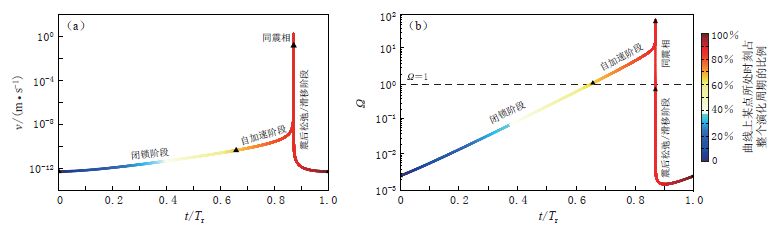
图 4 基于一维断层模型的滑移速率周期演化图(a)和相平面内的闭合轨迹图(b)
Tr为断层模型的完整演化周期,黑色三角形为各个阶段的分界标志,下同
Figure 4. Periodic evolution of slip rate based on 1D fault model(a)and the closed trajectories in phase plane(b)
Tr represents a complete earthquake recurrence time. The colour represents the time percentage of the cycle period at each point,the black triangles are the boundary between different stages,the same below
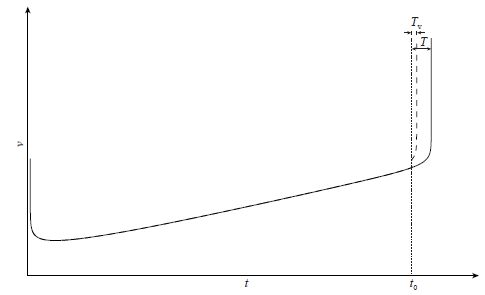
图 8 施加和未施加正向静态剪切应力扰动时的滑移速率演化示意图
实线为未扰动时的演化,虚线为存在扰动时的演化,t0为施加静态剪切应力扰动的时刻,T和Tv分别为未扰动时和扰动后从t0时刻至断层模型失稳所需的时间
Figure 8. Schematic diagram of the time evolution of slip rate with and without positive static stress perturbation
The solid and dashed lines represent the unperturbed and perturbed evolution,respectively. t0 is the onset time of the static shear stress perturbation. T and Tv represent the time from t0 to unperturbed failure time and the time from t0 to perturbed failure time,respectively
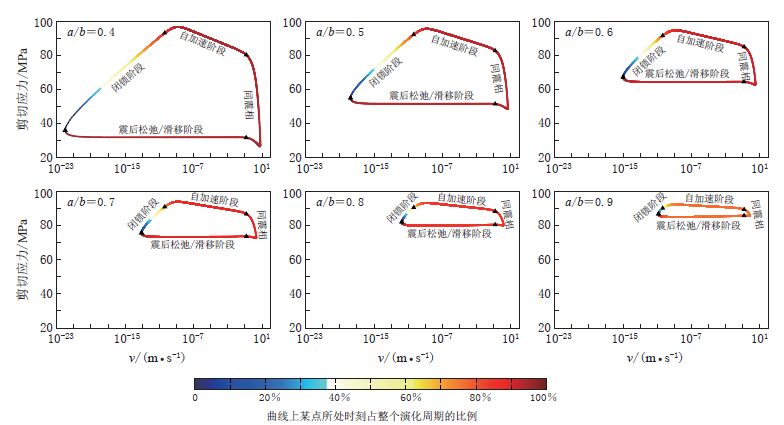
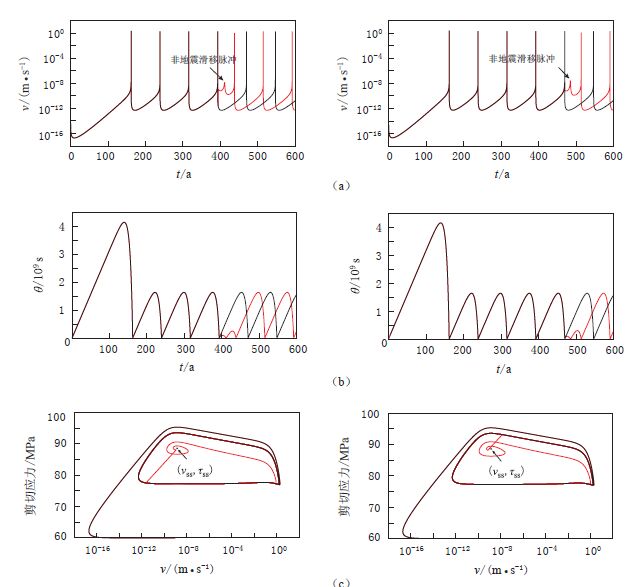
图 9 正向(左)和负向(右)静态剪切应力加载产生的非地震滑移
(a)滑移速率v演化图;(b)状态变量θ演化图;(c)断层面上剪切应力τ和滑移速率v的相图黑线和红线分别为未受应力扰动和受应力扰动时一维弹簧-滑块模型的演化情况,τss和vss分别为稳定状态下断层面上的剪切应力和滑移速率
Figure 9. Aseismic slip produced by a positive(left)and negative(right)static shear stress loading
(a)Evolution of slip rate v;(b)Evolution of state variable θ;(c)The plots of the closed trajectory(cycle)of shear stress τ and slip rate v in the phase plane. The black and red lines represent the evolution of 1D spring-slide block system on unperturbed and perturbed stress loading conditions,respectively. τss and vss represent the shear stress and slip rate,respectively,when the system is at steady state
表 1 模拟计算中使用的参数值(引自Kame et al,2013b)
Table 1 Parameters used for simulation(after Kame et al,2013b)
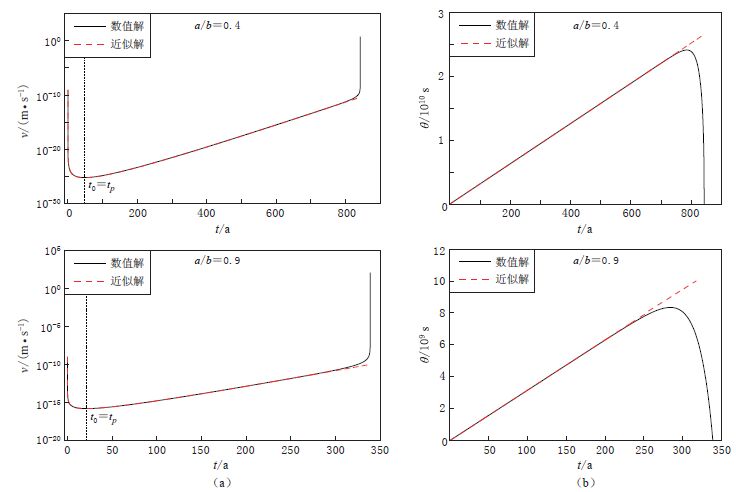
a b σ/MPa Dc/m k/kc v(0)/(m·s-1) vpl/(m·s-1) vo/(m·s-1) vco/(m·s-1) μ(0) μo ζ/(MPa·s·m-1) 0.017 0.0225 100 0.062 0.57 9.33×10-10 1.4×10-9 10-10 0.14 0.6 0.9 5 注: 状态变量初始值θ(0)由v(0)和μ(0)求得. 表 2 不同a/b值情况下近似解与数值解的对比
Table 2 Comparison of analytic approximate solutions with numerical solutions for different a/b values
a/b Tpost /a Tint /a Tpre /a Tco /s Tr /a ta /a 2πta /a 数值解 近似解 数值解 近似解 数值解 近似解 数值解 近似解 0.4 3.905 4.106 55.093 54.019 5.062 4.775 1.28 64.060 62.901 1.642 10.320 0.5 4.914 4.927 53.876 54.019 7.033 6.550 1.61 65.824 65.496 2.464 15.480 0.6 6.191 6.159 52.758 54.019 9.639 8.806 2.04 68.588 68.984 3.696 23.220 0.7 8.231 8.212 51.478 54.019 13.391 11.859 2.62 73.099 74.091 5.749 36.119 0.8 12.281 12.318 49.544 54.019 19.638 16.407 3.60 81.463 82.744 9.855 61.919 0.9 23.563 24.637 44.473 54.019 34.237 24.456 5.71 102.273 103.112 22.173 139.317 表 3 不同D′c/Dc值情况下近似解与数值解的对比
Table 3 Comparison of analytic approximate solutions with numerical solutions for different D′c/Dc values

Tpost /a Tint /a Tpre /a Tco /s Tr /a ta /a 2πta /a 数值解 近似解 数值解 近似解 数值解 近似解 数值解 近似解 10 100.925 100.786 505.352 540.192 163.818 141.255 31.18 770.094 782.233 76.150 478.463 5 50.269 50.393 252.878 270.096 81.900 70.627 15.49 385.047 391.117 38.075 239.232 2 20.194 20.157 101.064 108.038 32.761 28.251 6.20 154.019 156.447 15.230 95.693 1 10.059 10.079 50.572 54.019 16.378 14.126 3.11 77.009 78.223 7.615 47.846 0.5 5.033 5.039 25.276 27.010 8.195 7.063 1.55 38.505 39.112 3.808 23.923 0.2 2.012 2.016 10.114 10.804 3.276 2.825 0.62 15.402 15.645 1.523 9.569 0.1 1.005 1.008 5.057 5.402 1.638 1.413 0.31 7.701 7.822 0.762 4.785 注: Dc和D′c分别为表 1中给定的临界滑动距离和模拟计算时的临界滑动距离; Tint,Tpre,Tco和Tpost分别为断层演化过程中闭锁阶段、 自加速/成核阶段、 同震相和震后松弛/滑移阶段的持续时间; a和b为Dieterich-Ruina定律中的参数; ta为特征时间. -
解孟雨, 史保平. 2016. 数值模拟静态应力扰动下的断层失稳: 结果分析兼与Dieterich模型和Coulomb模型的对比[J]. 地球物理学报, 59(2): 593-605. http://www.cnki.com.cn/Article/CJFDTOTAL-DQWX201602018.htm Xie M Y, Shi B P. 2016. Numerical simulation of fault instability due to an arbitrary static stress perturbation: A comparison with the Dieterich model and Coulomb failure model[J]. Chinese Journal of Geophysics, 59(2): 593-605 (in Chinese). http://www.cnki.com.cn/Article/CJFDTOTAL-DQWX201602018.htm
仲秋, 史保平. 2012. 1976年MS7.8唐山地震余震序列持续时间及对地震危险性分析的意义[J]. 地震学报, 34(4): 494-508. http://www.dzxb.org/Magazine/Show?id=28774 Zhong Q, Shi B P. 2012. Aftershock time duration of the 1976 MS7.8 Tangshan earthquake and implication for seismic hazard[J]. Acta Seismologica Sinica, 34(4): 494-508 (in Chinese). http://www.dzxb.org/Magazine/Show?id=28774
Avouac J-P. 2015. From geodetic imaging of seismic and aseismic fault slip to dynamic modeling of the seismic cycle[J]. Annu Rev Earth Planet Sci, 43(1): 233-271. doi: 10.1146/annurev-earth-060614-105302
Barbot S, Lapusta N, Avouac J-P. 2012. Under the hood of the earthquake machine: Toward predictive modeling of the seismic cycle[J]. Science, 336(6082): 707-710. doi: 10.1126/science.1218796
Beeler N M, Lockner D A. 2003. Why earthquakes correlate weakly with the solid earth tides: Effects of periodic stress on the rate and probability of earthquake occurrence[J]. J Geophys Res, 108(B8): 2391. doi: 10.1029/2001JB001518
Beeler N M. 2004. Review of the physical basis of laboratory-derived relations for brittle failure and their implications for earthquake occurrence and earthquake nucleation[J]. Pure Appl Geophys, 161(9/10): 1853-1876. http://cn.bing.com/academic/profile?id=2008445517&encoded=0&v=paper_preview&mkt=zh-cn
Belardinelli M E, Bizzarri A, Cocco M. 2003. Earthquake triggering by static and dynamic stress changes[J]. J Geophys Res, 108(B3): 2135. http://cn.bing.com/academic/profile?id=2085003380&encoded=0&v=paper_preview&mkt=zh-cn
Bhattacharya P, Rubin A M. 2014. Frictional response to velocity steps and 1-D fault nucleation under a state evolution law with stressing-rate dependence[J]. J Geophys Res, 119(3): 2272-2304. doi: 10.1002/2013JB010671
Byerlee J. 1970. The mechanics of stick-slip[J]. Tectonophysics, 9(5): 475-486. doi: 10.1016/0040-1951(70)90059-4
Byerlee J. 1978. Friction of rocks[J]. Pure Appl Geophys, 116(4/5): 615-626. http://cn.bing.com/academic/profile?id=1977156689&encoded=0&v=paper_preview&mkt=zh-cn
Dieterich J H. 1978. Time-dependent friction and the mechanics of stick-slip[J]. Pure Appl Geophys, 116(4/5): 790-806. http://cn.bing.com/academic/profile?id=2128375302&encoded=0&v=paper_preview&mkt=zh-cn
Dieterich J H. 1979. Modelling of rock friction: 1. Experimental results and constitutive equations[J]. J Geophys Res, 84(B5): 2161-2168. http://cn.bing.com/academic/profile?id=2019935798&encoded=0&v=paper_preview&mkt=zh-cn
Dieterich J H. 1981. Constitutive properties of faults with simulated gouge[C]//Mechanical Behavior of Crustal Rocks: The Handin Volume. Washington DC: American Geophysical Union: 103-120.
Dieterich J H. 1992. Earthquake nucleation on faults with rate- and state-dependent strength[J]. Tectonophysics, 211(1/2/3/4): 115-134. http://cn.bing.com/academic/profile?id=2093509339&encoded=0&v=paper_preview&mkt=zh-cn
Dieterich J H. 1994. A constitutive law for rate of earthquake production and its application to earthquake clustering[J]. J Geophys Res, 99(B2): 2601-2618. doi: 10.1029/93JB02581
Gomberg J, Blanpied M L, Beeler N M. 1997. Transient triggering of near and distant earthquakes[J]. Bull Seismol Soc Am, 87(2): 294-309. http://cn.bing.com/academic/profile?id=1894687224&encoded=0&v=paper_preview&mkt=zh-cn
Gomberg J, Beeler N M, Blanpied M L, Bodin P.1998. Earthquake triggering by transient and static deformation[J]. J Geophys Res, 103(B10): 24411-24426. doi: 10.1029/98JB01125
Gu J C, Rice J R, Ruina A L, Tse S T.1984. Slip motion and stability of a single degree of freedom elastic system with rate and state dependent friction[J]. J Mech Phys Solids, 32(3): 167-196. doi: 10.1016/0022-5096(84)90007-3
Harris R A, Simpson R W. 1998. Suppression of large earthquakes by stress shadows: A comparison of Coulomb and rate-and-state failure[J]. J Geophys Res, 1032(B10): 24439-24451. http://cn.bing.com/academic/profile?id=2002797922&encoded=0&v=paper_preview&mkt=zh-cn
Kame N, Fujita S, Nakatani M, Kusakabe T. 2013a. Effects of a revised rate- and state-dependent friction law on aftershock triggering model[J]. Tectonophysics, 600: 187-195. doi: 10.1016/j.tecto.2012.11.028
Kame N, Fujita S, Nakatani M, Kusakabe T.2013b. Earthquake cycle simulation with a revised rate- and state-dependent friction law[J]. Tectonophysics, 600 : 196-204. doi: 10.1016/j.tecto.2012.11.029
Kaneko Y, Lapusta N. 2008. Variability of earthquake nucleation in continuum models of rate-and-state faults and implications for aftershock rates[J]. J Geophys Res, 113(B12): B12312. doi: 10.1029/2007JB005154
Kaneko Y, Avouac J-P, Lapusta N. 2010. Towards inferring earthquake patterns from geodetic observations of interseismic coupling[J]. Nat Geosci, 3(5): 363-369. doi: 10.1038/ngeo843
Liu M, Stein S, Wang H. 2012. 2000 years of migrating earthquakes in North China: How earthquakes in midcontinents differ from those at plate boundaries[J]. Lithosphere, 22(2): 33-44. http://cn.bing.com/academic/profile?id=2317751205&encoded=0&v=paper_preview&mkt=zh-cn
Marone C. 1998. Laboratory-derived friction laws and their application to seismic faulting[J]. Annu Rev Earth Planet Sci, 26(1): 643-696. doi: 10.1146/annurev.earth.26.1.643
Nagata K, Nakatani M, Yoshida S. 2012. A revised rate- and state-dependent friction law obtained by constraining constitutive and evolution laws separately with laboratory data[J]. J Geophys Res, 117(B2): B02314. http://cn.bing.com/academic/profile?id=2025142380&encoded=0&v=paper_preview&mkt=zh-cn
Noda H, Lapusta N. 2013. Stable creeping fault segments can become destructive as a result of dynamic weakening[J]. Nature, 493(7433): 518-521. doi: 10.1038/nature11703
Parsons T. 2005. A hypothesis for delayed dynamic earthquake triggering[J]. Geophys Res Lett, 32(4): L04302. http://cn.bing.com/academic/profile?id=1992339695&encoded=0&v=paper_preview&mkt=zh-cn
Perfettini H, Schmittbuhl J, Cochard A. 2003. Shear and normal load perturbations on a two-dimensional continuous fault: 1. Static triggering[J]. J Geophys Res, 108(B9): 2408. http://cn.bing.com/academic/profile?id=2008664307&encoded=0&v=paper_preview&mkt=zh-cn
Perfettini H, Avouac J-P. 2004a. Postseismic relaxation driven by brittle creep: A possible mechanism to reconcile geodetic measurements and the decay rate of aftershocks, application to the Chi-Chi earthquake, Taiwan[J]. J Geophys Res, 109(B2): B02304. http://cn.bing.com/academic/profile?id=2065169229&encoded=0&v=paper_preview&mkt=zh-cn
Perfettini H, Avouac J-P. 2004b. Stress transfer and strain rate variations during the seismic cycle[J]. J Geophys Res, 109(6): 117-132. http://cn.bing.com/academic/profile?id=2059866814&encoded=0&v=paper_preview&mkt=zh-cn
Perfettini H, Avouac J-P, Tavera H, Kositsky A, Nocquet J-M, Bondoux F, Chlieh M, Sladen A, Audin L, Farber D L, Soler P. 2010. Seismic and aseismic slip on the Central Peru megathrust[J]. Nature, 465(7294): 78-81. doi: 10.1038/nature09062
Ranjith K, Rice J R. 1999. Stability of quasi-static slip in a single degree of freedom elastic system with rate and state dependent friction[J]. J Mech Phys Sol, 47(6): 1207-1218. doi: 10.1016/S0022-5096(98)00113-6
Rice J R. 1983. Constitutive relations for fault slip and earthquake instabilities[J]. Pure Appl Geophys, 121(3): 443-475. doi: 10.1007/BF02590151
Rubin A M, Ampuero J-P. 2005. Earthquake nucleation on (aging) rate and state faults[J]. J Geophys Res, 110(B11): B11312. http://cn.bing.com/academic/profile?id=2025058161&encoded=0&v=paper_preview&mkt=zh-cn
Ruina A. 1983. Slip instability and state variable friction laws[J]. J Geophys Res, 88(B12): 10359-10370. doi: 10.1029/JB088iB12p10359
Ryder I. 2006. Elastic and Viscoelastic Modelling of Postseismic Motion and Fault Structures[D]. Oxford: Oxford University: 12. doi: 10.1007/978-3-319-32580-4_1/fulltext.html
Scholz C H. 1998. Earthquakes and friction laws[J]. Nature, 391(6662): 37-42. doi: 10.1038/34097
Scholz C H. 2002. The Mechanics of Earthquakes and Faulting[M]. New York: Cambridge University Press: 85, 266-267.
Segall P. 2010. Earthquake and Volcano Deformation[M]. Princeton: Princeton University Press: 332, 338-339, 349-350.
Shimazaki K, Nakata T. 1980. Time-predictable recurrence model for large earthquake[J]. Geophys Res Lett, 7(4): 279-282. doi: 10.1029/GL007i004p00279
Shirzaei M, Burgmann R, Uchida N, Pollitz F, Matsuzawa T. 2014. Seismic versus aseismic slip: Probing mechanical properties of the northeast Japan subduction zone[J]. Earth Planet Sci Lett, 406: 7-13. doi: 10.1016/j.epsl.2014.08.035
Stein R S. 1999. The role of stress transfer in earthquake occurrence[J]. Nature, 402(6762): 605-609. doi: 10.1038/45144
Stein S, Wysession M. 2003. An Introduction to Seismology, Earthquakes, and Earth Structure[M]. Malden: Blackwell Publishing: 215-217.
Stein S, Liu M. 2009. Long aftershock sequences within continents and implications for earthquake hazard assessment[J]. Nature, 462(7269): 87-89. doi: 10.1038/nature08502
Uchida N, Iinuma T, Nadeau R M, Burgmann R, Hino R. 2016. Periodic slow slip triggers megathrust zone earthquakes in northeastern Japan[J]. Science, 351(6272): 488-492. doi: 10.1126/science.aad3108
-
期刊类型引用(3)
1. 杨横涛,白冰,林杭. 基于速率状态摩擦定律的地震震级计算(英文). Journal of Central South University. 2023(08): 2671-2685 .  百度学术
百度学术
2. 王璞,王成虎,武志德,孙军昌,贾晋,侯正阳,杨汝华. 基于案例分析的库仑应力变化触发地震机理探索. 地震工程学报. 2019(04): 1006-1016+1052 .  百度学术
百度学术
3. 申文豪,薛腾飞,张景发,杨建钦. 基于速率-状态摩擦定律的地震二维准动力学数值模拟:浅层正应力变化对断层演化的影响. 地球物理学报. 2018(08): 3198-3210 .  百度学术
百度学术
其他类型引用(2)





 下载:
下载: